Essay Five: Why Motion Isn't A Contradiction
Visitors are encouraged to read this Essay in conjunction with Essay Four Part One and Essay Eight Parts One, Two and Three.
Internet Explorer 11 will no longer play the videos I have posted to this page. As far as I can tell, they play as intended in other Browsers. However, if you have Privacy Badger [PB] installed, they won't play in Google Chrome unless you disable PB for this site. They play in IE11 if you have upgraded to Windows 10! It looks like the problem is with Windows 7 and earlier versions of that operating system.
If you are using Internet Explorer 10 (or later), you might find some of the links I have used won't work properly unless you switch to 'Compatibility View' (in the Tools Menu); for IE11 select 'Compatibility View Settings' and add this site (anti-dialectics.co.uk). Microsoft's browser, Edge, automatically renders these links compatible; Windows 10 does likewise. I don't yet know if that is the case with Windows 11.
However, if you are using Windows 10, IE11 and Edge unfortunately appear to colour these links somewhat erratically. They are meant to be mid-blue, but those two browsers render them intermittently light blue, yellow, purple and red!
Firefox and Chrome reproduce them correctly.
Several browsers also appear to underline these links erratically. Many are underscored boldly in black, others more lightly in blue! They are all meant to be the latter.
Finally, if you are viewing this with Firefox, you might not be able to read all the symbols I have used; that browser often replaces them with a "º". Chrome and Edge reproduce them correctly; I don't know if that is the case with other browsers.
As is the case with all my Essays, nothing here should be read as an attack either on Historical Materialism [HM] -- a scientific theory I fully accept --, or, indeed, revolutionary socialism. I remain as committed to the self-emancipation of the working class and the dictatorship of the proletariat as I was when I first became a revolutionary over thirty-five years ago.
The difference between Dialectical Materialism [DM] and HM, as I see it, is explained here.
Several readers have complained about the number of links I have added to these Essays because they say it makes them very difficult to read. Of course, DM-supporters can hardly lodge that complaint since they believe everything is interconnected, and that must surely apply even to Essays that attempt to debunk that very idea. However, to those who find such links do make these Essays difficult to read I say this: ignore them -- unless you want to access further supporting evidence and argument for a particular point, or a certain topic fires your interest.
Others wonder why I have linked to familiar subjects and issues that are part of common knowledge (such as the names of recent Presidents of the USA, UK Prime Ministers, the names of rivers and mountains, the titles of popular films, or certain words that are in common usage). I have done so for the following reason: my Essays are read all over the world and by people from all 'walks of life', so I can't assume that topics which are part of common knowledge in 'the west' are equally well-known across the planet -- or, indeed, by those who haven't had the benefit of the sort of education that is generally available in the 'advanced economies', or any at all. Many of my readers also struggle with English, so any help I can give them I will continue to provide.
Finally on this specific topic, several of the aforementioned links connect to web-pages that regularly change their URLs, or which vanish from the Internet altogether. While I try to update them when it becomes apparent that they have changed or have disappeared I can't possibly keep on top of this all the time. I would greatly appreciate it, therefore, if readers informed me of any dead links they happen to notice.
In general, links to 'Haloscan' no longer seem to work, so readers needn't tell me about them! Links to RevForum, RevLeft, Socialist Unity and The North Star also appear to have died.
It is also worth pointing out that phrases like "ruling-class theory", "ruling-class view of reality", "ruling-class ideology" (etc.) used at this site (in connection with Traditional Philosophy and DM), aren't meant to suggest that all or even most members of various ruling-classes actually invented these ways of thinking or of seeing the world (although some of them did -- for example, Heraclitus, Plato, Cicero, and Marcus Aurelius). They are intended to highlight theories (or "ruling ideas") that are conducive to, or which rationalise, the interests of the various ruling-classes history has inflicted on humanity, whoever invents them. Up until recently this dogmatic approach to knowledge had almost invariably been promoted by thinkers who either relied on ruling-class patronage, or who, in one capacity or another, helped run the system for the elite.**
However, that will become the central topic of Parts Two and Three of Essay Twelve (when they are published); until then, the reader is directed here, here and here for further details.
[**Exactly how this applies to DM will, of course, be explained in several other Essays published at this site (especially here, here and here). In addition to the three links in the previous paragraph, I have summarised my argument (but this time written for absolute beginners!) here.]
It is also worth pointing out that a good 25% of my case against DM has been relegated to the End Notes. Indeed, in this particular Essay, much of the supporting evidence and argument is to be found there. That has been done to allow the main body of the Essay to flow a little more smoothly. In many cases, I have added numerous qualifications, clarifications and considerably more detail to what I have to say in the main body. In addition, I have raised several objections (some obvious, many not -- and some that might well have occurred to the reader) to my own arguments, to which I have then responded. [I explained why I have adopted this tactic in Essay One.]
If readers skip this material, then my replies to any qualms or objections they might have will be missed, as will my expanded comments and clarifications.
[Since I have been debating this theory with 'dialectical comrades' for well over 25 years, I have heard all the objections there are! Many of the more recent on-line debates are listed here.]
Update 07/03/2014: I have just received a copy of Burger et al (1980), the existence of which I had been unaware until a few weeks ago. One of the contributors to this book, Hyman Cohen [Cohen (1980)] seems to have anticipated (and answered) one or two of the points I have raised in this Essay. Unfortunately, Cohen's 'answers' also fail miserably; I will attempt to explain why that is so in a future re-write of this Essay.
Update 29/11/2016: I have now added a few thoughts about Cohen's egregious logical confusions to Essay Four Part One.
Finally, anyone puzzled by the unremittingly hostile tone I have adopted toward DM/'Materialist Dialectics' [MD] in these Essays might do well to read this first.
As of September 2023, this Essay is just over 96,000 words long; a much shorter summary of some of its main ideas can be accessed here, an even shorter one, here.
The material presented below does not represent my final view of any of the issues raised; it is merely 'work in progress'.
[Latest Update: 01/09/23.]
Anyone using these links must remember that they will be skipping past supporting argument and evidence set out in earlier sections.
If your Firewall/Browser has a pop-up blocker, you will need to press the "Ctrl" key at the same time or these and the other links here won't work!
I have adjusted the font size used at this site to ensure that even those with impaired vision can read what I have to say. However, if the text is still either too big or too small for you, please adjust your browser settings!
(1) Introduction
(2) Dialectical 'Contradictions'
(b) Formal And Colloquial Contradiction Vs 'Dialectical Contradictions'
(c) 'Resolving' Contradictions
(d) Hegel Screws Up
(e) Disambiguation
(3) Initial Problems
(b) "Solved" In What Way? And By Whom?
(e) Motion 'Itself'
(i) Are These Simply Mere Words?
(ii) Does DM 'Reflect Reality'?
(iii) Has Engels's Theory Of Motion Ever Been Tested In Practice?
(4) Do Contradictions Explain Motion? Or Merely Re-Describe It?
(b) Are Contradictions Causes?
(ii) Are These Even 'Dialectical' Contradictions?
(iii) Are These Contradictions Real Or Are They Merely Fictional?
(c) 'Internal Contradictions' And Motion
(5) Is Engels's Theory Comprehensible?
(c) A First Attempt At Disambiguation
(d) A Second Attempt At Disambiguation
(e) Fatal Ambiguity
(6) The Classical Response To Zeno
(a) The Devil Is In The Details
(b) Space To Let
(8) Further Problems
(a) The Background To Engels's Argument?
(i) Interlude One -- Moving Pictures
(b) Pick Your Favourite Contradiction
(i) Interlude Two -- 'Appearances To The Contrary'
(i) Interlude Three -- Terminology
(d) Samuel Beckett Eat Your Heart Out
(9) No Word Is An Island -- Philosophers Ignore Ordinary Language
(b) Ordinary Language And Paradox
(d) Ordinary Objects Regularly Do The Seemingly Impossible
(10) 'Dialectical Objects' Do The Oddest Things
(a) Moving While Remaining Perfectly Still
(b) 'Dialectical Objects' -- Do They Move Or Simply Expand?
(c) Or Do They Just Concertina?
(d) Coordinates To The Rescue?
(11) Everyday Miracles?
(a) Ordinary Objects Behave 'Miraculously'
(i) Interlude Four -- Further Examples Of Movement Where There Is No Movement
(b) Yet More 'Anti-Dialectical' Scenarios Hegel And Engels Blithely Ignored
(12) Inferences From Language To The World
(a) Thought Experiments In Place Of Scientific Investigation
(13) Conclusion
(14) Notes
(15) Appendix A: Thomas Weston On How To 'Resolve' A 'Contradiction'
(16) References
Summary Of My Main Objections To Dialectical Materialism
Abbreviations Used At This Site
In this Essay the role that 'contradictions' are supposed to play in explaining (or even describing) motion and change will be critically examined, and this will be done to a level of detail never before attempted by a revolutionary socialist in the entire history of Marxism.1
[DM = Dialectical Materialism/Materialist, depending on the context; FL = Formal Logic.]
In general, DM-theorists illustrate what they mean by the 'contradictory nature of reality' by appealing to a handful of examples (which they have been using and re-using, over and over, for more than a century), one of which is based on a paradox invented by an ancient Greek mystic called Zeno. To that end, and in order to highlight the limitations of FL, Engels directed the attention of his readers to the 'contradictory nature of motion', depicting it in the following way:2
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152.]3
Engels openly acknowledged he derived the above idea from the über-mystic himself, Hegel. [Notice how these ideas originated with mystics?] In the above passage, Engels linked change with motion and then both with 'contradictions' that supposedly exist in nature and society (something else he confidently asserted elsewhere in the same book).
The aim of this Essay will be to show that no matter what is done to try to make sense of the claim that motion is contradictory, none at all can be made of it. However, before the above passage is examined in detail there are several serious problems it presents that will need to be addressed first since they influence the overall interpretation placed on the conclusions drawn so effortlessly by Engels. Left unresolved, they threaten to undermine completely what he had to say about motion and change --, and much else besides.
But, even before Engels's enigmatic words are examined in the above manner, some sense needs to be made of the phrase "dialectical contradiction" and the idea that reality is 'fundamentally contradictory'.
[The following material used to form part of Note 1. I begin my actual analysis of Engels's words quoted earlier, here.]
As we are about to discover, it is impossible to form any clear idea of the theory that reality is fundamentally contradictory. This Essay will however concentrate on one aspect of that claim: Engels's (and derivatively on Hegel's) remarks concerning the supposedly contradictory nature of motion. In addition to the passage quoted in the previous sub-section, we have the following remarks that are connected (directly or indirectly) with that specific idea:
"Instead of speaking by the maxim of Excluded Middle (which is the maxim of abstract understanding) we should rather say: Everything is opposite. Neither in heaven nor in Earth, neither in the world of mind nor of nature, is there anywhere such an abstract 'either-or' as the understanding maintains. Whatever exists is concrete, with difference and opposition in itself. The finitude of things will then lie in the want of correspondence between their immediate being, and what they essentially are.
"Contradiction is the very moving principle of the world: and it is ridiculous to say that contradiction is unthinkable. The only thing correct in that statement is that contradiction is not the end of the matter, but cancels itself. But contradiction, when cancelled, does not leave abstract identity; for that is itself only one side of the contrariety. The proximate result of opposition (when realised as contradiction) is the Ground, which contains identity as well as difference superseded and deposited to elements in the completer notion." [Hegel (1975), p.174; Essence as Ground of Existence, §119. Bold emphases added.]
"[B]ut contradiction is the root of all movement and vitality; it is only in so far as something has a contradiction within it that it moves, has an urge and activity." [Hegel (1999), p.439, § 956. Bold emphasis added.]
"Dialectics…prevails throughout nature…. [T]he motion through opposites which asserts itself everywhere in nature, and which by the continual conflict of the opposites…determines the life of nature." [Engels (1954), p.211.]
"[Among the elements of dialectics are the following:] [I]nternally contradictory tendencies…in [a thing]…as the sum and unity of opposites…. [E]ach thing (phenomenon, process, etc.)…is connected with every other…. [This involves] not only the unity of opposites, but the transitions of every determination, quality, feature, side, property into every other….
"In brief, dialectics can be defined as the doctrine of the unity of opposites. This embodies the essence of dialectics…. The splitting of the whole and the cognition of its contradictory parts…is the essence (one of the 'essentials', one of the principal, if not the principal, characteristic features) of dialectics…. The identity of opposites…is the recognition…of the contradictory, mutually exclusive, opposite tendencies in all phenomena and processes of nature…. The condition for the knowledge of all processes of the world in their 'self-movement', in their spontaneous development, in their real life, is the knowledge of them as a unity of opposites. Development is the 'struggle' of opposites…. [This] alone furnishes the key to the self-movement of everything existing…. The unity…of opposites is conditional, temporary, transitory, relative. The struggle of mutually exclusive opposites is absolute, just as development and motion are absolute…." [Lenin (1961), pp.221-22, 357-58. Italic emphases in the original; bold emphases added. Several paragraphs merged.]
"Motion is a contradiction, a unity of contradictions." [Ibid., p.256.]
[For Hegel's more detailed (and even more obscure) remarks on this topic, see here.]
The problem is that this entire theory has been handled with the utmost lack of clarity by its proponents (the work of Graham Priest excepted, of course -- although it is arguable that the 'contradictions' he focuses on aren't even 'dialectical' to begin with; on that, see here and here).
In Essay Four Part One, Essay Six, Essay Eight Parts One, Two, and Three and Essay Eleven Part One (as well as here, in the present work), I have shown that while DM-theorists frequently use the term "contradiction" (negatively, in their endeavour to expose the (alleged) limitations of FL; positively, in their attempt to explain change and development), the vast majority display little or no comprehension of the subject matter itself (i.e., of FL and the word "contradiction", itself!). Nevertheless, that hasn't prevented them from claiming that their understanding is superior to any displayed by qualified logicians themselves.
[Essay Four Part One (link above) showed how far from the truth that claim itself lies!]
According to dialecticians, one of the advantages of a wider (Hegelian) application of the term "contradiction" is that it allows them to account for motion and change. Contrast that with those who confine themselves to the straight-jacket of FL, or even the cloying banalities of 'commonsense', and who employ conceptual resources totally inadequate to the task, howsoever much they seem to work in everyday contexts.
Or so it might be argued...
Despite this, as we will discover in what follows, the above claims are wildly inaccurate -- at least, with respect to motion and change. Indeed, as several other Essays published at this site also show, not only is DL incapable of accounting for change itself, dialecticians struggle to account for something as mundane as a bag of sugar!
[DM = Dialectical Materialism/Materialist, depending on the context; FL= Formal Logic; DL = Dialectical Logic.]
Formal And Colloquial Contradictions Vs 'Dialectical Contradictions'
Clearly, the word "contradiction" is also a technical term employed in FL, but, as we are about to find out, it is woefully misunderstood by DM-theorists themselves.
[LOC = Law of Non-Contradiction.]
However, as far as ordinary language is concerned, one of the ways in which we are able to speak about change involves the (implicit, or even explicit) use of a linguistic rule that many misconstrue as a logical truth (i.e., the LOC), which nevertheless enables us to draw specific inferences from what might otherwise appear to be contradictory propositions. So, if two apparently contradictory sentences are held true at different times, then (given other considerations) speakers of that language would normally conclude that the subject of those sentences had changed. For instance, consider the following two (semi-formal) sentences:
C1: NN isn't a member of the Labour Party, at t1.
C2: NN is a member of the Labour Party, at t2. [t2 > t1]
[Here, t1 and t2 are temporal variables; ">" means "later than".]
Obviously, a change like that would usually be expressed in ordinary language more directly, either by the use of tensed verbs or the employment of a paraphrase of some sort. For instance:
C1a: NN wasn't a member of the Labour Party last week.
C2a: NN is a member of the Labour Party today.
C2b: NN has just joined the Labour Party.
This means that apparently contradictory sentences like these -- coupled with a wider use of the negative particle (in all its forms), in tandem with the rich vocabulary available to us in the vernacular (e.g., the use of verbs, adjectives, adverbs, pronouns, etc.) -- are integral to our ordinary notion of change. This alone shows that the claim dialecticians make, that ordinary language and FL can't cope with change, is a gross distortion. As we can see, it is in fact the opposite of the truth.
Of course, this facility we have with language is rather obvious; indeed, it appears to be so blindingly obvious that DM-fans regularly misconstrue it, or they fail to see its significance.
[It is worth adding that the above isn't, of course, the only way we can speak about change in ordinary language! On the seemingly countless ways we are able to do that, see here. The above examples only appear to be banal and blindingly obvious because we are all familiar with the use of ordinary language in everyday life -- except when we try to do a little 'philosophising'. Then we become linguistic philistines.
[On the ability of FL to cope with change, see Essay Four.]
Hence, the accusation that ordinary language and FL can't cope with, or account for, change isn't just the opposite of the truth, it is quite bizarre. In fact, without the resources available to us in the vernacular, human beings wouldn't be able to conceptualise change at all, let alone evaluate the many and varied theories of change that philosophers regularly concoct.
[And that comment applies equally well to the theories that scientists and dialecticians construct. In fact, ordinary language is capable of handling change far better than the obscure jargon invented by metaphysicians. Indeed, it is demonstrably and markedly better than the wooden language concocted by Christian Mystics like Hegel. On that, see here.]
In that case, if, by their peculiar use of language, dialecticians end up undermining the vernacular, their theory can't fail to be problematic, if not incomprehensible --, which is indeed what this Essay will demonstrate (at least with respect to their 'theory of motion').
Now, as far as FL is concerned, two propositions are contradictory just in case they can't both be true and can't both be false, at once. [The second condition is almost invariably ignored by DM-critics of FL -- i.e., that two contradictory propositions can't both be false at the same time. Some even try to deny there is a genuine distinction here (which means such individuals readily confuse inconsistencies with contradictions), even after it has been pointed out to them! In fact, they tend to call such fine distinctions and careful attention to detail, "pedantry" (or they declare them "merely semantic"). The profound dialectical confusion that results if such distinctions are ignored can be seen in all its glory, here. Its importance will emerge as this Essay unfolds. On the confusion it would create in science, let alone anywhere else, if contradiction and inconsistency were conflated, see here.]
Naturally, when two contradictory propositions are conjoined -- as they have been in this formal sentence, ¬(p & ¬p) -- that represents the simplest form of FL-contradiction (and, in many cases, this is also the case in ordinary language (when interpreted)). But there far more complex contradictions than this in FL, and a potentially infinite number of them, too!
[The difference between "contradictory" and "contradiction", also ignored by DM-fans, is explained here.]
Two relatively short examples of the potentially infinite number of these more complex FL-contradictions are the following:
C3: ¬[(p → q) v (p → r) ↔ (p → (q v r))].
C4: ¬[¬(Ex)(Fx & ¬Gx) ↔ (x)(Fx → Gx)].
[In the above, "(E...)" is the existential quantifier (equivalent to "at least one" or "some"); "↔" is a biconditional sign, "iff" (which stands for "if and only if"); "(x)" is the universal quantifier (equivalent to "all" or "every"); "&" stands for "and"; "v" is the inclusive "or" (i.e., "and/or"); "¬" stands for the negation operator ("It is not the case that..."); "→" is the conditional sign (i.e., "if...then"); "p", "q", and "r" are propositional variables; "F" and "G" are one-place, first-level predicate letters; and "x" is a second-level, place-holding symbol. (More details can be accessed, here and here.)]
C3 reads: "It isn't the case that ((if p then q) or if (p then r)) if and only if (if p then (q or r))."
C4 reads: "It isn't the case that [(there isn't something which is F and not G) if and only if (everything which, if it is F, is also G)]"; or even,
C4a: "It isn't the case that [(there isn't anything which is F and not G) if and only if (everything which, if it is F, is also G)]."
[C4a is perhaps a little more colloquial.]
Some might wonder when formal sentences like these would ever be used. Well, Mathematical Logic, the Foundations of Mathematics, Discrete Mathematics, and Number Theory -- to name just four disciplines -- use propositions like these all the time, and, indeed, others that are far more complex. (This links to a PDF.)
These are, of course, just two of the potentially infinite number of logical contradictions which can be generated in MFL. DM-theorists would be hard-pressed to find space -- even in their quirky universe -- for contradictions like these (once they have been interpreted).
[MFL = Modern Formal Logic; LEM = Law of Excluded Middle; PB = Principle of Bivalence.]
Moreover, dialecticians often confuse the LEM, the PB, propositional bi-polarity and the LOC with one another -- and, as to compound the problem, all of them with opposites, inconsistencies, absurdities, contraries, paradoxes, puzzles, quandaries, oddities, irrationalities, oppositional processes, antagonisms, forces of attraction and repulsion, events that go contrary to expectations, alongside a host of other idiosyncratic, non-equivalent terms, ideas and concepts. In fact, they are so eager to see contradictions everywhere that they find they have to tinker with the meaning the word itself so that (for them) it becomes synonymous with "struggle", "conflict" or even "opposition".
[Numerous examples of these and other dialectical confusions in this area were given in Essays Four, Six, Eight Parts One, Two and Three and Eleven Part One.]
A typical example of DM-profligacy in this respect (that is, in the way that DM-fans carelessly and unthinkingly throw "contradiction" at the page or the screen) surfaced in a letter sent to Socialist Worker at the end of August 2011:
"I'm writing regarding Charlie Hore's article on economic growth during the reform period in China (Socialist Worker, 20 August). It doesn't mention the powerful contradictions that emerged within the ruling bureaucracy as a result of the reforms. Not all sectors of the bureaucracy have benefited from the reforms. There has been a shift from ideological campaigns towards a performance-based notion of state legitimacy. This has meant that many officials have experienced anxiety about their relevance in Chinese politics and have been dragged into protest movements. A socialist analysis has to make sense of these contradictions." [Bold emphasis added. Paragraphs merged. I have quoted several more cases of DM-profligacy over the use of this word in Essay Eight Part Two, here, here, here, here, and here -- but there countless other examples I could have used.]
So, according to the above, tensions within the communist hierarchy are 'contradictions'. But, no one ever explains why such things should be called "contradictions" to begin with, especially when they are far more accurately to be described as "tensions" or "conflicts". Nor do they even think to show that such 'contradictions' are 'dialectical'. In this case, for example, the above letter writer never once asked if the elements (the supposed 'dialectical opposites' involved) in any of these 'contradictions' implied each other. [The answer, it seems, is "No they don't imply one another". If anyone thinks differently, please email me with the details.] Was it even asked if either 'opposite' can exist without the other? No, that was neither asked nor answered. But, that is quite unlike the alleged 'contradiction' between the bourgeoisie and the proletariat. There, these two classes supposedly imply one another so that neither can exist without that other. [I have used "alleged" and "supposedly" here for reasons set out in Essay Eight Part Two.] There is no 'internal' relation exposed in the above example from China, no 'interpenetrated opposites'. Do the tensions in the Chinese ruling elite struggle with and then turn into each other (which is what the DM-classics assure all such 'dialectical opposites' do)? Not that anyone has noticed.
So, even if it were correct to call this a 'contradiction', it can't be a 'dialectical contradiction'. In that case, what is it doing in the above article?
But, this is just is standard practice in DM-circles. Anyone who harbours doubts should try the following experiment: Over the next few years, as you read the material pumped out by DM-fans, make a note of the number of times the word "contradiction" has been airily thrown at the page or screen and the number of times the individual(s) doing so bother to show that the 'contradictions' they see everywhere are indeed 'dialectical'. That is: how many times they ask the sort of questions posed in the last but one paragraph and how many times they are answered. After having read such material now for nigh on forty years, I have only ever seen this done twice: (i) Concerning the alleged 'dialectical relation' between the proletariat and the capitalist class, and (ii) In connection with the 'master-slave dialectic' -- both of which cases I have destructively criticised in Essay Eight Part Two, here and here. In fact, I can safely predict, here and now, those questions won't be asked (let alone answered) by the individuals carelessly using the word "contradiction" in the above manner. Naturally, this means the 'contradictions' they speak about aren't 'dialectical'.
Some might conclude that this is all academic and represents just another example of Ms Lichtenstein's abstract formalism and pedantry. But that isn't so. [On 'pedantry', see here.] There are important political reasons for rejecting the use of "contradiction" in the loose and cavalier way that word is employed by Dialectical Marxists. [On that, see Essay Nine Part Two.]
Specifically, these:
(1) The use of this word 'allows' dialecticians to argue in favour of anything they find expedient and its opposite -- this trick is often performed by the very same dialectician, on the same page, in same paragraph, or even during the same speech! --, no matter how anti-Marxist or counter-revolutionary that 'anything' might prove to be. These moves are then 'justified' on the basis that since everything is 'contradictory', and a 'unity of opposites', Marxist theory and practice should be contradictory, too! Here is a classic example of this (courtesy of 'The Great Teacher', Stalin):
"The flowering of cultures that are national in form and socialist in content under the dictatorship of the proletariat in one country for the purpose of merging them into one common socialist (both in form and content) culture, with one common language, when the proletariat is victorious all over the world and when socialism becomes the way of life -- it is just this that constitutes the dialectics of the Leninist presentation of the question of national culture. It may be said that such a presentation of the question is 'contradictory.' But is there not the same 'contradictoriness' in our presentation of the question of the state? We stand for the withering away of the state. At the same time we stand for the strengthening of the dictatorship of the proletariat, which is the mightiest and strongest state power that has ever existed. The highest development of state power with the object of preparing the conditions for the withering away of state power -- such is the Marxist formula. Is this 'contradictory'? Yes, it is 'contradictory.' But this contradiction us bound up with life, and it fully reflects Marx's dialectics." [Political Report of the Central Committee to the Sixteenth Congress of the CPSU(B), 27/06/1930. Bold emphases alone added; quotation marks altered to conform with the conventions adopted at this site. Paragraphs merged.]
So, less democracy was also more democracy, and the suppression of national culture is the opposite of what it appears to be (for those who don't 'understand' dialectics, of course)!
[Several more examples of this bizarre, almost Zen-like, phenomenon were given in Essay Nine Part Two.]
(2) This linguistic dodge is also used to rationalise substitutionist strategies, tactics and moves on the basis that even though Marx insisted on the self-emancipation of the working class, we can substitute one or more of the following classes or social fractions for them: (a) The Party, (b) The Red Army, (c) 'Third World' guerrillas, (d) 'Progressive' nationalists, (e) Students, (f) Sympathetic, left-leaning politicians, or (g) An assortment of social forces, 'rainbow coalitions' and protest groups, no matter how contradictory this might otherwise seem. And concerning those who might object..., well, they just don't 'understand' dialectics; nor, indeed, do they comprehend the 'contradictory' nature of Marxism, the class war, the former USSR..., etc., etc.
(3) The use of this word 'allows' DM-fans to look at the long-term failure of Dialectical Marxism and fail to see it for what it is: a protracted and profound refutation of their core theory, 'Materialist Dialectics'. Indeed, it 'allows' them to interpret this abysmal record as the opposite of what it is -- on the grounds that appearances 'contradict' underlying 'essence'. So, if Dialectical Marxism looks like it is hopelessly unsuccessful and seems to be a long-term, abject failure, the opposite is in fact the case. This then encourages dialecticians to stick their heads in the very same sands into which our entire movement has been running for several generations.
(4) Because of Alternative (3), above, this word also provides DM-acolytes with a source of consolation for the almost total ineffectiveness of the entire movement, -- its divisiveness, its sectarianism, its warring parties/tendencies: "Well, what else can you expect in a contradictory universe? Marxism should look the opposite of the way it really is! Dialectics allows us to grasp this contradiction." Indeed, as Lenin pointed out:
"The splitting of a single whole and the cognition of its contradictory parts...is the essence (one of the 'essentials,' one of the principal, if not the principal, characteristics or features) of dialectics.... The struggle of mutually exclusive opposites is absolute, just as development and motion are absolute...." [Lenin (1961), pp.357-58. Quotation marks altered to conform with the conventions adopted at this site. Paragraphs merged; bold emphasis alone added.]
So, "splitting" is an "essential", if not "the principle" aspect of this theory, with "struggle" an "absolute". Plainly, this "essential" feature of the movement must also involve the relations between 'comrades'. That was something Engels also emphasised, and he openly connected it with 'dialectics':
"It would seem that any workers' party in a large country can develop only through internal struggle, as indeed has been generally established in the dialectical laws of development." [Engels to Bernstein, October 20, 1882; MECW Volume 46, p.342. Bold emphasis added.]
[All of the above have been fully documented and substantiated in Essay Nine Part Two. Readers are directed there for more details. I have also posted many more examples of the peculiar things DM-fans say about the 'contradictions' they seem to see everywhere, in Essay Eight Part Two -- here and here, for instance.]
So, this isn't 'pedantry', nor is it merely 'academic' point-scoring. "Contradiction", as it has been -- and still is being -- used by DM-fans, possesses disastrous political and ideological implications, which must be challenged.
Be this as it may, DM-theorists themselves would be the first to point out that their interest doesn't lie in contradictory propositions, per se, but with real material forces that express or even constitute conflict in nature and society (but only if they have been confirmed in practice, etc., etc.). Furthermore, since the vast majority of classical DM-theorists believe that reality itself is fundamentally contradictory, then, according to them, propositions which accurately describe or picture the world ought to be contradictory, too -- i.e., they should truthfully reflect the contradictions that exist in nature and society.
However, because (contradictory) propositions are (obviously!) linguistic expressions, they clearly aren't material forces, as such. That must mean they aren't oppositional per se, even though they supposedly reflect, or can be used to reflect (at some level), the dynamic nature of reality -- again, according to dialecticians.
On the other hand, even if contradictory propositions were oppositional, they would only be so in a derivative sense. In any case, the idea here appears to be that while objects and processes in nature are contradictory (or their inter-relationships/inter-actions are), and subject to change, any use of language capable of depicting reality must reflect this fact accurately and adequately if it is to be both precise and objective.
Or, so a (very brief) case for the defence might proceed...
'Resolving' Contradictions
Nevertheless, the principles that underlie FL merely commit us to the view that two contradictory propositions can't both be true and can't both be false at the same time. Hence, on this basis any supposition or claim that two supposedly contradictory propositions can be, or, indeed, actually are, both true at once (or can be, or are, both false at once -- as noted above, dialecticians appear to be unaware of that particular caveat) would automatically be regarded as in some way mistaken or confused.
Indeed, that fact alone would provide sufficient grounds for questioning whether one or both of a pair of allegedly true 'contradictory' propositions so described were in fact propositions to begin with. If it is unclear what is being proposed (in the sense that something determinate has been put forward for consideration), then anyone attempting to do this can't be proposing anything determinate -- that is, without their words being disambiguated. At least, no more than a door can be (literally) opened and shut at the same time.
[Examples of disambiguation in action will be given below and then later in the main body of this Essay. See also here.]
Be this as it may, several factors might contribute to this apparent impasse:
(a) The said 'propositions' could contain typographically similar words that have different denotations;
(b) They could be using ambiguous, vague, or figurative language;
(c) They might be drawn from different areas of discourse; or,
(d) They might have been taken out of context, and so on.
Based on one or more of the above considerations, the presumption would always be that both 'halves' of an alleged contradiction (i.e., at least two supposedly true contradictory indicative sentences) could only be held true together (at the same time and in the same respect) by someone in the grip of some form of linguistic, interpretative or psychological confusion. 'Contradictions' that have been generated in this way wouldn't normally be viewed as capable of revealing 'fundamental truths about reality'. In fact, it is far more likely that any such claim would merely underline the linguistic naivety or the intellectual incapacity of the individual that had been so easily misled.
In that case, the expectation would be that a disambiguation or a clarification of any such alleged 'contradiction' would resolve the 'problem'. Only an exceedingly naive person (or worse, a Mad Dog Idealist, like Hegel) would conclude that just because certain 'concepts', or sentences, appeared to be contradictory, nature and society must be contradictory, too.
Indeed, under normal circumstances, one would be forgiven for concluding that the above 'austere' approach to what might seem to be 'contradictions' would recommend itself to those who at least claim to be materialists. Not only was the alternative view -- that there really are 'contradictions' in nature and society -- invented by card-carrying mystics and Idealists, it clearly 'implies' that the natural world possesses properties that are only rightly to be attributed to human beings -- such as, the ability to converse, argue, and disagree. In short, it implies that extra-mental objects and processes are capable of contradicting one another, which in turn would suggest that 'dialectics' may only 'get off the ground' by anthropomorphising 'reality'.
In addition, the 'austere' approach adopted at this site (which advocates the consistent disambiguation of putative contradictions) undermines another Idealist doctrine, that fundamental truths about reality may be inferred solely from the (supposed) logical and grammatical properties of language. Or, to be more accurate (in this particular case), that they may only be derived on the back of a series of sophomoric errors concerning the nature of contradictions themselves, outlined a few paragraphs back (but in much more detail, here).
Naturally, DM-apologists will view counter-claims like these with no little suspicion. Indeed, the above remarks might even appear to be both dogmatic and aprioristic. Furthermore, it could be argued that this obsession with the fine detail of linguistic use itself collapses into LIE, since it presumes to offer linguistic solutions to what are in fact philosophical, scientific and practical problems.
[LIE = Linguistic Idealism; follow the link for an explanation.]
The opposite is in fact the case. The approach adopted at this site seeks to undermine a longstanding metaphysical dogma (which dialecticians themselves have bought into): that fundamental truths about reality may be inferred solely from language, from 'thought', or even from certain 'concepts'. What Engels had to say about motion is, of course, a classic example of this. Clearly, it is the world (or, rather, our appeal to the facts) that makes what we say true or false; it isn't what we say, or how we say it, that determines the nature of reality. Nor does what we think dictate to nature and society what either or both must be like. It is the opposite approach -- i.e., the traditional view of Philosophy --, that such truths can be inferred from though/language alone, which is integral to Idealism, as George Novack pointed out:
"A consistent materialism cannot proceed from principles which are validated by appeal to abstract reason, intuition, self-evidence or some other subjective or purely theoretical source. Idealisms may do this. But the materialist philosophy has to be based upon evidence taken from objective material sources and verified by demonstration in practice...." [Novack (1965), p.17. Bold emphases added.]
[As Essay Seven will show, DM-'contradictions' can't be confirmed either in or by experience, nor can they be verified in any other way. (The accusation that this view of confirmation -- coupled with the emphasis placed on verification -- smacks of 'positivism', or even 'empiricism', has been neutralised, here.) In Essay Twelve, the ideological motivation underlying the contrary, traditional view will be exposed for what it is: a form of LIE itself (summary here).]
Nevertheless, it is important to recognise when the descriptive, representational or expressive capacities of language begin to break down. Those concerns certainly implicate the entire range of DM-theories since they have the disconcerting habit of breaking down alarmingly quickly when examined closely. They invariably turn out to be hopelessly vague, terminally confused, or even non-sensical and incoherent -- as several Essays at this site have amply demonstrated.
Furthermore, it is equally important to be able to distinguish spurious attempts to depict 'reality' from the genuine article. DM-theorists themselves try to do this when they highlight the confused or self-contradictory nature of rival theories, advocating their rejection on that basis alone. [That claim has been fully documented and substantiated in Essay Eleven Part One.]
Despite this, DM-theorists certainly believe that their approach begins with reality (albeit 'mediated' by the conceptual and practical resources available to human beings at any given time). They then require our linguistic resources, our vocabularies and concepts, be adapted, modified or even upgraded accordingly. On that basis, if nature is contradictory and ordinary language (or even FL) can't accommodate this (assumed) fact, that must be because those resources are either limited or are defective in some way. In that case, they require supplementation, revision or even reinterpretation, fortified by concepts drawn from 'Materialist Dialectics' -- or, where necessary, from the Mother Lode Itself, Hegel's 'Logic' (albeit, put 'back on its feet').
It isn't easy for any (effective) repudiation of the above claims to avoid appearing dogmatic. Language has been moulded throughout history by an evolving set of social norms, practices and conventions, which were themselves refined by factors that have operated across different Modes of Production for millennia. Because of this, it might seem possible to argue that when faced with situations which appeared to be 'contradictory', human beings not only could, they actually did develop and then invent words, categories and concepts that could rightly be described as "dialectical".
[However, the 'factual basis' underlying that supposition will be undermined in Essay Twelve Parts Two, Three and Seven, alongside Essay Fourteen Part One (summaries here and here), and again briefly, below.]
Even so, given other conventions that were actually adopted -- that is, in practice; no one supposes that overt decisions were taken -- the above scenario is extremely unlikely, if not impossible.
As the word itself literally suggests, to contradict someone is to gainsay or deny that what they have uttered is true (or false, as the case may be). So, to take a very simple example, if NN says it is raining and MM says it isn't, then (all things being equal) they would be contradicting one another. Furthermore, neither statement would be affected in the slightest if either one of the following were the case (in the local vicinity of these two individuals):
(i) It is pouring down with rain; or,
(ii) It is dry as a bone.
To be sure, those two sentences use the indexical expression, "it". Hence, it could be objected that the ambiguity and relativity of this particular word undermines the status of those two assertions as contradictories. However, that wouldn't be so if it were also stipulated that NN and MM agree over the meaning and reference of the "it" they have both used. In that case, these assertions would count as genuine contradictories. If so, what they had to say would now become something like the following (illustrated in more detail by J3 and J4):
J1: It is raining.
J2: It isn't raining.
J3: Right now, right here, or near where we are stood/sat/walking/driving (etc.), rain is pouring down.
J4: Right now, right here, or near where we are stood/sat/walking/driving (etc.), there is no rain at all.
As with much else in the vernacular, much greater precision is relatively easy to secure (but, of course, at the expense of increased pedantry).
However, whether or not "It is raining" is actually true in no way affects the fact that these two sentences are contradictory. All that is required is that if one of them is true, the other is false, and vice versa. Moreover, we can say this in advance of knowing which one of these is the case, and even if we never find out or wish to find out. We wouldn't be able to understand anyone who claimed that both NN and MM were mistaken and both J1/J2, J3/J4 were false -- except in the circumstances covered below. Fanciful situations to one side (which was partially the point of the "all things being equal" clause added to the above), how, for example, would it be possible for it to be false that it is raining and false that it isn't raining at the same time, in the same location, in the same respect?
[Recall: DM-fans are oblivious of this additional necessary condition for two sentences to be contradictory -- i.e., that they can't both be false at once and in the same respect.]
Some might point to the vagueness of sentences like "It is raining". That would seem to mean both of the above sentences could in fact be false, since it might be indeterminate whether or not it is raining. Perhaps the weather is clearing up, so that anyone who said it was raining would be wrong, just as anyone who said it wasn't would be mistaken, too. Maybe someone has turned on a powerful fire hose or rain machine like they use in movies. Undeniably, sentences like these are vague, but just as soon as it had been decided that it is actually raining, then just one of the following sentences would be false and the other true: (a) "It is raining", (b) "No, it isn't raining". The same is the case in reverse -- i.e., if it had been decided that it isn't in fact raining: one of (a) or (b) would be false, but not both. In circumstances like these, we wouldn't be able to make sense of anyone who said both were false, or both were true -- when the truth-status of at least one of them had been decided upon in the manner just specified.
But, what if we can't in principle decide whether or not it is or it isn't raining? In that case, sentences like these would (in principle) lack a truth-value; neither could be deemed true or deemed false -- that is, until a decision either way became possible. In such circumstances, these two sentences would fail even to be propositions. If, in principle, we couldn't decide whether or not it is (ever) raining then nothing determinate will have been proposed (i.e., put forward for consideration) by saying it is or by saying it isn't. I am of course speaking about a radical failure to decide, here; that is, where no one could decide, even in theory, whether or not it was raining in the vicinity of those attempting to determine whether or not it was the one or the other. If it were in principle impossible to decide in such cases, and it had always been impossible to so decide, then there would be no point to uttering such sentences and they wouldn't have entered the language. "It is raining" would lack both a sense and a use. Radically unusual circumstances like this would even call into question the concept of rain itself. If it were impossible to decide in this manner then the word "rain" itself would lack meaning.
Compare the above with what was said about 'meskonators' in Essay Eleven Part One. If it is impossible for you, dear reader, to decide if something is or isn't a meskonator, and, indeed, it turned out that no one could so decide, that word would surely lack a meaning.
[This brings in factors associated with what Wittgenstein called "criteria" and "symptoms". I have said more about that topic in Essay Twelve Part One; readers are directed there for more details.]
However, in what might be described as non-radical circumstances, where, for contingent reasons, it still can't be decided whether or not it is training -- maybe the interlocutors in question are trapped underground, are locked away in a dungeon, can't see outside, are unable to receive any information from the outside world for whatever reason, or they can't distinguish rainfall from water cascading from a fire hose or a rain machine, etc., etc. -- then these two sentences would still be contradictory, since if one of them were true (whether or not that fact is actually known), the other would automatically be false, and vice versa.
However, in everyday life (i.e., outwith the use of aesthetic, ethical, political and religious language (etc.) where the meaning of words is often "essentially contestable"), problems like this don't normally arise. So, when in doubt we say things like "It's trying to rain...", "It's spitting, I think...", "I reckon it's clearing up..." or even, "Well, it looks like rain to me!". Only a hardcore contrarian would come out with statements like "It is and it isn't raining" -- perhaps on the basis that there are gaps between the raindrops, or because it is raining in the vicinity, but not, say 100 metres down the road or in the next county. If someone were consistently to adopt such a contrarian approach to all such (indicative) sentences, they would either have very few friends or they would enjoy a severely limited social life. Either that, or they would be diagnosed with a Personality Disorder of some sort. If we all adopted such an attitude, inter-communication would soon grind to a halt. [On that, see here.]
[It is also worth noting that contradicting someone can be aimed at challenging truth and not always confronting falsehood, as many suppose.]
It could be objected that the following was claimed earlier:
...if NN says it is raining and MM says it isn't, then (all things being equal) they would be contradicting one another. Furthermore, neither statement would be affected in the slightest if either one of the following were the case (in the local vicinity of these individuals): (i) It is pouring down with rain, or (ii) It is dry as a bone.... However, whether or not "It is raining" is actually true in no way affects the fact that these two sentences are contradictory.
When it was asserted a few paragraphs later:
But, what if we can't in principle decide whether or not it is or it isn't raining? In that case, sentences like these would (in principle) lack a truth-value; neither could be deemed true or deemed false -- that is, until a decision either way became possible. In such circumstances, these two sentences would fail even to be propositions. If, in principle, we couldn't decide whether or not it is (ever) raining then nothing determinate will have been proposed (i.e., put forward for consideration) by saying it is or by saying it isn't. I am of course speaking about a radical failure to decide, here; that is, where no one could decide, even in theory, whether or not it was raining in the vicinity of those attempting to determine whether or not it was the one or the other. If it were in principle impossible to decide in such cases, and it had always been impossible to so decide, then there would be no point to uttering such sentences and they wouldn't have entered the language. "It is raining" would lack both a sense and a use. Radically unusual circumstances like this would even call into question the concept of rain itself. If it were impossible to decide in this manner then the word "rain" itself would lack meaning.
Which is it to be? If we can't decide whether sentences like these are true or they are false, then how can they be contradictory?
The (hypothetical) objector forgot to quote this caveat:
All that is required is that if one of them is true, the other is false, and vice versa.
And we can arrive at that conclusion well in advance of knowing whether one of them is in fact true or one of them is in fact false. As noted below, those options are based on rules we have for the use of the negative particle, and, as with any rule, it is possible to decide how that rule can or can't, must or mustn't, be applied in advance of actually applying it. For instance, we can decide ahead of time what would and what wouldn't count as offside in football (soccer) even if there is no game actually being played at the time, even if no more games were ever to be played, and even if, during a game, we lost sight both of the pitch and the alleged offence itself (if the pitch were fogbound, for example). Plainly, that is because rules aren't capable of being true or false themselves; they may only be practical or impractical, useful or useless, applied or mis-applied, etc., etc. Hence, this particular rule (connected with the ordinary use of "contradiction" and the negative particle) is independent of any alleged truth or falsehood, as such.
This topic is, of course, connected with the so-called 'Law of Excluded Middle' [LEM], as that 'law' supposedly features in ordinary discourse. In that case, some might find themselves agreeing with Hegel when he asserted the following:
"Instead of speaking by the maxim of Excluded Middle (which is the maxim of abstract understanding) we should rather say: Everything is opposite. Neither in heaven nor in Earth, neither in the world of mind nor of nature, is there anywhere such an abstract 'either-or' as the understanding maintains. Whatever exists is concrete, with difference and opposition in itself. The finitude of things will then lie in the want of correspondence between their immediate being, and what they essentially are." [Hegel (1975), p.174; Essence as Ground of Existence, §119. Bold emphasis added.]
In relation to which Engels had this to say:
"To the metaphysician, things and their mental reflexes, ideas, are isolated, are to be considered one after the other and apart from each other, are objects of investigation fixed, rigid, given once for all. He thinks in absolutely irreconcilable antitheses. 'His communication is "yea, yea; nay, nay"; for whatsoever is more than these cometh of evil.' [Matthew 5:37. -- Ed.] For him a thing either exists or does not exist; a thing cannot at the same time be itself and something else. Positive and negative absolutely exclude one another, cause and effect stand in a rigid antithesis one to the other.
"At first sight this mode of thinking seems to us very luminous, because it is that of so-called sound common sense. Only sound common sense, respectable fellow that he is, in the homely realm of his own four walls, has very wonderful adventures directly he ventures out into the wide world of research. And the metaphysical mode of thought, justifiable and even necessary as it is in a number of domains whose extent varies according to the nature of the particular object of investigation, sooner or later reaches a limit, beyond which it becomes one-sided, restricted, abstract, lost in insoluble contradictions. In the contemplation of individual things it forgets the connection between them; in the contemplation of their existence, it forgets the beginning and end of that existence; of their repose, it forgets their motion. It cannot see the wood for the trees.
"For everyday purposes we know and can say, e.g., whether an animal is alive or not. But, upon closer inquiry, we find that this is, in many cases, a very complex question, as the jurists know very well. They have cudgelled their brains in vain to discover a rational limit beyond which the killing of the child in its mother's womb is murder. It is just as impossible to determine absolutely the moment of death, for physiology proves that death is not an instantaneous momentary phenomenon, but a very protracted process. In like manner, every organic being is every moment the same and not the same, every moment it assimilates matter supplied from without, and gets rid of other matter; every moment some cells of its body die and others build themselves anew; in a longer or shorter time the matter of its body is completely renewed, and is replaced by other atoms of matter, so that every organic being is always itself, and yet something other than itself." [Engels (1976), pp.26-27. Bold emphases added; quotation marks altered to conform with the conventions adopted at this site. Some paragraphs merged.]
However, as I have argued in Essay Nine Part One (slightly edited):
Nevertheless, it is difficult to see what Hegel was trying to say here. That is because any attempt to interpret him requires the implicit or explicit use of the very terms he claims are misleading. The construal of his work requires decisions be taken about whether he meant either this or that by what he actually said. If an author always means both -- or maybe even neither -- then interpretation is rendered impossible and any attempt to unravel their meaning becomes self-defeating (as we are about to discover).
So, if Hegel were correct, if absolutely "everything is opposite" and there is no "either-or" anywhere in the universe, it would be impossible to disentangle what he meant from what he didn't, since we would be unable to decide whether he believed of, say, any two sentences, P and Q, one or more of the following:
H1: (i) Both P and Q; (ii) either P or Q; (iii) neither P nor Q; or (iv) either P or Q, but not both.
But, if, say, P and Q were inconsistent (that is, if, for instance, Q implies not P, or vice versa -- for clarity's sake an example will be given below), and we interpreted his words one way -- perhaps that he believed both P and Q, since to do otherwise would involve the implicit or explicit use of the dread 'either-or' --, then, plainly, we would have to conclude that he accepted both as part of an "unfolding of truth" here (as he might have put it). By his own lights that would mean, of course, that he would be unfolding error in place of truth!
Hence, in order to reject one or other of the above options, we, too, would be forced to appeal to, or employ, an "either-or" -- that is, we would have to conclude that Hegel accepted P or he accepted Q, but not both.
On the other hand, if we were to remain true to Hegel's dictum -- that "neither in heaven nor in Earth, neither in the world of mind nor of nature, is there anywhere such an abstract 'either-or' as the understanding maintains" --, we would have to conclude he accepted both.
So, any attempt made now to specify exactly what Hegel meant would undermine what he actually said about the use of the "either-or of understanding", for we would have to accept that Hegel asserted one thing, P, or he asserted something else, Q, but not both. Without that assumption it would become impossible to comprehend or defend him. If Hegel genuinely cast doubt on the "either-or of understanding" (and he wasn't being deliberately enigmatic, disingenuous, mendacious or merely playful) -- and assuming he was correct doing so --, then any attempt to interpret him as asserting P or asserting Q would have to conclude that he asserted both. [Again, I give a clear example of this, below.]
In that case, any determinate interpretation of Hegel (that is, any interpretation that settled on one option, not both) would have to ignore his own advice, by reluctantly accepting the protocols expressed in and by the "either-or" of ordinary language (or of 'commonsense', along with its corollaries), and acknowledge that, concerning either P or Q, Hegel accepted only one of them, not both -- that is, that he was a fully paid-up member of The Society For The Promotion Of The Either-Or Of Abstract Understanding.
In that case, truth would advance (as a result of yet another dialectical inversion) by forcing us to disregard Hegel!
In order to make this more concrete, let us suppose that:
"P" is: "Neither in heaven nor in Earth, neither in the world of mind nor of nature, is there anywhere such an abstract 'either-or' as the understanding maintains";
and,
"Q" is: "There is in fact an abstract 'either-or' somewhere in the world of mind or of nature (etc.)."
[As above, Q implies not P, and vice versa.]
So, either Hegel accepted P or he accepted Q -- which would, of course, imply that there is at least one 'either-or' "in heaven or in earth (etc.)" -- i.e., here, in front of us, right here, right now.
On the other hand, if Hegel took his own advice and accepted both P and Q -- thereby rejecting this particular "either-or" --, then not much sense could be made of what he was trying to say.
Incidentally, the above criticism isn't affected by Hegel's own interpretation of these controversial words, nor any technical meaning his epigones might want to attribute to them, since they, too, would have to conclude that he meant this or he meant that, not both. The issue here solely concerns how we are to understand him now, in this world, by our consideration of those very material words (in print, or reproduced on a screen), quoted earlier.
Hence, it is beside the point whether the rationale for Hegel's criticism of the use of such words by the "abstract understanding" is legitimate or not (irony intended). His writings now appear before us as phenomenal objects, hence, given the additional fact that they aren't self-interpreting (especially when we recall that Hegel is no longer alive to explain himself -- but, even then we would have to accept he meant either P or Q, not both), his words face the ordinary cannons we employ elsewhere to understand anyone's speech. In order to read and perhaps interpret Hegel as believing this or that, but not both, we are forced to ignore his advice and employ the dread "either-or".
Naturally, this is just one more reason why ordinary language can't be by-passed or undermined, no matter which 'genius' tries to fool some of us into thinking otherwise.
Once again, it is little use complaining that this is not how Hegel wanted his use of the "either-or" of "understanding" to be interpreted (i.e., we should perhaps view it ironically -- that is, that we should interpret it this way but not that), since he himself holed that complaint well below the water line when he asserted:
"Instead of speaking by the maxim of Excluded Middle (which is the maxim of abstract understanding) we should rather say: Everything is opposite. Neither in heaven nor in Earth, neither in the world of mind nor of nature, is there anywhere such an abstract 'either-or' as the understanding maintains. Whatever exists is concrete, with difference and opposition in itself. The finitude of things will then lie in the want of correspondence between their immediate being, and what they essentially are. Thus, in inorganic nature, the acid is implicitly at the same time the base: in other words, its only being consists in its relation to its other. Hence also the acid is not something that persists quietly in the contrast: it is always in effort to realise what it potentially is." [[Hegel (1975), p.174; Essence as Ground of Existence, §119. Bold emphasis added.]
Hence, if "everything is opposite", and Hegel's works were written somewhere on this planet, and copies of them still take on a physical form in this universe(!), then anything he committed to paper must be its own opposite, too -- or, he was wrong.
[Irony intended again.]
Either way, it would be foolish to believe Hegel was serious (or, and what is far more likely, that he had thought things through with due care) when he wrote the above words, while also agreeing with what he said about the LEM, the dread "either-or".
So, following Hegel's own advice, the above passage should in fact be re-written -- more consistently -- along the following 'Hegelian', deny-there-is-an-'either-or', lines:
"Instead of both speaking and not speaking by the maxim both of Excluded Middle and not Excluded Middle and (which is and is not the maxim of abstract understanding) we should and we shouldn't rather say: Everything is, and some things are not, opposite. Neither in heaven nor in Earth, and both in heaven and in earth, neither in the world of mind nor of nature, and both in the world of mind and of nature, is there anywhere such an abstract 'either-or' as the understanding maintains, but there is, and it is everywhere, too, while it is nowhere as well. Whatever exists is concrete, and it isn't, with difference and opposition, and also without difference or opposition, in itself, and not in itself, too. The finitude of things will and will not then lie in the want of correspondence, but also with actual correspondence, between their immediate being, and what they essentially are, or are not, and, indeed, both. Thus, in inorganic nature, and outside it, the acid is and is not implicitly at the same time, and at other times, the base, but it isn't the base, either: in other words, but also in the same words, its only being, and its many other beings, consist, and do not consist, in its relation, and absence of any relation, to its other, and whatever isn't its other. Hence also the acid is not something, and it is something, that persists quietly, and not quietly, in the contrast, or the accord: it is always, and is it is never, in effort to realise what it potentially is, and what it actually is not." [Hegelianised version of Hegel (1975), p.174; Essence as Ground of Existence, §119.]
Everyday, boring old non-abstract understanding will, I think, readily see what arrant nonsense results from Hegel's 'genius' when we apply his ideas to his own words -- providing we remain in this universe.
Any who object to the above re-write can, of course, neutralise its implications by demonstrating that Hegel's work wasn't actually written in this universe, or on real paper, but was written on Ideal paper, neither in heaven nor on earth -- and that they themselves don't exist anywhere, either (or both, or neither), in order to do that (or not).
[On the 'acid and base' example -- even if we were to take Hegel's comments about such reagents seriously -- see here.]
In a recent book [Stewart (1996)], several misinterpretations and misrepresentations of Hegel's work were 'corrected' by a handful of Hegel scholars. However, there would seem to be little point to such an exercise if Hegel's ideas about "either-or" are to be believed. If he were right -- that in the entire universe there is no "either-or" -- there would be some truth even in the wildest allegations about him and his work.
[LOI = Law of Identity.]
For instance, these: that (i) Hegel fully accepted without question the unlimited applicability of the LOI in every conceivable circumstance, without any qualifications whatsoever (and that includes its use in dialectical and speculative thought, as well as in relation to change, conceptual or material), and he did not; that (ii) he flatly denied that reality or thought is contradictory in any sense at all, and he did not; that (iii) he doubted the truth of every single one of his own ideas all the time, and he did not; that (iv) he wrote nothing at all in German in his entire life, and he did not; that (v) everything he wrote was actually written by Schelling -- in fact it was published only yesterday, and it wasn't --; that (vi) he was a Shape-shifting Martian, and he wasn't...
[Anyone who attempts to reject one or more of the above alternatives -- on the grounds that Hegel must have accepted one of them, or one of them must be true, but not both, or, indeed, that such objectors must do likewise, too -- will, alas, have to employ the dread LEM in order to do so, vitiating Hegel's challenge, as well as their own objections to the above argument.]
It could be objected that this completely misunderstands the nature of DL, at least as Hegel himself conceived it. Unfortunately, even that response is framed in ordinary language -- and, it was foolishly written in this universe! --, so, since a decision has to be taken over whether or not it is valid, a quick reference to DL will indicate it is both.
[DL = Dialectical Logic.]
This means that until DL-fans commit themselves to one or other view (but not both), it is impossible even to begin to evaluate anything they say -- and neither can they!
However, just as soon as they specify what they mean (i.e., that they genuinely intend this but not that), we must cease to take them seriously, since they would then have employed the dread LEM, thereby undermining their own criticisms of it!
Either way, such defenders of Hegel may be ignored even before they decide whether they agree with the above critical comments, or not (or both).
It could be countered that the above conclusions are ridiculous and fail to follow from a consistent application of the dialectical method; hence Hegel can't be saddled with any of them.
Once more, these 'ridiculous conclusions' either do or they do not follow from what Hegel wrote. If the above DM-rebuttal is correct, and they don't follow, then there is at least one either-or at work here, namely this one -- since, in that case, both options wouldn't be correct -- only one option would be the right one, namely, that they don't follow. And, if that is so, these 'ridiculous conclusions' do indeed follow, after all, since Hegel would, in that case, be wrong to assert there is no either-or anywhere in existence when one such has just been used to reject one option in favour of the other!
So, taking each 'ridiculous conclusion' individually: if we maintain that one of them doesn't follow, we will have applied the LEM, once more. That is because we would thereby have denied that that particular 'ridiculous conclusion' does and does not follow, and thus that an either-or option obtains in this case. Hence, we arrive at the same result.
On the other hand, if they do follow, then they do anyway.
Either way, they follow.
The problem with sweeping claims like the one quoted above (which litter Traditional Philosophy and not just Hegel's ill-considered 'Logic') -- in this case, concerning the supposed limitations of certain principles of FL (and especially those that express patterns of inference mirrored in our use of ordinary language, such as the LOI, the LOC and the LEM) -- is that they invariably collapse into incoherence, as we have just seen.
[FL = Formal Logic; LOC = Law of Non-Contradiction.]
Which is why, once again, we can say with complete confidence that no one (not even Hegel) could possibly understand Hegel!
It is, of course, also possible to 'adapt' Engels's comments (from earlier):
"To the metaphysician, things and their mental reflexes, ideas, are isolated, are to be considered one after the other and apart from each other, are objects of investigation fixed, rigid, given once for all. He thinks in absolutely irreconcilable antitheses. 'His communication is "yea, yea; nay, nay"; for whatsoever is more than these cometh of evil.' [Matthew 5:37. -- Ed.] For him a thing either exists or does not exist; a thing cannot at the same time be itself and something else. Positive and negative absolutely exclude one another, cause and effect stand in a rigid antithesis one to the other.
"At first sight this mode of thinking seems to us very luminous, because it is that of so-called sound common sense. Only sound common sense, respectable fellow that he is, in the homely realm of his own four walls, has very wonderful adventures directly he ventures out into the wide world of research. And the metaphysical mode of thought, justifiable and even necessary as it is in a number of domains whose extent varies according to the nature of the particular object of investigation, sooner or later reaches a limit, beyond which it becomes one-sided, restricted, abstract, lost in insoluble contradictions. In the contemplation of individual things it forgets the connection between them; in the contemplation of their existence, it forgets the beginning and end of that existence; of their repose, it forgets their motion. It cannot see the wood for the trees.
"For everyday purposes we know and can say, e.g., whether an animal is alive or not. But, upon closer inquiry, we find that this is, in many cases, a very complex question, as the jurists know very well. They have cudgelled their brains in vain to discover a rational limit beyond which the killing of the child in its mother's womb is murder. It is just as impossible to determine absolutely the moment of death, for physiology proves that death is not an instantaneous momentary phenomenon, but a very protracted process. In like manner, every organic being is every moment the same and not the same, every moment it assimilates matter supplied from without, and gets rid of other matter; every moment some cells of its body die and others build themselves anew; in a longer or shorter time the matter of its body is completely renewed, and is replaced by other atoms of matter, so that every organic being is always itself, and yet something other than itself." [Engels (1976), pp.26-27. Bold emphases added; quotation marks altered to conform with the conventions adopted at this site. Some paragraphs merged.]
It might be instructive if we applied Engels's advice to his words (but, to save the reader's sanity, that tactic has been inflicted on only part of one of the above paragraphs):
"At first sight and not at first sight, this mode of thinking and of not thinking seems, and it doesn't seem to us, and not to us, very luminous and not at all luminous, because it is and it isn't that of so-called sound common sense and not so-called common sense. Only sound common sense, and anything other than common sense, respectable fellow that he is and isn't, in the homely realm of his own four walls and outside them too, has very wonderful adventures and slightly non-wonderful adventures directly he ventures out into the wide world of research, or not...." [Edited, 'Hegelianised', version of Engels's words.]
So, not even Engels could have taken his own advice and hope to have made sense.
[Incidentally, his argument concerning the status of living organisms has been critically analysed here and here.]
Any who still harbour doubts (about the use of "It is raining", etc.) might like to focus instead on sentence pairs like the following:
(i) "The Nile is longer than the Thames"; and,
(ii) "The Nile isn't longer than the Thames"; or,
(iii) "Hitler was a Nazi"; and,
(iv) "Hitler wasn't a Nazi".
Now, can both (i) and (ii), or both (iii) and (iv), be true and false at the same time and in the same respect?
Finally, some readers might object that an earlier example (concerning off-side in football (soccer)) in fact underlines the limitations of FL. So, in a live game, many players (in or near an off-side configuration) will be moving. Hence, at any point they might be situated in a borderline case between on-side and off-side. FL insists there is an "either...or" here when it is often a "both...and".
Or so it might be argued...
Admittedly, there are occasions when even those who have access to video evidence can't decide if a player is actually off-side; different referees and observers might have (and sometimes do) have divergent opinions about such things. But, that doesn't affect the fact that when it is decided that the player concerned is off-side, it then becomes false that he/she isn't off-side.
DM-fans might then be tempted then to respond: FL obviously breaks down when such things are on the point of change.
Or so it could be further maintained...
[DL = Dialectical Logic.]
Ok, so what if it can't be decided? How would DL help here? How does it help a game of football (soccer) for a 'dialectical referee' to declare that a player is both off-side and not offside at the same time? The question answers itself. DL is even more useless.
However, decisions are always made. Never in the history of the game has it been permanently left hanging without a decision (in such circumstances) being made. As noted above, such a decision, once made, will make one of these propositions true -- "Player NN is off-side" or "Player NN isn't off-side" -- and the other false. At no point would anyone come out with "NN is both on-side and off-side!". Not even a referee who is also a DM-fan would conclude this. And that will still be the case even if those reviewing video evidence still can't agree. Someone will arbitrate here (the third referee, perhaps?), and a decision will be made. In fact, in such circumstances, a new rule is invariably adopted whereby the decision is given in favour of the forward/attacker. Often this is called "Giving the forward the benefit of the doubt".
But, let us imagine there actually are some hardcore DM-fans who would happily claim there are situations where both "Player NN is off-side" and "Player NN isn't off-side" are both true. Well, we needn't indulge in such flights-of-fancy since there is a dialectician who fits that description, Graham Priest. He has argued that there are states of affairs where it is true to say a moving object not only can be but is in both states at once. I have discussed his theory in Note 18a. Readers are directed there for more details.
If no decision can in principle be made, then, as we saw earlier, neither of these 'propositions' (time stamped for the game in question -- so that they are now spoken token sentences, not type sentences when combined as follows, "Player NN is off-side and player NN isn't off-side", location, date and time included)" is now a proposition. That is because it is unclear what is being proposed (no more than you, dear reader, would know what you were being asked to do if someone said "Open the door and close it at the same time!"), and such non-propositions have zero to do with ordinary life or FL. In which case, this scenario presents no challenge to FL or the vernacular, contrary to what dialecticians imagine.
[I have said much more about the failure of sentences like this even to count as propositions in Essay Eight Part Three, here, here, here and here.]
It is also worth remembering that not even DL has any use for them, as we have also just seen.
Despite this, there are other strategies that would in all likelihood be adopted in such circumstances, and it is to them that I now turn.
[Some of the points made in this subsection might appear to be rather dogmatic, but subsequent sections will fully substantiate them. They will also be defended in this Essay (and others at this site) from several obvious, and a few not so obvious, objections.]
The facility we have in language to contradict one another (which apparently goes back as far as records last, or as far back as human beings have been able to argue, never mind converse -- indeed, without it, we wouldn't be able to comprehend indicative sentences before we knew whether they were true or whether they were false (why that is so, and why it is important, was explained in Essay Twelve Part One)), means that our ancestors clearly failed to take the route that dialecticians subsequently took. And it isn't difficult to see why. In fact, given current linguistic practices (in tandem with the social relations on which they are based), it is now impossible to make sense of the claim that a contradiction could be true (or, rather, that two contradictory propositions could both be true or could both be false at once -- that is, without (retroactively) altering the meaning of the word "contradiction" itself). Indeed, we would fail to comprehend anyone who claimed that in a dispute (where someone gainsaid what someone else had asserted) both sides could be speaking the literal truth -- ambiguous examples excepted, of course.
[In order to prevent what follows from sliding off into some form of Linguistic Psychologism, it should be read in conjunction with the distinctions established in Shanker (1998), particularly Chapter Three, and especially pp.97-120. Of course, there is nothing wrong with anyone employing the word "contradiction" in a completely novel way, but that having been done, any new use of a typographically identical word can't affect that word's current (ordinary) employment -- nor can it even be related to its ordinary use, let alone to the role it occupies in FL.]
In cases where disputants might appear to be doing this (i.e., where both parties to an argument are gainsaying each other and both also seem to be speaking the literal truth), the most likely response would be to try to disambiguate their words in order to resolve the serious problems that 'true contradictions' would create in everyday life.
And this can be asserted with some confidence because, as noted above, the conventions we now have prevent us from understanding how a contradiction could be true (or, rather, how two contradictory propositions could both be true or both be false, at once). Not only that, but these conventions prevent us from understanding anyone who might think otherwise, or who might try to persuade us to conclude otherwise. Even worse still, they also prevent us from now understanding how our ancestors could have developed alternative conventions -- or, indeed, how we could make sense of anyone who supposed they might have.
This is one intellectual river we can't now step back into, even once -- to paraphrase Cratylus.
In fact, these observations are connected with: (i) The way that negation works in language, and (ii) The capacity language has for allowing us to understand an empirical proposition (i.e., a fact-stating indicative sentence) before we know whether it is true or whether it is false.
[There is much more on this in Essay Twelve Part One.]
[Incidentally, I have added the following codicil: "Or, rather, how two contradictory propositions could both be true or both be false, at once", since most logicians (particularly mathematical logicians) classify contradictions as false, whereas the LOC is a rule of language (or, even better, the LOC is an indirect expression of the rules we have for the use of the negative particle). In that case the LOC is neither a logical truth nor a logical falsehood, and this means that any individual contradiction is therefore senseless, not false.
[The word "sense", as it is used in most of these Essays in such contexts, is explained here -- but the short version is that "senseless", when applied to an indicative sentence, means it is neither true nor false and as such fails even to be a proposition. Once again, I have said much more about the failure of such sentences even to count as propositions in Essay Eight Part Three, here, here, here and here.]
If, per impossible, a contradiction could be false, then it could be true (for reasons explained in Essay Twelve Part One), which would create intractable problems for how we use the negative particle. It is partly for this reason that contradictions are categorised as senseless at this site.
Once again, the above assertions might seem both dogmatic and controversial, so I will now defend each in turn.
Take the first of these -- which was that we should fail to understand anyone who believed a contradiction could be true -- or, rather, how we would fail to understand how two contradictory propositions could both be true or could both be false, at once --, and that we would seek to disambiguate any such contender in order to make sense of what it had to say. Consider the following example:
B1: John Rees wrote and did not write The Algebra of Revolution.
B1a: John Rees wrote The Algebra of Revolution.
B1b: John Rees did not write The Algebra of Revolution.
Let us suppose someone asserted that B1a and B1b were both true. Faced with that it is a safe bet that we would all find it difficult to take this individual and what they said either literally or seriously. That is because, as they stand, both halves of B1 (i.e., B1a and B1b) couldn't be true -- nor could they both be false, at once.
[Some might think that these aren't the type of contradiction of interest to dialecticians; that objection will be dealt with presently. Of course, if there were no such person as John Rees (and there never had been), or no such book had ever been written and published -- meaning The Algebra of Revolution had never even existed --, the above three sentences would fail to be propositions to begin with. Admittedly, B1 is exceedingly trite, but it was deliberately chosen so that the strategy of disambiguation could be made clear to all.]
However, if both B1a and B1b were still held true, then, trivial cases aside (such as: "John Rees" referred to two separate individuals and "The Algebra of Revolution" to two different books, both with the same name), we could only make sense of the contradiction they seem to express by noting the ambiguous use of the word "write". In one sense of that term it would imply that John Rees was the author of the said work; in another quite ordinary sense it might suggest that the book wasn't hand-written, but was perhaps word-processed. [Or, even that Rees had used an amanuensis.] In that case, B1 would be expressing the fact that although John Rees authored the said book he didn't hand-write it (or he didn't do so himself); he, or someone else, typed it. It would then be clear that B1 only appeared to be contradictory because of a simple equivocation. We wouldn't automatically think that there were 'real material forces' at work behind the 'struggle' to produce the said book, no matter how well-confirmed each half of B1, or even their conjunction, happened to be.
This shows that observation or empirical checks in such circumstances aren't relevant to what is in fact a logical or a conceptual issue.
Again, someone might object, arguing that the above considerations highlight the LIE implicit in conclusions like this, for it seems to restrict the options available by appealing to logical or linguistic protocols. This would appear to make language the arbiter of truth, not the facts.
[LIE = Linguistic Idealism; that term is explained here.]
But, that would be to mistake the approach adopted at this site for its opposite.
The strategy employed here seeks to undermine the idea that substantive truths about reality can be derived (solely) from logical, conceptual or even contingent features of language. In this case, that has been done on the basis of what we would now try to do (prior to and independently of the adoption of a philosophical theory), in order to interpret or understand what might appear to be a contradiction as and when that situation might arise. Hence, these Essays appeal to rules we already employ (or with which we comply -- i.e., normative social practices that shape how we already use language), not a series of Super-Truths that some try to infer from a misconstrual of their nature.
Hence, no 'philosophical truths' are being derived (by me) from the above observations, merely a denial that any such truths can be obtained from the misinterpretation of a string of words.
Indeed, it is the opposite (dialectical) view that collapses into LIE, for it confuses linguistic and logical rules with empirical -- or, what are in effect treated as Super-Empirical -- truths. In DM, this occurs when, for example, dialecticians treat the LOC as a supposed truth that they think could be (and often is) false -- or which is at least only true 'within certain limits' -- especially in relation to motion and change. Their criticism of the LOC leads them to argue that contradictions themselves can be true (or even that they actually exist in nature and society). But, if, as noted earlier, the LOC is a rule (or it expresses a rule we ordinarily employ in relation to specific uses of the negative particle), it isn't the sort of thing that could be true or false, no more than orders or instructions can be.
[Further ruminations on this theme will be resumed in Essay Twelve Part One, where it will be argued in detail why the aforementioned confusion of rules with substantive truths about the world is endemic to, and drives the development of, Traditional Thought and DM. Spoiler: this is because that approach to 'philosophical knowledge' is predicated on the ancient, mystical idea that there is a 'hidden world anterior to experience', accessible to thought alone, that is more real than the world we see around us. It is from ideologically-inspired theories like this that Metaphysics and 'dialectics' originally arose.]
B1: John Rees wrote and did not write The Algebra of Revolution.
Nevertheless, it could be objected that DM-theorists' interest is restricted to the study of real material forces at work inside Capitalism, in order to assist in its demise/overthrow. In that case, trite examples like B1 aren't remotely relevant. Nor are they even dialectical contradictions.
Or, so it could be argued...
In order to counter that response, the sort of contradictions DM-theorists are interested in will be analysed elsewhere at this site (and in unprecedented detail, too -- for example, here, here and here -- as well as later in this Essay, here). There, it will be shown that "real material contradictions" turn out not to be contradictions to begin with (in any sense of the word -- on that, see here and here), and they can't be turned into "real material contradictions" howsoever they are interpreted or 'surgically enhanced'.
With respect to the other assertion advanced above -- that we would fail to understand alternative conventions given those we already have -- the key point is that as social beings we may only succeed in understanding something when (plainly!) it is presented to us in a language and a form with which we are familiar. Typically, but not exclusively, that takes place in ordinary language. And that, too, can be asserted with some confidence since the word "understand" is already (and patently) an ordinary language term. [The significance of that specific point will emerge in Essay Thirteen Part Three.] Clearly, language isn't a free-floating phenomenon. As all Marxists agree (at least in principle), its invention and subsequent evolution were, and still are, a function of our social, historical and technological progress or development (albeit heavily constrained by class division, the class war itself, and all that that entails). In addition, our use of language is subject to the constraints we inherited from previous generations, which we plainly had no hand in shaping (since we have no access to a time machine!). Indeed, every single one of us had to be socialised by parents, siblings, carers, teachers and peers (etc.) into using language within, and in compliance with, these constraints. As social beings, we manifestly didn't individually socialise ourselves (although each of us certainly adapt and mould these linguistic and social resources and conventions to our own ends).
While we can form thoughts as we please, we can't do so under logico-linguistic and social conditions of our own choosing (to paraphrase Marx):
"Human beings make their own language, but they do not make it as they please; they do not make it under circumstances of their own choosing, but under circumstances that already exist, given and transmitted from the past." [Edited misquotation of Marx (1968), p.96.]
As should seem obvious, we reveal a mastery of this complex socio-linguistic tool (language) as we begin to communicate and interact with one another and the world around us.
Now, it is tempting to think that these 'limitations' present some sort of physical barrier -- or, at least, that they merely represent contingent constraints on our use of language, but that, too, would be a mistake. There certainly are physical and contingent constraints on language (for example, no one could utter or understand a trillion word sentence, or one spoken in the space of a millisecond), but those aren't the limitations intended.
[A clue concerning the nature of these limitations may be ascertained by anyone who reads the Essays posted at this site, especially where it has been demonstrated time-after-time how readily DM-theories fall apart and can't be repaired no matter what is done with them. That sort of limitation isn't physical; it is conceptual. Another such example was outlined, here; several more will be aired in this Essay. However, these limitations aren't those that words exercise upon us; the word "limitations" underlines how we collectively -- through our socialisation -- understand and thus use the vocabulary and grammar we already have. To suppose otherwise would be to fetishise language, turning it into the agent, and humanity into the patient. (Incidentally, I am using the word "patient" here in its older sense; that is, it relates to that which is acted upon, not that which acts.)]
B1: John Rees wrote and did not write The Algebra of Revolution.
Fortunately, however, the negative criticisms of DM laid out at this site don't depend on the validity of this latest batch of seemingly dogmatic assertions. Doubters need only think about how they themselves would interpret B1 (or, indeed, B2, below), and the point should become a little clearer.
In relation to understanding others who currently speak a different language from English, or even characters from the past who did, while we often (obviously) translate what they have to say into our own language, we may do so only within the constraints that currently operate on our use of that language. [Unless, of course, we aim to restrict ourselves merely to transliteration.] This means that because we can't make sense of contradictory speech now, we would also find it equally difficult to comprehend how contradictions could ever have been held true by anyone using different languages, either now or in the past.
Of course, there have been, and there still are mystics who utter and promulgate all manner of odd, or even contradictory, ideas. Other than Hegel, this more typically includes, for instance, Buddhist mystics, logicians and 'teachers' -- even though it is still a moot point whether anyone has ever actually understood the peculiar things they come out with. Indeed, mystics themselves tell us they don't understand the conundrums they cough up, which is part of their superficial appeal. Would anyone listen to those claiming they were communicating the 'divine word' if the 'message' they conveyed were straight-forward and easy to grasp? Mysticism and obfuscation go hand-in-hand -- as, it seems, is also the case with DM.
Francis Bacon summed-up this mind-set admirably well (although he confined his criticism to the tangled verbal spaghetti weaved by Medieval Schoolmen, i.e., the Scholastics, but it clearly applies more generally):
"This kind of degenerate learning did chiefly reign amongst the Schoolmen: who having sharp and strong wits, and abundance of leisure, and small variety of reading, but their wits being shut up in the cells of a few authors (chiefly Aristotle their dictator) as their persons were shut up in the cells of monasteries and colleges, and knowing little history, either of nature or time, did out of no great quantity of matter and infinite agitation of wit spin out unto those laborious webs of learning which are extant in their books. For the wit and mind of man, if it work upon matter, which is the contemplation of the creatures of God, works according to the stuff, and is limited thereby; but if it work upon itself, as the spider works his web, then it is endless, and brings forth indeed cobwebs of learning, admirable for the fineness of thread and work, but of no substance or profit." [Bacon (2001), pp.25-26. Bold emphasis added; Stuart/Elizabethan words have been replaced by their contemporary English equivalents.]
As one commentator noted about the reasoning behind the use of obscure jargon:
"Sociologist C. Wright Mills, in critically examining 'grand theorists' in his field who used verbosity to cover for a lack of profundity, pointed out that people respond positively to this kind of writing because they see it as 'a wondrous maze, fascinating precisely because of its often splendid lack of intelligibility.' But, Mills said, such writers are 'so rigidly confined to such high levels of abstraction that the "typologies" they make up -- and the work they do to make them up -- seem more often an arid game of Concepts than an effort to define systematically -- which is to say, in a clear and orderly way, the problems at hand, and to guide our efforts to solve them.'
"Obscurantism is more than a desperate attempt to feign novelty, though. It's also a tactic for badgering readers into deference to the writer's authority. Nobody can be sure they are comprehending the author's meaning, which has the effect of making the reader feel deeply inferior and in awe of the writer's towering knowledge, knowledge that must exist on a level so much higher than that of ordinary mortals that we are incapable of even beginning to appreciate it.... The harder people have to work to figure out what you're saying, the more accomplished they'll feel when they figure it out, and the more sophisticated you will appear. Everybody wins." [Quoted from here. Quotation marks altered to conform with the conventions adopted at this site. One link added; several paragraphs merged.]
I don't often quote Nietzsche, but these words of his seem uncannily relevant:
"Those who know that they are profound strive for clarity. Those who would like to seem profound to the crowd strive for obscurity. For the crowd believes that if it cannot see to the bottom of something it must be profound." [Quoted from here.]
Finally on this point, no less a DM-fan than Lenin agreed with the above about the use of obscure language, even as he (inconsistently) quoted page-after-page of it from Hegel(!):
"The flaunting of high-sounding phrases is characteristic of the declassed petty-bourgeois intellectuals." ["Left-Wing" Childishness.]
It's a pity Lenin didn't take the implied advice the above remark seems to present.
[There will be more on this in Essay Fourteen Parts One and Two.]
In addition, we are equally incapable of translating (note: translating, not transliterating) any language, ancient or modern, into our own in comprehensible terms while attempting to depict its users employing contradictory speech while holding any such contradictions true, or even proceeding with the assumption that those who engaged in such speech also held them true. That doesn't imply we have to reject the idea (as false) that such individuals actually believed these contradictions were true (we can suspend judgement, for instance), but we certainly can't hold them true. While we might acknowledge the fact that some individuals (in the past, or whenever) speak, or have spoken, in paradoxical ways, given what we currently mean by the words we use it is now impossible to make sense of the supposed 'content' that these ancient/mystical 'sayings' could have presented to those who wrote or uttered them. Or, indeed, determine whether or not they represented anything determinate, to begin with. Moreover, since odd individuals like this invariably fail to explain themselves (or, at least, in ways that aren't equally obscure), it is quite clear they couldn't make sense of their own words, either.
Of course, mystics often assert that what they have to say deals with the "ineffable", which, by definition, can't be put into words.
But that just makes my point for me!
In like manner, DM-fans can't explain their 'contradictions', either, and regularly complain that critics don't "understand" dialectics. Or, as is often the case with Academic Marxists and HCDs, the 'explanations' they come out with are no less obscure, expressed in jargon that is, to coin a phrase, as 'clear as mud'. [A recent 'debate' I had with one such individual illustrates this point admirably well. I have also quoted several examples of the obscure use of language (by Marxist academics) in Essay Nine Part Two.]
[HCD = High Church Dialectician; follow the link for an explanation.]
In which case, if it is now impossible to make sense of the claim that individuals in the past could have held certain contradictions true, we plainly can't understand the supposition that contradictions could ever have been true, whether or not some might (now) imagine they had been viewed that way by such individuals.
[Once again, the various 'true contradictions' to which DM-theorists appeal will be examined elsewhere at this site (for example, follow the links posted above). Graham Priest's much more detailed (and logically well-informed) attempt to defend the idea that there can be, and actually are, 'true contradictions' in nature and society will be examined in a later Essay. In the meantime, readers are directed to the following critics of his: Berto (2007), Goldstein (1992, 2004), Field (2008) and Slater (2002, 2007a), as well as this review. Update: I have now added a few comments to Note 18a concerning Priest's much more sophisticated attempt to show that motion and change are contradictory; or as he puts it: "I {have argued in support of} the idea that contradictions not only occur in certain sorts of change but actually are the states of change themselves." [Priest (2006), p.172.]
Some might take exception to many of the above assertions, perhaps claiming that they can imagine speakers holding certain contradictions true (and, what is more, those contradictions actually do represent real material forces/conflicts), namely they, themselves! So, the following question naturally arises: are dialecticians themselves living disproof of the above sweeping generalisations?
In response, the present Essay will show that Hegel and Engels's theory (that motion is, or involves, a 'contradiction') is far too vague and confused for it to be assessed for its truth or its falsehood (it doesn't make it that far!). This means that the 'contradiction' they and their followers claim to 'see' in moving bodies isn't a contradiction in any sense of that word -- nor is it even 'dialectical'! Other examples of 'dialectical contradictions' will similarly be dealt with in Essay Eight Parts One, Two and Three, as well as Essay Eleven Part One. In addition, the theory that reality itself contains, or is composed of, countless trillion UOs in each kilogram of matter (namely, each sub-atomic 'particle') will be destructively criticised in Essay Seven Parts One and Three.
[UOs = Unity of Opposites.]
Hence, because it isn't possible to make sense of any of the examples of 'dialectical contradictions' offered up by DM-fans, the above "sweeping generalisations" have everything going for them. The fact that dialecticians have shown that they themselves are incapable of explaining these mysterious 'contradictions' to anyone -- least of all to one another -- provides further supporting evidence in its favour. [On that, see here.] Indeed, on Internet discussion boards, when Academic Marxists and revolutionaries alike are asked (often repeatedly, by the present author and others) to explain exactly what 'dialectical contradictions' are they all fail miserably to do so --, or, they just ignore the question. [Links to many of those discussions have been posted here.]
Admittedly, as noted earlier, there have been -- just as there still are -- mystics and religious believers who come out with, or even assent to, all manner of apparently contradictory ideas, but that doesn't falsify the above counter-claims. Their talk is often non-propositional -- indeed, it is frequently little more than wall-to-wall, incoherent babble, as will be demonstrated in a later Essay. The same comment applies to 'ideas' expressed by Buddhists (this links to a PDF) -- more specifically, Zen Buddhists -- who also seem to glory in paradox and the production of endless streams of gobbledygook.
[Concerning the odd things Buddhists come out with, see my other comments below.]
In relation to the earlier claim that we wouldn't be able to make sense even of the possibility that there might have been individuals in past generations who believed, or who could have believed, there were true contradictions, consider this example:
B2: This four thousand year old inscription says that its author wrote and did not write it.
Now, despite the fact that dialecticians assure us that reality is contradictory, not even they would attempt to understand B2 literally. That isn't because it would be particularly difficult for them to do so, but because any claim to the contrary would undermine the meaning of the word "literally", at the very least.
But, even supposing a few Mad Dog Dialecticians [MDDs] could be found who did attempt to do this, they would find it impossible to explain to anyone else in literal terms what sense they made of B2 -- other than by disambiguating it.
[Again, see below for a few instances of such.]
As noted earlier, trite examples like B2 were deliberately chosen to illustrate a point that is all too easily missed: when faced with the paradoxical things people sometimes say, we automatically make an effort to disambiguate their words or their actions. We often adopt what Donald Davidson once called the "principle of charity" when trying to grasp either their meaning or their intentions. [Davidson (2001).]
[Of course, in so doing we have to distinguish between speakers' meaning (i.e., what an individual hopes to convey or achieve by his/her words) and word/sentence meaning (i.e., what such words actually mean in the language). Failure to do so leads to the sort of confusion that undermines, for example, Voloshinov's work, as well as that of his epigones. (I have discussed this phenomenon in extensive detail in Essay Thirteen Part Three.)]
Hence, when confronted with someone who asserts an apparent contradiction we would normally employ the above policy -- trivial examples excepted, of course. That doesn't mean this approach will necessarily distort what the said individual has uttered or written; rather, it is that we wouldn't be able to understand such individuals if we didn't do this.
In any case, if there are any MDDs out there (i.e., extremist DM-supporters who reject some or all of the above points), they would be hard-pressed to explain to anyone else what they themselves took the sense of a true contradiction to be -- that is, without playing yet another Nixon card --, as the rest of this Essay and this site both aim to show.
And that comment also applies to any 'dialectically-inspired' responses elicited from those who might think to question the above claims.
Clearly, that doesn't mean we shouldn't exercise some level of sensitivity toward other belief systems (past or present), but we may only do so in terms of current linguistic and social protocols. When confronted with what appear to be weird or paradoxical beliefs, it would prove to be impossible to translate them and interpret them literally while at the same time claiming to understand them (disambiguation excepted, again). On the other hand, if someone claimed they could do this, it would automatically throw into doubt the validity of their translation (unless the meaning of the word "translate" itself had been modified/altered) -- always supposing, of course, that they hadn't merely transliterated the relevant words instead.
However, if what had been 'translated' were held to be literally true, and it still remained paradoxical, then whatever else could be made of it, all talk of its literal truth would have to be abandoned. Either that, or, once again, we would have to understand the words "literal" and "truth" non-literally!
[This topic is currently under intense and lively debate; on this, see, for instance, Miguens (2019), Creary and Read (2000) and Cerbone (2000). See also Conant (1991) and Forster (1998).]
So, unless and until DM-theorists explain themselves (in non-paradoxical terms), we are forced to conclude that 'dialectical contradictions' fail to depict, or express, anything in any meaningful sense (that is, other than the confused state of mind exhibited by anyone who thought contradictions could be true). [On that, see Slater (2007a).]
The above isn't being asserted because I personally think that reality contains no contradictions, or because I have concluded that the world either is or isn't as these allegedly 'true contradictions' supposedly depict it -- or even because contradictions are always false (which is the classical view). To argue along those lines would be to fall into the same trap that ensnares DM-theorists, since it would amount to the derivation of yet another dogmatic theory about reality that was the opposite of the one promoted by DM-theorists, and which was based on an alternative linguistic convention (which I might, in this case, have found more acceptable).
On the contrary, contradictions fail to picture the world, not because they are false, but because they aren't pictures to begin with. They represent a breakdown in the depictive and representational capacities of language, since they violate socially-grounded rules we already have for the use of the negative particle. [On that, see Essay Twelve Part One.]
Finally, it could be argued that the above comments are misguided since dialecticians don't question the general application of principles drawn from FL, such as the LOC; they merely point to their limitations when applied to motion and change. In that case, the contradictions illustrated by B1 and B2 are completely irrelevant.
B1: John Rees wrote and did not write The Algebra of Revolution.
B2: This four thousand year old inscription says that its author wrote and did not write it.
Such a critic might continue to object on the basis that since the above even don't depict change, they are doubly beside the point.
Or, so it could be argued...
That particular response will be put under considerable pressure throughout this site, where it will be shown that it is dialecticians who can't actually account for motion and change, irrespective of the examples chosen.
Update 12/02/2010: A story on the BBC website ("Do speedy elephants walk or run?") illustrates how an "either-or" question is actually answered by scientists without them having to agree with Hegel, or even (once) consult his work:
"Instead of speaking by the maxim of Excluded Middle (which is the maxim of abstract understanding) we should rather say: Everything is opposite. Neither in heaven nor in Earth, neither in the world of mind nor of nature, is there anywhere such an abstract 'either-or' as the understanding maintains. Whatever exists is concrete, with difference and opposition in itself. The finitude of things will then lie in the want of correspondence between their immediate being, and what they essentially are. Thus, in inorganic nature, the acid is implicitly at the same time the base: in other words, its only being consists in its relation to its other. Hence also the acid is not something that persists quietly in the contrast: it is always in effort to realise what it potentially is." [Hegel (1975), p.174; Essence as Ground of Existence, §119. Bold emphasis added. The ridiculous nature of these words was exposed earlier.]
The answer scientists give in this case is that elephants do both, they run and walk. Is this a contradiction? Does it undermine any of the earlier claims made in this Essay? Can this apparent 'anomaly' be resolved -- DM-style, maybe, by means of a series of a priori, dogmatic assertions about all of reality, for all of time? Or, can it be defused by some form of disambiguation? Which tactic is going to work? Which one was actually used?
Well, here is the article's explanation how this conundrum was in fact resolved:
"With their awkward, lumbering gait, elephants moving at high speed are not the most graceful of animals -- but are they walking or running? Now scientists believe they have an answer: new research confirms that they do both -- at the same time. By observing elephants moving across a hi-tech track, the team found the hefty creatures run with their front legs but walk with their back legs. The research is published in the Journal of Experimental Biology. Earlier research had suggested that elephants perform a strange, part-walk/part-run while travelling at speed. But a team from Belgium, Italy and Thailand was able to investigate this further by using a specially built track that was able to precisely measure (sic) the forces exerted with each weighty elephant step. Professor Norman Heglund, an author of the paper from the Catholic University of Louvain, Belgium, told BBC News: 'We had to build the plates -- you just can't go down to your local hardware shop and pick up an elephant-sized force plate.' Armed with these, the researchers headed to the Thai Elephant Conservation Centre to study the big beasts, which ranged from an 870kg baby to a four tonne adult. The Asian elephants were encouraged to move across the track, at speed, by their keepers....
"They were...filmed using high-speed cameras. By comparing the measurements from the sensitive force-measuring platform with each frame of the footage, the scientists were able to look at every tiny movement that the elephants were making. This enabled them to calculate the amounts of potential energy (stored energy) and kinetic energy (the energy that is associated movement), that the creatures were using. Measuring the relationship between potential and kinetic energy is the key to defining whether something is walking or running. For example, when walking, as an animal raises its foot from the ground and moves it forwards, it is converting the stored energy in its muscles and tendons -- the potential energy -- into kinetic energy. As its foot lands, the kinetic energy converts back into potential energy, and then back into kinetic energy as the foot is once again raised, and so on. All the time the creature is walking, the energy is transferred back and forth between potential and kinetic energy.
"But while running, the exchange between potential energy and kinetic energy is continuous -- rather than one form of energy being recycled into the other, back and forth, the energy exchange is happening all the time. Professor Heglund explains: 'The running gait, in most animals, is a bouncing mechanism. In this case, the potential and kinetic energy are in phase, they both hit a maximum at the same time and a minimum at the same time, so they cannot be transferred back and forth.' However, the researchers found that fast-moving elephants seem to both run and walk at the same time. Professor Heglund said: 'When an elephant goes at higher and higher speeds, the kinetic and potential energy shift and start to become more in phase. But when we looked in detail, we see that the animal appears to be running -- bouncing -- with the front legs, and walking with the back legs. It is as if he is getting up to a transition speed where he wants to transition from a walk to a run, but he can't quite do it. It's like he can't quite get up into second gear.'...
"The scientists now plan to look at other large animals, such as hippos and rhinos, to find out if they run or walk. This latest study confirms the findings of other research, published in the journal Nature and the Journal of Experimental Biology, that have previously shown that elephants perform a run-walk hybrid. However, there are some differences -- while this latest paper suggests the front legs run and the back legs walk, the other studies suggested the opposite." [Quoted from here. Bold emphasis alone added. Several paragraphs merged. Minor typo corrected.]
Hence, this apparent contradiction was resolved by detailed observations (coupled with an appeal to clear definitions, in tandem with a modicum of common sense), which led to a new discovery: that elephants run with their front legs and walk with their back legs (or the other way round!). Had these researchers been dialecticians, it is highly unlikely this advance would ever have been made; we would merely have been told to "grasp" this 'contradiction' and move on (no pun intended).3a
So, dear reader, which strategy was actually used?
Those who think this is unfair, and who might argue that no DM-theorist of any sophistication would resolve this apparent contradiction in the way suggested earlier, need only read the truly weird things DM-theorists have to say about motion (covered in this Essay), the LOI (Essay Six), wave-particle duality (Essay Seven Part One), change (Essay Seven Part Three), FL (Essay Four), Mathematics (Essay Seven Part One), universal inter-connection (Essay Eleven Part One) or the relation between parts and wholes (Essay Eleven Part Two), to see that the negative comments above were not just well deserved, they are on point.
Update 06/09/2023: Well, we needn't invent problems for hypothetical DM-fans to try and solve, we have several they happily volunteer (in addition to Engels's theory of motion) -- we might even label this 'dialectically leading with your chin'. A handful were volunteered in an article co-authored by Graham Priest, which lists several contradictions Buddhists have come out with in the past. Here are two such:
W1: "What the realised one has described as the possession of distinctive features is itself the non-possession of distinctive features."
W2: "Everything is real and is not real".
[Both of the above come from an on-line article by Yasuo Deguchi, Jay Garfield and Graham Priest (this links to a PDF).]
With respect to W1, it would be interesting to see the above three authors make any attempt to explain what "distinctive features" means without that explanation itself having "distinctive features" of its own. [They didn't!]
Independently of that, W1 isn't even a contradiction! [It certainly isn't 'dialectical'!] That is because: (i) "What the realised one has described as the possession of distinctive features" is plainly not the same as what this refers to, (ii) "the non-possession of distinctive features". (i) refers to some other subject of the conversation, while (ii) refers to (i), not what (i) itself is talking about!
So, W1 is really saying this:
W3: "The realised one has described X as the possession of distinctive features, but 'The realised one has described X as the possession of distinctive features' is itself the non-possession of distinctive features."
The second half is talking about what "the realised one" is saying, while the first half is talking about "X". So, this is no contradiction, even if it is an odd thing to come out with -- but it is, alas, standard fare for those whose aim seems to be to confuse the easily confused (and maybe even separate them from their money).
What about W2?
W2: "Everything is real and is not real".
That certainly looks contradictory, but can anyone hold it true? If so, it would be interesting to see them try explain how it could be true -- what state of affairs it could conceivably picture or represent. Certainly the above three authors didn't even attempt to do that. In fact, they said the following:
"Some may argue that none of these contradictions is meant to be accepted as true, that each should, in fact, be interpreted in some other way. Others may argue that the contradictions are meant to be taken this way, but that this shows that the views espoused are some kind of irrational mysticism. The point of the present note is to examine the matter. We will argue that at least some contradictions found in the texts are indeed meant literally and to be accepted as true. We will also argue that this is not a mark of irrationality, but, indeed, a consequence of rationality itself." [Quoted from here. (This links to a PDF.)]
Although they say some of these 'contradictions' were "meant literally" and were "accepted as true", they neglected to say what that (supposed) truth actually consisted in, or in what way any were indeed "literal".
So, this philosophical cheque has yet to be cashed...
Even worse, as yet we have no idea what its cash value even is!
[By "cash value" I simply mean we have no idea what the sentence is even trying to propose. If true, what state of the world has made, or makes, it true? (No answer was forthcoming from the authors of this article.) On the other hand, of course, if it isn't true, we can ignore it.]
But, even worse: with respect to W2, "everything" is said to be "real", but in the next breath that is (supposed) 'fact' is denied. But who is to say the first "everything" is quantifying over the same set or class as the second? If a shop has this notice stuck to its main window "Mammoth Sale Today!! Everything Must Go!" who in their left mind would interpret that "everything" as ranging over the same set or class as the same word in the following sentence? "The cops sealed the crime scene so that everything remained where it had been left by the unknown assailant." So, while it seems reasonably clear in relation to the shop and the crime scene example that the word "everything" doesn't always range over the same set or class, that appears to be less clear in relation to W2. But even if it is less obvious, it is still clear that with respect to W2 the two "everythings" can't be ranging over the same set or class since one such set/class is said to be "real", while that is denied of the other. The two sets/classes concerned are thus totally different, which means that the two occurrences of "everything" can't be equated, and hence W2 can't even be a contradiction.
[I have entered in to this topic in more detail in Essay Eight Part Three, here, here, here and here.]
Readers are invited to check the article (by Yasuo Deguchi, Jay Garfield and Graham Priest) for themselves to see if I have misrepresented what its authors said -- or didn't say.
They do say this, though:
"It might be suggested that although such contradictions are true, their truth is in comprehensible. Such truths, in this view, have the deictic function of ostending the incomprehensibility of ultimate reality, but cannot themselves be understood. This view concedes our point that such contradictions are intended as true, but we do not concede the view that they are incomprehensible. Those who hold that contradictions are always and obviously only false will of course find supposing them to be true incomprehensible. However, despite various orthodoxies, East and West, the view that some contradictions are true is a perfectly coherent and intelligible view, as modern studies in dialetheism and paraconsistency have established." [Ibid. (This links to a PDF.) Links added.]
Well, until they succeed in explaining these 'contradictions', the conclusion must remain that they are indeed incomprehensible, and can in no way be true, or held to be true.
Finally, their closing appeal to paraconsistency and dialetheism should be taken with a large pinch of salt until those who promote such doctrines explain how these other 'contradictions' can also be true. So, far they have failed miserably.
[I deal with one such in Note 18a. See also my earlier comments about Priest's rather odd ideas, and my other remarks about his theory, 'dialetheism, throughout Essay Four.]
Once again, W1 and W2 aren't the sort of contradiction of interest to DM-theorists. But, as noted earlier, the types of contradiction that do concern them have been dealt with at length later in this Essay (in fact, beginning in the next main Section), in Essay Eight Parts One, Two, and Three, and Essay Eleven Part One.
W1: "What the realised one has described as the possession of distinctive features is itself the non-possession of distinctive features."
W2: "Everything is real and is not real".
In which case, my claim still stands: it is impossible to hold any of these 'contradictions' true, or say the same about anyone who thinks they are even 'contradictions', let alone 'dialectical' to begin with.
Initial Problems With Engels's Theory
We are now in position to examine Engels's actual argument in support of his theory that motion is a 'contradiction':
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152.]
However, and annoyingly, there are five initial difficulties with the above passage that must first of all be addressed.
(1) "Asserted" By Whom?
Engels's closing sentence is rather odd:
"Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Ibid. Bold emphasis added.]
Exactly who is supposed to do the "asserting" and who the "solving", here? It could be argued that these words were meant to be taken metaphorically. But, if that is so, what then is the force of Engels's use of the word, "precisely"?
Even more to the point: if Engels were speaking figuratively, what would "assertion and simultaneous solution" have to do with motion? This isn't even a good metaphor!
Perhaps Engels intended to say that his words merely apply to the description of motion? In that case, the conclusions he reached will be limited to the language we use to talk about motion, not 'motion itself'.4
An article by Thomas Weston [i.e., Weston (2012)] appears to have an answer to some of the above questions. His detailed analysis will be examined in Appendix A.
[Spoiler Alert - 01: As Appendix A shows, Weston failed miserably to explain how such 'contradictions' can be "solved"/'resolved'.]
(2) "Solved" In What way?
How exactly are contradictions "solved"? Are they like puzzles, riddles, brainteasers, mazes and mysteries? If they are, do they disappear once they have been "solved"? Riddles, brainteasers, puzzles, mazes and mysteries cease to be problematic when they have been unravelled. Is that the same with these contradictions?4a If so, do new contradictions immediately take their place? In which case, is each "solved" contradiction replaced by the 'same' contradiction further along the trajectory of that moving body or by an entirely new one? How might any of this be confirmed? And, how do we know if there is only one contradiction present, or countless thousands (for each unit of time involved)? If there is more than one contradiction, how are they all connected with a given body in motion? Does each contradiction arise and fall as that body moves? Or is there a single, extended contradiction spread out, as it were, right along the entire trajectory? Is this 'extended contradiction' then perhaps the following: that a moving body is "here and not here, in general", so to speak? [Whatever that means!]
More puzzling still: Are these contradictions "solved" by some mind or 'intelligence' first comprehending them? If not, what sense can be given to the word "solved"? And, what precisely is there to understand in a contradiction so that a 'solution' is required in the first place? This now mysteriously still helps further propel the moving object along (if that is what it does). If so, how does a 'solved contradiction' manage to do that? On the other hand, if a 'solution' is required, how was that achieved before human beings evolved?
[Again, Thomas Weston appears to have an answer to some of these questions. His response will be examined in Appendix A.]
[Spoiler Alert - 02: As Appendix A shows, Weston failed miserably to explain how such 'contradictions' can be "solved"/'resolved'.]
At first sight, Engels appears to be arguing that it is only our understanding of motion that is contradictory:
"[A]s soon as we consider things…then we…become involved in contradictions…." [Engels (1976), p.152. Bold emphases added.]
This might help explain why the passage refers to the "continual assertion" of such contradictions, since it is evident that only human beings can assert anything. If so, it looks like Engels thought that human observers can't avoid "asserting" such contradictions, or maybe asserting there are contradictions present whenever they attempt to describe or conceptualise motion. That in turn might itself be a consequence of a partial understanding of the 'absolute truth' about motion (if such exists). On the other hand, the fact that this presents humanity with a conundrum could be a fault of logic, or even of language, both of which are said by DM-theorists to be inadequate to the task (i.e., with respect to change, etc.). But, that would fail to explain how and why contradictions, upon being "asserted", are immediately "solved" and then promptly re-"asserted". If we don't understand such contradictions, how can they be 'solved'? On the other hand, if they are 'solved', why re-assert them?
The above considerations would appear to mean that it is only human understanding of motion that is contradictory, not 'reality itself' -- unless, of course, we are to suppose that nature is Mind (or the product of Mind). If that were the case, it would certainly help explain why Engels said such contradictions are "asserted". Maybe he meant that it is the 'self-development of Mind' that propels bodies along (which is a faint echo of Hegel's theology)? After all, Engels was brought up in the strict Pietist faith, which was openly based on Jakob Böhme's (Hermetic) theology, who was also an important formative influence on Hegel. [On Pietism, Engels, Böhme and Hegel, see Martin (2014), Boer (2013), especially Chapter Nine, Green (2008), Carver (1989), Hunt (2009) and Magee (2008).]
As Engels himself acknowledged in a letter:
"But...our good Lord God has given me an excellent sense of humour.... If I had not been brought up in the most extreme orthodoxy and piety, if I had not had drummed into me in church, Sunday school and at home the most direct, unconditional belief in the Bible and in the agreement of the teaching of the Bible with that of the church, indeed, with the special teaching of every minister, perhaps, I would have remained stuck in some sort of liberal supernaturalism for a long time." [Engels to Wilhelm Graeber, 30/071839, in Marx and Engels (1975b), pp.465-66. Italic emphasis in the original.]
Anyway, one of the alternatives aired above suggests that when reality is fully understood all such contradictions would cease, desist or disappear. Indeed, Engels himself believed they would:
"If one does not loiter here needlessly, but presses on farther into the immense building, one finds innumerable treasures which today still possess undiminished value. With all philosophers it is precisely the 'system' which is perishable; and for the simple reason that it springs from an imperishable desire of the human mind -- the desire to overcome all contradictions. But if all contradictions are once and for all disposed of, we shall have arrived at so-called absolute truth -- world history will be at an end. And yet it has to continue, although there is nothing left for it to do -- hence, a new, insoluble contradiction. As soon as we have once realized -- and in the long run no one has helped us to realize it more than Hegel himself -- that the task of philosophy thus stated means nothing but the task that a single philosopher should accomplish that which can only be accomplished by the entire human race in its progressive development -- as soon as we realize that, there is an end to all philosophy in the hitherto accepted sense of the word. One leaves alone 'absolute truth', which is unattainable along this path or by any single individual; instead, one pursues attainable relative truths along the path of the positive sciences, and the summation of their results by means of dialectical thinking. At any rate, with Hegel philosophy comes to an end; on the one hand, because in his system he summed up its whole development in the most splendid fashion; and on the other hand, because, even though unconsciously, he showed us the way out of the labyrinth of systems to real positive knowledge of the world." [Engels (1888), p.590. Spelling modified to agree with UK English; quotation marks altered to conform with the conventions adopted at this site. Minor typo corrected.]
The above passage appeared in published work, so it represents Engels's more considered thoughts. Here he argues that "if all contradictions are once and for all disposed of, we shall have arrived at so-called absolute truth...". That would appear to mean contradictions aren't 'objective' or "real" features of the world but are the product of our limited knowledge. That is, they are only "apparent". Even though 'absolute truth' will never be attained, the fact that there is such a thing as, or there is a possible state of knowledge that can be described as, "absolute truth" implies contradictions aren't really real but are a consequence of humanity's imperfect knowledge. Why else would they disappear? If they were 'objective' features of 'reality', they would still exist even if humanity attained 'absolute truth'. Engels wouldn't have surmised that "all contradictions" could be "once and for all disposed of" if contradictions were 'objective'. That is because only 'subjective' or 'apparent' contradictions (those that are a result perhaps of limited knowledge) can be "disposed of". Engels pointedly speaks about "all contradictions", which must include those supposedly involved in motion. This further implies that the more we know the fewer contradictions we should observe in nature and society, or the fewer we should assert actually exist.
Plainly, this in turn implies that motion could one day come to a halt, all contradictions having been "solved"/"disposed of"! Indeed, if contradictions actually 'cause' motion (or they are a consequence of it), then their complete resolution/disposal should freeze nature in its entirety. On the other hand, maybe motion will just stop being (or appearing to be) contradictory and will simply carry on as normal? Or, does this mean that nature will simply slow down as it is better understood (i.e., if what we know about motion and change becomes less and less partial/relative and hence less and less 'contradictory-looking')?
Who can say? Certainly not DM-fans. In the 150 or so years since Engels wrote these enigmatic words they have been more content merely to regurgitate them than they have been concerned to raise, let alone consider or attempt to answer, these glaringly obvious questions.
Admittedly, DM-theorists distinguish between subjective and objective dialectics -- the former relating to our (perhaps decreasingly) partial grasp of the 'nature of reality', the latter referring to processes in the 'objective world' independent of our will or our knowledge. Even so, it is still unclear how that helps answer the above questions. If the human mind "solves" the contradictions involved in motion, wouldn't that mean things just stop moving? Or wouldn't it confirm the suspicion that movement only seems to be contradictory because of the partial nature of human knowledge, implying that it isn't really contradictory? Clearly, that is because these 'subjective contradictions' ought to disappear as knowledge grows, which in turn means that (in the limit) 'reality' can't be 'contradictory', after all. In that case, it is only our 'one-sided'/'partial' knowledge of nature that fools us into concluding otherwise!
As should now seem obvious, this implies 'objective reality' is now actually contradiction-free, which it must be if knowledge is only slowly catching up with that fact.
Alternatively, this might suggest we don't really understand such 'contradictions', to begin with. But, if that were so, it would fail to explain why contradictions are promptly reasserted upon being "solved". Nor is it at all clear how they could be "solved" if no one understands them, or if no one fully understands 'reality' (since they aren't in possession of 'absolute knowledge').
Perhaps even more alarmingly, it might mean that the objects in question aren't really moving, as Zeno originally contended! That is, that there is no such thing as motion! If so, 'Reality; would therefore be the opposite of what we 'perceive' it to be -- which is another core principle Hegel dreamt up. Here is Herbert Marcuse expressing this rather odd idea:
"Under the rule of formal logic, the notion of the conflict between essence and appearance is expendable if not meaningless; the material content is neutralised; the principle of identity is separated from the principle of contradiction (contradictions are the fault of incorrect thinking); final causes are removed from the logical order....
"Existing as the living contradiction between essence and appearance, the objects of thought are of that 'inner negativity' which is the specific quality of their concept. The dialectical definition defines the movement of things from that which they are not to that which they are. The development of contradictory elements, which determines the structure of its object, also determines the structure of dialectical thought. The object of dialectical logic is neither the abstract, general form of objectivity, nor the abstract, general form of thought -- nor the data of immediate experience. Dialectical logic undoes the abstractions of formal logic and of transcendental philosophy, but it also denies the concreteness of immediate experience. To the extent to which this experience comes to rest with the things as they appear and happen to be, it is a limited and even false experience. It attains its truth if it has freed itself from the deceptive objectivity which conceals the factors behind the facts -- that is, if it understands its world as a historical universe, in which the established facts are the work of the historical practice of man. This practice (intellectual and material) is the reality in the data of experience; it is also the reality which dialectical logic comprehends." [Marcuse (1968), pp.114-17. Bold emphasis alone added.]
"The doctrine of Essence seeks to liberate knowledge from the worship of 'observable facts' and from the scientific common sense that imposes this worship.... The real field of knowledge is not the given fact about things as they are, but the critical evaluation of them as a prelude to passing beyond their given form. Knowledge deals with appearances in order to get beyond them. 'Everything, it is said, has an essence, that is, things really are not what they immediately show themselves. There is therefore something more to be done than merely rove from one quality to another and merely to advance from one qualitative to quantitative, and vice versa: there is a permanence in things, and that permanent is in the first instance their Essence.' The knowledge that appearance and essence do not jibe is the beginning of truth. The mark of dialectical thinking is the ability to distinguish the essential from the apparent process of reality and to grasp their relation." [Marcuse (1973), pp.145-46. Marcuse is here quoting Hegel (1975), p.163, §112. Quotation marks altered to conform with the conventions employed at this site. Minor typo corrected.]
What FL has got to do with any of this Marcuse stubbornly kept to himself, and it isn't hard to see why: it has nothing to do with it --, any more than basket weaving and stamp collecting have. Nevertheless, it seems that if Hegel says there is a clash of some sort between 'appearance' and 'essence', Marcuse is happy to take his word for it, with zero proof under his belt. Even worse, if correct, what 'appears' (to Marcuse) to be the case in Hegel's work must itself be subject to the same suspicion, that it too clashes with what Hegel in 'essence' was trying to say -- or, for that matter, between what Marcuse appears now to be saying and what he 'really' meant. If, on the other hand, there is no such mis-match between what either of these two 'appear' to be saying and what they 'essentially' intend, then Hegel and Marcuse need to explain why they get a pass when nothing else does.
[I have exposed the serious problems implied by this approach to philosophy -- also invented by Ancient Greek ruling-class theorists -- in Essay Three Part Two, here. I also explain why they invented this perverse way of viewing the world.]
Some readers might object, and claim that "appearances" are connected with transient existence while "essence" is linked with permanence. Those awkward facts mean the above comments are as misguided as they are ignorant.
Or so it might be argued...
I will deal with that objection in what follows (and at much greater length in Essay Three Part Two -- link above).
George Novack weighed in on this topic, too, with his very own brazen example of dogmatic apriorism:
"What distinguishes essence or essential reality from mere appearance? A thing is truly real if it is necessary, if its appearance truly corresponds to its essence, and only so long as it proves itself to be necessary. Hegel, being the most consistent idealist, sought the source of this necessity in the movement of the universal mind, in the Absolute Idea. Materialists, on the other hand, locate the roots of necessity in the objective world, in the material conditions and conflicting forces which create, sustain and destroy all things. But, from the purely logical standpoint, both schools of philosophy agree in connecting reality with necessity.
"Something acquires reality because the necessary conditions for its production and reproduction are objectively present and operative. It becomes more or less real in accordance with the changes in the external and internal circumstances of its development. It remains truly real only so long and insofar as it is necessary under the given conditions. Then, as conditions change, it loses its necessity and its reality and dissolves into mere appearance.
"Let us consider a few illustrations of this process, this contradiction between essence and appearance, resulting from the different forms assumed by matter in its motion. In the production of the plant, seed, bud, flower and fruit are all equally necessary phases or forms of its existence. Taken separately, each by itself, they are all equally real, equally necessary, equally rational phases of the plant's development. [We see here that Novack isn't ashamed to cite Hegel's batty idea about blossoms which we met earlier -- RL.]
"Yet each in turn becomes supplanted by the other and thereby becomes no less unnecessary and non-real. Each phase of the plant's manifestation appears as a reality and then is transformed in the course of development into an unreality or an appearance. This movement, triadic in this particular case, from unreality into reality and then back again to unreality, constitutes the essence, the inner movement behind all appearance. Appearance cannot be understood without an understanding of this process. It is this that determines whether any appearance in nature, society or in the mind is rational or non-rational." [Novack (1971), pp.86-87. Bold emphases added.]
As I have pointed out Essay Three Part Two about the above passage:
It isn't my immediate concern to criticise this (almost classic) example of mystical Natürphilosophie (however, it will be in a later Essay), but merely to note:
(a) The fanciful way that the term "contradiction" has been employed (without justification) by Novack; and,
(b) His idiosyncratic use of the word "appearance".
Exactly why a seed turning into a plant makes the seed an "appearance" Novack failed to say (except he regards Hegel's word on such matters as Gospel Truth), but why any of this is a 'contradiction' he left entirely mysterious. Of course, this might be a faint echo of an idea Hegel floated that anything that is transient or which lacks permanence is an 'appearance'. [Inwood (2002), pp.408-13.] Indeed, in relation to that it is worth asking how Novack knows that something is real only if its "appearance" coincides with its "essence" (always assuming that there are such things as 'essences', to begin with). That is, over and above merely accepting Hegel's diktat, to that effect, once more.
This peculiar belief is in turn connected with the idea that some things appear for a short time and then disappear or fade away, like someone who:
(i) Has a part in a play (as in "NN, now appearing in Death of a Salesman on Broadway");
(ii) Occupies an official position for a year;
(iii) Testifies in court (as in "NM is appearing for the prosecution");
(iv) Is famous only for fifteen minutes; or, maybe even when we,
(v) Describe how someone looks -- as in "Her appearance gave her away; she was clearly terrified", or "His appearance changes from day-to-day; he is a master of disguise."
In one or other of the above senses we might, at a stretch, say that a plant or a flower is "an appearance". At a stretch that is certainly a valid (if trivial) point, but it is still unclear what it has to do with 'essence' and 'appearance'. Originally, the point here was, of course, to contrast the transient existence of certain phenomenal objects and processes compared with those that are perhaps more permanent. For example, the play mentioned above might be on Broadway for a season, but Broadway will still be there after the play is well gone. So, it looks like 'essence' is somehow connected with permanence, 'appearance' with transience (no pun intended). And yet, do these more permanent features of the world have the 'ground of their being' within themselves (to paraphrase Hegel), or do they not also 'rely on God'? If the latter is the case, then even 'essences' are also 'appearances'. Will Broadway still be there in five billion years time? Will plants and seeds? I think there is room to suppose they might not. So, it seems they too are 'appearances', if we were to accept Hegel's defective typology.
Furthermore, what connection is there between 'appearances' that might deceive us -- like the way that sticks appear to bend when partially immersed in water or the way the Sun appears to rise in the East and fall in the West -- and the sort of 'appearances' mentioned in the previous paragraph, those associated with a lack of permanence? Presumably sticks will still look bent when partially immersed in water in ten million years' time, just as the apparent motion of the Sun will remain the same as long as the Sun, the Earth and human beings still exist. Given the considerations mentioned above, these 'appearances' must also be 'essences' since they look like permanent features of the natural world, which means, of course, that the distinction itself has now become absurd.
[I will return to consider such phenomena again, here, here and here.]
[Several passages from Hegel and others (including Marxist dialecticians) who promote the idea that there is a contradiction between underlying 'essence' and superficial 'appearance' (as noted earlier, this is a distinction that goes back as far as Parmenides and Plato -- ruling-class hacks, if ever there were any) are also quoted in Essay Three Part Two.]
So, this aspect of Hegel's loopy 'logic' seems to imply motion is an illusion, the opposite of the way it appears in everyday life. If so, that will explain why, for anyone (or any 'being') that possesses 'absolute knowledge' these 'contradictions' will have disappeared (indeed, as we saw Engels himself confirm earlier).
That also helps explain why Engels said this:
"…the continual assertion and simultaneous solution of this contradiction is precisely what motion is…." [Engels (1976), p.152. Bold emphasis added.]
This appears to confirm the idea that motion isn't really 'contradictory-in-itself', it is simply our 'one-sided'/'relative' perspective that misleads us into concluding otherwise. Here, Engels tells us that the "continual assertion" and "solution" of this contradiction is "precisely what motion is".
However, there is one small, nagging problem with that interpretation: Why then does he say this reveals "precisely" what motion is, as opposed to arguing that it merely depicts what we subjectively think it is? [It is a pity Engels didn't employ a sub-editor to check the consistency of his use of language! Or maybe, DM is meant to be full of just such inconsistencies -- as a sort of indirect 'reflection' of the way the world is supposed to be?]
Be that as it may, an appeal to "objective dialectics" can't help us comprehend Engels any the better, either. That is because neither assertions nor solutions occur in nature (apart, that is, from the intelligent beings who make or who advance them). And, if that is so, these non-objective assertions and solutions can't have been reflected in the mind of observers as part of an 'objective scientific theory', either; or, indeed, as part of 'objective dialectics'. If assertions and solutions don't actually exist in the world independent of the individuals who make or invent them, there would be nothing there, in 'extra-mental reality' for the minds of scientists and dialecticians to reflect or latch onto. As Lenin pointed out, objectivity is connected with "truth independent of man":
"To be a materialist is to acknowledge objective truth, which is revealed to us by our sense-organs. To acknowledge objective truth, i.e., truth not dependent upon man and mankind, is, in one way or another, to recognise absolute truth." [Lenin (1972), p.148. Bold emphasis added.]
"Knowledge can be useful biologically, useful in human practice, useful for the preservation of life, for the preservation of the species, only when it reflects objective truth, truth which is independent of man." [Ibid., p.157. Bold emphasis added.]
Since 'assertions' and 'solutions' are dependent on 'man', they can't be 'objective' (if Lenin is to be believed).
If so, what has assertion and solution got to do with motion in the real world, in the first place? And why did Engels think they were at all relevant?
So many questions -- so few answers...
(3) Yet More Vagueness
As we have seen, Engels informed his readers that:
"Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
More specifically, however, in relation to moving bodies it is pertinent to ask the following question that no one else ever seems to have asked: How far apart are the two proposed "places" that a moving object is supposed to occupy while at the same time not occupying one of them? Is there a minimum distance involved?
I can find no evidence that anyone -- in the entire history of philosophy, science and mathematics -- has ever bothered to ask this highly pertinent question. Why it is quite so important will soon become apparent.
The answer can't be "It doesn't matter, any distance will do." That is because, as we will see, if a moving object is in two places at once, then it can't truly be said to be in the first of these before it is in the second, since it is in both of them at the same time. So, unless great care is taken specifying how far apart these "two places" are, the DM-theory-of-motion would imply, for instance, that an aeroplane lands at its destination the same moment it takes off from the departing airport! If any distance will do, then the distance between the two airports involved is as good as any.
Indifference here, in this respect, would have you arriving at your destination at the very moment you left home!
[I return to this topic and discuss it in much more detail later in this Essay, where we will see that the absence of an answer to this question, least of all from Hegel or those who unwisely look to him for inspiration, has quite remarkable implications.]
Anyway, whatever the answer to that annoying question happens to be, between any two locations there is a potentially infinite number of intermediate points (that is, unless we are prepared to impose an a priori limitation on nature by denying this). As we will see, even Hegel acknowledged this point (no pun intended).
So, does a moving body:
(i) Occupy all of these intermediate points at once?
Or,
(ii) Does it only occupy each pair of such points successively? And which privileged pair is that, then?
Finally, for now:
(iii) Are these contiguous points, or is there a gap between them?
If (i) were the case, would that not imply a moving object can be in a potentially infinite number of places at the same time, not just in two, as Engels asserted? On the other hand, if Engels's words are meant to be interpreted strictly and literally, implying that a moving body occupies at most two places at once, wouldn't that suggest motion is discontinuous? As we are about to find out, it seems it does because that would picture motion as a peculiar stop-go, staccato sort of affair (that is, if a moving 'dialectical' object is restricted to being in at most two places at any one time). That in turn, also means it must be stationary at the second of those two locations, even if only momentarily.
[The following argument is based on the uncontroversial observation (explicitly accepted by Hegel, Lenin and also, it seems, by Trotsky (e.g., Trotsky (1971), pp.65-66)) that if a body is located at the same point for two contiguous instants in time, it must be stationary, even if only momentarily.]
This is why:
A1: Let us first of all suppose that body, B, is moving and occupies two points -- say p1 and p2 -- at the same time, t1 (in accord with Engels's theory).
A2: But, if B continues to move while it is at p2, it must still occupy two points at the same instant, t1, otherwise it will be motionless there (according to the "uncontroversial observation" noted above). If that were so, B would be located at p2 during two instants, t1 and t2, not one (here assuming these two 'moments in time' are themselves contiguous). But, if B is in that second place, p2, during these two instants it must be stationary there (even if only momentarily).
A3: On the other hand, if we reject the above conclusion and continue to assume B is still moving while it is located at p2, then, according to Engels, it must be the case that it occupies two places at once, p2 and p3, also at t1! But, as we will see later on in this Essay, that result will prove to be even more disastrous for Hegel and Engels's theory. So, in order to avoid that (as it turns out, fatal) result, we might now try to argue that B will be at p2 and p3 at a later moment, t2. That is because B has to be in two locations at the same time if it is still moving (according to Engels), and we have just rejected the possibility that B is in p2 and p3 at t1. But, that now means B must be located at p2 during two instants, t1 and t2, which, as we have also seen, implies it would now be motionless there (even if only momentarily).
A4: The bottom line here is that if B is still moving while in the second of these two locations, it must also occupy a third place at the same time, otherwise it would be stationary in that second location, since it would be there during two moments in time.
A5: Unfortunately, the restrictive "in at most two places at once" qualifier (also mentioned earlier) rules out any possibility that B can occupy a first, second and third place at the same time. That is why this specific alternative means B must occupy the second and third of these locations at a later time, implying once again that B will be located at p2 during two instants, t1 and t2, which entails it will be motionless there (even if only momentarily).
A6: However, the same argument applies to all the points along B's trajectory. The "in at most two places at once" restriction implies that B will occupy each successive point for two instants, not one, meaning its motion will be discontinuous, a stop-go, staccato sort of affair (mentioned earlier). But, that runs contrary to the hypothesis that motion is continuous and therefore contradictory -- or, it does so if we accept the "at most two" caveat.
A7: Any attempt to reject the "in at most two places at once" caveat will only succeed in re-introducing all the problems we met earlier that this restriction sought to avoid (and, as it turns out, was meant to sidestep several even more problematic implications we will meet later on in this Essay), namely that a moving object must occupy a potentially infinite number of points in the same moment, not two, contrary to Engels, once more!
But, it was surely the continuous nature of motion that (supposedly) presents logic (i.e., FL) with insoluble problems, which system -- so we have been informed by DM-fans -- is built on a static, discontinuous view of reality, a tall tale they never tire of telling, but which they fail to substantiate equally often.
Any who find the above argument difficult to follow might then find the following of some assistance (where all the 'moments in time' mentioned are meant to be contiguous and successive, such that tk+1 > tk, and where ">" means "later than"; so t2 > t1, and so on):
[1] Let us track moving object, B, along its path.
[2] Assume B begins its journey at point, p1, at time, t1.
[3] Further assume this journey takes B through the following points: p1, p2, p3,..., pi-1, pi, pi+1,..., pn-2, pn-1, pn, where it finally stops, or we lose interest in it.
[4] Normally, in every day life, we would say that B occupies each of these points at successive moments in time.
[5] If so, B would be in p1 at t1, p2 at t2, p3 at t3, p4 at t4, and so on.
[6] But, if Engels is to be believed, we can't say this; we have to say something like the following: B is in p1 and p2 at t1.
[7] The problem now becomes one of trying to decide what happens at all the other points along B's path.
[8] For reasons aired above, let us now restrict, or interpret what Engels meant by (a) "a body being both in one place and in another place at one and the same moment of time" as the following, (b) "a body being in at most two places at one and the same moment of time".
[9] We will also assume that if an object is located in one place for two or more contiguous moments, it will be at rest there. [This is the uncontroversial point made earlier.]
[10] With that in mind, let us try and make sense of the movement of B from p2 to p3.
[11] One and only one of these will be the case:
(α) B is in p2 and p3 at t1.
(β) B is in p2 and p3 at t2.
(γ) B is in p2 at t1 and in p3 at t2.
[12] If (β) were the case, then from [6] above, B will be in p2 at two times, t1 and t2. But [9] reminds us this means B would now be stationary at p2.
{From earlier:
{[6] However, if Engels is to be believed, we can't say that; we have to say something like this: B is in p1 and p2 at t1.}
{[9] We will also assume that if an object is located in one place for two or more contiguous moments, it will be at rest there.}
[13] If (γ) were the case, then Engels was wrong, since he held that all moving bodies occupy two places at the same time, not two places at successive moments in time, which is what (γ) implies.
[14] If (α) were the case, then, also from [6], B would occupy three places at the same time: p1, p2 and p3, all at t1.
[15] But [14] contradicts [8](b), which told us that a moving body is "in at most two places at one and the same moment of time".
[16] So, if we hold on to [6] and [8](b), both (α) and (γ) must be rejected.
[17] Hence, (β) must be the case: (β) B is in p2 and p3 at t2.
[18] In that case, B does come to rest at p2, since it is there for two moments, t1 and t2. [Based on: (β), [6] and [9].]
{From earlier, again:
{[6] However, if Engels is to be believed, we can't say that; we have to say something like this: B is in p1 and p2 at t1.}
{[9] We will also assume that if an object is located in one place for two or more contiguous moments, it will be at rest there.}
[19] However, the same applies when B moves from p3 to p4, p4 to p5, p5 to p6,..., pn-1 to pn.
[20] Hence, B must come to rest at all these points (even if only momentarily)!
[21] This means that while B might move from one point to the next, it does so in a stop-go, staccato sort of way, halting at every point along its path (even if only momentarily).
I trust that that makes the earlier argument a little clearer!
[Later on in this Essay, I will return to this argument and derive even more weird results from it, where I also consider every conceivable response and objection.]
It could be countered that no matter how much we 'magnify' the path of a moving body, it will still occupy two points at once, being in one of them and not in it at the same time.
And yet, that doesn't actually solve the problem, for if there is indeed a potentially infinite number of intermediary points between any two, a moving body must occupy more than two at once, contrary to what Engels said:
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Ibid. Bold emphasis added.]
Hence, between any two points, P and Q -- located at, say, (Xp, Yp, Zp) and (Xq, Yq, Zq), respectively -- which moving object, B, occupies (at the same "moment in time", t1), there are, for example, the following intermediary points: (X1, Y1, Z1), (X2, Y2, Z2), (X3, Y3, Z3),..., (Xi, Yi, Zi),..., (Xn, Yn, Zn) -- where n itself can be arbitrarily large. Moreover, the same applies to (X1, Y1, Z1) and (X2, Y2, Z2): there is a potentially infinite number of intermediate points between those two, and so on (again, as even Hegel acknowledged).
So, if Engels is to be believed, B must occupy not just P and Q at the same instant, it must occupy all these intermediary points, as well -- all at t1. That can only mean that B is located in a potentially infinite number of places at the same "moment". It must therefore not only be in and not in P at t1, it must be in and not in each of (X1, Y1, Z1), (X2, Y2, Z2), (X3, Y3, Z3),..., (Xi, Yi, Zi),..., and (Xn, Yn, Zn), at t1, just as it must be in all the intermediary points between (X1, Y1, Z1) and (X2, Y2, Z2), if it is also to be in Q at the same "moment" -- if we reject the "in at most two places at once" caveat.
And, what is worse: B must move through (or be in) all these intermediate points with time having advanced not one instant!
That is, B will have achieved all this in zero seconds!
B must therefore be moving with an infinite velocity between P and Q!
Unless, of course, we decide to re-define "velocity" so that it is no longer the expression of a functional relation between distance and time -- calculated by dividing the former by the latter.
But, if not, what then is it?
[An appeal to the Calculus here -- or, rather, to a DM-reinterpretation of the Calculus -- would be to no avail, as we will see in Essay Seven Part One. See also this sub-section of the present Essay.]
Of course, we could always claim that by "same moment" Engels meant "same temporal interval", but as we will soon see, that reply would scupper his theory even faster. [No pun intended.] That is because, if by the "same moment" Engels did mean the "same temporal interval", then there is no reason why "same point" can't also mean "same spatial interval", at which point the alleged 'contradiction' simply vanishes. [Again, no pun intended!]
[Why that is so will be explained below. Indeed, we will also see that this alternative (i.e., that a moving body occupies all the intermediate points between any two points, at the same time) poses even more serious problems for Engels's theory than this --, that is, over and above implying that 'dialectical' objects move with infinite velocities. (For some of the social and political consequences of the idea that between any two points there is a third point, or that any point can be divided into two points -- i.e., that there are no "indivisibles" -- alongside the controversy over "infinitesimals" in the post-Renaissance world, see Alexander (2016).]
However, if B moves from P to Q in temporal interval, t, comprised of sub-intervals, t1, t2, t3, ..., tn, each of which is also comprised of its own sub-intervals, then B will be located at P at t1 and then at Q at tn, which will, of course, mean that B won't be in these two places at the same time, although it will be located at these two points in the same temporal interval. Plainly, the 'contradiction' Engels claims to see here will now have vanished. Few theorists, if any, think it is the least bit contradictory to suppose that B is in P at one moment and then in Q a moment later.
Consider a car travelling north across Texas during a three-hour temporal interval. Let us suppose it is in the centre of Lubbock at 08:00am and in the centre of Amarillo (approximately 124 miles away) at 11:00am. Hence, it will have been in two locations during the same temporal interval (spanning these three hours), but not in two places in the same moment in time. Once again, the alleged contradiction has disappeared. Indeed, this car won't even be in Lubbock and not in it at, say, 08:01, even while it is moving -- since it will be in Lubbock for several minutes (i.e., until it reaches the city boundary). So, in this example, the car isn't in one place and not in it in this specific sub-interval of the longer interval. If that is so, only a very short-sighted DM-fan will want to take advantage of this escape route (no pun intended) -- i.e., referring to temporal intervals as opposed to 'moments in time'. That is probably why Engels didn't refer to them, and, as far as can be ascertained, no DM-theorist has done so since.
[Graham Priest has argued that changes from one state to another -- for instance, in the above example, doing so in the actual moment the car left the city boundary -- are contradictory (in Hegel and Engels's sense of that word -- if it is granted for the purpose of argument that this use of "contradictory" makes some sort of sense!). I have neutralised his argument in Note 18a. (The car example is, of course, mine not Priest's!)]
[In what follows, I am of course using "accelerate" as it is employed in everyday speech, not as it is used in Physics and Applied Mathematics! Same with "speeds up".]
On a different tack: Do these contradictions increase in number or stay the same if an object speeds up? [This is a problem that, for example, exercised Leibniz; more on that below.] Or, are the two locations depicted by Engels (i.e., the "here" and the "not here") just further apart? That is, are the two points that B occupies at the same moment, if it accelerates, just further apart (so that this object will have moved further in a given instant than it would have done had it not picked up speed)? But, if it occupies them at the same time, it can't have accelerated! That is because it hasn't moved from the first to the second, since it is in both at once. Speeding up, of course, involves covering the same distance in less time, but that isn't allowed here, nor is it even possible. Whether or not P and Q from earlier are closer together or further apart, B occupies them both at the very same 'moment', according to Engels.
It could be argued that acceleration in fact involves:
(a) A moving body travelling further in the same amount of time; or,
(b) That body covering the same distance in a shorter amount of time.
But, B takes no time at all -- zero seconds -- in order to occupy both P and Q, so there is here no "amount of time" to be compared. Whichever one of (a) or (b) applies here, both involve no time at all. So, if (a) were the case, B can't have travelled further in the same amount of time, since there is no amount of time for us to compare. And (b) can't apply, either, since there can be no amount of time that is less than zero.
It could be objected that (a) does apply here, since B will have gone further in the same amount of time.
However, that argument will only work if we are prepared to count zero seconds (or zero minutes (etc.)) as actual amounts of time. If you are paid £0 (or $0), have you been paid an amount of money? Of course, 0ºC (or 0ºF) is an actual temperature, but 0 cm (or 0 in) isn't an actual distance. If zero people attend a meeting there is no one in the audience. So, it is clear that, except in relation perhaps to temperature, zero isn't an actual amount. It certainly isn't in terms of time or distance. So, if a drink has zero amount of poison in it, there isn't any poison in that drink by default! Similarly, zero seconds isn't an amount of time by default. Indeed as Trotsky himself noted:
"How should we really conceive the word 'moment'? If it is an infinitesimal interval of time, then a pound of sugar is subjected during the course of that 'moment' to inevitable changes. Or is the 'moment' a purely mathematical abstraction, that is, a zero of time? But everything exists in time; and existence itself is an uninterrupted process of transformation; time is consequently a fundamental element of existence. Thus the axiom 'A' is equal to 'A' signifies that a thing is equal to itself if it does not change, that is if it does not exist." [Trotsky (1971), p.64. Bold emphasis added.]
But, let us suppose a few desperate (and perhaps also anti-Trotsky) DM-fans can be found who think that zero seconds (etc.) is an actual amount of time, so that option (a) applies, here. The problem is that the alternatives on offer were artificially restricted; they should have been (c) and (d), not (a) or (b).
Hence, acceleration in fact involves:
(c) A moving body travelling further in the same amount of time; and,
(d) That body covering the same distance in a shorter amount of time.
It isn't an "either...or" choice in this case (but don't DM-fans reject the 'either...or' of FL and 'commonsense', anyway?).
Both (c) and (d) must both apply if a body is to accelerate. We can see this if we re-examine, and then adapt, an earlier argument -- with respect to the following intermediary points between P and Q, (X1, Y1, Z1), (X2, Y2, Z2), (X3, Y3, Z3),..., (Xi, Yi, Zi),..., (Xn, Yn, Zn):
If Engels is to be believed, B must occupy not just P and Q at the same instant, it must occupy all these intermediary points, as well -- all at t1. That can only mean that B is located in a potentially infinite number of places at the same "moment". It must therefore not only be in and not in P at t1, it must be in and not in each of (X1, Y1, Z1), (X2, Y2, Z2), (X3, Y3, Z3),..., (Xi, Yi, Zi),..., and (Xn, Yn, Zn), at t1, just as it must be in all the intermediary points between (X1, Y1, Z1) and (X2, Y2, Z2), if it is also to be in Q at the same "moment" -- if we reject the "in at most two places at once" caveat.
But, if (a)/(c) are applicable, and B travels further in zero seconds than it did before, this will now have to be the case:
An accelerating body, B, must occupy not just P and Q at the same instant, it must occupy R as well (assuming R is further from P than Q is, meaning B will have travelled further in zero seconds that it did before). That would (somewhat fittingly) contradict Engels who says that such bodies occupy two places at once; but B now occupies three at the same time, P, Q and R. If we now ignore Engels's caveat, B must still occupy all the intermediary points between P and Q at t1 -- even if B is also at R at the same time. B must therefore not only be in and not in P at t1, it must be in and not in each of (X1, Y1, Z1), (X2, Y2, Z2), (X3, Y3, Z3),..., (Xi, Yi, Zi),..., and (Xn, Yn, Zn), at t1, if it is also to be in Q and R at the same "moment". But if (a)/(c) apply and B is accelerating, it will be in all these intermediary points in less time, which isn't possible. There is no amount of time less than zero.
And if we now take note of another earlier question, it might well be wondered how (a) (or (a1), below) could possibly apply on its own:
In relation to moving bodies it is pertinent to ask the following question that no one else ever seems to have asked: How far apart are the two proposed "places" that a moving object is supposed to occupy while at the same time not occupying one of them?
If we don't know how far apart any of these Engelsian points are, how can they be deemed to be further apart? How can B travel further in zero seconds when we haven't a clue how far it travels anyway?
(a1) An accelerating body travels further in the same amount of time.
In which case, it is difficult to see how, in a DM-universe, moving bodies can possibly accelerate (if they are in two places at once)
Accelerated motion (in the above sense of the phrase) involves a body being in (or passing through) more places in a given temporal interval than had been the case before it accelerated. But, if B is in these two places at the same time, how can it pick up speed?
[For some of the complexities involved here, see Note 18c.]
Hence, as we have seen, if we insist on analysing motion this way, a moving object must be in an 'infinite number' of points at the same time -- in which case it can't speed up. This conundrum was brought out rather well by Professor Joseph Mazur:
"Consider three adjacent points labelled A, B, and C. By this I mean that B is immediately to the right of A and that C is immediately to the right of B. In one indivisible instant, an object cannot travel from point A to point C. If it could, there would be no instant when it could be at point B. Of course, this is absurd, because that would mean all motion must take place at the same speed. [Mazur is arguing that if the above were the case, no object could speed up or have a different speed from any other. If they could, there would be more of these intermediate points that such bodies occupied in no instant -- RL.] The only way out of this is to reject the thought that points or instants are consecutive, i.e., arranged in a hierarchy from left to right and vice versa. This leads to equally puzzling thoughts about how a moving body gets from one point to another. If an object moved from A to C, there must have been a moment when it was at a point B between A and C. And there must have been a moment when it was at a point between A and B. This can go on indefinitely." [Mazur (2007), pp.29-30. Spelling modified to agree with UK English. Bold emphasis alone added.]
A B C
So, if the above remarks are correct, a moving object must be in these intermediate points 'outside of time'. Hence, if Trotsky is to be believed (quoted earlier), such an object must cease to exist between any two points!
"Or is the 'moment' a purely mathematical abstraction, that is, a zero of time? But everything exists in time; and existence itself is an uninterrupted process of transformation; time is consequently a fundamental element of existence. Thus the axiom 'A' is equal to 'A' signifies that a thing is equal to itself if it does not change, that is if it does not exist." [Trotsky (1971), p.64. Bold emphases added.]
There thus seems to be no way of making sense of the everyday phenomenon of movement/locomotion if we insist on using language this way.
(4) Yet More A Priori Dogmatics?
Quite apart from the foregoing, Engels's attempt to provide an overtly linguistic -- or perhaps even a 'conceptual' -- solution to the 'problem of motion' suggests that there is more than a hint of LIE to his theory. That should surprise no none given that he imported these ideas from Hegel, an Idealist of the worst possible kind, who in turn relied on the ideas of other mystics and Idealists.
[LIE = Linguistic Idealism; that term is explained here.]
This purely 'conceptual' approach to motion is plain for all to see from the way that Engels's attempt to depict it depends on a 'one-sided' consideration of a handful of the concepts that seemed to be relevant to both him and Hegel -- albeit expressed in what appears to ordinary-looking language, the meaning of which both simply took for granted (more on that later).
So, based on thought alone, Engels imagined he could conclude what must be true of every moving object in the entire universe, for all of time, without exception. But, how could he possibly have known so much with so little evidence -- in fact, no evidence at all -- to back it up?
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
"Motion is the mode of existence of matter. Never anywhere has there been matter without motion, nor can there be…. Matter without motion is just as inconceivable as motion without matter. Motion is therefore as uncreatable and indestructible as matter itself; as the older philosophy (Descartes) expressed it, the quantity of motion existing in the world is always the same. Motion therefore cannot be created; it can only be transmitted…. A motionless state of matter therefore proves to be one of the most empty and nonsensical of ideas…." [Ibid., p.74. Bold emphases alone added. Paragraphs merged.]
Attentive readers will no doubt notice that Engels explicitly contrasts what we can see (when he refers to the "limits of this sphere of observation") with how things seem when we stop to "consider things". In other words, his only 'evidence' is based on what (he claims) we think about motion, not what we actually observe.
Clearly, Engels possessed a truly remarkable skill: the ability to uncover fundamental truths about reality, valid for all of space and time, from the presumed meaning of a handful of words (or 'concepts')! Indeed, Engels's claims about motion are all the more impressive when it is recalled that he hit upon these ideas in abeyance of any supporting evidence, let alone a significant body of it. As it turns out (and as this will also be demonstrated in what follows), even had any evidence been available to him, it would have been unnecessary, anyway.
As we have already seen (in Essay Two), all that an aspiring dialectician like Engels need do in such circumstances is briefly 'reflect' on the supposed meaning of a few words or 'concepts', and substantive truths about fundamental aspects of 'reality', valid for all of space and time, 'effortlessly spring to mind'.
Or, to be more honest, all they have to do is read Hegel's 'Logic' (or the work of some other mystic, such as Heraclitus or Zeno). This a priori approach to 'knowledge' is the unifying thread that runs through the entire history of ruling-class thought, unfortunately imported into the workers' movement by incautious non-workers like Engels. [On that, see Essays Nine Parts One and Two, Twelve Part One and Fourteen Part Two.]
So, the only 'evidence' offered in 'support' of Engels's claims about motion was this highly compressed (and, as we will also see, rather badly formulated) 'argument' -- or, rather, this brief and highly superficial 'thought experiment'. Pressed for a justification of the conclusions he reached, all that Engels could provide in response would be a rather weak claim that this is what the word "motion" really means. It is difficult to see how he could offer any other substantiation. He certainly offered no actual evidence in anything else about motion in any of his other writings (published or unpublished).
[If anyone knows differently, please let me know. I have been searching his writings since the late 1970s and have yet to find any -- or, indeed, any in his successor DM-theorists.]
Such a (proffered) rejoinder would clearly give the game away, since it would confirm the accusation that substantive truths about motion had indeed been derived from the supposed meaning of a few words (or in this case, perhaps just one), and nothing more.
[The significance of that observation will emerge in as this Essay unfolds, but more extensively in Essay Twelve Part One.]
As noted above, an appeal to evidence would be irrelevant, anyway. That is because the examination of a finite number (no matter how large) of moving objects would fail to confirm Engels's assertion that they occupy two places at once. That would still be the case irrespective of the instruments or devices chosen to help effect these observations and regardless of the extent of the magnification used to that end. Furthermore, it would remain the case independently of the level of microscopic detail enlisted in support. No observation (human or mechanical) could confirm that a moving object is in two places at once (except in the senses noted below), in one of them and not in it at the same time. That helps explain why Engels offered absolutely no scientific evidence in support of his belief that motion is contradictory. As noted earlier, too, that picture hasn't changed in the intervening years. Indeed, the author of no book, article or speech devoted to DM (by one of its supporters) even so much as thinks to quote or cite any such evidence, and, what is more, that situation isn't ever likely to change.5
What is perhaps even more peculiar -- when we recall that DM-fans never tire of telling us that their theory enjoys convincing support from the available evidence and that they would never dream of imposing their ideas on the facts (on that, see Essay Two and below) -- is that not one of them has noticed this core part of their theory enjoys absolutely no evidential support.
Of course, it could be objected to accusations like this that if, say, a photograph were taken of a moving object, it would show by means of the recorded blur that such a body had occupied several places at once. In that case, therefore, there is, or could be, evidence in support of Engels's claims.
The problem with such a response is that no matter how fast the shutter speed, no camera (not even this one, or this) can record an instant in time, merely a temporal interval -- that is, such devices record what happens in the space of time between the opening and the closing of its shutter (or other light/energy permitting aperture). Clearly, in order to verify the claim that a moving object occupies at least two places in the same instant, a physical recording of an instant would be required. However, since instants (i.e., in the sense required) are mathematical fictions, it isn't possible to record them!
Update December 2016: The New Scientist recently reported the following:
"For the first time, physicists have measured changes in an atom to the level of zeptoseconds, or trillionths of a billionth of a second -- the smallest division of time yet observed. In this case, the speed demon was an electron escaping the bonds of its parent atom when struck by a photon. This electron ejection is known as the photoelectric effect, and was described by Albert Einstein in 1905. Previous experiments could only measure what happened after the electron was kicked out, says Martin Schultze at the Max Planck Institute of Quantum Optics in Garching, Germany. Now, he and his colleagues have measured the ejection of electrons from a helium atom from start to finish with zeptosecond precision (10-21 seconds), marking the smallest time slot ever measured. To do this, they fired ultraviolet laser pulses lasting 100 to 200 attoseconds (10-18 seconds) at a helium atom to start exciting its pair of electrons. By making many readings and calculating their statistical spread the team could measure events with a resolution of 850 zeptoseconds. They found the ejections took 7 to 20 attoseconds (Nature Physics, doi.org/bszd). The results are an important window into the quantum behaviour of atoms, especially how their electrons interact with each other, says Schultze." [New Scientist, 19/11/2016, p.14. Paragraphs merged. One link added.]
Not even this ultra-precise measurement captures an 'instant', merely an interval, albeit an extremely brief one.
It could be countered that as we increase a camera's shutter speed any photographs taken will always show some blurring. This supports the conclusion that moving objects are never located in one place at one time. Despite this, it still remains the case that no photograph can catch an instant, and thus no device can verify Engels's contention. Naturally, the situation hasn't been helped by the fact that neither Zeno, Hegel nor Engels were very forthcoming about how long they thought a "moment" or an "instant" in time was supposed to last. Indeed, it would seem that one of these "instants" is in fact a zero of time (i.e., they have no duration), which means, at least according to Trotsky, they don't actually exist!
Again, it could be argued that it is reasonable to conclude from the above that moving objects occupy two locations at the same moment. Once more: since an 'instant in time' is a mathematical fiction, it isn't reasonable to so conclude.
Furthermore, not even a mathematical limiting process (via the Calculus, etc.) could capture such ghostly 'entities' in the physical world, whatever else it might appear to achieve in theory. And, even if it could, no camera (radar device or other equipment) could capture it. Hence, if an appeal to a mathematical limiting process were viable (or available), it would still be of no help. No experiment or observation is capable of substantiating any of the conclusions Engels and Hegel reached about moving bodies.
And that explains why they (and those who accept these ideas) have had to foist this theory of motion on nature.
But, as we will see later, the idea that a moving object is in two places at once possesses rather nasty consequences of its own (for this theory), so DM-fans had better hope that no camera will ever be able to record this alleged fact. Of course, there were no such cameras in the 19th century, but Hegel and Engels still seemed happy to assert the truth of something that was impossible to confirm in their day -- and now even in ours!
Hence, Engels's conclusion about moving bodies wasn't derived from a consideration of the facts, it has been imposed on them, in defiance of what Engels himself said:
"Finally, for me there could be no question of superimposing the laws of dialectics on nature but of discovering them in it and developing them from it." [Engels (1976), p.13. Bold emphasis added.]
"All three are developed by Hegel in his idealist fashion as mere laws of thought: the first, in the first part of his Logic, in the Doctrine of Being; the second fills the whole of the second and by far the most important part of his Logic, the Doctrine of Essence; finally the third figures as the fundamental law for the construction of the whole system. The mistake lies in the fact that these laws are foisted on nature and history as laws of thought, and not deduced from them. This is the source of the whole forced and often outrageous treatment; the universe, willy-nilly, is made out to be arranged in accordance with a system of thought which itself is only the product of a definite stage of evolution of human thought." [Engels (1954), p.62. Bold emphasis alone added.]
"We all agree that in every field of science, in natural and historical science, one must proceed from the given facts, in natural science therefore from the various material forms of motion of matter; that therefore in theoretical natural science too the interconnections are not to be built into the facts but to be discovered in them, and when discovered to be verified as far as possible by experiment. Just as little can it be a question of maintaining the dogmatic content of the Hegelian system as it was preached by the Berlin Hegelians of the older and younger line. Hence, with the fall of the idealist point of departure, the system built upon it, in particular Hegelian philosophy of nature, also falls. It must however be recalled that the natural scientists' polemic against Hegel, in so far as they at all correctly understood him, was directed solely against these two points: viz., the idealist point of departure and the arbitrary, fact-defying construction of the system." [Ibid., p.47. Bold emphasis alone added. Paragraphs merged. Unfortunately, this passage is longer available at the Marxist Internet Archive, but it can nevertheless be accessed here.]
From recently published Preparatory Writings for Anti-Dühring, we find the following entirely reasonable comment, also by Engels:
"The general results of the investigation of the world are obtained at the end of this investigation, hence are not principles, points of departure, but results, conclusions. To construct the latter in one's head, take them as the basis from which to start, and then reconstruct the world from them in one's head is ideology, an ideology which tainted every species of materialism hitherto existing.... As Dühring proceeds from 'principles' instead of facts he is an ideologist, and can screen his being one only by formulating his propositions in such general and vacuous terms that they appear axiomatic, flat. Moreover, nothing can be concluded from them; one can only read something into them...." [Marx and Engels (1987), p.597. Bold emphases alone added. Quotation marks altered to conform with the conventions adopted at this site.]
Again, as we will see, Engels is guilty of doing precisely what he accused Dühring of doing. In which case, the following characterisation of Idealism clearly applies to his 'analysis' of motion:
"A consistent materialism can't proceed from principles which are validated by appeal to abstract reason, intuition, self-evidence or some other subjective or purely theoretical source. Idealisms may do this. But the materialist philosophy has to be based upon evidence taken from objective material sources and verified by demonstration in practice...." [Novack (1965), p.17. Bold emphasis added.]
Zeno and Hegel are equally guilty in that regard; all three "proceed[ed] from principles which are validated by appeal to abstract reason, intuition, self-evidence or some other subjective or purely theoretical source." None of them "verified [this theory] by demonstration in practice...." All proceed "from 'principles' instead of facts"; all "construct [their theories in the] head, take them as the basis from which to start, and then reconstruct the world from them [which] is ideology...".
Of course, part of the problem here depends on what the word "instant" means. [I am taking it to mean the same as the phrase "moment in time", used by Engels.] In that case, some might think this 'problem' could be resolved by re-defining our terms. However, even if that were possible, such an 'adjustment' would merely amount to the adoption of a new linguistic convention, which would have no bearing on 'the nature of reality'. It would also further confirm the suspicion that this 'theory' had been imposed on nature, not 'read from it'. What else is the introduction of a new linguistic convention, adopted for the sole purpose of making a given theory 'fit the facts', but an imposition?5a
Again, as Trotsky argued: if motion were to take place in one of these "moments" (interpreted as an "instant"), that would clearly mean it couldn't exist -- unless, of course, we're also prepared to reject the dogmatic, a priori, opinion Trotsky himself expressed (in the passage quoted earlier)!
But, if motion actually takes place -- as it clearly does --, what are we to make of the claim that if something is moving it must be in at least two places in the same instant when (according to Trotsky) the latter don't exist? Does this refute Trotsky, Engels, or both? Is there even a straw-sized 'contradiction' here for dialecticians to "grasp" to save their floundering theory?
Some might claim that the above points are all rather abstract and 'academic', but that (proffered) deflection can't rescue Engels's theory. His analysis of motion is no less abstract! Even worse, his concept of motion can't have been derived by 'abstraction' from all (or any) of the forms of motion hitherto experienced by himself or by humanity in general -- or from a finite sub-set of either --, or even from what had been observed by scientists and philosophers in his day, or now even in ours. That is because Engels's theory clearly appeals to objects and processes that, according to Trotsky, move in a way that implies they don't exist -- such as "moments" in time and changes of place that take place 'outside of time'. What is more, even if they did exist, we couldn't experience or observe them, and hence we couldn't use them to confirm what Engels asserted. As seems plain, observations take place in time, and have a duration (and, in theory, can be perceived); "instants" don't (and can't, even in theory).
Clearly, it isn't possible to 'abstract' from such non-existent 'instants' in order to agree with Engels!6
So, whichever way we turn we hit yet other non-dialectical brick wall.
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152.]
In the above passage, Engels promptly changed direction (no pun intended), arguing that it is motion itself that is contradictory, not just our thoughts about it that are:
"Motion itself is a contradiction…." [Ibid. Bold emphasis added.]
In which case, it could be argued that Engels was merely pointing out that our thoughts about motion are contradictory because motion itself is. That is, our theories depict the world more fully, accurately and truly when they faithfully reflect its contradictory nature. In that case, substantive claims about the universe are fully justified --, indeed, are objective -- when our ideas capture changing reality more precisely (but, only if they have been tested in practice).
Unfortunately, if that (proffered) response were correct, it would be inimical to DM itself; that is because it would mean DM contains contradictions, which would clearly imply it is a contradictory theory!7
[The disastrous implications that that particular observation has for DM were set out in Essays Seven Part One and Eleven Part One.]
Despite this, a reply along those lines would give the game away since it confirms the validity of an earlier accusation that this theory has been imposed on nature. That is because there is no way Engels could know, or have known, that nature is contradictory and that all motion in the entire universe, for all of time, is as he says it is. The very best he could claim is that our thoughts about motion are contradictory and that this appears to suggest that motion might be, too.
However, as will become apparent by the end of this Essay, the insurmountable problem that confronts the above suggested fall-back position is that our thoughts about motion aren't contradictory, either!7a
Be this as it may, the above (proffered) pro-DM response fails to neutralise the serious difficulties these ideas face, outlined earlier. That is because Engels's philosophical theory, which was the result of an extrapolation from what he thought were the meaning of a handful of words (and/or 'concepts') to a conclusion about fundamental aspects of reality, is itself overtly Idealist (on that, see Essays Two and Twelve Part One). Indeed, as Engels himself pointed out (quoted earlier):
"The general results of the investigation of the world are obtained at the end of this investigation, hence are not principles, points of departure, but results, conclusions. To construct the latter in one's head, take them as the basis from which to start, and then reconstruct the world from them in one's head is ideology, an ideology which tainted every species of materialism hitherto existing.... As Dühring proceeds from 'principles' instead of facts he is an ideologist, and can screen his being one only by formulating his propositions in such general and vacuous terms that they appear axiomatic, flat. Moreover, nothing can be concluded from them; one can only read something into them...." [Marx and Engels (1987), Volume 25, p.597. Italic emphases in the original; bold emphases added. Quotation marks altered to conform with the conventions adopted at this site.]
Compare those remarks (again) with George Novack's:
"A consistent materialism cannot proceed from principles which are validated by appeal to abstract reason, intuition, self-evidence or some other subjective or purely theoretical source. Idealisms may do this. But the materialist philosophy has to be based upon evidence taken from objective material sources and verified by demonstration in practice...." [Novack (1965), p.17. Bold emphasis added.]
Worse still, and for reasons aired earlier, not only is there no way this 'theory' can be confirmed (by an appeal to evidence), its subject matter (i.e., the theory that a moving body occupies and does not occupy a given location in the same moment, and is therefore in two places at once) resists all attempts to make sense of it, as we will also soon see.
Substantive philosophical 'truths' like this (about motion, for example) are ambitiously universal in intent but are at the same time embarrassingly parochial in origin. Indeed, their promulgators' epistemologically expansive intentions (which purport to stretch across all regions of space and time) remain stubbornly unmatched by any capacity to satisfy such hyper-bold philosophical goals with adequate supporting evidence --, or, in this case, any at all.
A contrary argument might proceed as follows: If knowledge results from the reflection in the mind of the complexities found in reality (mediated by practical activity, etc.) -- which knowledge is "relative" and hence correct only "within certain limits" -- then, even a provisionally correct theory must faithfully represent the contradictory nature of the world. In this limited sense, human and/or social categories will be capable of reflecting the world (in the way DM-theorists suppose), they won't have been imposed on it.
A reinterpretation or reconfiguration along these lines might then allow dialecticians to draw substantive conclusions about the world by applying the concepts and categories developed in and by dialectical thought. Howsoever the latter actually got there, the theories that result from this process would still only approach absolute truth asymptotically:
"The identity of thinking and being, to use Hegelian language, everywhere coincides with your example of the circle and the polygon. Or the two of them, the concept of a thing and its reality, run side by side like two asymptotes, always approaching each other but never meeting. This difference between the two is the very difference which prevents the concept from being directly and immediately reality and reality from being immediately its own concept. Because a concept has the essential nature of the concept and does not therefore prima facie directly coincide with reality, from which it had to be abstracted in the first place, it is nevertheless more than a fiction, unless you declare that all the results of thought are fictions because reality corresponds to them only very circuitously, and even then approaching it only asymptotically…. In other words, the unity of concept and phenomenon manifests itself as an essentially infinite process, and that is what it is, in this case as in all others." [Engels to Schmidt (12/03/1895), in Marx and Engels (1975a), pp.457-58. Bold emphases alone added.]
[Some might want to call such 'concepts and categories', "presuppositions". A sophisticated version of this approach has even been given the rather grandiose title, "Descriptive Metaphysics", by several Analytic Philosophers -- or, to be more accurate, by Sir Peter Strawson (Strawson (1959)). [On that, see here.]
On the other hand, if a Kantian or Hegelian route is taken by dialecticians, whereby the 'concepts and categories' of thought are what they are because of 'structural' principles that govern the very possibility of 'human cognition' -- or even because of the 'nature of dialectical reason', itself -- then they should be honest and admit that they have imposed their ideas on the world, contrary to what they say they never do. Currently, only a handful of HCDs seem prepared to take that detour into open and honest Idealism.]
[HCD = High Church Dialectician; follow the link for an explanation of that term.]
Of course, as we have seen, this flies in the face of George Novack's insightful comment (quoted earlier):
"A consistent materialism cannot proceed from principles which are validated by appeal to abstract reason, intuition, self-evidence or some other subjective or purely theoretical source. Idealisms may do this. But the materialist philosophy has to be based upon evidence taken from objective material sources and verified by demonstration in practice...." [Novack (1965), p.17. Bold emphases added.]
Be this as it may, such counter-claims have already been critically examined and rejected, here and here.
In addition to the above, it is worth highlighting several serious problems that this 'quasi-Kantian' approach to knowledge brings in its train:
(1) Elsewhere, it will be argued that this way of looking at language forms part of what I have called the RRT, which turns out to be a theory that projects 'knowledge' onto nature under the pretence that language and 'cognition' supposedly 'reflect' what is already there. The RRT in effect uses language to pre-judge and pre-determine what the world must be like irrespective of what it is actually like. So, instead of the world deciding (or being used to decide) whether what we say is true or false, the theory decides what must be true or must be false. In that case, the language employed to that end doesn't 'reflect the world', the reverse happens: the world is a reflection of this forced use of language. The presence of modal terms, such as "must" and "necessarily", is often a dead give-away.
[RRT = Reverse Reflection Theory; that theory will be both explained and examined in Essay Twelve Part Four (to be published in 2024).]
It is because of their (implicit, and sometimes, explicit) acceptance of the RRT that dialecticians imagine they can state in advance of experience what the world must be like long before anyone knows what it is like. This involves them in specifying what certain 'concepts' or jargonised-terms actually correspond with (in 'reality'), and this they do solely on the basis of the presumed structure of the mode of expression in which they have been framed.
Here are Engels and Lenin doing precisely that:
"The fact that identity contains difference within itself is expressed in every sentence, where the predicate is necessarily different from the subject; the lily is a plant, the rose is red, where, either in the subject or in the predicate there is something that is not covered by the predicate or the subject...." [Engels (1954), pp.214-15. Bold emphasis alone added.]
"To begin with what is the simplest, most ordinary, common, etc., [sic] with any proposition...: [like] John is a man…. Here we already have dialectics (as Hegel's genius recognized): the individual is the universal…. Consequently, the opposites (the individual is opposed to the universal) are identical: the individual exists only in the connection that leads to the universal. The universal exists only in the individual and through the individual. Every individual is (in one way or another) a universal. Every universal is (a fragment, or an aspect, or the essence of) an individual. Every universal only approximately embraces all the individual objects. Every individual enters incompletely into the universal, etc., etc. Every individual is connected by thousands of transitions with other kinds of individuals (things, phenomena, processes), etc. Here already we have the elements, the germs of the concept of necessity, of objective connection in nature, etc. Here already we have the contingent and the necessary, the phenomenon and the essence; for when we say John is a man…we disregard a number of attributes as contingent; we separate the essence from the appearance, and counterpose the one to the other….
"Thus in any proposition we can (and must) disclose as a 'nucleus' ('cell') the germs of all the elements of dialectics, and thereby show that dialectics is a property of all human knowledge in general." [Lenin (1961), pp.359-60. Bold emphases alone added. Quotation marks altered to conform with the conventions adopted at this site.]
Of course, they were both simply copying Hegel, who openly and brazenly tried to derive universal, omnitemporal 'truths' from the structure of subject-predicate sentences (which makes some sore of crazy sense if you're a Mystical Idealist who thinks everything is Mind or the product of Mind, but not if you're a materialist):
"The interpretation of the judgment, according to which it is assumed to be merely subjective, as if we ascribed a predicate to a subject is contradicted by the decidedly objective expression of the judgment. The rose is red; Gold is a metal. It is not by us that something is first ascribed to them. A judgment is however distinguished from a proposition. The latter contains a statement about the subject, which does not stand to it in any universal relationship, but expresses some single action, or some state, or the like. Thus, 'Caesar was born at Rome in such and such a year waged war in Gaul for ten years, crossed the Rubicon, etc.', are propositions, but not judgments. Again it is absurd to say that such statements as 'I slept well last night' or 'Present arms!' may be turned into the form of a judgment. 'A carriage is passing by' should be a judgment, and a subjective one at best, only if it were doubtful, whether the passing object was a carriage, or whether it and not rather the point of observation was in motion: in short, only if it were desired to specify a conception which was still short of appropriate specification....
"The abstract terms of the judgement, 'The individual is the universal', present the subject (as negatively self-relating) as what is immediately concrete, while the predicate is what is abstract, indeterminate, in short the universal. But the two elements are connected together by an 'is': and thus the predicate (in its universality) must contain the speciality of the subject, must, in short, have particularity: and so is realised the identity between subject and predicate; which being thus unaffected by this difference in form, is the content." [Hegel (1975), p.233, §167. Bold emphases alone added. Quotation marks altered to conform with the conventions adopted at this site.]
"To say 'This rose is red' involves (in virtue of the copula 'is') the coincidence of subject and predicate. The rose however is a concrete thing, and so is not red only: it has also an odour, a specific form, and many other features not implied in the predicate red. The predicate on its part is an abstract universal, and does not apply to the rose alone. There are other flowers and other objects which are red too. The subject and predicate in the immediate judgment touch, as it were, only in a single point, but do not cover each other.... In pronouncing an action to be good, we frame a notional judgment. Here, as we at once perceive, there is a closer and a more intimate relation than in the immediate judgment. The predicate in the latter is some abstract quality which may or may not be applied to the subject. In the judgment of the notion the predicate is, as it were, the soul of the subject, by which the subject, as the body of this soul, is characterised through and through." [Ibid., p.237, §172. Bold emphases added. Hegel's longer, more involved and hence much more opaque version of the above 'argument' -- published in Hegel (1999), pp.631-43 -- has been quoted in full in Appendix A to Essay Three Part One.]
[I have subjected passages like these to searching criticism in Essay Three Part One.]
Naturally, Marxist dialecticians wouldn't want to characterise their approach to 'knowledge' this way, nor would they even recognise it as what they actually do or even intend to do. Nevertheless, hundreds of examples (and that figure is no exaggeration!) were given in Essay Two that show that all -- i.e., 100% of -- dialecticians dogmatically assert 'fundamental truths about reality', valid for all of space and time, (i) based solely on the supposed meaning of a handful of words/'concepts', (ii) from some sort of 'thought experiment', or (iii) from the structure of subject-predicate sentences. Virtually all of the latter were borrowed from Hegel and other mystics (e.g., Heraclitus, Plotinus and Spinoza), or from Traditional Philosophy in general.
[A detailed analysis of the class-compromised origin and nature of core DM-ideas can be accessed here, here and here.]
Of course, without setting off an infinite regress, the DM-theory that language reflects the world can't have been derived from the world, as the following brief argument shows:
D1: Language reflects the world.
D2: The theory expressed in D1 reflects the world.
D3: D2 is also a theory, so it must reflect the world, too.
D4: D3 is also a theory, so it must reflect the world, too.
D5: D4 is also a theory, so it must reflect the world, too...
D(k-1): D(k-2) is also a theory, so it must reflect the world, too.
Dk: D(k-1) is also a theory, so it must reflect the world, too.
And so on...
The only way to halt this infinite regress would be either to argue that (i) D2-Dk aren't theories (in that case what are they?), or claim that (ii) Some theories do not in the end reflect the world -- but how might that theory itself be justified (while leaving the overarching idea intact)? If so, this theory -- which claims that knowledge is a complex (if still only 'relatively true') 'reflection of reality' -- must itself have been imposed on nature (contrary to the DM-claim that that is never done).
Nevertheless, it could be argued that an additional principle operates behind the scenes here: constant reference to experience, observation and/or practice is necessary if scientists, philosophers and DM-theorists aim to weed out objects and processes that aren't actually found in nature and society, or, indeed, for them to be able to reject ideas that fail to reflect 'objective reality' (for whatever reason). Otherwise, of course, an appeal to empirical checks, or even to practice itself -- in order to test which linguistic expressions (and theories) are genuinely 'represented' by real material processes/relations in nature and society -- would be an empty gesture. So, because it is impossible to specify ahead of time which parts of the DM might never be eliminated after repeated and long-term testing, all knowledge has to be deemed provisional.
Or, so it could be, and has been, argued...
Despite this, DM-theorists still aim to have uncovered fundamental truths about reality. So, they tell us that everything in nature and society is contradictory and constantly changing, that all objects and processes are powered by, and change because of, the interplay between 'interpenetrated internal opposites', and that the entire world is a single 'interconnected Totality' composed of 'mediated' parts/wholes governed by the 'laws of dialectics'. This means the universe is capable of being explained 'rationally'. We are also told that each and every part is dependent on every other part, and that the nature of the whole is determined by the complex interconnections between the parts, and vice versa, etc., etc.
[Dozens of quotations to that effect have been posted in Essay Two.]
But, it turns out that the only 'evidence' substantiating such universalist claims is a series of hasty extrapolations from a few 'doctored' linguistic expressions -- as we will discover later in this Essay --, which terminological tricks are then 'justified' by an appeal to a further series of highly dubious, but nonetheless seriously garbled principles drawn from what can only be described as Sub-Aristotelian Logic, supported by a handful of highly clichéd, specially-selected, constantly recycled, contentious examples. What little actual evidence is offered in support turns out to be watery-thin, at best, and in the end fails to support DM, anyway.
[On that, see Essay Seven Part One. In fact, that Essay has labelled the amateurish evidential display offered by DM-fans, Mickey Mouse Science and Minnie Mouse Philosophy.]
Unfortunately, this means that if anyone were looking for a theory that was capable of explaining, or helping us understand, nature, DM would fail to make the bottom of the reserve list of viable candidates, and not just because of the above considerations. As Essay Seven Part Three shows, if DM were true, change would be impossible.
Furthermore, if nature were reflected in thought, so that certain features of reality had somehow been 'embodied' in language, and if it were assumed for the purpose of argument that this ('fact') justified inferences from language to the world, it would be impossible to account for falsehood. If thought is indeed a reflection of the world, then it not only wouldn't it be incorrect, it couldn't be -- in roughly the same way that a mirror image or reflection is never wrong.
Of course, it might be thought that a sophisticated version of the RTK (not to be confused with the RRT), with its emphasis on the 'partial' or 'relative' status of knowledge, on the input of practice and the "one-sided" nature of abstractions (etc., etc.), is capable of neutralising this latest 'difficulty'. After all, mirrors can and do distort reality -- at least with respect to left-right symmetry, or even in relation to an object's shape or size, in, say, a hall of mirrors (etc.). Few are taken in by this. Moreover, it could be maintained that when other criteria are taken into consideration (such as, increasing consistency, greater explanatory and predictive power), defective ideas can be weeded out as part of a successful search for an increasingly accurate, all-embracing theory of the world --, and, of course, how to change it.
[RTK = Reflection Theory of Knowledge.]
Or so it could be objected, once more...
Maybe so, but mirrors can't reflect what isn't there. Hence, if language and thought were indeed 'mirrors' (or even lenses, to vary the metaphor) -- distorting or otherwise -- we would have to conclude that everything that can be and is expressed in language must exist in reality. Even though they might distort things, mirrors can't conjure into existence objects and processes that aren't there. But -- disciples of Meinong excepted --, who in their left mind is prepared to admit that whatever language contains must exist -- or 'subsist' -- somewhere in nature and society? Who wants to allow for the existence of, say, Harpies and Gorgons -- even in a distorted form -- simply because we have words for them?
On the other hand, if it were so easy to incorporate such 'entities' into 'Being' by the simple expedient of naming them, why bother looking for any evidence in support? In fact, if this approach to knowledge were viable, any such search that went beyond leafing through every Dictionary, Thesaurus, Encyclopaedia of Mythology, Textbook of Grammar or Phone Book on the planet would be superfluous. In that case, Science itself would become a sub-branch of Lexicography, or even of Hermeneutics.
It could be argued that even mythical beasts and fictional characters are composed of 'images'/.components' (i.e., colours, sounds, shapes, textures, etc.) that have been derived from experience. That is partly where human cognition and judgment often go wrong: they 'knit together' some of these elements in incorrect and fanciful ways.
Figure One: A 'Dialectical' Harpy?
For example, a Harpy is formed from a combination of human and animal images and characteristics, but experience tells us that these beasts don't exist. Hence, while we can certainly imagine all sorts of 'possible beasts' or 'possible objects', only some of them actually exist (as far as we know).
Or, so it could be maintained...
That specific response will be tackled in Essays Three Part Five and Thirteen Part One. Suffice it to say here that:
(a) The theory under review in this Essay is that it is words not 'images' that supposedly reflect reality. In that case, the 'reflection' metaphor seems to be committed to the view that if we have words for something, it must exist; and,
(b) If instead we were to rely on 'images' as a basis for knowledge (even if the latter were only approximate, partial or temporary), we would be 'trapped' in a solipsistic universe. [I have dealt with this in much more detail in Essay Thirteen Part One (link a few paragraphs down).]
Of course, anyone who accepted the theory that words 'reflect reality' would have serious problems specifying the 'ontological equivalent' of prepositions, conjunctions, adverbs, definite or indefinite articles, and the like. Precisely what is it in the world that these parts of speech 'reflect'?
It might be wondered why anyone who thought that 'images' somehow 'reflect' the world and are the basis of our knowledge (as Lenin, for example, certainly did) would (allegedly) be 'trapped' in a "solipsistic world". The answer is quite simple: in such circumstances there would be no way of validating any of these 'images' except by checking them against yet more 'images'. Hence, there would be no independent way of ascertaining whether or not they were figments of the imagination or genuinely represented the world. After all, no one can 'jump out of their heads' to check.
An appeal to practice (or even to science itself) would be no use, either, since all that such a 'trapped' individual would have access to would be images of practice (and images of science). Nor would it help referring to 'commonsense', or even to the "naive beliefs of ordinary people" (as Lenin attempted to do). That is because, once more, all that such a 'trapped' individual would have are images of what constituted 'commonsense' and images of ordinary folk and their beliefs. That is why it was asserted above: "In such circumstances there would be no way of validating any of these 'images' except by checking them against yet more 'images'." Hence, as noted above, they would be 'trapped' in a world composed of their own 'subjective images' -- a "solipsistic world" -- if this theory were true.
[That is a greatly shortened version of a much more detailed argument developed in Essay Thirteen Part One.]
Putting even this worry to one side, it might be difficult, too, for anyone who accepts this interpretation of language to explain how words for non-existent beings (such as Harpies and Gorgons) -- even if they were based on 'images' stored in each dialectical head -- can be harmonised with the Marxist view that language as a social phenomenon. [I have discussed that specific topic in Essay Twelve Part One; readers are directed there for more details.]
However, the specific point under consideration here was in fact the following counter-response:
A reinterpretation or reconfiguration along these lines might then allow dialecticians to draw substantive conclusions about the world by applying the concepts and categories developed in and by dialectical thought. Howsoever the latter actually got there, the theories that result from this process would still only approach absolute truth asymptotically...
We have already established that DM-theorists go way beyond seemingly modest disavowals like these, and claim to know what fundamental features of reality are -- valid for all of space and time -- derived solely from the alleged meanings of certain words.
Some might think to mention ideology here (in order to try to account for falsehood), but that can't affect the veracity of the above comments themselves. Ideology supposedly 'inverts' things. But, even if that were an apt metaphor, ideology (if based only on 'images') can't invert or reflect what isn't there and has never been there.
[Of course, the 'reflection' metaphor itself might not be apt, anyway. I will say more about that in Essay Three Parts Four and Five. Until then, see here.]
It could be further objected that ideologies invent things all the time. Maybe so, but on the basis of an image-centred, or even a "words reflect reality", sort of theory (both of which are central to DM-epistemology), such inventions would still only amount to a re-shuffling of 'images' that are already there, which, according to this theory, may only 'reflect' what is actually there in 'reality'. If so, this (supposed) inversion, too, would simply amount to the recycling of images already present (if DM-epistemology were to be believed).
Moreover, an earlier reference to the hermeneutic gyrations required to make this theory work was deliberate. The word "hermeneutics" is in fact derived from the Greek 'deity', Hermes, the 'Interpreter of the Gods'. It was specifically chosen because of the numerous accusations advanced at this site that DM is just a re-vamp of Hermeticism, minus the use of openly religious language.
These allegations are also linked with another ancient idea that both Philosophy and Theology were invented by Hermes (or, in Egypt, by 'his' equivalent, Thoth -- on that see Boylan (1999), Faivre (1995), and Fowden (1993)). Of course, Philosophy as a discipline was invented by open and honest ruling-class theorists, but it was also an important ideological weapon in the class war aimed at basing the 'legitimacy' of that class on the supposed thoughts and intentions of the 'gods'.
[Why that is so will be explored in much more detail in Essay Twelve Parts Two and Three (summary here), where the phrase "ruling-class theorist" will also be more fully explained. Until then, see here, here, here and here.]
Furthermore, the above claims aren't as wild as might at first sight seem (to some). In fact, they are based on what Marx himself had to say:
"Feuerbach's great achievement is.... The proof that philosophy is nothing else but religion rendered into thought and expounded by thought, i.e., another form and manner of existence of the estrangement of the essence of man; hence equally to be condemned...." [Marx (1975b), p.381. I have used the on-line version, here. Bold emphasis and link added.]
[Indeed, it is arguable that this is part of the reason why Marx abandoned Philosophy in the mid-, to late-1840s; on that, see here.]
This ancient approach to knowledge -- which has in one form or another dominated much of 'Western' and 'Eastern' thought ever since -- sought to connect the obscure philosophical jargon (expressly invented for this purpose) with the divine, 'rational', a priori structure of reality (i.e., with 'Being' Itself).
It is that observation which partially motivates the claim advanced in these Essays that Traditional Thought represents, and is an 'image' of, not the material world, but ancient ruling-class interests and priorities -- as well as picturing an Ideal World that supposedly 'exists' anterior to experience, which is more real that the universe we see around us. Ruling-class hacks have consistently argued that this 'hidden world' -- which is accessible to thought alone -- underlies 'appearances' and lends to reality its 'substance', its 'essence'. That world is thoroughly Ideal -- indeed, as George Novack pointed out earlier.
Given this traditional approach to knowledge, it is the language invented and used by such theorists that tells them what this hidden world must be like -- since this is the only way they can access it. Hence, this 'hidden world' is a reflection of specific forms-of-thought and specific forms-of-language --, not the other way round. [As noted earlier, I call this the RRT, which is a sub-branch of LIE. More about that in Essay Twelve Part Four, when it is published.]
In each Mode of Production, different versions of the same general belief in the divine, a priori structure of 'reality' have been used by Traditional Thinkers to rationalise and 'justify' the wealth and power of contemporaneous ruling elites, and thereby the different relations of production that have dominated human beings as each Mode of Production rose and fell. It is precisely here -- where dialecticians have accepted, appropriated and imported into Dialectical Marxism significant aspects of this ancient world-view -- that ruling ideas have succeeded in ruling (otherwise) militant minds.
Some might object that philosophical ideas can't have remained the same for thousands of years, across different Modes of Production; that idea runs counter to core ideas in Historical Materialism. I responded to that objection elsewhere in this Essay.
[This topic will be discussed in more detail in Essay Fourteen Part One (summary here); the pernicious and deleterious effects these ruling-class thought-forms have had on Dialectical Marxism were exposed here.]
Admittedly, the (proffered) pro-DM-account of the origin of mythical beings is more sophisticated than the brief comments in this Essay might seem to suggest. But, a distorted view of reality, howsoever it is produced, whether or not it is a result of alienation or is based on a "one-sided" theory/ideology, or, indeed, is a spin-off from the process of abstraction itself, even if it results in an upside down image, a blurred one, or even one wearing a pink tutu, it matters not. It is still a view of reality (given the applicability of the reflection metaphor, sophisticated version or otherwise). In that case, as we have seen, it is an Ideal view. A mirror can't invent. Hence, this metaphor clearly implies that things like dragons, fairies, ghosts and hobgoblins -- not to mention Atlantis, heaven, hell and even Nowhere -- must exist, in some form or other, just because we have the words for each of them!
On the other hand, if such 'entities' don't exist, then the 'words-mirror-reality' metaphor is defective and should be dropped.
[DL = Dialectical Logic.]
Of course, it could be objected that raising superficial objections like those above, based on certain contingent features of mirrors, or even the world, entirely miss the point. Dialecticians are interested in the essential nature of reality, and they are reflected in (or by) DL.
Nevertheless, more-or-less the same objections can also be levelled against the principles supposedly encapsulated in (or by) DL. But far worse, as we have seen (here, here, here, here and here), DL is far too vague and confused to be able to 'capture' anything, distorted or otherwise.
Or, to put the same point in reverse: if the 'essential nature of reality' were genuinely reflected in (or by) DL, reality would be a complete madhouse. Something like this, in fact:
Figure Two: What The World Might Look Like If DL
Were An Accurate 'Reflection' Of It
Furthermore, since these general or 'essential' features of reality are often highly abstract (or they are expressed in suitably abstract language), the contention advanced here -- that the supposed 'general or essential features of reality' are the direct result of theorists' misconstrual of rules of grammar, they aren't 'truths' in any shape or form -- has more than a little prima facie plausibility going for it.
[Incidentally, the above comments may also count as an answer to the objection that the a priori concepts and categories found in DL capture the form but not the content of reality. Again, since that topic was examined in more detail in Essay Three Part One and Essay Twelve, no more will be said about it here.]
(2) The phrases "relative adequacy" and "relative truth" are themselves completely unclear. Expressions like these are obviously linked to the DM-theory that human knowledge "asymptotically" approaches 'absolute truth' over time, which implies that at any specific point knowledge is incomplete, perhaps even radically so. If that were the case, it would turn out to be inimical to DM itself. That is because it implies humanity is and always will be infinitely ignorant of everything, no matter how "relatively" complete their knowledge might seem to be (at any given point in history). On that basis, far from being "relatively adequate", or even "relatively true", knowledge will always remain infinitely far from the truth, and hence it would possess an infinitely high probability of being false. That in turn is because the difference between a finite and an infinite body of knowledge is itself infinite.
A passage from Engels quoted earlier comes to mind again (which was also commented on in detail in Essay Two):
"The identity of thinking and being, to use Hegelian language, everywhere coincides with your example of the circle and the polygon. Or the two of them, the concept of a thing and its reality, run side by side like two asymptotes, always approaching each other but never meeting. This difference between the two is the very difference which prevents the concept from being directly and immediately reality and reality from being immediately its own concept. Because a concept has the essential nature of the concept and does not therefore prima facie directly coincide with reality, from which it had to be abstracted in the first place, it is nevertheless more than a fiction, unless you declare that all the results of thought are fictions because reality corresponds to them only very circuitously, and even then approaching it only asymptotically…. In other words, the unity of concept and phenomenon manifests itself as an essentially infinite process, and that is what it is, in this case as in all others." [Engels to Schmidt (12/03/1895), in Marx and Engels (1975a), pp.457-58.]
However, Engels failed to say how he knew that knowledge is convergent. Indeed, if what Engels said were correct, paradoxically, it would also mean it was infinitely incorrect. Or, as noted above, it would possess an infinitely high probability of being false. That is because, that claim itself must be infinitely far from the 'truth'. What is more, and as should seem obvious, the theory that the growth of knowledge is an infinitary process can't be confirmed in practice (or, indeed, in any other way). So, this is yet another dogmatic assertion Engels came out with that he not only failed to prove, it can't be proved.
The idea of an asymptotic approach in mathematics is connected with the concept of a limit -- if the limit concerned has been shown to exist. Alas, Engels failed to prove that there is such a limit for knowledge to approach in the required manner. In fact, as noted above, he didn't even attempt such a proof; and, as far as can be ascertained, no dialectician since has bothered to fill in the gaps, either -- or, indeed, shown they are even aware there is a problem here! In that case, Engels's 'mathematical metaphor' is doubly inappropriate; if there is no limit, human knowledge must be divergent. And, if that is so, at any point in human history our knowledge must be infinitely far removed from 'Epistemological Valhalla' -- which, it is worth recalling, still hasn't been shown to exist. Given this view, Engels's inapt metaphor means that humanity will always be infinitely ignorant of anything and everything! As noted above, that is because the gap between a finite and an infinite body of knowledge is itself infinite.
Kant's Noumenon by any other name?
[On convergence, see here. Yes, I know that mathematicians have 'shown' that certain divergent series, those that are Cesàro summable, do 'have a sum', but this area of mathematics is highly controversial and it is far from clear that it will be of any use to Engels and other DM-fans, anyway. That is because these series produce notoriously paradoxical and ridiculously implausible results -- such the following: 1 + 2 + 3 + 4 + 5 +... = -(1/12) -- that is no misprint! This 'result', which has (amazingly!) convinced many leading mathematicians, tells us that the sum of all the counting numbers is -1/12! If any DM-fans want to go down that route, I can only wish them good luck.]
Of course, it could be argued that just because certain iterative functions in mathematics yield infinite sequences that doesn't mean that the distance between any intermediate value given by a partial sum of that function and the point toward which it is converging is itself infinite. For example, the sequence: 1 + 1/2 + 1/4 + 1/8 +...+ 1/2n converges on 2 (as n → +∞), but none of the rational numbers formed from the partial sums of this series are infinitely far from 2.
That isn't strictly true since the (mathematical) distance between any two Rationals is itself infinite (because an infinite number of Reals and Rationals will 'exist', or 'can be counted', in any sub-interval of that interval, if we accept modern Infinitary Set Theory (but, on that see here)). However, even if it were correct, the above would have been an effective response had Engels bothered to prove that the limit he claims exists (implied by the asymptote metaphor) actually does exist. But, since he didn't, it isn't.
The only way the above sceptical conclusions can be avoided would be to deny the search for 'absolute knowledge' is in any way infinitary. Clearly, that would place a condition on the object of knowledge before we knew what it was! Naturally, it would also mean that several passages from the DM-classics (quoted elsewhere at this site) will have to be revised -- or even ditched -- along with the above 'asymptote' metaphor, since they clearly imply such an infinitary task. Indeed, they do more than merely imply it, they say it is infinite, even calling it a "demand".
At this point, it is worth noting that in Hegel's system the existence of 'real contradictions' made some sort of crazy sense (but only if it proved possible to agree with Kant that there are or could be 'real negations', which is partly from whom Hegel got this odd idea; as we will see in Essay Eight Part Two, it isn't possible, and Kant was royally mistaken about this).
Hence, if alongside Hegel we assume that 'Reality' is just "thought" writ large, then it might seem possible, if not entirely legitimate, to project (i.e., "foist") linguistic categories onto reality since, in that case, nature would simply be 'self-developing Mind'. That means this entire approach would (and could) only work if all of 'Reality' were anthropomorphised (i.e., if human categories were read into nature). That done, the supposed fact that objects, events and processes were capable of arguing with (i.e., 'contradicting') one another would cease to be patently ridiculous, but not otherwise.
However, as we will see in Essay Twelve, the idea that nature could be anthropomorphised was itself a throwback to Ancient Greek (and even earlier) religious ideas, where conflicts in nature and society were reconfigured in mythical, quasi-human, 'theological' terms, as a matter of course. That is, where the universe was considered the playground of evil/benevolent forces, 'intelligences', agents and 'spirits'. Anthropomorphic fantasies like these were soon translated into ethical, political, conceptual and purely abstract linguistic form as ruling-class philosophical speculation itself took shape in the theories concocted by the Pre-Socratics (indeed, as Professor Havelock argued).
Finally, on this specific metaphor: as I have shown in Essay Four Part One, language isn't a theory, nor does it contain one. So, it can't 'reflect' anything.
[Readers are referred to that Essay for more details.]
Has Engels's Theory Of Motion Ever Been Tested In Practice?
More to the point: how could this theory possibly be 'tested in practice'? That isn't an academic point, since DM-theorists never tire of telling us things like the following:
"From living perception to abstract thought, and from this to practice, -- such is the dialectical path of the cognition of truth, of the cognition of objective reality." [Lenin (1961), p.171. Bold emphasis alone added.]
"Marxists hold that man's social practice alone is the criterion of the truth of his knowledge of the external world. What actually happens is that man's knowledge is verified only when he achieves the anticipated results in the process of social practice (material production, class struggle or scientific experiment)." [Mao (1961b), p.296. Bold emphasis added.]
Our old friends, Woods and Grant, agree:
"The validity of any theory must be demonstrated, sooner or later, in practice." [Woods and Grant (1995/2007), p.85/89.]
So does John Rees:
"[H]ow are we to be sure that our theory is correct? The answer is that there is a point where the theory and the consciousness of the working class meet -- in practice." [Rees (1998), p.236.]
They aren't alone; here are two STDs who concur:
"We now conclude: practice, the activity of man, is the test of the possibility and extent of his knowing things. If from oxygen and hydrogen I can compose water, then to this extent I have correct knowledge of the nature of water." [Thalheimer (1936), p.153. Bold emphasis added.]
"Dialectical materialism is a philosophy of practice, indissolubly united with the struggle for socialism.... This is the source of all its teachings, and in that service its conclusions are continually tried, tested and developed." [Cornforth (1976), p.125. Bold emphases added. Paragraphs merged.]
[STD = Stalinist Dialectician.]
But, has a single DM-fan ever wondered (out loud, or even during episodes of 'inner speech') exactly which examples of practice have ever, or could ever, confirm what Engels had to say about moving bodies? According to the above, therefore, this theory can't be true. Why on earth then is it accepted by DM-fans?
Of course, as we have already seen, this theory of motion can't be tested in practice.
Throughout history Traditional Theorists -- like Engels, but more particularly, Hegel -- privileged speculation ahead even of a perfunctory search for supporting evidence. Indeed, they assumed that all of nature must be as their 'word-magicked' ideas appear to depict it. The world had to conform to what their theories said, not the other way round. Engels's thoughts on this topic (and others) dictate to the world how it must be and how it must behave, the exact opposite of what he said he would do:
"Finally, for me there could be no question of superimposing the laws of dialectics on nature...." [Engels (1976), p.13. Bold emphasis added.]
When what he should have said is the following:
"Finally, for me there could be no question of not superimposing the laws of dialectics on nature...." [Engels corrected -- but now more accurate and honest.]
But there are other pressing problems this theory faces. It is to these that I now turn.
(5) Explanation Or Re-Description?
Here is the 'controversial' passage, again:
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152.]
In addition to the problems concerning the above words that have been raised in the first few sub-sections of this Essay, the following questions are also worth posing (since DM-fans just don't ask them):
Q1: What exactly is the point of this theory (i.e., that motion is contradictory or involves a contradiction)?
Q2: How does the contradictory nature of motion in any way help explain it?
In relation to Q2: at best, this theory merely seems to re-describe this phenomenon (and in a paradoxical way, too). There is no obvious way in which it helps explain motion. How then can it form part of a scientific theory?
The answer often given (to Q1) is that the point of looking at motion this way illustrates:
(i) How reality is fundamentally dialectical;
(ii) How ordinary thought, commonsense and FL mislead us; and,
(iii) The superiority of DL.
[FL = Formal Logic; DL = Dialectical Logic.]
Responses (i) and (ii) will be tackled throughout the rest of this Essay (and this site), while (iii) will be examined in detail in this sub-section (and, indeed, also throughout the rest of this site).
If DL is quite as 'superior' (to FL) as DM-theorists seem to think it is, we should be able to answer Q2 based on what Engels (or Hegel and Lenin) had to say.
Q2: How does the contradictory nature of motion in any way help explain it?
More specifically:
Q3: How is one 'part' of this supposed 'contradiction' capable of exercising a causal influence over any other 'part' of it?
Q4: How can one or both of the UOs involved in this phenomenon -- presumably, the UO here is the "here" and the "not here" (or more accurately, presumably, it is the moving object in question being "in one place" and "not in it" at the same time) -- how can that actually make anything move?
[A more generalised objection to this way of interpreting movement and change has been aired here.]
[UO = Unity of Opposites.]
The above questions, of course, assume there is a UO of some sort present or at work here, which there would have to be if the 'contradiction' involved is accurately to be described as a 'dialectical', and not just an 'ordinary contradiction'. As noted in Q4, the only viable candidates for such 'dialectical opposites' seem to be the "here" and the "not here" (or more accurately, the moving object itself being "in one place" and "not in it" at the same time). Even so, exactly how these UOs are supposed to 'struggle' with and then turn into each other-- which the DM-classics tell us is an "absolute" -- is something of a mystery. How does a "here" and a "not here" (or an "in one place" and "not in it" at the same time) engage in any sort of 'struggle'? How can a moving object being "in one place" and "not in it" at the same time do what the DM-classics tell us must always happen with such UOs? How does a "in one place" 'struggle' with a "not in it"?
Again, has a single DM-fan in the entire history of Dialectical Marxism asked these rather obvious questions? Or even tried to answer them?
Are you serious!?
DM-supporters no more ask such questions than theists wonder why a beneficent 'deity' put humanity on a planet that is 99.9% molten rock in a universe full of lethal radiation, deadly meteorites and exploding stars! Or, indeed, why they were put on a dangerous rock replete with dangerous organisms/viruses (so that they would need a complex immune system just to stay alive).
However, as Engels depicts things, both 'parts' of the above UO appear to operate together. That is, a moving body is "here" and "not here" -- "in one place" and "not in it" -- all at once, as it were:
"Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it." [Engels (1976), p.152.]
In that case, it looks like such awkward questions (concerning the proximate cause of motion) can't actually be answered by anyone who accepts this theory. That is because the mere fact that a moving body is "here" doesn't appear to be capable of making it become "not here" -- or, more accurately, how a moving body being "in one place" is capable of making it move to "no longer that place". [Henceforth, for simplicity's sake I will just call these two locations, "here" and "not here".] Clearly, there is no 'struggle' going on between this "here" and this "not here" (or none that Engels and Lenin cared to mention). Indeed, this 'contradiction' seems to lack any causal power, any capacity to make something happen or move.
It isn't as if the 'dialectical batteries', so to speak, have run down; it looks more like there don't appear to have been any supplied with the original article, and even of there had been, there is nowhere for them to be plugged in!
More problematic, however, is the following: as we have already seen, 'dialectic objects' don't move from one place to another, since that would take time. Dialectical objects are in both places at the same time! Their 'journey between' "here" and "not here" takes zero seconds.
[On this, see also Note 22b.]
That might help explain why Engels didn't even attempt to construct a causal theory of motion based on the contradiction he claims to have identified here -- and, as far as can be ascertained, no subsequent DM-theorist has made any effort to fill in the details or close the explanatory gap (and that includes Graham Priest!). But, even if a ('dialectical') causal account were eventually to be provided by a DM-fan, one day, it still would be far from easy to see how these 'contradictions' would help explain motion. After all, how does being "here" and "not here" (all at once) explain why anything actually moves? What work does such a 'contradiction' do? Even if you sincerely believe there were any at work here?
It could be objected that this radically misconstrues DM, since the above argument misleadingly splits the assumed 'parts' of a contradiction, one from the other, when DM itself requires contradictions to be constituted by (or to be based upon) "interpenetrated opposites". A dialectical contradiction is a relation, not a thing. Contrary to the above, therefore, DM doesn't depict motion (or change) in such mechanical, causal terms. Dialecticians' various discussions of causation are specifically aimed at countering mechanistic and reductionist accounts like this.
Or, so a response might go...
Nevertheless, even if such a reply were acceptable, no attempt was made by Engels -- and, to my knowledge, none has been made by anyone else since -- to explain how contradictions can have any effect on anything at all, anywhere, anyhow or in whatever preferred causal or 'mediational'/'dialectical' language they were expressed (that is, other than perhaps metaphorically or poetically). [There is more on this in Essay Eight Parts One and Two.] And, contrary to the (proffered) DM-objection (above), this supposed contradiction (in motion) doesn't appear to be relational at all. What precisely is being related to what? What 'relation' is this specific example (i.e., motion) meant to picture or reflect? Is a body related to itself as it moves? Even if it were, how would that make it move?
[One of the best 'dialectical' attempts I have so far encountered (i.e., written by a Dialectical Marxist) that tries to explain the rationale supposedly lying behind this view of motion and change has been taken apart here. In Note 18a I have done something similar (but on a smaller scale) to another attempt to account for motion along Hegelian/Engelsian lines (this time authored by Graham Priest, but, alas, in connection with something that might not actually be a 'dialectical contradiction', to begin with!)]
Of course, it could be countered that it is the dialectical and dynamic relation between bodies and processes that makes objects move and change. That response will be examined below and in more detail in Essay Eight Part Two, as will the idea that contradictions can be accounted for in terms of "opposing forces".
Furthermore, it is difficult to see how a contradiction could exercise any sort of effect on anything else unless it was translated into, or was expressed somehow in, physical or material terms. [That will be attempted below.] At some point, material bodies are just going to have to be moved about the place, and something else material is going to have to make that happen. But, a physically inconsequential word (such as "contradiction") doesn't seem to have the required power -- the necessary oomph, as it were -- to allow it to carry out such a menial task.8
[HM = Historical Materialism.]
Moreover, if the volunteered DM-response above were itself correct (but see below), contradictions wouldn't be any help explaining social change, never mind changes in nature. If no causal role is assignable to contradictions (in DM, with respect to motion, or, indeed, with respect to anything whatsoever!), they certainly can't serve in such a capacity in HM. And if that is so, the (alleged) contradictions at work in Capitalism couldn't themselves make anything actually happen. At best, they would be the result of other things happening, or having happened.
[Incidentally, DM-theorists would now have no explanation for the aforementioned 'anything whatsoever', since any object or process fitting that description won't have been caused by contradictions, either. They would be the result of specific social relations (i.e., if they took place in human society). Again, I have dealt with whether or not even they could legitimately be labelled 'contradictions' in Essay Eight Part Two.]
Given the above (proffered) DM-rejection of any causal role for contradictions in 'dialectics', the cause or causes of social development would now be totally mysterious. In that case, we seem forced to conclude that if there are indeed any contradictions in 'reality', they must play some sort of causal role, at some level, in some shape or form, otherwise dialecticians wouldn't be able to explain why anything actually happened, anywhere.
[Of course, that might be the real reason why dialecticians can't actually explain anything, but they certainly don't see things that way, to state the obvious!]
On the other hand, it could mean that if the development of class society is still to be accounted for in terms of the supposed contradiction between the forces and relations of production -- or even the alleged contradictions between opposing classes -- contradictions themselves could be dispensed with at no loss to HM, since -- given the above (proffered) DM-response --, contradictions would do no work in HM, either, playing no causal role there, too! In that case, the sooner they are pensioned-off the better. Attention could then be focussed on the genuinely causal nature of the above social relations, suitably expressed in appropriately phrased historico-materialist terms. Naturally, that would involve a radical re-write of HM, abandoning much of the traditional, Hermetically-inspired jargon Hegel bequeathed to Dialectical Marxism, which has only succeeded in enveloping much of it in a suffocating philosophical fog for over a century.
If so, this means that dialecticians need to specify -- as a matter of some urgency! -- what, if anything, is so causal about the contradictions they see (literally) everywhere, so that these Hegelian chimeras can at least do some genuine work (in HM). At present they don't appear to be part of the action. At best, they look merely ornamental.
On the other hand, the assignment of a causal role to contradictions in either HM or DM -- so that they cease to be merely decorative -- would generate insuperable problems for both theories, as we are about to find out.
As intimated above, even if it were possible to assign some sort of causal role to contradictions (albeit expressed in suitably acceptable 'dialectical language'), it would still fail to help DM-theorists account for motion. That is because (according to Engels) motion involves a body being in one place and not in it, being in two places at one and the same 'moment'. The problem with that is: How does it actually explain motion causally -- or, indeed, in any other way? What exactly does it add to a scientific account or description of the same phenomenon? All it appears to offer is a paradoxically-worded re-description.
In order to make the last point a little clearer, it might be worth pondering once again (possible) DM-answers to the following questions:
(a) Do contradictions cause motion (i.e., do they make objects move)?
Or:
(b) Does motion merely reveal the presence of contradictions as it unfolds?
Here is Engels, again:
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
On one reading of the above words it looks like it is motion that causes (or creates) these contradictions. So, according to that way of reading the above passage, something must first be in motion for it to bring about a contradictory, simultaneous occupancy and non-occupancy of successive locations (with time having advanced not one instant). But, as we will soon see, that would mean one (or even both) of the following hypotheticals would have to be true:
(i) If there were no contradictions, movement could still take place. [In other words, there could be movement with no contradictions implied.]
(ii) If movement ceased, there would still be contradictions. [In other words, stationary bodies could still involve what appear to be contradictions.]
Taking each in turn:
(A) The relevance of option (i) is brought out by the fact that unless motion was already present no contradiction could be inferred or would have been present.
At the very least, Option (i) prompts a further question: Which came first, the movement or the contradiction? One possible answer might involve what seems to lie behind Engels's comment that such contradictions could be "solved", then "re-asserted", since, on that basis, it looks like motion causes contradictions, not the other way round.
Of course, it could be argued that these two factors (i.e., contradiction and motion) go hand-in-hand. In which case, it makes no more sense to ask which came first, movement or contradictions than it would to wonder: "Which came first, counting or numbers?"
But, as we will see later on in this Essay, there are actual examples of motion (in the real world) where no 'contradiction' is implied, directly or indirectly. So, perhaps that is the case here, too.8a
(B) Option (ii) -- repeated below -- follows from the simple observation that a stationary body can also occupy two places at once, just as it can be in one place and not in it at the same time. [Examples of both of these possibilities are given below.]
In that case, Option (ii) suggests that contradictions aren't a sufficient cause of motion, while Option (i) implies they aren't even necessary.
(i) If there were no contradictions, movement could still take place. [In other words, there could be movement with no contradictions implied.]
(ii) If movement ceased, there would still be contradictions. [In other words, stationary bodies could still involve what appear to be contradictions.]
Furthermore, and with respect to (i), once more: Engels himself appears to have reasoned from what he thought was the case with respect to motion to its alleged contradictory implications. In that case, it looks as if there is no causal role for contradictions to play with respect to 'motion itself', at least, as far as Engels was concerned. That is, there seems to be no way that a contradiction could make anything move. At best, as noted above, such contradictions appear to be conceptually derivative, not causal; they depend on motion, not the other way round. Hence, as things now stand, it looks like bodies first of all move, and only then do contradictions arise -- and, even then, that only applies to our supposed depiction of this phenomenon!
If so, it might be more accurate to say that contradictions operate solely at the conceptual level; they appear to have no part to play in the physical action, on the ground, as it were. Given this 'modified'/corrected view, it would seem that objects in the world just move, but they don't do so because they (first) become embroiled in literal contradictions.
[So, for example, moving bodies don't argue among themselves about the occupancy or non-occupancy of this or that particular "place", which would be the clear implication of the ordinary, literal use of the verb "to contradict". Nor do they become entangled in 'time-and-motion' wrangles about who or what was where, when or why. Again, they would have to do that if literal contradictions (as opposed to a figurative, DM-extension to this word) were implied in such circumstances. (On this, see an earlier sub-section, as well as Essay Eight Parts One and Three.)]
In fact, given Engels's theory of motion, it seems that it is we who derive these paradoxical conclusions in our attempt to depict something that just takes place (without any such fuss) in nature and society.
In other words, according to this interpretation of Engels's views, it looks like the 'fault' lies in us (as it were), not in objects and processes themselves.
Nevertheless, this way of depicting motion would clearly be unacceptable to DM-theorists. They insist that we must begin with material reality -- or our perception of, and interaction with, it --, and not simply start with a description. From there, according to them, we must postulate only those contradictions that actually exist in nature and society -- based, perhaps, on their reflection in human thought, confirmed in practice, etc. In which case, human beings study motion and its attendant contradictions, using whatever conceptual resources they have available to them, which, unfortunately, might not always be up to the job.
Or, so a counter-claim might go...
But, even this (proffered) response is no help. That is because there seems to be nothing in reality that thought could latch onto, or reflect -- and hence, nothing for anyone to abstract from, or reason toward, and hence test in practice -- that even remotely resembles the contradictions postulated by dialecticians.
[Why that is so will occupy the last three quarters of this Essay -- as well as all of this one.]
In relation to Engels's account of motion, as will soon become apparent, there is no clearly specifiable set of possibilities -- or even actualities -- in nature and society with which his description could conceivably correspond. In fact, his words turn out not to be a depiction of the physical world in any shape or form. That isn't because he got the details wrong, or because he failed to capture nature accurately enough --, nor yet because 'reality' is too complicated for us to describe or conceptualise -- it is because his words fail to be a description, to begin with.
Hence, Engels's 'description' of motion isn't just empty or devoid of content, it isn't even one!
It turns out to be far too vague and incoherent to be given that name.
Again, it could be objected that the analysis presented in this Essay is completely misguided since it compartmentalises reality, distorting DM, as that theory was set out by its originators.
In response, it is worth pointing out that we don't have to divide the 'parts' of a contradiction, one from another (or from other relevant aspects of 'reality'), to make the case presented above work.
If each and every contradiction postulated by dialecticians (whether derived from "really existing material forces", or not) is given a sufficiently complex, 'dialectical background' (interconnected within "the Totality", as required by the theory, and verified in practice, etc., etc.), that still wouldn't amount to an explanation of the causal or the "mediated" links their theory requires. Widening the domain (to the entire "Totality" if need be) can't suddenly provide an explanation of how the simultaneous presence and absence of an object in one and the same place at the same time could possibly make it move -- or even how it could account for its movement in any way.
An appeal to forces here would be to no avail, either -- as will be demonstrated in detail in Essay Eight Part Two. Unless forces are anthropomorphised, they, too, can't account for movement and change, in DM-terms.
[That cryptic comment will also be expanded upon in Essay Eight Parts One and Two.]
Are These Even 'Dialectical' Contradictions?
Furthermore, the supposed reflection of contradictions 'in the mind', which might be thought capable of providing the 'conceptual connection' that is imagined to exist between a cause and its effects (or between various mediated items, objects and processes in "the Totality", or even in part of it), can't establish a genuine connection if there aren't any contradictions in reality for it to reflect. Contradictions must have some sort of material basis, constitution or presence if they are to be reflected in thought. As far as materialists are concerned, they can't just be conceptual. And yet, what physical form do the contradictions that are supposed to be involved in motion have?
Of course, it could be objected once more that this is all woefully mistaken. Contradiction arise from a relation; they don't assume an 'independent material form'. In which case, the above paragraph is misguided in the extreme.
Or so it could be claimed...
However, when we ask what this supposed relation consists in no answer returns. Certainly DM-theorists have yet to say what this "relation" amounts to with respect to moving objects. As noted above, since this contradiction appears to revolve around the supposed fact that a moving body is here and not here at the same moment, and in two places at once, the relation can't simply be these two places, this "here" and that "not here". The DM-classics are quite clear: for something to count as both dialectical and contradictory:
(a) There must be a 'dialectical relation' between a pair of 'dialectical opposites', such that they imply one another, and to the extent that one can't exist without the other -- like the relation between the proletariat and the capitalist class;
(b) These 'opposites' must (at some point) struggle with each other; and,
(c) They must turn into one another.
[Dozens of proof texts, quoted from the DM-classics, along with even more passages from 'lesser' DM-works that support all three contentions, have been posted here.]
This now highlights a serious problem: Is what Engels speaks about here (in relation to moving objects) even a 'dialectical relation' to begin with? How can a "here" possibly struggle with a "not here"? How can the two places a moving body occupies at the same moment 'struggle' with one another? Even worse, how on earth do they turn into each other? If a moving body is located at P1 and P2 at the same time, how does P1 manage to struggle with and then change into P2? Surely, P2 remains P2 throughout. Even if P2 were to change into P1, that would imply the moving object in question had actually gone backward!
Consider moving object, B, again: Let us suppose the two opposites in this case are, "B is here" and "B is there", at the same time, or even "B is here" and "B isn't here", at the same moment. The question is, "How on earth do these struggle with and then change into one another?" If "B is here" were to change into "B is there" and "B is there" were to change into "B is here" (which is what the DM-classics say must happen with all such opposites) then, clearly, nothing will have actually changed! Or as noted above, the object will have reversed direction!
Furthermore, do the above candidates in this presumed relation even count as 'dialectical opposites'? The latter are supposed to imply each other such that they can't exist without one another -- again, rather like the proletariat implies the capitalist class (and vice versa), such that the existence of one supposedly presupposes the existence of the other. How do two locations imply one another such that the existence of one presupposes the existence of the other?
It could be countered that if we consider the Cartesian Plane, each point implies all the rest. That is undeniable, but the Cartesian Plane is a mathematical structure. No such structure exists in the material world, so the question remains: How do two locations imply one another? There is no obvious way they can. [If anyone knows how this is possible, please email me with the details -- and the evidence.]
That being the case -- if these locations don't struggle with and then change into one another, and don't even seem capable of implying each other --, then whatever else this is, it can't be a 'dialectical relation, and hence it can't be a 'dialectical contradiction'.
If so, why is any of this even part of DM?
Not only does it seem to play no role in the action, it doesn't even appear to be 'dialectical'!
[I will have much more to say about this below.]
Are These Contradictions Real Or Are They Merely Fictional?
Returning to an earlier point (no pun intended), unless some sort of physical sense can be given to the idea that contradictions are capable of affecting anything in the required way -- i.e., in reality, not just 'in the mind', in order to provide some grist for the DM-causal, or -mediational mill to grind away at -- unless that were possible, an appeal to 'reflection' fails even to advance the DM-explanation of motion one millimetre (or sixteenth of an inch, if you live in the USA!).
Assuming for the purposes of argument that it could be shown that contradictions do in fact represent or constitute a material (or physical) relation of some sort (between objects or between processes), and which had been abstracted from (or read into) the phenomena (in an as yet unspecified way!) -- they still couldn't account for motion. That is because it would (once more) simply amount to a re-description of the phenomena. We still await the explanatory punch-line: how do contradictions actually make anything move, or even affect movement? What is the material point to this Hegelian 'thought experiment'? Where does the rubber actually hit the road (to use an Americanism)?
If it is now claimed that a causal (mediational) link of some sort between events must be postulated (i.e., if it is just assumed that one exists in order to make the theory work), then that would merely provide a conceptual link between the said events, and such it would remain until the physical details had been filled in. Without the latter, the contradictory nature of motion would remain, at best, a conceptual, not a material, part of 'reality'.
[Of course, that conclusion should come as no surprise to anyone cognisant of the Idealist origin of such an odd use of the word "contradiction".]
If, on the other hand, it is claimed that the mere presence of the said conceptual connection indicates that these causal links must exist in reality -- that is, if the Complex Reflection Theory of Knowledge is assumed to be true (wherein the human mind actively acquires knowledge in practice and via practice, etc.) --, then that would still fail to explain how contradictions are actually capable of causing motion. Precisely how do contradictions succeed in moving things about the place? It would seem that here the dialectical spade isn't just turned, it snaps in two.
Anyway, as should seem clear, any such hasty attempts to repair this theory are the equivalent of imposing it on the facts, which is something DM-theorists at least say they never do.
Clearly, the above difficulties will only be resolved if a clear explanation were given, detailing exactly how contradictions can make anything move (or even keep them moving). The only other way to avoid or circumvent 'problem' like this would be to show how and in what way the above anti-DM argument is itself defective. But there isn't much chance of that happening if we were to rely on DM-fans. They either:
(i) Retreat into a prolonged dialectical sulk (whenever such 'difficulties' are brought to their attention);
(ii) Say they have 'better things to do'; or,
(iii) Simply become abusive.
Be this as it may, as should now also seem reasonably clear, the role that contradictions supposedly play in motion hasn't been helped by a theory that depicts them as:
(a) The product, but not the cause, of motion (implying they are derivative, at best); or,
(b) A consequence of human reflection on 'the nature of motion', or on 'motion in itself' (even if there were such a thing), which suggests such 'contradictions' are merely conceptual, and hence Ideal.
Hitherto, DM-theorists have been content merely to label certain states-of-affairs "contradictory" without (apparently) giving any thought to the lack of explanatory role this empty ritual occupies in their theory. Why call anything "contradictory" and claim so much for that description if no account can be given of how it explains why anything actually changes or moves?
[DM-theorists who even bother to tackle such questions generally point to "opposing forces", or even "instabilities" in a body, process or system, in order to explain how contradictions actually result in change. Those issues have been examined in depth in Essay Eight Parts One, Two and Three. Also see the next sub-section.]
'Internal Contradictions' And Motion
It could be argued that the above objections are irrelevant since DM-theorists are committed to the theory that motion and change are caused by internal contradictions. The above sub-sections seem to be fixated on external causation.9
Unfortunately, in connection with motion, there don't appear to be any internal contradictions capable of impelling objects along. No one supposes -- it is to be hoped! -- that an 'internal contradiction' works like some sort of metaphysical motor, humming away inside a moving object, powering it on its way!9a Nor do there appear to be any 'dialectical struggles' taking place within moving bodies that drive them ever onward (something the DM-classics call an "absolute"). And, that would still be the case even if it were true that every body/process is in fact a UO. No matter how intense this supposed 'internal battle' is supposed to be, a 'metaphysical boxing match' of this sort seems incapable of generating, or maintaining, self-propulsion.
[UO = Unity of Opposites.]
Lenin's "demand", therefore, looks rather impotent (if this is what he meant):
"Dialectics requires an all-round consideration of relationships in their concrete development…. Dialectical logic demands that we go further…. [It] requires that an object should be taken in development, in 'self-movement' (as Hegel sometimes puts it)…." [Lenin (1921), p.93. Bold emphases added. This entire topic is examined in much greater detail in Essay Eight Parts One and Two.]
Furthermore, there don't appear to be any identifiable 'contradictions' situated at the leading edge of a moving body 'dragging' it along, as it were, just as there seem to be none at the back, 'pushing'.
Worse still: both of those scenarios (even if they were remotely plausible) would clearly involve the creation of kinetic energy out of thin air. For example, precisely which 'internal contradiction' is capable of keeping, say, a billiard ball moving?
In that case, with regard to individual bodies, at least, motion can't be an example of change through "internal contradiction".
Or, rather, it can't be such unless DM-fans come up with some new physics to explain it all.
However, as we will see in Essay Eight Part One, part of the problem here is that DM-theorists continually equivocate between two meanings of the phrase "internal contradiction". Sometimes, it refers to:
(i) A logical, "internal relation" between objects and processes.
Sometimes, it merely implies:
(ii) A spatial connection.
Hence, an "internal contradiction" of Type (i) could exist between two widely separated bodies; they would be 'logically' connected, or would 'mediate' and/or inter-define one another. So, for instance, a section of the working class in one part of the globe could still be locked in a contradictory relation with a group of capitalists thousands of miles away. When Marxists speak about class relations, spatial separation doesn't enter in to it.
By way of contrast, Type (ii) 'internal contradictions' would seem to require some sort of proximity in order to work inside a given body, process or system. For example contradictions inside an atom are supposed to be what hold them together. A proton separated from an electron by billions of light years would not normally be held to be 'internally contradictory' to one another.
However, parties/entities locked in relations of either type would still have to 'contradict' each other by 'struggling' among themselves (if the DM-classics are to be believed), but, with respect to those of Type (ii), there would appear to be no (necessary) logical connection implied between them. Such opposites would simply be spatially-, not logically-connected.
In which case, there appear to be at least four possibilities, here:
(a) Something, or some configuration, could be spatially-internal to an object or system without any 'opposites' involved being logically-internal to one another;
(b) Something, or some configuration, could be spatially-internal to an object or system while any 'opposites' involved are logically-internal to each other;
(c) Something, or some configuration, could be spatially-external to an object or system without any 'opposites' involved being logically-internal to each other; or, finally,
(d) Something, or some configuration, could be spatially-external while any 'opposites' involved are logically-internal to one another.
[In fact, there are far more possibilities than these, but further consideration is beyond the scope of this Essay. "Logically-internal" refers to the (supposed) fact that each item involved in the relation implies the other, such that one can't exist without the other. That part of theory was challenged in Essay Eight Part Two.]
However, as I have pointed out elsewhere (slightly edited):
The expressions "internal contradiction" and "internal relation" (or what they supposedly represent) clearly underpin the idea that there are, or can be, internal, 'inter-penetrated' opposites. We can perhaps illustrate what an internal opposite is by recalling what DM-theorists say about the relation between the two main classes under Capitalism, the Proletariat and the Bourgeoisie. It is quite clear that for DM-theorists the Bourgeoisie and the Proletariat presuppose, inter-define and condition one another; each provides the condition for the other's existence; without the one the other not only wouldn't, it couldn't exist. They are thus internally related, not externally or accidentally connected.
This is what dialecticians mean by "interpenetration"; they don't mean these factors spatially interpenetrate one another, but that the one can't exist without the other, nor vice versa; the existence of the one logically implies the existence of the other, and vice versa. And this is where the presumed "contradiction" arises; the Proletariat and the Bourgeoisie are logically locked together, they can't exist independently of one another. This means that they have diametrically opposed material interests which force them into unremitting class conflict. None of this is accidental or external; the interplay between capitalist and worker is both reciprocal and inter-dependent.
Plainly, an external relation doesn't possess these logical properties. Concerning any two items (i.e., objects and/or processes), if they are externally related, the one can exist without the other; they don't presuppose one another, nor do they inter-define each other.
But, as noted above, something can be (i) spatially-internal to an object or system without it being logically-internal, just as something can be (ii) spatially-external while also being logically-internal.
[Indeed, something can be spatially-internal to an object or system and logically-internal to it, too, just as something can be spatially-external while also being logically-external, as well.]
Here is an example that might illustrate (i), above: an Amazonian tribe is logically-external to capitalism (since there seem to be no internal opposites in the capitalist system that condition that tribe and which are conditioned in return by it, or which define it and which it defines in return). Capitalism can live without Amazonian tribes, but it can't live without the Bourgeoisie and the Proletariat. Even so, this tribe would still be spatially-internal to the capitalist system in that it still exists in a Capitalist country, Brazil.
Alternatively, to illustrate (ii): consider the relation between, say, tenants and their landlords. They presuppose and inter-define one another; each is the condition for the other's existence; so they are logically-internal to one another. However, no landlord lives inside his or her tenants, nor vice versa. In that case, landlords and tenants, while being logically-internal to each other are at the same time spatially-external to one another. [Naturally, this assumes each landlord has at least one tenant!]
Of course, one could always say that landlords and tenants are spatially-internal to whatever social or economic system they happen to exist within, and that is the problem. As we will see (in Essay Eight Part One), this ambiguity lies behind the equivocation mentioned above: When we consider wider economic, social, or even physical systems, it turns out that there are in the end no such things as spatially-external 'dialectical' opposites, and hence no spatially-external contradictions! Indeed, it is even arguable that there are no logically-external 'dialectical' opposites either! [See also here.]
Be this as it may, it could be argued that since locomotion and development in a system are the result of forces acting on bodies or processes at work in that system, the contradictory nature of motion can easily be accounted for on the basis of a network of internal, systematically-opposed forces, or "instabilities". This might therefore turn out to be an example of a Type-(ii) , or even a Type (b), "internal contradiction". That would make the unit within which contradictions (and thus motion) occur the whole, not the part, which seems to be the assumption underlying the argument developed in earlier paragraphs/sub-sections of this Essay.
Or, so a DM-reply might proceed...
Naturally, that response would make a mockery of the claim that all objects change through self-development, or that they locomote because they are self-motivated. Just as it would make a mockery of Lenin's contrast between a mechanical, 'external' account of movement/change and a dialectical version of the same. Given this (proffered) modified 'theory', it now turns out that no object would be self-motivated -- never mind what Lenin demanded -- it would be moved by forces internal to the system of which it is a part, but external to any of the objects caught up in that system.
"Dialectics requires an all-round consideration of relationships in their concrete development…. Dialectical logic demands that we go further…. [It] requires that an object should be taken in development, in 'self-movement' (as Hegel sometimes puts it)…." [Lenin (1921), p.93. Bold emphases added.]
"The identity of opposites...is the recognition...of the contradictory, mutually exclusive, opposite tendencies in all phenomena and processes of nature (including mind and society). The condition for the knowledge of all processes of the world in their 'self-movement,' in their spontaneous development, in their real life, is the knowledge of them as a unity of opposites. Development is the 'struggle' of opposites. The two basic (or two possible? Or two historically observable?) conceptions of development (evolution) are: development as decrease and increase, as repetition, and development as a unity of opposites (the division of a unity into mutually exclusive opposites and their reciprocal relation).
"In the first conception of motion, self-movement, its driving force, its source, its motive, remains in the shade (or this source is made external -- God, subject, etc.). In the second conception the chief attention is directed precisely to knowledge of the source of 'self'- movement.
"The first conception is lifeless, pale and dry. The second is living. The second alone furnishes the key to the 'self-movement' of everything existing; it alone furnishes the key to 'leaps,' to the 'break in continuity,' to the 'transformation into the opposite,' to the destruction of the old and the emergence of the new.
"The unity (coincidence, identity, equal action) of opposites is conditional, temporary, transitory, relative. The struggle of mutually exclusive opposites is absolute, just as development and motion are absolute...." [Lenin (1961), pp.357-58. Italic emphases in the original; bold emphases added. Quotation marks altered to conform with the conventions adopted at this site.]
Despite this, even if systematically-opposed forces could somehow be interpreted as contradictions -- or if they could at least be regarded as constituting them in some way -- that would still fail to show how they could explain motion (or, rather, how they could account for any change in motion), let alone how they could initiate it. Nor would it explain the contradictory nature of motion itself. At best, all this would do is appeal to the allegedly contradictory nature of the system of forces that supposedly produced, or changed, any motion already in the system. The fact that a moving body appears to be in at least two places at once (and hence 'contradictory in itself' while moving) is in no way connected to whatever allegedly initiated/changed that motion, or even with whatever now maintains it (if anything does) -- at least not in any obvious way. Certainly, dialecticians have yet to connect contradictory forces themselves with the alleged fact that moving bodies appear to be in two places at once, in and not in at least one of them at the same time. Nor is it easy to see how that might even be done on their behalf (since they appear to have given precious little thought to this problem over the last 140 years, or shown any sign they intend to address it).
Hence, whether or not it is true that movement is caused -- or even 'mediated' -- by a disequilibrium/instability that exists within a system of incipient forces ('internal' or 'external'), that still wouldn't affect the (supposed) fact that once set in motion a body appears to do contradictory things. Given the truth of such an 'internalist', or even 'externalist', account of 'contradictions' and forces (i.e., assuming both Types (i) and (ii) senses of "internal" are correct), the fact that a body is in two places at once is a consequence of this. But, the "in two places at once" (etc.) descriptor -- or its physical correlate -- doesn't also cause motion in addition to the forces at play in the system. Indeed, while forces might cause a change in motion, the alleged contradictory nature of any subsequent movement has no part to play in the action.
So, even if both the 'internalist' and the 'externalist' models were correct, Engels's analysis of motion would still amount to nothing more than a re-description of that phenomenon; it would remain the case that motion makes bodies do allegedly contradictory things, not the other way round. Hence, the 'contradiction' that Engels highlights is still derivative, not explanatory.
It is worth re-emphasising this point: even if opposing forces could explain contradictory motion (which theory has been demolished in Essay Eight Part Two, anyway), the nature of the connection between the supposedly contradictory nature of motion itself and the forces operating on moving bodies still remains to be established. All that the addition of opposing forces has achieved is to account for the origin of one (supposed) contradiction (motion) in terms of another (oppositional forces). The contradictory nature of motion itself is still locked in the descriptive mode -- it does no work. Whether or not forces can explain change in motion isn't being questioned here -- yet. Even supposing they could, the contradictions Engels supposedly saw in moving bodies remain descriptive. We are still owed an explanation as to why a moving body being "here and not here at the same time" and "in two places at once", accounts for or explains its motion, as opposed to merely re-describing it.
Of course, even supposing this theory were correct, change in motion would be causally related to the action of forces, but that just divorces the latter from the contradictory behaviour of moving bodies (a point Engels himself seems to have conceded -- on that, see Note 10 (link below)). So, even if it were the case that opposing forces caused/changed motion, they would still occupy no useful role in any the theory that models motion itself as contradictory. As far as DM is concerned (that is, on the basis of one particular interpretation of it that appears to be inconsistent with what Engels himself said about forces -- again, see Note 10), what seems to be important here is the alleged fact that opposing forces are contradictory. The other notion (about the contradictory nature of motion) still appears to be redundant; it serves no obvious purpose, and, once more, it plays no effective role 'in the action'.10
[As will be argued at length in Essay Eight Part Two, an appeal to oppositional forces in order to account for contradictions, or even contradictory 'totalities', is no less misguided. There, it will be argued (in detail and at length) that not only is there no conceivable interpretation of opposing forces that could account for contradictions (in FL or DL), there is no viable literal or figurative way of modelling contradictions as opposing forces, nor vice versa, either.]
[DL = Dialectical Logic; FL = Formal Logic.]
But even worse: contradictory forces can't account for contradictory motion, anyway. Indeed, they have nothing to do with it. We can see that from the simple fact that where no forces at all are required to keep a body moving (for example, a billiard ball, or any object travelling at constant velocity), dialecticians still view such motion as contradictory.
So, the alleged contradictory nature of a moving body has nothing to do with the forces that set it in motion.
Of course, even more revealing is the fact that in Classical Physics, forces are supposed to change the motion of bodies (i.e., their velocity); this means that the idea that something has to maintain movement (whether that "something" is contradictory or not) depends on an obsolete, Aristotelian theory of motion. That being the case, the fact that contradictions can't provide a causal explanation of motion is all to the good, for if the supposedly contradictory nature of motion caused and maintained movement, much of post-Aristotelian (i.e., Newtonian/Lagrangian/Hamiltonian) mechanics would have to be binned.11
But, if 'contradictions' don't, or can't, explain motion (i.e., if they don't change or initiate it), why situate them at the centre of your theory?
Despite the above, it could be objected that this entire way of looking at DM seriously misunderstands the nature and role of contradictions. As John Rees pointed out:
"[These] are not simply intellectual tools but real material processes…. They are not…a substitute for the difficult empirical task of tracing the development of real contradictions, not a suprahistorical master key whose only advantage is to turn up when no real historical knowledge is available." [Rees (1998), pp.8-9.]
Hence, it could be argued that the problem with the above, anti-DM criticisms is that they substitute an abstract analysis of motion and change for one that should have been focused on real material forces.
Or so it might be objected...
That (partially valid) objection has been considered in detail elsewhere at this site (for example, here, here, here and here), where Rees's and other dialecticians' epistemological and methodological claims have been examined in detail -- alongside a consideration of actual examples of "real material contradictions", to which most DM-theorists appeal in order to illustrate, explain or render their theory 'concrete'. That has been carried out in tandem with the additional claim that DM isn't a "master key" that unlocks all of reality -- when clearly it is intended to be just that!
[On that, see Essay Two.]
The other (anti-DM) claim -- i.e., the objection that 'material contradictions' can't account for change since they, too, are locked in 'descriptive mode' -- will also be revived and re-examined, for instance, here and here, but, more specifically here, here and here.
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152.]
However, one further possibility hasn't yet been looked at (and this introduces a concern that will dominate much of the rest of this Essay): What if it is completely unclear what Engels was trying to say in the above passage?
Indeed, what if it could be shown that he was in fact saying nothing at all comprehensible?
If that were so, it would be completely beside the point whether or not there are any genuine examples of "material contradictions" in nature and society (at least, not as Engels conceived them). Well, no more than there would be any point in Christians, for example, trying to locate the actual Trinity somewhere in outer space. The difficulty here lies not so much with the search itself (in that it might be too difficult or would take too long), but with the nature or the portrayal of the problem itself. That is, the problem whether something, anything, could conceivably answer to, or 'reflect', what turns out to be an incoherent description to begin with, and hence actually be searched for.
If we are offered nothing comprehensible to explore, plainly no search can either commence let alone be contemplated.
[As noted in Essay Six, you can certainly look for your keys if you don't know where they are, but not if you do not know what they are.]
But, is there any substance to this latest (seemingly wild) allegation?
The next few sections aim to show that there is -- and plenty more than enough, too.
Is Engels's Theory Comprehensible?
Before an (empirical, evidence-based) investigation into the 'real' cause and nature of motion can even begin we need to be clear about precisely what it is we are being asked to examine, consider or seek out. But, as we are about to discover, it isn't possible to determine what Engels was even trying to say when he wrote the following about motion:
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152.]
In advance of any attempt made to substantiate or clarify the above claim (about the comprehensibility or otherwise of Engels's theory), several further ambiguities in Engels's account will need to be addressed and resolved.
Engels tells us that a body must be:
"[B]oth in one place and in another place at one and the same moment of time, being in one and the same place and also not in it." [Ibid., p.152.]
Here, he appears to be noting two separate conditions that don't immediately look equivalent:
L1: Motion involves a body being in one place and in another place at the same time.
L2: Motion involves a body being in one and the same place and not in it.
L1 asserts that a moving body must be in two places at once, whereas L2 says that it must both be in one place and not in it, while leaving it unresolved whether it is in that second place at the same, or some later, time -- or even whether it could be in more than two places at once. Admittedly, it could be argued that it is implicit in what Engels says that the latter occur in the "same moment of time", and, further, that if an object also isn't in a given location, it must be in a second place (at the same time), since it has to be somewhere, somewhen. However, since I am trying to consider every conceivable possibility connected with Engels's words, it is possible that he not only didn't actually say any of the above, he didn't even intend to do so. [The significance of those remarks will emerge as this Essay unfolds.]
It is also difficult to see how a moving body can be "in one place and not in it", and yet still be in two places at once. If moving object, B, isn't located at X -- that is, if it is not in X (and we have just been told it isn't) --, then it can't also be located at X (contrary to what Engels asserts). On the other hand, if B is located at X, then it can't also not be at X! Otherwise, Engels's can't mean by "not" what the rest of us mean by that word. But, what then did he mean?
At this point, we might be reminded that there is a special sort of 'dialectical' "not" [henceforth, "notD"] at work here, and it is totally different from our ordinary "not" [henceforth, "notV", short for "vernacular 'not'"], the former of which seems to connote something like this: "NotD = This isn't a 'notV' at all; in fact it means the exact opposite of 'notV'". But, if the meaning of "notD" is quite so malleable, so accommodating, such that it in fact means the exact opposite of "notV", how can we be sure we know what the words "motion" and "place" mean, let alone "dialectical"? If "notD" can mean the opposite of the everyday, ordinary "notV", then perhaps "motion" can also mean "stationary", and "dialectical" can mean "metaphysical" (as that word was understood by Hegel and Engels).
More puzzling still, when a DM-theorist informs us that "notD" does not mean "notV", what are we to say about the "not" in the middle (i.e., the one coloured red)? If "not" can slide about effortlessly in this manner, then perhaps that red "not" might do likewise and mean its opposite, too? If so, when a DM-theorist tells us that "notD" does not mean "notV", who can say whether or not they actually mean the following: "'NotD' does not not (sic) mean 'notV'" -- which, of course, pans out as "'NotD' means 'notV'", at which point the 'dialectical "notD" collapses back into an ordinary "notV"!
A rather fitting 'dialectical inversion' if ever there was one.
These seemingly minor quibbles turn out to be all the more pertinent when we recall that DM-theorists accept the validity of the following dogmatic Hegelian assertion, which few dialecticians fail to quote approvingly (and which we have met several times already):
"Instead of speaking by the maxim of Excluded Middle (which is the maxim of abstract understanding) we should rather say: Everything is opposite. Neither in heaven nor in Earth, neither in the world of mind nor of nature, is there anywhere such an abstract 'either-or' as the understanding maintains. Whatever exists is concrete, with difference and opposition in itself. The finitude of things will then lie in the want of correspondence between their immediate being, and what they essentially are. Thus, in inorganic nature, the acid is implicitly at the same time the base: in other words, its only being consists in its relation to its other. Hence also the acid is not something that persists quietly in the contrast: it is always in effort to realise what it potentially is." [Hegel (1975), p.174; Essence as Ground of Existence, §119. Bold emphasis added.]
If, as Hegel asserts, "Everything is opposite" (emphasis added) then that must also apply to words like "move" and "dialectical"; they must also be their opposites (unless by "everything" Hegel meant its colloquial opposite, "nothing", which he must have done if the above statement applies to his own words!) Hence, if Hegel is right, "move" must mean "stationary" and "dialectical" must mean "metaphysical"!
Anyone who objects to the above hasn't read the DM-classics, where we are told that everything in the universe -- and that must include words, which, it seems, do exist in the universe -- struggles with and then turns into its opposite. [Nor have they read these passages (from other DM-theorists).]
Or are we to conclude that Hegel's words the only thing in the entre universe that gets a pass?
On the other hand, if this theory can only be made to work by fiddling with the meaning of certain words, how is that different from imposing DM on the facts, something Engels, at least, affected to disavow?
But, of course, no one in their left mind actually believes a word of this (that all words mean their opposite!). So, why does anyone on the far left take Hegel seriously? Why have so many been conned into believing that "notD" actually means the opposite of "notV"?
Until DM-theorists come up with non-question-begging criteria that inform us, unambiguously, which words don't 'dialectically' develop into, or mean, their opposites, and which do, the above 'reminder' can be filed away in the rather large and ever-growing waste bin labelled "Dialectical Special Pleading".
Be this as it may, and as we have already seen, if B is in two places at once -- e.g., if it is in X and Y at the same time --, then it can't just be in Y, but must be in Y and a third place at the same time -- otherwise it will be stationary while it is at Y!
[Later on we will return to the above 'problem' in order to ascertain if there is some way, any way, of avoiding such disastrous consequences.]
L1: Motion involves a body being in one place and in another place at the same time.
Returning to the main feature: It is important to be clear about what Engels meant here because L1 is actually compatible with the relevant body being at rest! That can be seen if we consider a clear example -- i.e., where an extended body is motionless relative to an inertial frame. Such a body could be at rest and in at least two places at once. Indeed, unless that body were itself a mathematical point, or were maybe discontinuous in some way, it would occupy the entire space between at least two distinct spatial locations (i.e., it would occupy a finite volume interval -- or more colloquially, it would occupy some space, or take up some room). But, since all real, material bodies are extended in this way, the mathematical point option doesn't appear to be relevant. [Anyway, it, too, will be considered below, as will the 'motion of point masses'.]
A commonplace example of the above would be where, say, a train is at rest relative to a platform. Here, that train will be in many places (not just two), at once, but still stationary with respect to some inertial frame.
Of course, that depends on what we mean by "place"; I will return to consider this topic in more detail later -- here and here -- and again immediately below.
[There are countless examples of this everyday phenomenon, as I am sure the reader is well aware. In this and subsequent paragraphs I will endeavour to illustrate the alleged ambiguities in Engels's account by appealing to everyday situations (for obvious, materialist reasons). However, they can all be translated into a more rigorous form, using vector algebra and/or set theory. In the last case reviewed below just such a translation will be given in order to substantiate that particular claim.]
Unfortunately, as noted above, even this ambiguous case (concerning that train) could involve a further equivocation regarding the meaning of the word "place" -- the import of which Engels simply took for granted (as did Hegel and Zeno before him).
As seems plain, "place" could either mean the general location of a body (roughly identical with that body's own topological shape, and equal in volume to it --, or, on some interpretations of this word/concept, very slightly larger than its volume, so that the body in question can fit 'inside' its containing volume interval!). Alternatively, it could mean something much more general and far less precise (such as the place where you, dear reader, live --, that is, the solar system, specific planet, hemisphere, continent, country, state, county, city, town, village, precinct, neighbourhood, street, building, or even the flat/room where you happen to reside. Clearly, each of these is a place -- as is where you are now sat (if you are seated), stood or lying down (if not!)). On the other hand, it could mean something even more specific, involving the use of a system of precise spatial coordinates (which would, naturally, achieve something similar), perhaps even pinpointing its centre of mass, using that to locate the body in question more exactly, etc.
But, the word "place" is even more vague/polysemic than the above might suggest (on that, see here), something that will be used to great effect throughout the rest of this Essay.
So, if we now ignore the verb form of "place" (as in "Place you luggage in the rack above your head!", or "Place your bets!"), "place" can mean all sorts of things. For example, it can connote a position in a queue (which might or might not be physically occupied by someone -- for example, it might be a queue on the phone, when one is "put on hold"), or in a team (as in "She regained her place in the squad last week!"). It could refer to the order in which someone was positioned in a race (as in "She finished the marathon in second place"), or to a page in a book (as in "I lost my place in the novel I was reading because the damn bookmark fell out!"). It could even mean something far less tangible (as in "There's a special place for her in my life", "There's a time and a place for that, and this isn't it!", "This might be a good place to end the meeting", "It's not your place to raise objections. You're not even in the union!", "You're obviously confused. Your argument is all over the place!", etc., etc.).
However, it might seem (to some) perfectly obvious what Engels meant by "place", but as we will see, it isn't. In fact, this is one more reason why little or no sense can be made of anything he was trying to say in the passage under review, as we will also find soon out.
As noted earlier, Engels might have been referring to the motion of mathematical points, or point masses. But, even if he were, it would still leave unresolved the question of the allegedly contradictory nature of the movement of ordinary (gross) material bodies -- and, how the latter relate to the former. Anyway, since DM-theorists hold that their theory accounts for motion in the real world, the movement of mathematical points -- even where literal sense can be made of such 'abstractions' or, indeed, of the idea that they can move -- won't in general be entered into in this Essay.
[After all, if such points don't exist in physical space, they can hardly be said to move. And even if they could, what on earth do they move into? Other points? How does one point move into another point? In connection with this, it is worth adding that Graham Priest's (otherwise sophisticated) attempt to defend Hegel and Engels (in, for example, Priest (2006)) largely depends on (i) The use of mathematical and metaphysical idealisations like these (i.e., mathematical points, planes, manifolds, 'instants' in time, etc., etc.), and (ii) Interpreting the material world as a mathematical object or model of some sort -- which makes much of what he has to say of little or no relevance to a defence of Hegel or Engels. Quite the reverse, in fact; it would make movement itself either impossible, or far more paradoxical than even Hegel himself imagined -- as we will also discover as this Essay unfolds, for example, here and here.]
Turning to L2 (quoted again, below): this particular option involves further ambiguities that similarly fail to distinguish moving from stationary bodies. Thus, a body could be located within an extended region of space and yet not be totally or wholly inside/in it. In that sense, it would be both in and not in that place, at once, and it could still be motionless with respect to some inertial frame.
Here, the equivocation would centre on the use of "in", in L2. Of course, it could be objected that "in" has been illegitimately replaced by "(not) totally or wholly inside/in", in the previous paragraph. Nevertheless, it is worth noting that Engels's actual words hold it open that that could be a legitimate interpretation of what he meant:
L2: Motion involves a body being in one and the same place and not in it.
If a body is "in and not in" a certain place it can't be totally in that place, on one interpretation of those words, since it is somewhere else also at that time. So, Engels's own words allow for his "in" to mean "not wholly in".
A mundane example of this might be where, say, a 15 cm long pencil is sitting in a pocket that is only 10 cm deep. In that case, it would be perfectly natural to say that the said pencil was in, but not entirely in, the pocket -- that is, in this case it would be both "in and not in" the pocket at the same time (thus fulfilling Engels's definition) --, but still at rest with respect to some inertial frame. No one would think that NN was lying if she said she had a pencil in her pocket, if this were the case. L2 certainly allows for such a situation, and Engels's use of the word "in", alongside the rest of what he asserted, plainly carries such an interpretation.
If so, it seems that Engels's words are compatible with a body being motionless relative to some inertial frame!
What is more, that would remain the case even if L1 and L2 were combined, in the way Engels intended; even then his words would be compatible with there being no movement at all (with reference to some inertial frame):
L3: Motion involves a body being in one place and in another place at the same time, being in one and the same place and not in it.
An example of an L3-type -- but apparently contradictory -- 'lack of motion' would involve a situation where, say, a car is parked half in, half out of a garage. Here the car is in one and the same place and not in it ("in and not in" the garage), and it is in two places at once (in the garage and in the driveway of a given house), even while it is at rest relative to a suitable inertial frame.
In which case, the alleged contradiction that interested Engels can't be the sole result of movement, since his own words are compatible with a body being at rest. Given this way of interpreting such vague and ambiguous language, stationary bodies also appear to be contradictory (until we disambiguate them along the lines suggested earlier).
Hence, what Engels argued isn't unique to moving bodies.
In that case, 'contradictions' like these are clearly a result of the vagueness and ambiguity in Engels's sketchy, Hegelian attempt to describe moving bodies.
Or, it might even be the result of the polysemic meaning of many of the words he used, which meanings he simply conflated.
[That possibility will be explored later in this Essay.]
As we have seen, and will see again as this Essay unfolds, objects at rest relative to some inertial frame can and do display the same apparent 'contradictions' as those that are in motion (and with respect to the same inertial frame, too). Naturally, if bodies at rest share the very same vague or ambiguous features/'properties' as those that are in motion, then Engels's description/theory clearly fails to pick out what is unique to moving bodies.
This isn't a good start.
Alas for DM-fans, it only gets worse...
So, we are still in want of a clear and unambiguous DM-description of motion!
Or, indeed, any indication that such 'contradictions' aren't an artificial consequence of a sloppy and careless use of language.
Of course, it might prove possible to repair Engels's attempt to depict 'dialectical motion', maybe by the use of more precise formulations. I will leave that for others to decide. However, given what we are about to find out (in connection with the sort of language that has traditionally been associated with previous attempts to study motion -- especially when that language has been incorporated into a 'philosophical theory'), global scepticism is perhaps the best policy.
Again, at best, L3 depicts the necessary, not the sufficient, conditions for motion. [But, as we will also see, not even that is true!]
If so, the alleged contradictory nature of L3 has nothing to do with any movement actually occurring, since Engels's words also apply to bodies at rest, which plainly share the same 'necessary conditions':
L3: Motion involves a body being in one place and in another place at the same time, being in one and the same place and not in it.
As already noted, 'paradoxes' like this arise from the ambiguities implicit in the language Engels himself used -- and, as it turns out, in the language he also misused.
[Those issues will be discussed in greater detail below.]
Nevertheless, in the next few subsections several (genuine) attempts will be made to resolve these equivocations in order to ascertain what, if anything, Engels could possibly have meant by the things he tried to say about moving bodies.
A First Attempt At Disambiguation
As was also demonstrated in Essay Six in relation to Trotsky's (and indirectly Hegel's) attempt to analyse the LOI, Engels's theory of motion is far too vague and ambiguous to be of much use.11a
[LOI = Law of Identity.]
It might be a good idea at this point (no pun intended!) to remind ourselves (again!) what Engels tried to say about motion:
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
I now propose the following disambiguation of Engels's rather enigmatic comments in order to ascertain whether or not any sense can be made of them:
L5: A moving body, B, involves a change of place such that:
L6: B is at (X1, Y1, Z1) at t1 and at (X2, Y2, Z2) at t1.
L7: (X1, Y1, Z1) isn't the same place as (X2, Y2, Z2).
[Where, (Xi, Yi, Zi), etc., represent coordinate triples, and ti is a temporal variable. These can, of course, be combined to yield (Xi, Yi, Zi, ti).]
L5-L7 look a little more promising. However, it is worth noting that this clarity has only been achieved because of the introduction of the phrase "change of place", in L5. Unfortunately, if that expression succeeds in bringing out what Engels meant it would suggest that change explains motion, not the other way round. Perhaps this minor difficulty can be circumvented in some way. Again, I will leave that for others to decide.
Still others, of course, might wonder exactly how, given DM, the word "change" could be explained without an appeal being made to definitions that involved the word "motion" (a definition, it is worth remembering, that has yet even to be attempted by dialecticians -- Graham Priest excepted, of course). Naturally, the use of that term wouldn't alter the validity of L5, but it would make it entirely circular.
However, even if this minor problem were in some way resolvable, the initial promise L5-L7 seemed to hold out soon evaporates when it is recalled that propositions like these fail to rule out cases where an extended object might move at a later time, say t2, but not at t1. That is, B could still be stationary at t1, and in two different places at once (because it is just such an extended body, occupying a volume interval -- i.e., it "takes up room"), and is at rest with respect to some inertial frame. Subsequent motion could then take place at t2, not at t1 -- as we saw above with that car.
[What is worse, even a "change of place" can occur where there is in fact no motion involved -- examples of which will be given later in this Essay.]
It is no use objecting that Engels clearly referred to the "same moment" in which all this takes place since we don't know if this same moment is t1 or t2, or is even perhaps a temporal interval. The significance of either of those observation is all too easily lost, but both revolve around the fact that Engels's account is compatible with an object being stationary at t1, and it is no reply to be told that the said object moved later when we are still owed a 'dialectical' description of motion that captures its necessary and sufficient conditions, not a promissory note that the said object will move at some later time.
Anyway, attempts to capture the necessary and sufficient conditions related to the future (predicted or hypothesised) motion of this object will only attract the same objection -- that is, if L5-L7 were replaced with propositions that simply changed the temporal variable to t2, no other adjustments having been being made. In that case, questions will only arise as to why this minor alteration is capable of turning L5-L7 into necessary and sufficient conditions when the use of t1 had failed to do so earlier.
Any who might still be puzzled by the above considerations need only reflect in the fact that L5-L7 are fully compatible with B remaining perfectly motionless at t1 (with respect to the same inertial frame), as well as at any subsequent time.
L5: A moving body, B, involves a change of place such that:
L6: B is at (X1, Y1, Z1) at t1 and at (X2, Y2, Z2) at t1.
L7: (X1, Y1, Z1) isn't the same place as (X2, Y2, Z2).
L6-L8 below will attempt to fix this annoying 'difficulty'. The problem, it seems, lies with L5, since it fails to connect the motion it mentions with the same instant recorded in L6 and L7. In that case, the following adjustment seems to be required:
L8: A moving body, B, involves a change of place only at t1, such that:
L6: B is at (X1, Y1, Z1) at t1 and at (X2, Y2, Z2) at t1.
L7: (X1, Y1, Z1) isn't the same place as (X2, Y2, Z2).
[L5: A moving body, B, involves a change of place such that... ]
Of course, the same caveats could be applied to later instants so that such a description captures the movement of the body in question along its entire trajectory. That would entail the use of "tk" in the place of "t1" in L8 and L6. That complication will be ignored here since it doesn't seem to affect the points at issue. [No pun intended.]
Unfortunately, however, L6-L8 don't appear to imply a contradiction -- that is, not unless it is clear that B is no longer at (X1, Y1, Z1) at t1, since, as we have seen, it is possible for a (stationary) body to be in two places at once. For example, few would regard it as a contradictory feature of reality that a cake, say, could be in a box and in a supermarket at the same time (hence be in two places at once), and stationary with respect to some inertial frame. Or, think about yourself, dear reader; while sat perfectly still in front of the screen you are now viewing (assuming you are seated!), your arms are both in and not in the same place as your legs. So, while your limbs are in the same place as you (i.e., they are also where you are sat -- no one has dismembered you!), they also aren't in the same place as you (i.e., you haven't been squashed into a mathematical point!). In short, even you occupy several places at the same time as occupying only one place (a seeming contradiction!), all the while remaining perfectly still! As we have seen, the word "place" can be highly ambiguous.
On the other hand, if a die-hard dialectician could be found who thought that the above scenario (depicted in L6-L8) was indeed contradictory, they would need to explain to the rest of us exactly what the alleged contradiction there amounted to. That is, they will need to explain how (i) In virtue of B being in two such places at once there was some sort of 'struggle' going on (which the DM-classics tell us must happen in all such cases); and then (ii) Exactly what was 'struggling' with what. If we now switch back to re-consider the cake example mentioned in a previous paragraph: What 'struggle' is taking place there, too, and with what is this cake 'struggling'?
Furthermore, as we will see in Essay Seven Part Three, the dialectical classics also inform us that all such objects turn into their opposites -- that is, they turn into that with which they have 'struggled'. In connection with the cake, once more: if a cake being in two places at once is indeed a contradiction, that would involve it 'struggling' with and then turning into the tin, or maybe even the building, that contained it (if the DM-classics are to be believed)! Since no one in their left mind could reasonably be expected to believe this fantasy, cakes in supermarkets can't be viewed as in anyway contradicting the bricks, mortar and tins that contain them. Any who do so think are advised to seek immediate professional help.
If not, then this, too, can't be a 'dialectical contradiction' and should be disambiguated along lines explored in earlier sections if this Essay.
Of course, it could be argued that the first location of the said cake (i.e., inside a tin) is itself located inside the second (i.e., the building), which isn't at all what Engels meant by the two places a moving body must occupy at the same moment. But, on which set of words that Engels committed to paper is that interpretation itself based? We have already seen that Engels didn't tell us what he meant by "place", so it isn't possible to rule out the above counter-example because of anything specific that Engels himself said. Indeed, as we have already seen, and will see again later, the word "place" is far more complex than Engels, Hegel and Zeno imagined (in their theoretical deliberations).
Nor will it do to be told (again!) that these aren't the sort of 'contradiction' dialecticians are interested in --, at least not until they produce a clear and unambiguous definition of those that do concern them. As we will see in Essay Eight Parts One, Two and Three, they have yet to so.
Anyway, even if these aren't the sort of 'contradiction' of interest to DM-theorists, the whole point of the last few paragraphs (and, indeed, much of the rest of this Essay) is to show that a careless use of words like "move" and "place" artificially creates the 'contradictions' Engels and Hegel claim are implied by moving objects.
In order to circumvent this latest 'problem', we need to replace L6 with L9 and L7 with L7a, as follows:
L8: A moving body, B, involves a change of place only at t1, such that:
L9: B is at (X1, Y1, Z1) at t1 and not at (X1, Y1, Z1) at t1, and B is at (X2, Y2, Z2) at t1.
L7a: (X1, Y1, Z1) isn't the same place as (X2, Y2, Z2), nor is one contained by the other.
[L6: B is at (X1, Y1, Z1) at t1 and at (X2, Y2, Z2) at t1.]
This set (henceforth, "ℒ") certainly looks inconsistent. The question is: Can all of its constituent sentences be false at once? Only if we can rule out that possibility will we be able to construct a contradiction from all and only elements of ℒ.
[At this point it is worth recalling that a set, S, of indicative sentences is inconsistent just in case not all of its elements can be true at once. But, a "contradiction" requires more than this. In the simplest case, the elements of a binary sub-set of sentences taken pair-wise from elements of S are contradictory just in case (i) Those sentences are inconsistent and (ii) They can't also be false together. Or: in the simplest case, the elements of a binary sub-set of sentences taken pair-wise from elements of S are contradictory just in case they can't both be true and they can't both be false. That necessary condition is invariably overlooked by DM-fans, which, naturally, leads them into regularly confusing contradictions with inconsistencies and contraries -- and, in many cases, with a whole host of other unrelated concepts, into the bargain. (Any who object to the 'pedantry' here should read this, and then maybe think again.)]
The question is: Can all of the elements of ℒ be false at once? If they can, then it won't be possible to construct a contradiction from all and only elements of ℒ. I propose to resolve this issue by considering each of ℒ's constituent sentences in turn, but in reverse order:
(1) L9 would be false if at least one of its conjuncts was false. But, the first part of L9 ["B is at (X1, Y1, Z1)"] could be false in several ways: for example, if B is at (X3, Y3, Z3) at t1.
L9: B is at (X1, Y1, Z1) at t1 and not at (X1, Y1, Z1) at t1, and B is at (X2, Y2, Z2) at t1.
In fact L9 is an inconsistent sentence anyway, and hence it is automatically false (either that, or it isn't a proposition in the first place (which is what I would maintain, anyway -- no pun intended!)11b -- depending on which branch of the Philosophy of Logic one accepts). However, since DL is based on the claim that an inconsistent sentence, or pair of sentences, can be true, I have ignored that pre-condition since it would beg the question.
(2) L8 is linked to L9 by means of a "such that" phrase, so the truth or falsehood of L8 is sensitive to the truth or falsehood of L9. Hence, when L9 is false, L8 is, too.
L8: A moving body, B, involves a change of place only at t1, such that....
(3) L7a would be false if (X1, Y1, Z1) were the same place as (X2, Y2, Z2). This would make L9 false, as well.
L7a: (X1, Y1, Z1) isn't the same place as (X2, Y2, Z2), nor is one contained by the other.
In which case, it looks like we can imagine situations in which, while not all of ℒ's elements could be true at once, all could be false at once. This means that it isn't possible to construct a contradiction from all and only elements of ℒ.
Knowledgeable readers will no doubt have noticed the illegitimate way that some of the schematic sentences of ℒ (and others) have been interpreted to derive the above spurious result. The reason for that particular ploy (and what its implications are) will be commented upon presently. Such readers are advised to shelve their qualms until then.
Finally, it could be objected that the above argument is fallacious anyway, since the contradiction to which Engels was referring here is a dialectical; it isn't meant to be a formal contradiction. But, even if it could be shown that ℒ was a contradictory set (in the above sense), in no way could it be, or express, a 'dialectical contradiction'. None of the elements of this set imply one another (in a way that, for instance, the proletariat supposedly implies the capitalist class), such that any one element can't exist without the others. Nor is there a 'struggle' going on, which, as we have seen, the DM-classics insist is an 'absolute'. So, whatever else it is, this isn't a 'dialectical contradiction'.
[Readers are referred to earlier discussions of this topic (here, here and here).]
A Second Attempt At Disambiguation
From this point on it will be assumed that the difficulties with Engels's account noted in the previous section (whether or not they are legitimate) can be resolved, and that there exists some way of reading his words that does imply a contradiction, and which manages to distinguish moving from stationary bodies!
Perhaps the following will succeed in so doing?
L10: For some body, B, for some time, t, and for two places, p and q, B is at p at t and not at p at t, and B is also at q at t; p is not the same place as q.
L10 looks pretty contradictory. With suitable conventions about the use of variables we could abbreviate L10 somewhat to yield this slightly neater version:
L11: For some B, for some t, for two places, p and q, B is at p at t and not at p at t, and B is also at q at t.
One point first of all needs underlining: none of the strictures dialecticians impose on the LOI can be allowed to stand if L11 is to be of any use, otherwise we would lose the ability to talk about "the same body", "the same time" or "the same place". These concerns would also affect the application of established conventions governing the use of phrases such as "same variable", "same meaning" and "same reference". Hence, if we are to depict the contradictory nature of motion successfully we are forced to accept as valid the application of the LOI when is comes to the use of the same words and the same variables ranging over temporal instants (but, note, applied as a rule of language, not a 'logical truth' (or, indeed, any sort of truth); why that distinction is important is explained in Essay Twelve Part One). Since protracted instances of motion (plainly!) take place over extended periods of time, we can't appeal to the 'relative stability of language' to fix the (temporary) reference of variables (or, indeed, the (temporary) reference of their ordinary language counterparts), if, according to DM-fans, the LOI isn't applicable in all cases involving motion and change.
[Anyway, the 'relative stability' argument was batted out of the park in Essay Six; see below.]
[FL = Formal Logic; MFL = Modern FL; LOI = Law of Identity.]
But, if the LOI is still to be rejected then Engels's description would become hopelessly and irredeemably vague. Many of the 'spurious' objections rehearsed toward the end of the previous section (in relation to ℒ) depend on ignoring some or all of these conventions; as a result they were (obviously) illegitimate. Of course, that ploy was deliberately chosen since it highlighted this very point: the use of FL-variables is based on conventions that DM-theorists must themselves accept or apply in ordinary discourse (and in informal logic) if Engels's analysis of motion is to be at least minimally comprehensible. However, their acceptance of these conventions would (ironically) undermine their criticisms of the LOI! Naturally, it is a moot point which horn of this dilemma dialecticians will want to "grasp":
(i) Accept Hegel's criticisms of the LOI and sink Engels's (and, indeed, Hegel's own) analysis of motion; or,
(ii) Accept Engels's theory and reject Hegel's criticism of the LOI.
It could be objected that the above comments amount to ridiculous caricature of the case dialecticians make against the unrestricted acceptance of the LOI. The relative stability of both material bodies and linguistic expressions permits talk about such things as the "same body", "same word", "same place", "same variable", "same moment", and so on. Dialecticians do not flatly deny or reject the LOI. They simply point out that that law is only true within "certain limits". In addition, they hold that objects and processes undergoing change possess "identity-in-difference"; so while they are approximately identical from moment to moment, they are also at the same time different.
Those responses were examined in detail in Essay Six; the 'relative stability argument', for example, was neutralised here, here and here. 'Dialectical contradictions' themselves were analysed earlier in this Essay -- as well as: here, here, here, here and here.
Of course, hard-nosed dialecticians might choose to ignore MFL altogether. That is, of course, their right (not that they need my permission or acquiescence!). But, as a result of unwise moves like this they will find it rather difficult to specify exactly what Engels actually meant in the quoted passage above.
Be this as it may, that rather desperate move will also be blocked later on in this Essay.
Unfortunately, however, even as it stands, and despite the foregoing considerations (that is, if the contentious claims made above about the LOI and MFL are misconceived and were as a result withdrawn by the present author), L11 would still fail to be a logical contradiction, and that is because of several further, even more annoying and troublesome ambiguities.
In fact, this new batch turns out to be far more intractable than the relatively minor quibbles we have so far met.
This new set of equivocations revolves around the supposed reference of the "t" variable in L11:
L11: For some B for some t, for two places, p and q, B is at p at t and not at p at t, and B is also at q at t.
It is always possible to argue that L11 really amounts to the following (a translation into more ordinary language will soon follow!):
L12: For some B, during interval, T, and for two 'instants', t1 and t2, where both t1 and t2 are elements of T, such that t2 > t1, and for two places, p and q, B is at p at t1, but not at p at t2, and B is at q at t2.
[In the above, t1 and t2 are sets of nested sub-intervals themselves, which can be put into an isomorphism with suitably chosen intervals of real numbers; hence the 'scare' quotes around the word "instant" in L12. (Incidentally, t2 > t1 means that t2 is later than t1.)]
Clearly, the implication here is that the unanalysed variable, t, in L11 actually represents a temporal interval, T (as opposed to an instant in time, the latter of which is a mathematical 'abstraction' that, according to Trotsky at least, can't exist!), and which alternative was expanded upon in and by L12, during which the supposed movement takes place. Plainly, this would licence a finer-grained discrimination among the sub-intervals of T (e.g., resulting in the introduction of t1 and t2) when the said action unfolds.12
Two possible translations of L12 into less formal/intimidating language might read as follows:
L12a: A body, B, observed over the course of a second, is located at point, p, in the first millisecond, and is located at point, q, a millisecond later.
Or,
L12b: A body, B, observed over the course of a millisecond, is located at point, p, in the first nanosecond, and is located at point, q, a nanosecond later.
And so on...
[Some might reply that the above analysis of motion would in fact freeze it, since it speaks about the body in question being "located" at a certain point at a certain time. So, if a body is actually located somewhere, it can't be moving. That is certainly a point both Hegel and Engels wanted to draw our attention to. It will be addressed across much of the rest of this Essay.]
Indeed, L12, L12a and L12b actually express how motion is normally conceived: that is, as change of place in time, i.e., with time having advanced while the object in question moves.
[The "ordinary view", as I have labelled it, mustn't be confused with what Graham Priest has called "The Orthodox Account Of Motion", classically represented in Russell (1937), pp.469-74 (this links to a PDF of the 2010 Routledge edition, where this specific passage can be found on pp.476-80); cf., Priest (2006), pp.172-75.]
If that weren't so (i.e., if L12 -- and/orL12a/L12b -- were to be rejected for some reason), then L11 would seem to imply that the supposed change of place must have occurred outside of time -- or, perhaps worse, that it 'happened' independently of the passage of time --, which is either incomprehensible (again, as even Trotsky would have admitted, see below), or it would imply that for certain parts of its trajectory a moving object (no matter how low its speed) actually moved with infinite velocity! [That 'inconvenient' consequence was pointed out earlier. Mathematical objections to this specific point will be considered below. In addition, the reason for encasing the word "happened" in 'scare' quotes will also be explained presently.]
L11: For some B, for some t, for two places, p and q, B is at p at t and not at p at t, and B is also at q at t.
L12: For some B, during interval, T, and for two 'instants', t1 and t2, where both t1 and t2 are elements of T, such that t2 > t1, and for two places, p and q, B is at p at t1, but not at p at t2, and B is at q at t2.
And yet, how else are we to understand Engels's claim that a moving body is actually in two places at once? If that were so, a moving body would move from one place to the next outside of time -- that is, with time having advanced not one nanosecond (or even less!). In that case, it would be in one place at one instant, and would 'move' to another place with no lapse of time. Clearly, such 'motion' would thus take place outside of time. But, according to Trotsky, that sort of 'motion' can't exist, for it wouldn't have taken place in time.
Indeed, if that were so, we would lose the right to say that a moving body was in the first of these Engelsian locations before it was in the second. That is because "before" implies an earlier time, which has just been ruled out in this case. Hence, by a suitable induction clause, and in relation to the entire trajectory of a body's motion, it would be impossible for a dialectician to say that a moving body was at the beginning of its journey before it was at the end!
[The precise reasons for saying that have been set out in detail below, but the above conclusion depends on an argument developed here, which readers would be wise to consult first. Trotsky's entirely legitimate worries about 'instants' will also be re-examined, below. The contrary idea that if a body is located at a point at an instant, it must be stationary, will be re-considered below, too.]
The reason why certain verbs (such as "happened") have been put in 'scare' quotes is that if the supposed movement between any two of these Engelsian points take place outside of time, no action verb can be used legitimately, since they imply the passage of time. Theologians who try to inform us that 'god lives outside of time' have also found it impossible to say how such a 'being' is able to do anything if no action verbs can be used to describe what 'he' supposedly 'does'. If so, no object can move between any two Engelsian points.
I'll leave DM-fans to try to solve that knotty problem, which has flummoxed theologians for centuries. One can only wish them 'good luck!'...
Despite this, it might seem that this latest batch of difficulties could be neutralised by means of a stipulation to the effect that whereas time isn't composed of an infinite series of embedded sub-intervals (or, rather, our depiction of it isn't) -- such a condition perhaps depicted by suitably defined nested sub-sets of real numbers --, location is.
[Once more, such a stipulation would have to reject Trotsky's argument that events take place only in time.]
That response would mean that while we may divide the space occupied by a body (as it moves along) as finely, or as fine-grained, as we wish -- so that no matter to what extent we 'zoom in' on its location we would always be able to distinguish two contiguous points in its path, which would then allow a theorist to say that the body in question is in both of these places at the same time --, while we can do that with respect to location, we can't do the same with respect to time!
In short: it would be to stipulate that while space is infinitely divisible, time isn't.
Clearly, that would represent an inconsistent approach to the divisibility of time and space -- wherein we are allowed to divide one of them (space) as much as we like, we can't do the same with the other (time).
In fact, this is actually how and why this alleged 'contradiction' originally seems to have arisen. Indeed, this 'problem' reared its ugly head all subsequent attempts to analyse this phenomenon since it had been inserted there, right from the start, by just such an inconsistent (implicit) assumption about the inconsistent divisibility of space and time.
Hence, it is no big surprise to see it 're-emerge' later on.
Anyway, this inconsistent protocol might at first sight appear to neutralise an earlier objection -- e.g., that even though a moving body might be in two places at the same time, we could always set up a one-one correspondence between the latter and two separate instants, because time and space can be represented in an equally fine-grained manner.
But, plainly, it only achieves that result by stipulating, without justification, that while it is possible to map occupied places onto nested intervals of real numbers (to give them the required density and continuity), it isn't possible to do so with temporal intervals/'instants'.
Continuing on from the issues raised in the previous sub-section: there seem to be three distinct Alternatives with respect to these two variables, location and time:
(1) Time and space are both infinitely divisible.
(2) Infinite divisibility is true only of space.
(3) Infinite divisibility is true of either but not both (i.e., it is true of time but not space, or it is true of space but not time).
Naturally, these aren't the only alternatives, but they seem to be the only ones relevant to matters in hand.
Of course, one of the classical responses to this dilemma ran along the lines that the infinite divisibility of time and space implies that an allegedly moving body is actually at rest at some point. So, if we could specify a time at which an object is located at a given point in space, and only that point, it must be at rest there. This appears to be what Zeno himself concluded; it is at least what his argument implied or what he assumed must be the case given this line-of-thought. Here is Philosopher of Science, John Norton, on this:
"In ordinary experience, we see change everywhere. Flies buzz about. Apples fall from trees. Leaves slowly turn yellow in the fall and are blown off the trees. And so on in innumerable variation. Parmenides held that all this motion is an illusion. Nothing changes. We just have the mistaken impression that it does. This view is an instance of a perennially reappearing form of skepticism: that reality is other than what our senses overwhelmingly tell us. The enduring and fatal difficulty of this form of skepticism is that the evidence of our senses is powerful. Perhaps, with tenacity and even some clever sophistry, a skeptic can shake our confidence in the strength of that evidence. However that falls far short of what skeptics need. They must provide positive evidence for their alternative, fantastical conception: that nothing changes; or that we are brains in vats fed spurious perceptions; or whatever other bizarre imaginings they propose.
"Zeno's paradoxes of motion are targeted at one particular form of change: locomotion -- that a body changes (motion) its position (locus) in space. They seek to show that the idea of ordinary motion is internally contradictory. It is hard to believe that Parmenides and Zeno really believed that motion is impossible. The evidence of our senses is powerful, unrelenting and, I believe, irrefutable. Someone who genuinely believes that all change is illusion would seem to be massively deluded and in the grip of a mad fantasy.
"We can cast a kinder light on Parmenides and Zeno's project if we understand them not to be challenging change, but to be challenging the accounts we give of it. Can we really reason reliably about motion using the concepts we have? We think we can. Zeno says otherwise. Look at them more closely and you will find them to be an internal mess. If that was Zeno's goal, then his efforts have met with great success. In one form or another, his paradoxes involve infinities associated with our normal thinking about motion. His paradoxes have forced us to think with great rigor about these infinities and, in immunizing ourselves from his paradoxes, we have brought greater clarity to these notions....
"We do not have Zeno's wording for his paradoxes. Rather, we are in the unfortunate position that our best account of the paradoxes come from a critic of them, Aristotle. Nonetheless, that is what we have and that is what we must work with. Here is the totality of Aristotle's account in his work Physics, Book 6, Part 9:
'Zeno's reasoning, however, is fallacious, when he says that if everything when it occupies an equal space is at rest, and if that which is in locomotion is always in a now, the flying arrow is therefore motionless. This is false; for time is not composed of indivisible nows any more than any other magnitude is composed of indivisibles.'" [Quoted from here; accessed 10/09/2023. Several paragraphs merged. Norton is here quoting Aristotle (1995b), p.404; Book 6, Part 9. Bold emphases and one link added. A minor typo corrected,]
Nevertheless, as Norton notes, it seems equally clear to others that moving bodies can't be depicted in this way, some arguing further that motion (i.e., continual change of place) must be an 'intrinsic' (a 'necessary' or even an 'inherent'), property -- not an 'extrinsic' (i.e., not an accidental or contingent) property -- of moving objects. This means we can't depict moving bodies in any way that would imply they are also stationary during their movement (unless, of course, it had actually stopped moving). So, while it is moving it can't be pictured in a way that suggests it might not be. In turn, this implies that at all times and places a moving body must actually be in motion, and that is indeed how it later came to be argued, which seemed (to some) to mean that such a body must both be in and not in a given location at one and the same moment.
[That appears to be Hegel's view of the matter, but good luck to anyone trying to find anything that clear in what he had to say about this topic! His 'analysis' has, anyway, been defended with great sophistication in Priest (2006). In what follows I aim to show that he is mistaken about this, too. In that case, it will turn out he hasn't successfully defended Hegel; he has merely exposed the latter's incoherence even more starkly.]
So, here is Hegel himself on this (make of it what sense you can!):
"If we wish to make motion clear to ourselves, we say that the body is in one place and then it goes to another; because it moves, it is no longer in the first, but yet not in the second; were it in either it would be at rest. Where then is it? If we say that it is between both, this is to convey nothing at all, for were it between both, it would be in a place, and this presents the same difficulty. But movement means to be in this place and not to be in it, and thus to be in both alike; this is the continuity of space and time which first makes motion possible. Zeno, in the deduction made by him, brought both these points into forcible opposition. The discretion of space and time we also uphold, but there must also be granted to them the over-stepping of limits, i.e. the exhibition of limits as not being, or as being divided periods of time, which are also not divided. In our ordinary ideas we find the same determinations as those on which the dialectic of Zeno rests; we arrive at saying, though unwillingly, that in one period two distances of space are traversed, but we do not say that the quicker comprehends two moments of time in one; for that we fix a definite space. But in order that the slower may lose its precedence, it must be said that it loses its advantage of a moment of time, and indirectly the moment of space.
"Zeno makes limit, division, the moment of discretion in space and time, the only element which is enforced in the whole of his conclusions, and hence results the contradiction. The difficulty is to overcome thought, for what makes the difficulty is always thought alone, since it keeps apart the moments of an object which in their separation are really united....
"[A]ccording to Aristotle...Zeno says: 'The flying arrow rests, and for the reason that what is in motion is always in the self-same Now and the self-same Here, in the indistinguishable;' it is here and here and here. It can be said of the arrow that it is always the same, for it is always in the same space and the same time; it does not get beyond its space, does not take in another, that is, a greater or smaller space. That, however, is what we call rest and not motion. In the Here and Now, the becoming 'other' is abrogated, limitation indeed being established, but only as moment; since in the Here and Now as such, there is no difference, continuity is here made to prevail against the mere belief in diversity. Each place is a different place, and thus the same; true, objective difference does not come forth in these sensuous relations, but in the spiritual.
"This is also apparent in mechanics; of two bodies the question as to which moves presents itself before us. It requires more than two places -- three at least -- to determine which of them moves. But it is correct to say this, that motion is plainly relative; whether in absolute space the eye, for instance, rests, or whether it moves, is all the same. Or, according to a proposition brought forward by Newton, if two bodies move round one another in a circle, it may be asked whether the one rests or both move. Newton tries to decide this by means of an external circumstance, the strain on the string. When I walk on a ship in a direction opposed to the motion of the ship, this is in relation to the ship, motion, and in relation to all else, rest.
"In both the first proofs, continuity in progression has the predominance; there is no absolute limit, but an overstepping of all limits. Here the opposite is established; absolute limitation, the interruption of continuity, without however passing into something else; while discretion is pre-supposed, continuity is maintained. Aristotle says of this proof: 'It arises from the fact that it is taken for granted that time consists of the Now; for if this is not conceded, the conclusions will not follow.'" [Hegel (1995), pp.273-75. Bold emphases added. Quotation marks altered to conform with the conventions adopted at this site. Several minor typos corrected. I have informed the editors over at the Marxist Internet Archive.]
"If, now, the first determinations of reflection, namely, identity, difference and opposition, have been put in the form of a law, still more should the determination into which they pass as their truth, namely, contradiction, be grasped and enunciated as a law: everything is inherently contradictory, and in the sense that this law in contrast to the others expresses rather the truth and the essential nature of things. The contradiction which makes its appearance in opposition, is only the developed nothing that is contained in identity and that appears in the expression that the law of identity says nothing. This negation further determines itself into difference and opposition, which now is the posited contradiction.
"But it is one of the fundamental prejudices of logic as hitherto understood and of ordinary thinking that contradiction is not so characteristically essential and immanent a determination as identity; but in fact, if it were a question of grading the two determinations and they had to be kept separate, then contradiction would have to be taken as the profounder determination and more characteristic of essence. For as against contradiction, identity is merely the determination of the simple immediate, of dead being; but contradiction is the root of all movement and vitality; it is only in so far as something has a contradiction within it that it moves, has an urge and activity.
"In the first place, contradiction is usually kept aloof from things, from the sphere of being and of truth generally; it is asserted that there is nothing that is contradictory. Secondly, it is shifted into subjective reflection by which it is first posited in the process of relating and comparing. But even in this reflection, it does not really exist, for it is said that the contradictory cannot be imagined or thought. Whether it occurs in actual things or in reflective thinking, it ranks in general as a contingency, a kind of abnormality and a passing paroxysm or sickness....
"Now as regards the assertion that there is no contradiction, that it does not exist, this statement need not cause us any concern; an absolute determination of essence must be present in every experience, in everything actual, as in every notion. We made the same remark above in connection with the infinite, which is the contradiction as displayed in the sphere of being. But common experience itself enunciates it when it says that at least there is a host of contradictory things, contradictory arrangements, whose contradiction exists not merely in an external reflection but in themselves. Further, it is not to be taken merely as an abnormality which occurs only here and there, but is rather the negative as determined in the sphere of essence, the principle of all self-movement, which consists solely in an exhibition of it. External, sensuous movement itself is contradiction's immediate existence. Something moves, not because at one moment it is here and at another there, but because at one and the same moment it is here and not here, because in this 'here', it at once is and is not. The ancient dialecticians must be granted the contradictions that they pointed out in motion; but it does not follow that therefore there is no motion, but on the contrary, that motion is existent contradiction itself.
"Similarly, internal self-movement proper, instinctive urge in general, (the appetite or nisus of the monad, the entelechy of absolutely simple essence), is nothing else but the fact that something is, in one and the same respect, self-contained and deficient, the negative of itself. Abstract self-identity has no vitality, but the positive, being in its own self a negativity, goes outside itself and undergoes alteration. Something is therefore alive only in so far as it contains contradiction within it, and moreover is this power to hold and endure the contradiction within it. But if an existent in its positive determination is at the same time incapable of reaching beyond its negative determination and holding the one firmly in the other, is incapable of containing contradiction within it, then it is not the living unity itself, not ground, but in the contradiction falls to the ground. Speculative thinking consists solely in the fact that thought holds fast contradiction, and in it, its own self, but does not allow itself to be dominated by it as in ordinary thinking, where its determinations are resolved by contradiction only into other determinations or into nothing
"If the contradiction in motion, instinctive urge, and the like, is masked for ordinary thinking, in the simplicity of these determinations, contradiction is, on the other hand, immediately represented in the determinations of relationship. The most trivial examples of above and below, right and left, father and son, and so on ad infinitum, all contain opposition in each term. That is above, which is not below; 'above' is specifically just this, not to be 'below', and only is, in so far as there is a 'below'; and conversely, each determination implies its opposite. Father is the other of son, and the son the other of father, and each only is as this other of the other; and at the same time, the one determination only is, in relation to the other; their being is a single subsistence. The father also has an existence of his own apart from the son-relationship; but then he is not father but simply man; just as above and below, right and left, are each also a reflection-into-self and are something apart from their relationship, but then only places in general. Opposites, therefore, contain contradiction in so far as they are, in the same respect, negatively related to one another or sublate each other and are indifferent to one another. Ordinary thinking when it passes over to the moment of the indifference of the determinations, forgets their negative unity and so retains them merely as 'differents' in general, in which determination right is no longer right, nor left left, etc. But since it has, in fact, right and left before it, these determinations are before it as self-negating, the one being in the other, and each in this unity being not self-negating but indifferently for itself.
"Opposites, therefore, contain contradiction in so far as they are, in the same respect, negatively related to one another. Ordinary thinking when it passes over to the moment of the indifference of the determinations, forgets their negative unity and so retains them merely as 'differents' in general, in which determination right is no longer right, nor left left, etc. But since it has in fact right and left before it, these determinations are before it as self-negating, the one being in the other, and each in this unity being not self-negating but indifferently for itself." [Hegel (1999), pp.439-41, §955-§960. Bold emphases alone added.]
Detailed comments about the second of the above two passages (as it has been interpreted by one particular DM-theorist, James Lawler) can be accessed here; several more remarks will be posted in Essay Twelve Part Five at a later date. This Essay is focussed on what Engels had to say, not so much with what Hegel argued, hence I will say little more about the above in the present work.
If this also represented Engels's view (although I know of nowhere in his writings where he is at all clear about this, but it seems to follow from what he has said), one or other of the above Alternatives must be rejected. [It is also unclear which Alternative Hegel accepts. Email me if you think you can determine which one from the above passages.]
Based on what Engels has said about this, it seems that (1) and (3) must be dropped, leaving (2):
(1) Time and space are both infinitely divisible.
(2) Infinite divisibility is true only of space.
(3) Infinite divisibility is true of either but not both (i.e., it is true of time but not space, or it is true of space but not time).
Nevertheless, it is important to note that the paradoxical conclusions classically associated with these three Alternatives only arise if other, less well appreciated (but often implicit) assumptions are either left out of the picture or are totally ignored (that is, in addition to those mentioned above concerning the continuity of space and the (assumed) discrete nature of time). As it turns out, and as we are also about to discover, the precise form taken by several of these suppressed and unacknowledged premisses depends on what view is taken of the 'real' meaning of words like "motion", "place", "space", "location", "point", "moment" and "instant".
In Essays Three Part One and Twelve Part One, it was shown that philosophical 'problems' like these arise when ordinary words are twisted almost beyond recognition (which criticism, incidentally, was endorsed by Marx), or they are employed in contexts far removed from their ordinary, more normal surroundings. The problems false moves like these create are then further compounded by failing to interpret the results as a new set of conventions governing this novel use of language -- which is what actually happened here -- but viewing them as Super-Empirical facts concerning 'the deep structure reality underlying appearances', valid for all of space and time.
[The justification for these seemingly dogmatic claims can't be entered into in this Essay; I have substantiated them in Essay Twelve Part One -- link above.]
In short, the 'classical' approach to this 'problem' only gets off the ground if linguistic conventions (or rules) are misconstrued as Super-Scientific, Mega-Truths, which express, reflect, or represent 'industrial strength' facts.
This (traditional) approach to 'philosophy' interprets what in fact amounts to a new use familiar words as if that were capable (on its own) of uncovering such Super-Truths, instead of it merely being the by-product of a set of novel linguistic conventions, at best, or plain and simple linguistic confusion/distortion, at worst.
Indeed, this is how ruling-class ideologues in Ancient Greece began to misread and misconstrue the linguistic by-product of social relations (i.e., the aforementioned linguistic rules and conventions) as if they were actually the real relations between things, or those things themselves -- thereby fetishising language. Because of that, such theorists imagined they could 'derive' Super-Truths like these from the 'philosophical' jargon they had invented for that express purpose!
Indeed, as the late Professor Havelock (who was, incidentally, a socialist) pointed out:
"As long as preserved communication remained oral, the environment could be described or explained only in the guise of stories which represent it as the work of agents: that is gods. Hesiod takes the step of trying to unify those stories into one great story, which becomes a cosmic theogony. A great series of matings and births of gods is narrated to symbolise the present experience of the sky, earth, seas, mountains, storms, rivers, and stars. His poem is the first attempt we have in a style in which the resources of documentation have begun to intrude upon the manner of an acoustic composition. But his account is still a narrative of events, of 'beginnings,' that is, 'births,' as his critics the Presocratics were to put it. From the standpoint of a sophisticated philosophical language, such as was available to Aristotle, what was lacking was a set of commonplace but abstract terms which by their interrelations could describe the physical world conceptually; terms such as space, void, matter, body, element, motion, immobility, change, permanence, substratum, quantity, quality, dimension, unit, and the like. Aside altogether from the coinage of abstract nouns, the conceptual task also required the elimination of verbs of doing and acting and happening, one may even say, of living and dying, in favour of a syntax which states permanent relationships between conceptual terms systematically. For this purpose the required linguistic mechanism was furnished by the timeless present of the verb to be -- the copula of analytic statement.
"The history of early philosophy is usually written under the assumption that this kind of vocabulary was already available to the first Greek thinkers. The evidence of their own language is that it was not. They had to initiate the process of inventing it.... Nevertheless, the Presocratics could not invent such language by an act of novel creation. They had to begin with what was available, namely, the vocabulary and syntax of orally memorised speech, in particular the language of Homer and Hesiod. What they proceeded to do was to take the language of the mythos and manipulate it, forcing its terms into fresh syntactical relationships which had the constant effect of stretching and extending their application, giving them a cosmic rather than a particular reference." [Havelock (1983), pp.13-14, 21. Bold emphases and links added; quotation marks altered to conform with the conventions adopted at this site. Spelling modified to agree with UK English. Several paragraphs merged. See also Havelock (1982).]
As a result of this philosophical 'wrong turn' (on that, see below, as well as here), Traditional Philosophers reasoned that the word "motion" itself presented some sort of 'problem'; apparently it implied a 'contradiction', even a 'paradox', which, of course, needed their good services to resolve -- indeed, as we saw John Norton point out earlier. All the while they imagined they were talking about 'motion itself' and not (indirectly) about the distorted meaning of the words they had just used to talk about it (or, rather, about what these words supposedly 'reflected'/'represented'). But, as we will see, since they focused on a strictly limited (and unrepresentative) range of examples, in tandem with a severely restricted set of terms associated with this phenomena (which they also misconstrued), what they had to say about motion in the end was severely distorted. Indeed, few, if any, questioned the original distortion, or fetishisation, that had been inflicted on ordinary words used to speak about motion, change and location -- which linguistic chicanery had conjured into existence these 'philosophical problems', to begin with.
As Keith Thomas pointed out in relation to 16th century magicians:
"It would be tempting to explain the long survival of magical practices by pointing out that they helped provide many professional wizards with a respectable livelihood. The example of the legal profession is a reminder that it is always possible for a substantial social group to support itself by proffering solutions to problems which they themselves have helped to manufacture." [Thomas (1972), p.295. Bold emphasis added.]
The same could be said -- but perhaps with even more justification -- about the 'philosophical problems' invented over the centuries by Traditional Philosophers.
That is because these thinkers came from sections of society that were divorced from the world of collective labour and communal life, whose theories reflected an Ideal view of 'reality' that their privileged life-style seemed to embody or encourage. Their view of the world was also born out of an ideologically-driven denigration of the lives and experience of working people, and hence also of the vernacular and 'commonsense'. [Again, these allegations will be fully-substantiated in Essay Twelve (summary here).]
This then filtered into Marxism. As I have argued elsewhere at this site (slightly edited):
The founders of our movement weren't workers; they came from a class that educated their children in religion, the classics and philosophy. Almost from day one they were spoon-fed ruling-class ideas and forms-of-thought. These were an integral part of an ancient tradition which taught that behind appearances there lies a hidden (often 'spiritual') world, accessible to thought alone, which is more real than the material universe we see around us.
Ruling-class ideologues concocted this world-view because,
if you belong to, benefit from or help run a society that is based on gross
inequality, oppression and exploitation, you can keep 'order' in one or more of
the following ways:
The first and most obvious way is through violence. That will work for a time,
but it is not only fraught with danger, it is costly and stifles innovation
(among other things).
Another way is to win over the majority -- or, at least, a significant
proportion of "opinion formers"/"influencers" (i.e., bureaucrats, judges, bishops, generals,
'intellectuals', philosophers, editors, teachers, administrators, etc., etc.) -- to
the view that the 'present order' either (i) Works for their benefit,
(ii) Preserves and protects 'civilised values', (iii) Is ordained of the 'gods',
or (iv) Is 'natural' and thus can't successfully be fought against or reformed.
Hence, a world-view that helps rationalise one or more of the above is necessary for
a ruling-class to carry on ruling
"in the
same old way". While the content of this tradition may have
evolved in line with each change in the
mode of production, its
form has remained largely the same
for at least two-and-a-half thousand years: Ultimate Truth about this
'hidden world' underlying appearances -- often called "Being", or even a world of
'essences'/'abstractions' -- may be accessed by
thought alone, and hence can be imposed on reality dogmatically and
aprioristically....
So, the non-worker founders of our movement -- who had been educated from
childhood to believe there was just such a hidden world underlying appearances,
and which governed everything in existence --, when they became revolutionaries looked for 'logical' principles in
that abstract world that told them change was inevitable and part of the
'cosmic order'. Enter dialectics, courtesy of the dogmatic musings of that ruling-class mystic, Hegel. The dialectical classicists latched onto this
theory and were happy to
impose it on the world (upside down
or the "right way up"),
since -- because of their early education -- it seemed to them quite natural and
uncontroversial to do so. After all, that is what 'genuine' philosophers are
supposed to do -- or, so
they had been socialised to
believe....
Some might object to the above assertion that certain philosophical ideas have remained the same for thousands of years, across different modes of production, since that contention runs counter to core ideas expressed in HM.
But, we don't argue the same in relation to religious belief. Marx put no time stamp on the following, for example:
"The foundation of irreligious criticism is: Man makes religion, religion does not make man. Religion is, indeed, the self-consciousness and self-esteem of man who has either not yet won through to himself, or has already lost himself again. But man is no abstract being squatting outside the world. Man is the world of man -- state, society. This state and this society produce religion, which is an inverted consciousness of the world, because they are an inverted world. Religion is the general theory of this world, its encyclopaedic compendium, its logic in popular form, its spiritual point d'honneur, its enthusiasm, its moral sanction, its solemn complement, and its universal basis of consolation and justification. It is the fantastic realization of the human essence since the human essence has not acquired any true reality. The struggle against religion is, therefore, indirectly the struggle against that world whose spiritual aroma is religion. Religious suffering is, at one and the same time, the expression of real suffering and a protest against real suffering. Religion is the sigh of the oppressed creature, the heart of a heartless world, and the soul of soulless conditions. It is the opium of the people." [Marx (1975c), p.244. Italic emphases in the original. Paragraphs merged.]
The above comments applied back in Ancient Babylon and Egypt, just as they did in China and India, in Greece and Rome, and they have done so right across the planet ever since. The same is true of the core thought-forms that run through all of Traditional Philosophy -- that there exists an invisible, 'abstract' world, accessible to thought alone, which is more real than the world we see around us -- especially since Marx also argued that:
"[P]hilosophy is nothing else but religion rendered into thought and expounded by thought, i.e., another form and manner of existence of the estrangement of the essence of man; hence equally to be condemned...." [Marx (1975b), p.381. Bold emphasis added.]
And:
"[O]ne fact is common to all past ages, viz., the exploitation of one part of society by the other. No wonder, then, that the social consciousness of past ages, despite all the multiplicity and variety it displays, moves within certain common forms, or general ideas, which cannot completely vanish except with the total disappearance of class antagonisms." [Marx and Engels (1848), p.52. Bold emphasis added.]
This, of course, helps explain why Marx also thought that this entire discipline (Philosophy) was based on distorted language and contained little other than empty abstractions and alienated thought-forms -- and, indeed, why he turned his back on it from the late 1840s onward. [On that, see here.]
Hence, if the world is Ultimately Ideal, it would be uncontroversial, if not perfectly legitimate, to derive Super-Scientific 'truths' about 'Reality' solely from language/'thought' -- indeed, as we also saw George Novack point out earlier.
The classical 'paradox of motion' is based solely on a set of surreptitious and, as it turns out, illegitimate linguistic moves like these. That accusation is itself confirmed by the fact that the acceptance or rejection of one or more of the three Alternatives listed above (repeated below) can't be, and has never been based on any actual evidence. Severally or collectively, those Alternatives are founded on linguistic conventions, overtly or covertly accepted or imposed on the facts by all parties involved in this age-old metaphysical con-trick. As noted above, these conventions are based on what is supposed to be the 'real' meaning of "motion" (and its cognates), or, indeed, on the 'real' meaning of words like: "place", "space", "point", "location", "same", "time", "moment" and "instant" -- all of which are assumed to have one 'real meaning' (known only to the theorists involved, of course!).
(1) Time and space are both infinitely divisible.
(2) Infinite divisibility is true only of space.
(3) Infinite divisibility is true of either but not both (i.e., it is true of time but not space, or it is true of space but not time).
Moreover, the choice of one more of the above Alternatives (as a way motivating a particular, or a preferred, 'solution' to this artificially-engineered 'problem') also depended on the acceptance of one or other of two further Choices (mentioned very briefly earlier):
(A) Even if the specification of the location of a moving body is in no way problematic (in that we can always and uncontroversially declare that a moving body is in two places at once), the specification of the time when that occurs is. So, while time can be divided as much as we like, space can't.
(B) Even if the specification of the temporal history of a moving body is in no way problematic (in that we can always and uncontroversially track a moving body and declare it to be wherever it is at a particular 'instant'), the specification of the moment in time when it is there is -- in that it is both "here and not here" at some specific time. So, while space can be divided as much as we like, time can't.
As far as Choice (A) is concerned, it seems that the focus on (point) instants in time is what motivates or underlies the classical 'problem of motion', while an analogous specification for locations in space hardly raises a eyebrow. The obverse of this, of course, provides the rationale for Choice (B).
With respect to DM, we can see the tension that exists between the above two approaches to this 'problem' in the way that Trotsky, for example, failed to draw the same conclusions about locations in space that he drew about 'instants in time':
"Or is the 'moment' a purely mathematical abstraction, that is, a zero of time? But everything exists in time; and existence itself is an uninterrupted process of transformation; time is consequently a fundamental element of existence. Thus the axiom 'A' is equal to 'A' signifies that a thing is equal to itself if it does not change, that is if it does not exist." [Trotsky (1971), p.64. Bold emphases added.]
So, he nowhere argued as follows:
"Or is a "point" a purely mathematical abstraction, that is, a zero of space? But everything exists in space; and existence itself is bound up with location; space is consequently a fundamental element of existence...." [Edited misquotation of the above; bold added.]
But why not? Unless, of course, he also thought space infinitely divisible, something he denied of time?
How is it possible to declare that an instant in time is an abstraction -- which can't actually exist -- but fail to say the same about point locations in space? It can't be that objects have to be somewhere in order to exist, since they also have to be there at some time, too.12a If, according to Trotsky, instants in time don't exist (since they are mathematical fictions), how is it possible for points in space (i.e., mathematical points in space) to exist? If the one can't exist, how can the other? If so, what on earth are DM-fans talking about when they say things like the following?
"[T]he position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
What exactly are the "places" and "moments" spoken about by DM-theorists like Engels, or even by mystics like Hegel and Zeno? Are such "places" and "moments" mathematical abstractions or are they somehow extra-mental and physical in some way? Search as long and as hard as you like you will find no answer to such questions in the writings of these serial obfuscators, nor even a hint that a single DM-fan has spent so much as one second pondering these questions.
And this is meant to be cutting edge science and philosophy?
Mathematical points are problematic in other respects, too. They have no shape, they aren't spherical, ellipsoidal, cuboid, prismatic or polyhedral -- otherwise they would have parts and wouldn't be mathematical points. Hence, they have no radius or circumference and so they can't be 'occupied' by moving bodies. Clearly they aren't containers -- or, once more, they wouldn't be mathematical points. But, which DM-theorist has ever expressed a single reservation about such rather obvious facts? Of course, in modern Mathematics and Physics issues like this are handled differently, where the functional relation between space and time (with respect to moving bodies) is expressed differently (in the Calculus, for example). But, that can't help resolve this 'problem'. That is because (a) It arose directly out of linguistic confusion and word-juggling, based on a theoretical con-trick stretching back over two thousand years, and (b) Mathematics isn't a description of the world. If it were, nature would be Mind. [Why that is so will be explored in Essay Twelve. I return to this topic again, below.]
Be this as it may, it seems reasonably clear that DM-theorists have in general opted for these two background assumptions: Choice (B) and Alternative (2):
(B) Even if the specification of the temporal history of a moving body is in no way problematic (in that we can always and uncontroversially track a moving body and declare it to be wherever it is a particular 'instant'), the specification of the moment in time when it is there is -- in that it is both "here and not here" at some specific time. So, while space can be divided as much as we like, time can't.
(2) Infinite divisibility is true only of space.
Nevertheless, Alternatives (1)-(3) appear to be among the fundamental issues that have exercised Traditional Philosophers for millennia, and now dialecticians. In their case, however, the preferred 'solution' appears to rule out the possibility of a moving object being in two contiguous places at two different times. This means that DM-theorists have indeed opted for Alternative (2) -- but with the word "indefinite" perhaps replacing "infinite".
(2a) Indefinite divisibility is true only of space.
As has already been noted, this was motivated by a surreptitious exclusion of another option: the indefinite division of time having been ruled out, while that of location hasn't.13
Finally, but more importantly, the 'solutions' offered by Traditional Metaphysicians over the last two millennia are also based on the rejection of at least one implication of the ordinary understanding of motion, part of which is that moving bodies are in different places at different times. [Other aspects of our ordinary concept of motion, ignored by Traditional Theorists, will be explored as this Essay unfolds. Suffice it to say at this juncture that the verb "move" and the noun "motion" posses far more than one meaning in the vernacular. This means that the phrase "the concept of motion" denotes an entire range of expressions, meanings and connotations, not a singular or unique meaning, typical of the 'Orthodox Account' mentioned earlier.] The fact that there is a broad spectrum of meanings available in the vernacular is such a (seemingly) mundane aspect of our everyday grasp of motion and change that it rarely, if ever, features in classical discussion of this topic, except perhaps where it is rejected out-of-hand as far too 'crude' to be worthy of consideration (and this also appears to be the implicit view adopted by DM-theorists).
[Or, it is erroneously conflated with the 'Orthodox Account'.]
However, as we shall soon see (indeed, as we will also discover in several other Essays published at this site), the protocols and conventions of ordinary language and common understanding aren't so easily by-passed, ignored, dismissed, depreciated, denigrated, down-played or hand-waved aside.
Here is Engels, again:
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152.]
However, there are and can be no a priori empirical constraints concerning the length of a temporal interval. In fact, as noted earlier, Engels's account of motion wasn't derived from observation or experiment, nor could it be -- whether or not it had been mediated via the naïve or the sophisticated version of the RTK. Hence, his conception of 'motion in general' can't have been materially-grounded, either; nor, indeed, could his concept of 'abstract motion' (or 'motion-in-itself').
[RTK = Reflection Theory of Knowledge.]
That is because human beings -- aided or not by the use of microscopes, computers, cameras or lasers (etc.) -- do not possess powers of observation fine-grained enough to allow the study of movement at the level of microscopic detail required by Engels's theory. This means that our 'reflective-', or even our supposed 'abstractive-powers', have nothing useable to work with, or upon, in order for anyone to be able to decide what does or doesn't happen to moving bodies in an 'instant'. Nor do any of the machines or devices we employ.
And this isn't ever likely to change.
It is little use objecting that "such-and-such" must be true of 'motion itself', for that would simply confirm the validity of the accusation that these ideas have been imposed on the facts, having been derived from the assumed 'real' meaning of a handful of words, the actual import of which is far less straight-forward than Traditional Theorists would have us believe -- as we are about to find out.
It could be argued that the classical analysis of motion follows deductively from certain incontestable, if not self-evident, premises or indubitable facts. There are only a handful of possibilities that the world could conceivably present us with. Engels's analysis, via Hegel, is based on several of the same.
Or so it could be objected...
Once more, the problem here is that the deducibility or otherwise of these 'contradictory conclusions' (from such premises) depends solely on the use of a limited range of words, the meanings of which have been artificially modified, construed or constrained -- e.g., "move", "place", "space", "point", "location", "same", "time", "moment" and "instant". These words have either been idiosyncratically (re-)defined, or they have had their meanings (implicitly) altered in order to derive the required result. In that case, nothing reliable can follow from their use (as I hope to show as the rest of this Essay unfolds). That was, of course, the point Marx was trying to make -- as was George Novack:
"A consistent materialism cannot proceed from principles which are validated by appeal to abstract reason, intuition, self-evidence or some other subjective or purely theoretical source. Idealisms may do this. But the materialist philosophy has to be based upon evidence taken from objective material sources and verified by demonstration in practice...." [Novack (1965), p.17. Bold emphasis added.]
And yet, the above is exactly what was being attempted in and by the above (proffered) DM-response. It seeks to derive substantive conclusions about fundamental aspects of 'reality' from "abstract reason, intuition, self-evidence or some other subjective or purely theoretical source". The aforementioned "incontestable, self-evident, premises and indubitable facts" are a direct and indirect bi-product of linguistic moves like these, as will also be demonstrated in what follows.
Perhaps even worse, not only does nothing legitimately follow from distorted language -- as should seem obvious --, it is impossible to give a clear sense (or any sense at all) to the classical account of motion (nor, indeed, to more modern versions of it, especially those that have yet to distance themselves from this ancient, defective tradition). In fact, as will be demonstrated in Essay Twelve Part One, all such accounts are non-sensical and incoherent; they not only fail to say anything comprehensible about the world, they can't say anything about anything.
In that case, if, for the sake or argument, it is conceded that humanity possesses an 'abstract' idea of motion (but, even that will be contested below), it can't have been derived by 'reflection', nor can it have been based on anything abstracted from the material world. And these observations become all the more pertinent when it is recalled that the traditional 'abstract idea of motion itself' originated from (i) an inequitable constraint arbitrarily imposed on temporal intervals but excused of spatial locations and, (ii) a ruling-class view of reality (i.e., that there exists an Ideal World behind 'appearances', which is more real than the physical universe we see around us, the nature and existence of which may be inferred from the 'real' meaning of a few words that only Philosophers can understand, as John Norton, pointed out earlier).
In short, Engels's theory wasn't based on 'reflection' (howsoever that word is understood), on evidence, or even on 'abstraction'' It was derived solely from a set of 'concepts' that were themselves the product of ancient stipulations and linguistic conventions, inequitably imposed on 'reality'.
I would be tempting retort: "You just couldn't make this s*it up!", but someone clearly did!
Returning now to a re-examination of several earlier approaches to this topic, consider L11 and L12 alongside Alternative (2a):
L11: For some B, for some t, for two places, p and q, B is at p at t and not at p at t, and B is also at q at t.
L12: For some B, during interval, T, and for two 'instants', t1 and t2, where t1 and t2 belong to T, t2 > t1, and for two places, p and q, B is at p at t1, but not at p at t2, and B is at q at t2.
Alternative (2): Indefinite divisibility is true only of space.
[L12a: A body, B, observed over the course of a second, is located at point, p, in the first millisecond, and is located at point, q, a millisecond later.
L12b: A body, B, observed over the course of a millisecond, is located at point, p, in the first nanosecond, and is located at point, q, a nanosecond later.]
However, if for some reason L12 were to be rejected as an alternative interpretation of L11 -- that is, if it is flatly denied that time is continuous and hence indefinitely divisible, while that is allowed of space; i.e., if Alternative (2a) above is simply imposed on the phenomena --, then there would seem to be no consistent way of ruling out the following as another possible version of it:
L13: For some B, for just one instant, t, for three places, P1, pi and pk, B is at P1 at t, but not at pi at t, and B is at pk at t (where pi and pk are proper parts of P1).
[An everyday example, involving a ship, will be given below to aid comprehension of L13.]
Here, a finer-grained discrimination of location alone means that L13 isn't contradictory, after all. That is because a body can be in two places at once whether or not it is moving (as we have also seen), with no implication that it both is and isn't in any one of them.14
Translated, L13 might be read as follows:
L13a: A stationary body, B, observed over the course of an instant is at (X1, Y1, Z1) and (xk, yk, zk), but not at (xi, yi, zi), where (xk, yk, zk) and (xi, yi, zi) are both located inside (X1, Y1, Z1).
L13b: A moving body, B, observed over the course of an instant is at (X1, Y1, Z1) and (xk, yk, zk), but not at (xi, yi, zi), where (xk, yk, zk) and (xi, yi, zi) are both located inside (X1, Y1, Z1).14a
[The obvious objection that mathematical points like (X1, Y1, Z1) aren't containers and so can't contain other points, has been neutralised in Note 14a (link above).]
In which case, this version also fails to distinguish moving from stationary bodies.
And here is an everyday example of L13: imagine a ship that has entered port. While there, it could be extended across several locations, and hence be in at least two places at once. The ship could also be moving, but with no implication that it is entirely in any one of these locations at one and the same time, or that it is fully occupying any specific location, nor yet occupying every point in the entire port. This also means it needn't be occupying other areas of the port at that time. So, while it is still inside the port it might be being moored next to the dry dock (which is also part of that port), even though it isn't in the staff canteen or the harbour master's office, or in any of the other countless places also in this port. Hence it would be in two places and not in one of them, at the same time.
Moreover, the same possibilities would still apply even if the ship were stationary with respect to some inertial frame, inside this port. Here, this ship could be in one place and not (fully) in all of it, in two or more places at once and stationary, without implying a contradiction. Plainly, that is because this specific example employs a finer-grained division of space to compensate for the arbitrary imposition of the opposite convention on time.14b
Hence, the alleged contradiction vanishes, once again.
[Any who object to my use here of the phrase "not (fully) in", in place of "in", should read this and then perhaps think again.]15
The following is an example of the above type of motion/non-motion (partially expressed in vector algebra):
V1: Let B be a body moving in ℝ3 (or some Vector Space) with respect to a given reference frame.
V2: Let B be at both (X1, Y1, Z1) and (X2, Y2, Z2), at t1.
V3: Let B be a complex composed of n segments, b1 to bn, arranged in an ordered n-tuple, <b1,..., bn>.
V4: Let the position vectors of the centre of mass of b1 and bn be u and v, respectively.
V5: Let v – u = w.
V6: Let the distance between (X1, Y1, Z1) and (X2, Y2, Z2) be mod d, (where d is a vector joining (X1, Y1, Z1) to (X2, Y2, Z2)).
V7: Let mod w > mod d.
V8: Let the direction vector parallel to d be λw (where λ is a Real Number).
V9: Let B be moving at time, t1, with velocity vector, s, such that s = μw (where μ is also a Real Number).
V10: In that case, part of B is at (X1, Y1, Z1), and another part of B is at (X2, Y2, Z2), but other parts of B are at neither of these two points, all at t1.
V11: So, B is moving parallel (assumed to be in one specific sense) to the line joining these two points, also at t1.
V12: Or, alternatively, B is stationary (with respect to some inertial frame); i.e., if s = 0 (or even if μ = 0), and all the above considerations would still apply.
[Two parallel vectors have the same sense (direction) if both λ and μ > 0; opposite senses (directions) if both λ and μ < 0. Follow the above link for more details.]
Here, we have a slightly more technical version of the ambiguous case mentioned above (concerning that ship).
Translated, it could apply to the following scenario:
Ports are generally bigger than ships, and are composed of countless sub-spaces (land, water, buildings, shorelines, etc., etc.). A ship can, therefore, be in port and be located at several points within that port, and yet not be located at every point, with no implication that it is both in and not in the entire port, even though it is both in and not in several parts of it -- for example, it could be in the dry dock, but not in the harbourmaster's office, at the same time. And these could all be the case independently of whether or not it is moving with respect to a suitable inertial frame (that is, if we set s above at zero). Furthermore, all these points could lie along the same line.
Figure Three: A Ship Both In And Not In The Same Place -- In Dry Dock But Not In Those Buildings While Still In Port --,
And In At Least Two Places At The Same Time -- In Cornwall And In The UK, For Example
(Image Credit: Greg Martin/Cornwall Live)
L12 and L13 may now only be rejected on the basis of an ad hoc stipulation -- to the effect that while space can be divided indefinitely, time can't --, but, even then, we have just seen that Engels's half-baked theory still doesn't work!
L12: For some B, during interval, T, and for two 'instants', t1 and t2, where t1 and t2 belong to T, t2 > t1, and for two places, p and q, B is at p at t1, but not at p at t2, and b is at q at t2.
L13: For some B, for just one instant, t, for three places, P1, pi and pk, B is at P1 at t, but not at pi at t, and B is at pk at t (where pi and pk are proper parts of P1).
In which case, of course, the supposedly contradictory nature of motion is, at best, a partial artefact of convention, which itself only works by constraining the divisibility of time but not space. In that case, this (implicit) convention isn't even based on any (supposed) 'objective' features of 'reality'.16
In fact, it is the product of a confused use of abstract, philosophical jargon.
[It should be noted that I don't think conventions are or can be based on 'objective reality', but those with whom I am taking issue certainly talk as if they think that is the case. Or, rather, they imagine their use of language is or can be a 'reflection of reality', which becomes more 'objective' over time if it is 'tested in practice', etc., etc. As we have seen, the linguistic conventions underlying Engels's theory of motion were based on (a) an inequitable constraint arbitrarily imposed on temporal intervals but excused of spatial locations, and (b) an unsustainable belief that he was dealing with the 'real meaning' of words like "motion", "moment", and "space", etc.]
Finally, it could be argued that no matter how much we partition time, no moving body can be located at a certain point (i.e., at one and only one such) at that time, otherwise it would be stationary.
It is to that core idea (and one that Hegel accepted -- as it seems Engels and Lenin did, too) that I now turn.
The Background To Engels's Argument?
So, in response to the points raised in the previous sub-section, some might concede that while it might not be possible to represent or express the contradictory nature of motion in ordinary (or even technical) language,17 nevertheless, motion in the real world must be contradictory.18
That in turn might involve the acceptance of one or more of the following (partly or partially suppressed) assumptions:
L14: An object can't be in motion and at rest at one and the same time (in the same inertial frame).
L15: If an object is located at a point it must be at rest at that point.18a, 18b
L16: Hence, a moving body can't just be located at a point otherwise it wouldn't be moving, it would be at rest.18b1
L17: Consequently, given L14, a moving body must both occupy and not occupy a point at one and the same instant.
In which case, it could be maintained that L14-L16 (or their 'dialectical' equivalent) capture the rationale behind Engels's (and perhaps even Hegel's) analysis of motion.
Indeed, if that weren't so, it would seem to suggest motion was either (a) impossible or (b) illusory, or even that (c) it is a 'stop-go', staccato sort of affair.18c
As far as (c) is concerned, dialecticians often point out that those who reject their particular theory end up talking as if motion is analogous to the way it is depicted in or by film. There, motion on the screen only appears to be continuous when it is in fact discontinuous, composed of rapidly sequenced 'freeze frames', as it were. When played at a certain speed this 'fools' the human eye into 'seeing' continuous movement where there really isn't any. If such a 'quasi-static' view of motion were the case, a 'moving' body (in the real world, not on film!) would occupy a point and be stationary at that point for a fraction of a second, occupy another point an instant later, be stationary there, too, and so on. Naturally, what the said object gets up to in-between each of these stages would be, on this view, entirely mysterious. But, on its own, that wouldn't be enough to invalidate this view of motion. At least, no more than certain quantum 'leaps' (which are discontinuous in this way) would falsify QM -- that is, given the way that motion is depicted in and by Traditional Philosophers.19
[QM = Quantum Mechanics.]
Interlude One -- Moving Pictures
In fact, the analogy with moving pictures creates problems of its own for dialecticians since even 'still' pictures of moving bodies capture motion in temporal intervals, not 'instants in time'.
Of course, this minor niggle can be neutralised somewhat by recalling how flip cards and cartoons work. Consider this 'moving horse', for example:
Video One: Eadweard Muybridge -- The Film That
Confounded Artists
The appearance of motion here is created by a series of still photographs:
"Eadweard Muybridge's famous 'Motion Studies' was the product of the wealth and the whim of the railroad baron, Leland Stanford. Stanford came to Muybridge because he had a rich man's problem. A passionate race horse breeder, he wanted to prove that a horse lifted all four feet off the ground when it trotted -- something that had evaded human perception for millennia. On a specially whited out section of track, Muybridge placed a row of 24 cameras with electric shutters, which would be triggered in sequence, four every second, as the horse passed by. By this means, Muybridge did more than freeze the moment; he took a scalpel to time itself.
"'Muybridge's photographs were the first source of accurate information about the gait of a horse, and it's the beginning of this change where suddenly the camera allows human beings to see faster than our own eyes, to break down the world and dissect motion. It's part of that intrusion into the flow of time. For Stanford, the project was always about horses, whereas Muybridge understood that this was potentially about everything he could possibly find and really create an encyclopaedia of zoological motion.' (Rebecca Solnit)" [Quoted from here; accessed 10/04/2015. Quotation marks altered to conform with the conventions adopted at this site. Link added, several paragraphs merged. On this, see Solnit (2004).]
If anything, this analogy is more closely in tune with one view of change captured by the use of episodic language in ordinary life: that is, where motion takes place in time and has nothing to do with abstract metaphysical 'instants' or 'moments' that Engels, Trotsky and other dialecticians seem fixated upon. In that case, what little evidence there is (from this source -- i.e., from ordinary experience) is consistent with the approach adopted at this site.
Having said that, 'the picture' presented by our use of ordinary language is highly complex; in fact, there is no such thing as the picture in this case. There are many such pictures, just as there many different ways of depicting motion and change -- indeed, as we will discover as this Essay unfolds. Be this as it may, I am certainly not advocating my own 'theory of motion', and that isn't just because I neither have one nor want one. I am merely pointing out that ordinary language isn't as obviously limited and/or defective as many clearly assume. In that case, Marxists have no need of a theory of motion -- at least, no more than they need a theory of Victorian postage stamps or Medieval Icelandic Pottery. That is because there is no such thing as 'motion in itself' for anyone to theorise about.
Admittedly, a 'stop-go' view of motion would present problems for certain fundamental laws in Physics (for example, the conservation of momentum); but if this is indeed how nature behaves, those laws will need revising, anyway. Other significant advances in science have certainly been predicated on overturning what at one time seemed to be the fundamental laws of nature. One thing we can't do is lay down a priori protocols that nature has to obey --, unless, of course, we wish to emulate the approach adopted by notorious Idealists (and, of course, DM-fans).
~~~~~~oOo~~~~~~
In order to reject the 'quasi-static' view of motion (i.e., (c) from earlier -- which held that motion is a sort of 'stop-go', staccato affair), attention might be directed toward one or more of the (following) considerations described by Hegel (each defined in relation to a suitable inertial frame, as necessary). Now, there is no suggestion that dialecticians have actually argued this way (but, as the above link shows, Hegel at least seems to have had many of these issues in mind when he said what he did about motion). Nevertheless, what follows could (plausibly) underpin some of the assumptions and conclusions DM-theorists might very well accept (even if only after having been informed of them by the present author, and for the very first time!):
L18: If a body, B, is located at a point it is at rest at that point.
[Unless otherwise stated, "located" should be taken to mean the said body is situated at/in a specific (non-mathematical) point over a finite temporal interval with respect to a suitable inertial frame. Unless otherwise stated, "point" should also be taken to mean what the ordinary word "space" often means. Note also what (c) from earlier stated: "Motion is a 'stop-go', staccato sort of affair."]
L19: If B is subsequently located at another point, it must be at rest there, too.
L20: Hence, if (c) were also the case, motion would be little more than successive point location. That in turn means it would be the result of one or other (but not both) of the following two scenarios:
(i) Motion involves successive states of instantaneous rest; or,
(ii) Motion involves the sequential existence and then non-existence of what appears to be an identical -- but numerically different -- body at each of the points along its trajectory, with that body falling into non-existence at the end of each successive change of place. That would then be followed by the subsequent entry into existence of a new seemingly identical body at the very next point in line (in the very next 'moment', or even the same 'moment'), giving the impression of motion and continuous existence.
So, we would then have the following sequence of events taking place. At the beginning of its 'journey', 'moving' body, B1, would exist at point, p1; it would then cease to exist there and a seemingly identical body, B2, would pop into existence at the next point in line, p2. B2 would then cease to exist and another seemingly identical body, B3, would pop into existence at the next point in line, p3. This would continue with a succession of seemingly identical bodies, B4, B5, B6..., Bn located at a series of consecutive points, p4, p5, p6..., pn, respectively, thus giving the appearance of motion.
[The above would resemble, for instance, the way that neon lights in a complex sign can be turned on and off in sequence to create the illusion of motion (there is a graphic that illustrates such apparent motion at this site). It looks like Leibniz accepted a version of this theory for a while.]
L21: L20(i) above involves B in discontinuous motion separated by periods of instantaneous rest, while L20(ii) pictures B, or a series of bodies, in discontinuous existence at successive locations.
L22: L20(ii) must be rejected as completely absurd.
L23: If L20(ii) is rejected then L20(i) implies that in between each successive point occupancy, B must pass through an indefinite (possibly infinite) number of intermediate points.
[Of course, L23 depends on the assumption that there is an infinite, or a potentially infinite, number of points between any two points. As noted earlier, Hegel appears to have accepted this assumption.]
L24: Hence, even on the assumption that motion is discontinuous (along the lines suggested in L20(i)), there will still be an indefinite number of intermediate points that moving body, B, has to occupy while it is passing between the points at which it is said to be at rest, but which intermediate locations B must both occupy and leave at one and the same instant.
[Plainly, B won't be at rest in any of these intermediate points. If it were it would make little or no progress. Hence, if that weren't the case, and B were at rest at each of these intermediate points -- which are possibly infinite in number -- B would either make no progress at all, or it would take a potentially infinite amount of time for it to pass between any two points!]
L25: Consequently, if motion takes place -- and it is either continuous or discontinuous -- moving body, B, must both be located and not located in a given place at one and the same time, namely at these intermediate points, at the very least.
L26: Therefore, the assumption that B is in motion only if it occupies and is at rest in successive locations at contiguous instants is false -- for even on that assumption, B must violate this condition for an indefinite number of intermediate points between each successive instance of 'rest', at successive instants.
L27: Therefore, either motion is impossible or illusory (which is absurd), or motion can't be wholly discontinuous.
It is possible to strengthen L27 by means of L27a, but that option won't be pursued any further here:
L27a: Therefore, either motion is impossible or illusory (which is absurd), or motion can't be discontinuous.19a
[QM = Quantum Mechanics.]
Having said that, it is worth noting that Loop Quantum Gravity, which is one of the main competitor theories in Modern Physics that attempts to explain gravity in terms of QM -- i.e., it pictures both space and time as discrete.
About which we read:
"Unifying quantum mechanics with general relativity is the ultimate dream -- or nightmare -- of physics. It would be a way to finally describe the force of gravity with the tools of quantum mechanics, unlocking how gravity works when it's really strong and at really small scales. Einstein's theory of general relativity tells us that the warping of space and time is what we experience as the force of gravity. Quantum mechanics tells us that what we experience as the forces of nature really come in discrete, tiny chunks, known as quanta. So, if gravity is the bending of space-time, gravity is a force and all forces are quantized, maybe space-time itself comes in discrete little blocks. Maybe there are fundamental units of space-time at some unfathomably tiny scale.
"One of the most annoying things that general relativity and quantum mechanics disagree about is the role that space-time plays in the physics. For quantum mechanics, space-time is just a background, a stage, a floor, a container for all the interesting interactions that make up the physics of the universe. Yes, that stage may bend and warp, and that bending and warping affect the paths of particles -- but that's about it. All physics happens 'on top' of that background space-time.... For general relativity, however, space-time isn't a background stage for the actors; it is the actor. General relativity doesn't assume a background; it creates it. General relativity is the language of the warping of space-time, and that very warping generates the physics of gravity. So, in our quest to unite quantum mechanics with gravity, maybe we should take Einstein's theory at face value. If gravity simply is the mechanics of space-time, then to seek a quantum theory of gravity, we really need to seek a quantum theory of space-time. If we can crack that quantization, then by default, we'll end up with a quantum theory of gravity, and the problem will be solved.
"This is the approach known as loop quantum gravity. The word 'loop' appears in the name because the theory's foundation is based on a rewriting of Einstein's general relativity in terms of lines (instead of points as it's usually done). It doesn't change any of the physics but makes some calculations easier, especially when it comes to quantizing space-time. What does it mean to quantize space-time? It means there's a fundamental unit, a discrete chunk, of space-timey-ness that sits at some imperceptibly small scale. If you were to zoom in to this screen, the smooth curves and clean edges of the letters would be revealed as a vast number of little squares -- pixels. In much the same way, if you were to zoom in to space-time, you would see that time doesn't advance into the future continuously but in quick little tick-tick-ticks of a discrete clock. When you move, it wouldn't be a smooth motion; it would just consist of stuttering steps from one space-time pixel to another
"The biggest benefit of this quantization of space-time is that singularities simply go away. Singularities appear in Einstein's general relativity as places where densities go infinitely high and gravity becomes infinitely strong. We know that this really means that our understanding of the physics is going off the wall, and that we have no clue as to what's happening deep inside a black hole or at the beginning of the Big Bang, where singularities appear. In loop quantum gravity, though, those singularities get replaced with really, really tiny chunks of ultradense (and, presumably, ultra-exotic) matter. We would simply banish those singularity demons from our universe and replace them with something understandable. What do those ultradense chunks of matter look like? Well, we're not sure. You see, loop quantum gravity isn't exactly complete. Although we've managed to develop some of the mathematics of 'pixellated' space-time using a mathematical tool called spin networks, the biggest problem is that loop quantum gravity is a theory of strong gravity at small scales, which should automatically also be a theory of weak gravity at normal scales. That means that, if you use the mathematics of loop quantum gravity in non-crazy situations, like Earth orbiting the sun, you should get all the same results as you would from general relativity or old-fashioned Newtonian physics.
"In other words, loop quantum gravity should contain within itself Einstein's general relativity, and we don't yet know if it does. You should be able to zoom out from the pixellated, quantum space-time view at the smallest scales and recover the smooth, undulating fabric of general relativity's space-time -- and nobody knows how to do that. There are other issues, too. Special relativity tells us that perceptions of time and space depend on our velocity but that our perception of fundamental physics should be the same. But different observers will have different views of the sizes of the quantized pixels of space-time, which will radically alter their views of physics. So that's a problem. Loop quantum gravity is incomplete, and it may not work out. Just like its cousin string theory, which also claims to be a quantum theory of gravity, the mathematics of loop quantum gravity aren't revealing any workable solutions. Some future scientist could crack the code, paving the way to a full understanding of the force gravity. Or, we could just be…wait for it…going in circles." [Quoted from here; accessed 03/07/2023. Several paragraphs merged; some links in the original -- however, two have been removed and three added. Spelling modified to agree with UK English; one minor typo corrected. Quotation marks altered to conform with the conventions adopted at this site. Bold emphases alone added.]
If the above theory pans out, we should finally be able to wave "Goodbye!" to any idea that motion is either continuous or 'contradictory'. The same can be said in relation to any successes enjoyed by Causal Set Theory:
"The causal set theory...approach to quantum gravity postulates that at the most fundamental level, spacetime is discrete, with the spacetime continuum replaced by locally finite posets or 'causal sets'". [Suraya (2019); accessed 03/07/2023. Quotation marks altered to conform with the conventions adopted at this site. Bold emphasis added.]
Since these two theories have yet to be either confirmed or generally accepted by scientists, I will say no more about them -- except to point out the obvious, that DM-fans will be looking over their shoulders for the next decade or so as these ideas are refined and tested, All I will add is that the conclusions drawn in this Essay in no way depend on either theory being correct. The theory that motion is 'contradictory' makes no sense to begin with, as this Essay aims to show, whatever theory of gravity Physicists finally end up with (if they do!).
Pick Your Favourite Contradiction
However, it is worth noting that the above argument begins with the rejection of one specific contradiction -- expressed in L14 (restated below, but re-numbered L28, and hence very slightly modified), alongside its supposed contradictory, L29:
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L29: A body can be at rest and in motion at the same time in the same inertial frame.
Naturally, much depends on whether these two are genuine contradictories; I will ignore that minor complication in what follows. On the other hand, if they aren't even propositions, then they can't be contradictories to begin with. Nevertheless, for the purposes of argument I will assume they are propositions and are contradictories.
[However, their status as propositions will be questioned in Essay Twelve Part One.]20
If these seemingly minor 'niggles' are put to one side, we can now conclude that L29 is true if and only if L28 is false, and vice versa.
As is well-known, an analogous series of background assumptions motivated Zeno into trying to 'prove' that motion was either impossible or illusory. DM-theorists clearly, and rightly, reject Zeno's ridiculous conclusion, but it seems they may only do so by accepting L28 (or its 'dialectical' equivalent), thus rejecting L29 (or its 'dialectical' equivalent), in order to derive their own contradiction, expressed in L17, which was:
L17: A moving body must both occupy and not occupy a point at one and the same instant.
Plainly, if L28 (below) were false, and L29 were true, that would imply that a body could be moving and at rest at the same time. [That certainly looks like a 'contradiction' -- one might be tempted to conclude!] However, if L29 turned out to be true, and a body could be at rest and in motion at the same time, L17 might not look quite so obvious or compelling to DM-fans. At any rate, it is quite clear that dialecticians have to reject one 'contradiction' (expressed in L29) in order to derive their own (in L17).
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L29: A body can be at rest and in motion at the same time in the same inertial frame.
[In response, it could be argued that the contradiction in L29 isn't dialectical. But, as we have seen (here, here and here), the 'contradiction' Engels and Hegel claimed to have found in motion isn't dialectical, either! On that, see also Note 18a.]
When L17 is conjoined with L28 we obtain the following:
L17a: Since a body can't be at rest and moving at one and the same time in the same inertial frame, a moving body must both occupy and not occupy a point at one and the same moment.
This seems to be the 'contradiction' that exercised Engels. If so, it is now worth asking: Which of the following two 'contradictions' is it legitimate to accept or legitimate to reject: L17 or L29?
L17: A moving body must both occupy and not occupy a point at one and the same instant.
L29: A body can be at rest and in motion at the same time in the same inertial frame.
Which of these 'contradictions' is the more absurd? If L29 were the case, L17 couldn't be derived in any obvious way from L14-L27. That would in turn mean that Engels's conclusion (L17) is false and must be rejected.
Nevertheless, it is clear from the way the above argument has been developed that L17/L17a depend on the truth of L28:
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L17a: Since a body can't be at rest and moving at one and the same time in the same inertial frame, a moving body must both occupy and not occupy a point at one and the same moment.
[L14: An object can't be in motion and at rest at one and the same time (in the same inertial frame).]
That is because L14-L17 began with the assumed truth of L14 (or, with its current equivalent, L28). The reverse inference doesn't appear to work.
L14: An object can't be in motion and at rest at one and the same time (in the same inertial frame).
[L28: A body can't be at rest and in motion at the same time in the same inertial frame.]
L15: If an object is located at a point it must be at rest at that point.
L16: Hence, a moving body can't just be located at a point otherwise it wouldn't be moving, it would be at rest.
L17: Consequently, given L14, a moving body must both occupy and not occupy a point at one and the same instant.
In this Essay, I am not too concerned whether or not the reverse implication (i.e., from L17 to L14) works. All I am trying to do here is make sense of the idea that motion is contradictory and beginning with the assumed truth of that L14 appears to be the only way to do this. Again, since DM-theorists refuse to be clear about what they actually mean, it has been left to me to try to:
(i) Make sense of what little they have committed to paper;
(ii) Understand how they arrived at the conclusions that finally emerged (even if only implicitly); and,
(iii) Provide some sort of rationale for it.
So, it looks like L28 (below) doesn't pre-suppose the truth of the conclusion drawn in L17a, whereas the conclusion drawn in L17a appears to depends on L28. This in turn suggests that L28 might be the more fundamental 'proposition' of the two. Indeed, because L17a physically contains L28 as its first clause (prefacing it with the word "since"), there is far more here than a simple "appears"; it is quite plain that L17a depends on the truth of L28, not the other way round.
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L17a: Since a body can't be at rest and moving at one and the same time in the same inertial frame, a moving body must both occupy and not occupy a point at one and the same moment.
It could be objected that this is an artificial result because of the way the present author has worded L17a. In that case, DM-fans are welcome to re-cast or re-formulate the above argument (along with all its complexities) in any way they feel doesn't imply the same overall conclusion -- i.e., that L17a depends on L28, not the other way round.
Recall, L17 was the end-point of all this; L17a was merely quoted to make the entire argument a little more concise. In addition, it also seems L17 depends on L28 not the other way round.
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L17: A moving body must both occupy and not occupy a point at one and the same instant.
Be this as it may, as we have seen, L28 would itself be false if L29 were true:
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L29: A body can be at rest and in motion at the same time in the same inertial frame.
Unfortunately, L29 is a familiar truth! An object can be at rest with respect to one inertial frame and yet be in motion with respect to another. The wording of L29 doesn't preclude it. In order to eliminate this latest difficulty, L29 should be modified -- perhaps along the following lines:
L30: With respect to the same inertial frame and the same instant in time, a body can be at rest and in motion.
It is worth noting that L30 is contradicted by L30a:
L30a: With respect to the same inertial frame and the same instant in time, a body can't be at rest and in motion.
However, L30 certainly looks 'contradictory' -- especially if the phrase "at rest" is taken to mean "not in motion with respect to the same inertial frame".
Nevertheless, it was the rejection of L30 (or its 'dialectical' equivalent) that led to the derivation of L17/L17a. But, if L30 is always false (i.e., if L30a is always true), it looks like L28 must always be true, too (given certain other assumptions, and if worded appropriately).
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L17a: Since a body can't be at rest and moving at one and the same time in the same inertial frame, a moving body must both occupy and not occupy a point at one and the same moment.
L17: A moving body must both occupy and not occupy a point at one and the same instant.
Consequently, if we deny that a body can be at rest and moving at the same time (in the manner indicated above), Engels's conclusion does (finally!) appear to follow.
That much seems reasonably clear.
Unfortunately, however, the following line of argument also shows that the derivation of L17a from the rejection of L30 isn't inevitable, and that means Engels's conclusion doesn't automatically follow, after all:
L31: A body can't be at rest and in motion with respect to the same inertial frame at the same time.
L32: If a body is wholly located at a point it can't be wholly located at any other point in the same reference frame at the same time.
L33: But, a moving body must be located wholly at two points at the same time, otherwise it would be at rest.
L34: Since L33 is impossible (by L32), motion can't take place. Hence, by L31, and despite appearances to the contrary, all bodies are at rest!
Of course, L34 is reminiscent of the conclusion Zeno himself drew, and flatly contradicts experience. It is therefore unacceptable -- but only if we allow experience to be decisive in such matters (indeed, as John Norton argued earlier). However, it is now clear that L31-L34 demonstrate that L17a doesn't have to follow from the rejection of L30, even if the alternative result proves unpalatable for other reasons.
L30: With respect to the same inertial frame and the same instant in time, a body can be at rest and in motion.
In which case, a refusal to accept the 'contradiction' expressed in/by L30 can lead off in separate directions, ending up with two distinct 'contradictory' conclusions. One of them is inconsistent with experience (the latter half of L34 -- i.e., L34b), while the other (i.e., L17a) is self-contradictory:
L17a: Since a body can't be at rest and moving at one and the same time in the same inertial frame, a moving body must both occupy and not occupy a point at one and the same moment.
L34b: Despite appearances to the contrary, all bodies are at rest.
Naturally, which one of the above two outcomes proves to be the least unacceptable will depend on other priorities. If it is felt that experience is unreliable, L34b might be preferable. On the other hand, if contradictions are regarded as fundamental features of reality, L17a might well be chosen. However, it is worth noting that neither option is empirically verifiable. In fact they both transcend any conceivable body of evidence and every possible observation.21
Interlude Two -- 'Appearances To The Contrary'
In response, it might be thought that L34b is both testable and false. But, L34b said the following:
L34b: Despite appearances to the contrary, all bodies are at rest.
As should seem obvious, it isn't possible to test something "despite appearances to the contrary".
That is because, plainly, any test has to depend on the appearances conveyed to us by eyesight or various instruments (e.g., cameras, computers and other devices). [There is more on this in Essay Three Part Two.]
It could be objected that the present author referred earlier to an example where something was tested "despite appearances to the contrary" -- namely the work of Eadweard Muybridge concerning horses and how they actually ran despite appearances to the contrary. To the naked eye, these mammals appear to run one way even though photographic evidence shows they don't. But, those photographs are also 'appearances' (if we adopt for the moment this way of talking). So, if we really do insist on embracing this odd way of speaking (i.e., about 'appearances'), what Muybridge in fact tested was one set of appearances (those clocked by the 'naked eye') against another (those recorded by a series of photographs -- also examined by the eye). Unless one day we manage to 'intuit nature directly', by-passing all those inconvenient 'appearances', there is no way out of this phenomenological 'prison'. As Essay Three Part Two shows (link above), I don't prefer this way of speaking, but when one finds oneself in the 'Land of the Idealists' one has to adopt their patois or risk failing to 'communicate'.
The bottom line is that I employ their way of speaking in order to help undermine it.
That isn't to suggest there are no theoretical problems either option faces (the options being: whether motion is continuous or discontinuous), but there is no way of experimentally testing, or even discriminating between, them. If a moving body occupied points in space (and was thus stationary) during intervals of the order of, say, 10-10,000,000,000,000,000,000,000,000,000 seconds (if that were even possible!), there is no way we would ever be able to detect it. At least, not without reference to yet more of those annoying 'appearances'.
On the other hand, if a moving body occupied at most two such places in the same 'moment' (in accord with Engels's analysis), we still wouldn't be able to tell. That shouldn't surprise us As they stand, both options are metaphysical and aren't, therefore, based on processes that take place in this universe, having been conjured into 'existence' by distorting the only language that secures our knowledge of it -- i.e., the vernacular (in conjunction with the everyday practices in which it is embedded) -- a defective strategy that was further compounded by imposing the results on reality, à la Engels/Hegel/Zeno.
Of course, there are certain protocols of modern Physics that postulate minimum times and distances:
Planck Mass: 2.17645(16) x 10-8 kg;
Planck Temperature: 1.41679(11) x 1032 K;
Planck Length: 1.61624(12) x 10-35 m;
Planck Time: 5.39121(40) x 10-44 s.
But, the above figures are the result of convention and required by theory. All but the first of them can't be measured, nor are they even measurable. Hence, there is no way any of them can be shown to be universally or eternally valid maxima or minima. To be sure, certain well-confirmed theories might be based on them, which might suggest they are valid extremes, but as we will see in Essay Ten Part One, that isn't a safe inference itself.
~~~~~~oOo~~~~~~
Of course, it could be argued that L32 begs the question, since, with respect to moving bodies, DM-theorists claim they can be wholly located at two points at once, and still be moving. In fact, L32 and L33 are both guilty of begging the question. [The verb phrase "to beg the question" has now assumed a new meaning -- it now means "to prompt the question". It is here being used in its older sense; follow the link for a definition.]
L32: If a body is wholly located at a point it can't be wholly located at any other point in the same reference frame at the same time.
L33: But, a moving body must be located wholly at two points at the same time, otherwise it would be at rest.
Or, so it could be maintained...
Nevertheless, given the fact that dialecticians also believe that appearances 'contradict' underlying 'essence', they are the very last ones who can legitimately appeal to experience in order to refute Zeno-esque conclusions, like L34b (below). In fact, if the DM-theory that underlying 'essence contradicts appearance' were itself correct, then, since it appears to be the case that there are moving bodies, in 'essence' the opposite must be the case! The inescapable conclusion now seems to be that, if 'appearances' do in fact 'contradict reality', the following must be the case: essentially no bodies actually move; which would, of course, mean Zeno was right all along!
L34b: Despite appearances to the contrary, all bodies are at rest.
[I hasten to add that I don't believe Zeno was right all along! Once again, I am merely drawing out the ridiculous implications of this strand of DM.]
Putting the above annoying corollary to one side for now, it is important to note that both of the two earlier 'derivations' (i.e., L32 and L33) rely on the ambiguities we encountered earlier with respect to L1-L13, alongside several more yet to be highlighted. As we will see, traditional dogmatic and aprioristic arguments and assumptions like these only seem to work because they are shot-through with equivocation, ambiguity and linguistic distortion. Indeed, that is partly why both of the above conclusions finally collapse into absurdity and incoherence -- as we are about to find out.
[Apologies are owed in advance to the reader: the next few sub-sections are somewhat involved and are quite technical, but there is no other way of exposing just how muddled and confused and the 'DM-theory of motion' actually is. However, where possible I have also translated parts of this material into more ordinary terms.]
The absurdity in L34b (below) is quite plain for all to see and needn't detain us any longer. However, the ludicrous nature of L17a isn't perhaps quite as obvious (although several clear pointers in that direction were outlined earlier).
L34b: Despite appearances to the contrary, all bodies are at rest.
L17a: Since a body can't be at rest and moving at one and the same time in the same inertial frame, a moving body must both occupy and not occupy a point at one and the same moment.
The ludicrous nature of L17a may nevertheless be made more plain by means of the following argument. [In fact, much of what follows depends on and develops an earlier argument, which showed that if Engels's theory were correct, 'dialectical motion' would be a stop-go, staccato sort of affair. The material in this sub-section derives several other absurd conclusions based on similar reasoning, but channelled in a new direction.]
In order to proceed it will first of all be taken for granted that L15b, L15c and L15d (below) are all (uncontroversially) true and that the phrase "moment in time" is well understood -- or at least is as well understood as it was when Engels used similar language. As we have seen, assumptions like these are (implicitly) accepted by all DM-theorists who agree with Engels.
Second, unlike earlier on in this Essay, it will now be assumed that the word "located" doesn't automatically imply a given object is stationary. For that to be the case it would have to be clear that the said object was located at a given point for more than one such moment (and for that to be the case howsoever "point" and "moment" were defined):
L15b: If an object is wholly located at a point for at least two contiguous moments in time, it must be at rest at that point.
L15c: So, no moving body can be in one and only one such location during two moments.
L15d: Hence, if an object is located at a point during a temporal interval of arbitrary length it must be at rest at that point.
L35: Motion implies that a body is in one place and not in it at the same time; in one place and in another at the same moment.
L36: Let body, B, be in motion and be located at (X1, Y1, Z1), at t1.
L37: L35 implies that B is also at some other point -- say, (X2, Y2, Z2) --, at t1.
L38: But, L35 also implies that B is at (X2, Y2, Z2) and at another point at t1; hence it is also at (X3, Y3, Z3), at t1.
L39: Again, L35 implies that B is at (X3, Y3, Z3) and at another point at t1; hence also at (X4, Y4, Z4), at t1.
L40: Once more, L35 implies that B is at (X4, Y4, Z4) and at another point at t1; hence also at (X5, Y5, Z5), at t1.
[This is just a re-statement of a result that was obtained and explained in more detail earlier, but it is being used here to reach a totally different conclusion.]
By n successive applications of L35 it is possible to show that, as a result of the 'contradictory' nature of motion, B must be everywhere along its trajectory if it is anywhere, all at t1!22
Expressing the same argument in more detail, but perhaps employing clearer language (and less intimidating symbols), we would have the following:
E1: Assume that body, B, is at rest. If so, it will be in a given location -- say, pk -- for at least two successive moments (leaving the word "moment" as vague as Engels left it). Call these "moments", tk and t(k+1). [Where tk is "any moment in time" and t(k+1) is "any successive moment in time".]
E2: Assume further that B is now set in motion at t1 and, hence, that it is in two places at once -- say, p1 and p2, both at t1.
E3: If so, B can't be in p2 at a later moment, t2, otherwise it will be at rest there (by L15b and that earlier result).
[L15b: If an object is wholly located at a point for at least two contiguous moments in time, it must be at rest at that point.]
E4: That is because B would then be located at p2 during two moments, t1 and t2, which would mean it had stopped moving. In that case, B must be in a third place, p3, at the same moment, t1.
E5: If B isn't located at p3 at the same moment, t1, it must be there at a later time -- say, t2.
E6: But, according to E2, B must be in p2 and p3 at the same time, since moving bodies must be in two places at the same moment, and we have already established that B is in p2, at t1. [From E2.]
E7: So, B must be in p2 and p3, at t1 -- otherwise we will have to abandon the claim that B is moving. [Here is where this argument diverges from the earlier one.]
E8: Hence, if B is in p2 and p3 at t1, and is still moving, it must be in three places at the same time, p1, p2 and p3.
E9: But, the same considerations apply to p3 and p4 as they did with p2 and p3; B has to be in those two places at the same time, too, which means that B is now in four places, p1, p2, p3 and p4, at t1.
E10: It takes very little 'dialectical logic' to see where this is going (no pun intended): if there are n points along its path, B will be in p1, p2, p3..., pk-1, pk, pk+1..., p(n-2), p(n-1), and pn, all at t1!
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory at the same time!
In order to aid those readers who aren't too familiar with semi-technical arguments (like the above), here it is again in summary form, with very few technicalities, and employing more familiar ordinary language:
According to Engels, a moving object has to be in two places at the same time; call that moment, t1. But, if it is still moving at the second of those two locations, it must be there and in a third place at the same moment in time, t1. If that weren't the case, and it was there at a later moment, t2, that would imply it was in that second location during two moments, t1 and t2, not one moment (thus contradicting Engels). That in turn would mean it was at rest there, contrary to the supposition that it is moving. So, if this object is still moving, the same considerations apply: this body must be in a third place also at t1. Similarly, if it is still moving, the same must be the case with the third and fourth places occupied, as well as the fourth and fifth, the fifth and the sixth, and so on.... They must all be occupied at the same time.
Hence, if Engels is to be believed, a moving object must be located at every point along is path at the same moment, t1!
But, that conclusion is even more absurd than L34b!
L34b: Despite appearances to the contrary, all bodies are at rest.
It might seem (to some) that there is an easy way to avoid the above outlandish conclusion by arguing that L35 implies that a moving body is in no more than two places (i.e., it is in more than one but less than three locations), at once. In other words -- as argued earlier -- such a body must be in at most two places at the same time. But, that is no help, for, as noted above, if a body is still moving and in the second of those two places, it won't still be in motion there unless it were in a third place at the very same time (by L15b and L35).
L15b: If an object is wholly located at a point for at least two contiguous moments in time, it must be at rest at that point.
L35: Motion implies that a body is in one place and not in it at the same time; in one place and in another at the same moment. [Emphasis added.]
Once again, just as soon as a body is located in a given spot for any length of time (i.e., two or more successive moments) it will be at rest there. Without assuming the truth of L15b (and hence without L35), Engels's conclusions simply wouldn't follow. Given his view, if a body is moving, it has to occupy at least two points at once, or it will be at rest. But, that is precisely what creates the absurdity, for if that body is located at the second of these two points, it will be at rest there unless it is also located at a third point, at the same time.
That follows from L17 (encapsulated in L17b):
L17: A moving body must both occupy and not occupy a point at one and the same instant.
L17b: A moving object must occupy at least two places at once.
~~~~~~oOo~~~~~~
Interlude Three -- Terminology
The following remarks are intended for those who aren't too familiar with the use of phrases like "at most two" and "at least two", which appear in mathematics and modern logic all the time (no pun intended).
[A] "At most two" means the same as "less than three" -- i.e., "two or less" --, which implies, in this case, the following locations: (i) p1 on its own, (ii) p1 and p2, or (iii) p2 and p3 (etc.), but (iv) not p1, p2 and p3 (etc.).
[B] "At least two" means the same as "more than one" -- i.e., "two or more" --, which implies, in this case, the following locations: (i) p1 and p2, (ii) p1, p2 and p3, or (iii) p1, p2, p3 and p4 (etc.), but not, say, (iv) p1 only, (v) p2 only, or (vi) p3 only (etc.).
[C] Finally, a combination of "at most two" and "at least two" means "exactly two", which implies, in this case, the following locations: (i) p1 and p2, (ii) p2 and p3, or (iii) p3 and p4 (etc.), but not (iv) p1 on its own; nor (v) p1, p2 and p3, either; nor (vi) p1, p2, p3 and p4, either (etc.).
~~~~~~oOo~~~~~~
Of course, it could be argued that L17b is in fact true of the scenario depicted in L35-L40 -- the said body does occupy at least two places at once, namely (X1, Y1, Z1) and (X2, Y2, Z2). Hence, the conclusions drawn above are fallacious.
L17b: A moving object must occupy at least two places at once.
Or, so it might be maintained...
The above anti-DM argument would indeed be misconceived if Engels had managed to show that a body can be in at most two (but not in at least two) places at once, which he not only failed to do, he couldn't do.
L17c: A moving object must occupy at most two places at once.
That is because, between any two points there is a third point (as we have seen, even Hegel conceded this!), and if the said body is in (X1, Y1, Z1) and (X2, Y2, Z2), at t1, it must also be in any point between (X1, Y1, Z1) and (X2, Y2, Z2), at t1 --, say, (Xj, Yj, Zj). But, just as soon as that is admitted, it becomes clear that a moving body must be in more than two places at once -- namely, at least (X1, Y1, Z1), (Xj, Yj, Zj), and (X2, Y2, Z2), at t1. Hence, there seems to be no way of avoiding the conclusion drawn above: if a moving body is anywhere, it is everywhere in its trajectory, at the same time.
[And that is just one of the reasons why the question was posed earlier about the precise distance between the points at or in which Engels concluded that moving bodies perform such 'contradictory' antics. Of course, whenever I use the phrase "a moving body is in a (certain) point", it should be taken to mean "a moving body is located at a (certain) point", since it has already been established that points aren't containers. (The reason that I have often used the first of these locutions (i.e., employing "in") in this Essay is that the word "located" tends to suggest (to some) a lack of movement.)]
Putting these annoying technicalities and quibbles to one side for now, some might think it unwise to assume Engels believed that a moving body occupies at most two points at the same time (or even that DM requires it), since, as we have just seen, if that body occupies the second of these two points, it would be at rest at that point, unless it also occupied a third point at the same time. Given the truth of L15b, there seems to be no way to avoid that fatal implication.
L15b: If an object is wholly located at a point for at least two contiguous moments in time, it must be at rest at that point.
However, we have also seen that if Engels did mean that a moving body occupies "at most two points" at once, then 'dialectical motion' would be discontinuous. If supporters and defenders of Engels want to maintain that 'dialectical motion' is continuous, they will need to reject the "at most two points" codicil. Unfortunately, as we have just seen, upon doing that the ridiculous conclusion reached above automatically follow -- i.e., E11:
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory, at the same time!
So, DM-fans are caught between a rock and a hard place. Either they admit 'dialectical motion' is discontinuous, or they acknowledge the truth of E11.
On the other hand, a combination of "at least two places" and "at most two places" at once would be the equivalent of "exactly two places", at once:
L17d: A moving object must occupy exactly two places at once.
But, any attempt to restrict a moving body to the occupancy of exactly two places at once would only work if that body came to rest at the second of those two locations! L15b is quite clear: if a body is located at a point (even if it is the second of those two points) for any length of time, it must be at rest at that point. Hence, this seemingly promising escape route (i.e., restricting a moving body to the occupancy of exactly two points at the same time) will only work if DM-theorists now reject their own characterisation of motion, partially captured by L15b:
L15b: If an object is wholly located at a point for at least two contiguous moments in time, it must be at rest at that point.
[That option also falls foul of the intermediate points objection, outlined earlier.]
In that case, if L15b is still held true, then, at the second of these two proposed DM-points -- in this case, (X2, Y2, Z2) --, if the said body is still moving, it will also be in and not in that second point at the same instant, too.
It is worth underling this conclusion: if L17d were accepted, and the said body were located at a second point -- say, (X2, Y2, Z2) --, at t1, it will be at rest there, contrary to the assumption that it is moving. So, for that body still to be moving at t1 it must be elsewhere also at t1, and so it must be at a third point -- in this case, (X3, Y3, Z3) -- at t1, contrary to L17d. Otherwise, the condition that a moving body must be both in a certain place and not in it at the very same moment will have to be abandoned. In that case DM-theorists can't afford to accept L17d --, unless, of course, they are also prepared to admit that motion isn't dialectical, isn't contradictory.
L17d: A moving object must occupy exactly two places at once.
Consequently, we end up with the unacceptable outcome, that: as a result of the 'contradictory' nature of motion, a moving body must be everywhere along its trajectory all at once.
In response, it could be argued that when body, B, is in the second place at the same instant, a new moment in time would begin. So, while B is in (X2, Y2, Z2) at t1, a new instant, say t2, would start. B would then be in (X2, Y2, Z2) and (X3, Y3, Z3), at the same moment, t2, not t1, thus rescuing Engels's theory.
Admittedly, that hasty, ad hoc, 'repair' seems to avoid some of the disastrous implications obtained above (in this case, expressed in E11):
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory, at the same time!
However, it only succeeds in doing that by introducing several serious problems of its own, since it implies that B would be located at (X2, Y2, Z2) at t1 and t2, which would in turn mean that B was in the same place for two 'moments in time', and that would mean it was stationary at that point! That is why proposition L15b was introduced earlier:
L15b: If an object is wholly located at a point for at least two contiguous moments in time, it must be at rest at that point.
It could now be argued, again (!!), that B-like objects do in fact occupy two places at once, namely (X1, Y1, Z1) and (X2, Y2, Z2), so the above anti-DM argument is clearly defective. Hence, the 'derivation' that purports to show that a moving body must be everywhere along its trajectory, if it is anywhere, at the same time -- i.e., E11, again --, is also fallacious.
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory, at the same time!
We can perhaps clarify this (proffered) DM-reply by means of the following:
L38: L35 also implies that B is at (X2, Y2, Z2) and at another place at t1, hence it is also at (X3, Y3, Z3) at t1.
[L35: Motion implies that a body is in one place and not in it at the same time; in one place and in another at the same moment.]
The idea here is that if we select, pair-wise, any two points that a body occupies, in any order (i.e., either (X1, Y1, Z1) and (X2, Y2, Z2), or (X1, Y1, Z1) and (X3, Y3, Z3)..., or (X1, Y1, Z1) and (Xn, Yn, Zn), and so on), then L17c will still be satisfied, and the above 'derivation' -- that a moving body must be everywhere along its trajectory if it is anywhere, at the same time -- fails. [This 'repair' was in fact considered earlier and rejected.]
L17c: A moving object must occupy at most two places at once.
Or, so it could be argued...
Unfortunately, this seemingly promising alternative soon self-destructs. Here is why:
The (proffered) DM-reply above is based on the counter-claim that Engels only needs a body to be in two places at once. He didn't specify where along that body's trajectory those two places were supposed to be. In that case, any two places will do. Hence, a third place introduced in the above attempted refutation of Engels -- i.e., (X3, Y3, Z3) -- isn't actually implied by his description of the 'contradiction' involved.
L38: L35 also implies that B is at (X2, Y2, Z2) and at another place at t1, hence it is also at (X3, Y3, Z3) at t1.
[L35: Motion implies that a body is in one place and not in it at the same time; in one place and in another at the same moment..]
The (proffered) DM-counter-argument then goes something like this:
L38 only follows if we ignore certain facts, perhaps the most important of which is that the other place where moving object, B, is located isn't (X3, Y3, Z3), it is (X1, Y1, Z1). B, can't be located at (X3, Y3, Z3) at the same time since it is at these two points, (X1, Y1, Z1) and (X2, Y2, Z2), at the same time.
So, the requirement that B should be located at any two points is satisfied when the following take place:
B is located at:
(i) (X1, Y1, Z1) and (X2, Y2, Z2); or it is located at,
(ii) (X1, Y1, Z1) and (X3, Y3, Z3); or it is located at,
(iii) (X3, Y3, Z3) and (X5, Y5, Z5); or it is located at,
(iv) (X7, Y7, Z7) and (X23, Y23, Z23); or it is located at...,
(n) (Xn, Yn, Zn) and (Xn+k, Yn+k, Zn+k), etc., etc.,
along its path, at the same moment. In that case, the said object will be located at any two points at the same time, not every point along its trajectory at once (contrary to L38 and E11). And these points do not have to be contiguous, there just have to be two of them.
L38: L35 also implies that B is at (X2, Y2, Z2) and at another place at t1, hence it is also at (X3, Y3, Z3) at t1.
[L35: Motion implies that a body is in one place and not in it at the same time; in one place and in another at the same moment.]
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory, at the same time!
Or so it might be maintained...
But, the original (anti-DM argument) was quite specific, and ended up deriving the following result (here re-phrased):
R1: If we select any two points that a moving body occupies in any order [i.e., (i) (X1, Y1, Z1) and (X2, Y2, Z2), and (ii) (X1, Y1, Z1) and (X3, Y3, Z3)..., and (k) (X1, Y1, Z1) and (Xk, Yk, Zk), and..., (n)..., and so on...], then the condition expressed in L17c will be violated, which means E11 still follows.
L17c: A moving object must occupy at most two places at once.
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory, at the same time!
It could be objected that the above (anti-DM) argument only works because an "and" has been surreptitiously substituted for an "or". The original (proffered) DM-reply in fact argued as follows (slightly modified and tidied-up):
R2: If we select any two points a moving body occupies in any order [either (i) (X1, Y1, Z1) and (X2, Y2, Z2), or (ii) (X1, Y1, Z1) and (X3, Y3, Z3)..., or (k) (X1, Y1, Z1) and (Xk, Yk, Zk), or.... (n)..., and so on...], then L17c will be satisfied, thus ruling out E11.
L17c: A moving object must occupy at most two places at once.
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory, at the same time!
It was not as follows:
R1: If we select any two points a moving body occupies in any order [i.e., (i) (X1, Y1, Z1) and (X2, Y2, Z2), and (ii) (X1, Y1, Z1) and (X3, Y3, Z3)..., and (k) (X1, Y1, Z1) and (Xk, Yk, Zk), and..., (n)..., and so on...], then the condition expressed in L17c will be violated, which means E11 still follows. [Emphasis added.]
Or so it could be objected...
Unfortunately, once more, this (proffered) DM-response simply catapults us back to an earlier untenable position, criticised along the following lines:
[B]etween any two points there is a third point (as we have seen, even Hegel conceded this!), and if the said body is in (X1, Y1, Z1) and (X2, Y2, Z2), at t1, it must also be in any point between (X1, Y1, Z1) and (X2, Y2, Z2), at t1 --, say, (Xj, Yj, Zj). But, just as soon as that is admitted, it becomes clear that a moving body must be in more than two places at once -- namely, at least (X1, Y1, Z1), (Xj, Yj, Zj), and (X2, Y2, Z2), at t1. Hence, there seems to be no way of avoiding the conclusion drawn above: if a moving body is anywhere, it is everywhere in its trajectory, at the same time.
In that case, the (proffered) DM-reply, encapsulated in R2 itself fails. So, if a body is in (X1, Y1, Z1) and (X2, Y2, Z2) at t1, it must also be in at least one of the intermediate points -- e.g., (Xj, Yj, Zj) --, also at t1. Hence, R1 is still a valid (anti-DM) objection.
R2: If we select any two points a moving body occupies in any order [either (i) (X1, Y1, Z1) and (X2, Y2, Z2), or (ii) (X1, Y1, Z1) and (X3, Y3, Z3)..., or (k) (X1, Y1, Z1) and (Xk, Yk, Zk), or.... (n)..., and so on...], then L17c will be satisfied, ruling out E11.
In order to see this, a few of the subscripts in R1 only need to be altered -- perhaps as follows:
R3: If we select any two points a moving body occupies in any order [i.e., (i) (X1, Y1, Z1) and (X2, Y2, Z2), and (ii) (X1, Y1, Z1) and (Xj, Yj, Zj), and (iii) (X1, Y1, Z1) and (Xq, Yq, Zq)..., and..., (n)..., and so on...], then the condition expressed in L17c will be violated, which means E11 still follows.
Compare R3 with R1:
R1: If we select any two points a moving body occupies in any order [i.e., (i) (X1, Y1, Z1) and (X2, Y2, Z2), and (ii) (X1, Y1, Z1) and (X3, Y3, Z3)..., and (k) (X1, Y1, Z1) and (Xk, Yk, Zk), and..., (n)..., and so on...], then the condition expressed in L17c will be violated, which means E11 still follows.
It is surely philosophically and mathematically irrelevant whether we label such points with iterative letters (i.e., "j" or "q") or with numerals ("1", "2" or "3"). [Recall, the variables labelled with iterative letters (i.e., "j" or "q") were meant to be intermediate points in R3.]
In which case, R3 implies that if a body is in, say, (X1, Y1, Z1) and (X2, Y2, Z2), at t1, it must also be in at least one of the intermediate points -- say, (Xj, Yj, Zj) --, at the same moment. R3 therefore implies L17c is false, and hence E11 still stands.
L17c: A moving object must occupy at most two places at once.
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory, at the same time!
Since there is a potentially infinite number of points between any two points, there is no way that L17c could be true, even if we were to accept the veracity of DM.
Moreover, it is also worth posing the following question in relation to L38: Is B at (X2, Y2, Z2), at t1?
If it is, then it must be elsewhere at the same time, or it will be stationary there. So much is agreed upon (even by DM-supporters). In that case, the only way to stop the absurd induction (i.e., the one that results in the conclusion that if a moving body is anywhere it must be everywhere at the same time -- i.e., E11) would be to argue as follows:
L38a: L35 also implies that B is at (X2, Y2, Z2) and at another place at t1, hence it is also at (X1, Y1, Z1), at t1, but not at (X3, Y3, Z3), at t1.
[L38: L35 also implies that B is at (X2, Y2, Z2) and at another place at t1, hence it is also at (X3, Y3, Z3), at t1.]
[L35: Motion implies that a body is in one place and not in it at the same time; in one place and in another at the same moment.]
E11: So, the 'world-view of the proletariat' would have a moving object occupying every point along its trajectory, at the same time!
However, the L38a 'straw', once clutched, has unfortunate consequences that beleaguered DM-fans might want to mull over before they grab at it too frantically:
L38b: If B is at (X2, Y2, Z2) and (X1, Y1, Z1), at t1, but not at (X3, Y3, Z3), at t1, then it must be at (X3, Y3, Z3), at t2.
L38c: If so, B will be at two places -- (X2, Y2, Z2) and (X3, Y3, Z3) -- at different times (i.e., at (X2, Y2, Z2), at t1, and (X3, Y3, Z3), at t2).
L38d: In that case, between these two locations (i.e., (X2, Y2, Z2) and (X3, Y3, Z3)), the motion of B will cease to be contradictory, cease to be 'dialectical' -- since it will not be in two places at the same time, but in two places at different times.
Finally, and perhaps more importantly, the above (proffered) DM-counter-argument also falls foul of an earlier result -- that is, that if a moving object is in at most two places at once, it must be stationary at the second of those two locations.
That will itself become clear if we re-examine the above pro-DM objection more closely -- i.e., that B-like objects occupy two places at once, namely (X1, Y1, Z1) and (X2, Y2, Z2), at t1.
The question now becomes: At what point in time does B move from (X2, Y2, Z2) to (X3, Y3, Z3)? If it does so at t1, that would imply B occupies more than two points at once -- namely, (X1, Y1, Z1), (X2, Y2, Z2) and (X3, Y3, Z3), at t1 -- which would in turn mean the above pro-DM objection itself fails. On the other hand, if B moves from (X2, Y2, Z2) to (X3, Y3, Z3) at a later moment, i.e., at t2, then B will have been located at (X2, Y2, Z2) for two moments in time, namely, t1 and t2, and that would mean B was stationary while it was at (X2, Y2, Z2).
Hence, it seems the only way that dialecticians can escape from this particular absurd consequence of their theory -- that a moving object is everywhere along its trajectory at the same time -- is by abandoning their belief in the contradictory nature of motion. That is, they will need to accept that these 'contradictions' cease to operate at an indefinite number of intermediate points in B's transit. For example, the alleged contradiction would disappear right after B left the first two places it occupied in its journey, since it would be stationary at each subsequent point. [Again, that result was established earlier.] That would, of course, concede the result that the said object was stationary at every point along its path!
In which case, the choice before DM-fans is now quite stark: a 'moving' dialectical object is either stationary at every point along its path, or it is everywhere in its trajectory, at the same time!
So, it now looks like the only way to avoid the above fatal results -- and hence maintain their belief in the 'contradictory' nature of motion -- would require DM-fans to impose a series of ad hoc stipulations on the world (of the sort mentioned above), none of which seem to work, anyway!
But, as we have already seen several times, such a response would be inimical in another sense: it would undermine the theory that reality itself is contradictory (rather than provide support for the conclusion that these results only apply to what we say or think about reality), all the while confirming the suspicion that it is only certain ways of representing nature that appear to imply it is contradictory, which "ways of representing nature", incidentally, still await clarification.
Once again: As we will see throughout this site, the source of these (and similar) 'problems' lies in the repeated attempt made by dialecticians (and metaphysicians alike) to state, or deduce, 'necessary truths' about reality solely from thought/language. Such theories are all based on an extrapolation from the supposed meaning of a few specially-selected words to fundamental truths about nature, valid for all of space and time --, and nothing more. Clearly, with respect to Engels's 'analysis' of motion, his predicament was further compounded by his attempt to circumvent several core conventions expressed in, or by, our use of ordinary language --, such as those expressed in, or by, the LOC and the LOI.
[I will endeavour to substantiate these claims below, but in much more detail in Essays Four Part One and Twelve Part One.]
[LOC = Law of Non-contradiction; LOI = Law of Identity; FL = Formal Logic; DL = Dialectical Logic.]
It could be objected that the above criticisms beg the question since dialecticians don't reject the application of principles drawn from FL -- such as the LOC and the LOI --, they merely highlight their limitations with respect to motion and change. However, that response was neutralised in Essays Four Part One and Eight Parts One, Two and Three. [Readers are directed there for more details.]
Suffice it to say that dialecticians themselves have yet to account for motion in anything remotely like a clear and comprehensible manner -- or even depict it accurately, for goodness sake! So, whether or not it is correct to say that FL can't account for motion and change, it is clear that DL itself fails miserably in that respect.
Even more annoying: as we saw in Essay Four Part One, and contrary to what dialecticians never tire of telling us, FL actually copes with motion and change with relative ease.
Yet Another Absurd Dialectical Consequence
Here is another, perhaps less well appreciated consequence of the 'dialectical' theory of motion and change -- which is even more absurd than those outlined earlier: If Engels's theory of motion were correct, we would have no right to say that a moving body was in the first of these 'Engelsian locations' before it was in the second.
L3: Motion involves a body being in one place and in another place at the same time, being in one and the same place and not in it.
According to Engels, that is because such a body is in both places at the same time -- so it can't be in one of them before it was in the second.
"Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
If the conclusions drawn in the previous section are valid (i.e., that moving 'dialectical objects' are everywhere along their path at the same time), then it follows that no moving body can be said to be anywhere before it is anywhere else in its entire journey! That is because such bodies are everywhere all at once. Hence, they can't be anywhere first and then somewhere else, second. In the 'dialectical universe', therefore, when it comes to motion and change, there is no before and no after -- hence there is no during or while, either!22a
So, according to the implications of this 'scientific theory', concerning the entire trajectory of a body's motion, it would be impossible to say it was at the beginning of its journey before it was at the end! In fact, it would be at the end of its journey at the same time as it sets off! So, even though you might foolishly think, for example, that in order to go on your holidays, you have to board an aeroplane before you disembark at your destination, this 'path-breaking theory', DM, tells us you are sadly mistaken: you not only must get on the plane at the very same moment as you get off it at the 'end', in fact, you do!
And the same caveat applies to everything that has happened 'since' the 'Big Bang'. While foolish and benighted non-dialecticians might think that this event took place billions of years ago, they, too, are sadly mistaken, if this 'super-scientific theory' is correct. The above argument clearly shows that any two events involving motion and change in the entire history of the universe must have taken place at the same instant. Naturally, this means that as you, dear reader, are reading this while the 'Big Bang' is now taking place!
I rather think 'Duck and cover!' should be le mot du jour...22b
Admittedly, this is completely absurd, but that's Diabolical Logic for you!
[When confronted with the truly absurd consequences of their theory, like the above (alongside others covered in this Essay, as well as those in Essay Seven Part Three), DM-fans with whom I have debated these issues seem to think that if they just flatly deny them (without giving any clear or coherent reason why they should be rejected other than they are patently absurd) they will simply disappear, which, of course, they won't. Others have tried to argue that since these are patently absurd consequences, we can't expect Hegel, Engels or Lenin to have accepted the --, or, indeed, for their theory to imply them. Naturally, that is just a variation on the fallacious Argument from Authority. In fact, they regard it as a slur on the good name of Engels and Lenin (which it isn't, it is merely to question their philosophical judgment and expertise). When asked how these absurd consequences may be avoided, circumvented or neutralised --, or, indeed, where the above (anti-DM) arguments have gone wrong --, either deafening silence ensues or DM-fans attempt to deflect onto me and my assumed defects/quirks/peccadilloes, or, indeed, anything else that can be thrown in the air, any 'shiny' object dangled to distract the attention of puzzled fellow-travellers. Failing that, they just become abusive. Having to face barrage after barrage of responses like these from the DM-fraternity (for at least three decades!) has helped convince me that they (i) Are Grade-A B.S. merchants; (ii) Don't really think about DM much beyond what they have read in the classics (since they are so easily stumped by such rather obvious objections); or, (iii) Don't care what disrepute they bring to Marxism by their advocacy of such crazy, off-the-wall ideas. (Other replies and objections from DM-supporters have been dealt with here.)]
And Therefore Never Send To Know For Whom The Bell Tolls, It Tolls For DM
Several of the points raised above require further elaboration -- in the course of which we will discover once again that Engels was actually saying nothing at all intelligible. As we have seen several times already, Engels asserted the following:
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
However, by so doing he was clearly appealing to what he regarded as the established, inter-subjective meaning of terms like "motion", "change", "place", "moment" and "time". This can be seen from the fact that he didn't even think to define or explain what he meant by these words.
Engels did, however, offer an aside (which was hardly a definition) in the above passage, to the effect that motion is a "simple mechanical change of place", an idea he reiterated in DN:
"All motion is bound up with some change of place, whether it be change of place of heavenly bodies, terrestrial masses, molecules, atoms, or ether particles. The higher the form of motion, the smaller this change of place. It in no way exhausts the nature of the motion concerned, but it is inseparable from the motion. It, therefore, has to be investigated before anything else." [Engels (1954), p.70. Bold emphasis added.]
[I will re-examine what Engels had to say in the above passage, later on in this Essay.]
Ordinarily, this lack of precision wouldn't be a problem since we understand words like these perfectly well in our day-to-day lives, and we typically do so without the need to refer to or check any definitions. But, in highly specialised areas of philosophy and science -- particularly those associated with any attempt to revise or correct the way we understand, or even perceive, objects and events --, such a sloppy approach to the use of language isn't just unacceptable, it is counter-productive. Indeed, a cavalier attitude to ordinary language like this has a tendency to backfire on anyone foolish enough to so indulge. Again, this is especially true of those who try to press the vernacular into service way beyond its prosaic remit.
The ability to manoeuvre with apparent ease one's way around linguistic conundrums like this, and (as we will see) often sweep them under the carpet, is supposedly what dialecticians mean by "grasping a contradiction". This seems to imply that when confronted with the many 'contradictions' that nature allegedly displays, dialecticians merely have to "grasp" them and all is well. That neat trick then 'allows' such serial 'graspers' to ignore the internal contradictions this cavalier approach introduces into their own theory. [The serious problems this tactic brings in its train have been explored and exposed here and here.]
However, as we will see in Essay Seven (and here), DM-theorists are highly selective when it comes to deciding which 'contradictions' they should "grasp", which they should simply drop -- or, and far more likely, which they should simply ignore, and which they should simply blame on the defective nature of a rival theory. Hence, when dialecticians "grasp" the 'contradictions' they claim to see in motion and change they readily attribute them to nature itself -- failing to blame them on Hegel's logical incompetence, Engels's lack of clarity, or, indeed, on Zeno's confused Idealist fantasies.
On the other hand, when a contradiction is detected in a rival theory, that becomes a handy excuse to berate anyone foolish enough to accept or promote it, and which then provides a convenient excuse to reject it out-of-hand. By way of contrast, DM-apologists are remarkably forgiving of the contradictions implied by their own theory, which aren't allowed to suggest -- under any circumstances -- that it is defective or in need of revision. Quite the reverse; the 'contradictions' conjured by DM must be worn proudly, as a badge of honour!
So, for example, we are told that by 'resolving' certain contradictions science has been able to progress. [I have covered this topic in detail in Essay Eleven Part One; readers are directed there for the relevant proof texts and analysis. Also see Appendix A to this Essay.] But, if science advances by rejecting or 'resolving' contradictions in and between theories, or in and between a theory and observation, it would seem that the scientific theory of motion itself can't advance unless and until the 'dialectical contradictions' implied by a moving body (according to Engels) have also been resolved. However, as soon as that has been done, dialecticians will surely have to abandon their belief in the 'contradictory' nature of motion -- or, of course, risk holding up the progress of science.
This self-inflicted quandary I have elsewhere called "The Dialecticians' Dilemma".
As seems obvious, 'dialectically grasping' a 'contradiction' doesn't make it disappear. Even if we grant (for the purposes of argument) the veracity of DM, motion would still be 'contradictory' whether or not anyone else viewed it that way. Hence, the significance of "grasping a contradiction" appears to be little more than this: Certain processes in nature and society, which might seem puzzling or paradoxical (to some) cease bothering others when they become dialecticians. They have seen the light and have "grasped" these oddities, which means they can now move on (no pun intended). But, this 'sweep everything problematic under the carpet' tactic only works if it is also acknowledged that this is the way the world actually is -- i.e., that it is indeed contradictory. In that case, on this basis, DM-theorists clearly think they can stop worrying about the contradictions at the heart of their own theory. While they openly accept the supposed fact that nature is deeply perplexing, a pair of well-adjusted DM-spectacles allows it to be viewed correctly -- where "viewed correctly" appears to mean "Ignore what you can't explain and then accuse critics of 'not understanding' dialectics".
Rinse and repeat...
Despite the DM-spin, the above approach nevertheless implicitly admits it is impossible to explain what, for instance, it means for something to be in two different places at once (save in the ambiguous manner described earlier in this Essay, and again below). If that is so, the dialectical 'analysis' of motion turns out be of little use to anyone, least of all dialecticians. That is because it is clear that not even they can explain motion, since all their theory does is re-describe it in an equally perplexing manner. All that Engels's 'analysis' seems to have achieved, therefore, is to stop dialecticians worrying about their own defective theory (defective since it contains, or implies, so many contradictions!), all the while leaving motion, as they see it, no less 'paradoxical'.
We have also seen that, even if DM were a correct description of, or a valid theory about, 'reality', Engels's view of motion does no actual work, least of all anything of use in connection with revolutionary practice. After all, how does it help anyone change the world to be told that motion is contradictory? How does it even help scientists to be told that motion is a contradiction? Can they use it to predict anything? Can technologists and engineers use it to help control nature, or design and then construct something? How many bridges can be built on the basis of the belief that motion is a contradiction? How many strikes won? Or even leaflets printed?
What dialectical --, or, indeed, any -- use is this aspect of DM?
In that case, if there is in fact a rational solution to this 'paradox' -- if we but knew what it was --, it is no good looking to dialecticians for assistance trying to find it. They gave up on that score the moment they leafed through Hegel's 'Logic' and began "grasping" 'contradictions'.
Left to DM-fans, the advance of at least this branch of Physics and Applied Mathematics would grind to a halt.23
[Irony intended.]
However, Engels did at least make some attempt to use what looked like ordinary words in his effort to show that they, or what they supposedly reflected, weren't all they seemed -- i.e., that when considered 'dialectically' the vernacular reveals more about reality than might otherwise have been suspected, especially by those mesmerised by 'commonsense' or blinded by 'formal thinking'. Or, indeed, those who have been bamboozled by that 'inner fifth-columnist', the "abstract understanding" (very helpfully identified for us by Hegel without the use of a single consulting couch, or even a brain scan, let alone a degree in psychology).
Nevertheless, anyone who disagrees with the 'dialectical' conclusions Engels drew would no doubt be reminded that these few words -- or, the 'concepts', objects and processes they supposedly 'represent' -- clearly and unambiguously imply the 'contradictions' that Engels and Hegel said they did. In that case, defenders of the 'dialectical view' of 'reality' could claim that Hegel and Engels had actually made explicit what were in fact implicit 'contradictions' in our knowledge of the world and how it works, not just in the language we use to express that (assumed) fact. Hence, whether we acknowledge it or not, we are all 'unconscious dialecticians'.
"Every individual is a dialectician to some extent or other, in most cases, unconsciously. A housewife knows that a certain amount of salt flavours soup agreeably, but that added salt makes the soup unpalatable. Consequently, an illiterate peasant woman guides herself in cooking soup by the Hegelian law of the transformation of quantity into quality…. Even animals arrive at their practical conclusions…on the basis of the Hegelian dialectic." [Trotsky (1971), pp.106-07. Italic emphasis in the original.]
[I have commented at length on this unfortunate passage, which does Trotsky few favours, in Essay Seven Part One, here, here and here.]
Intentionally or not, by arguing the way he did Engels succeeded in connecting this paradoxical idea with an ancient metaphysical tradition stretching back to Zeno, Parmenides and Heraclitus, a tradition that ordinary working people had no hand in building but which was (demonstrably) based on ruling-class priorities, forms-of-thought and a distortion of the vernacular, the only language that links humanity directly with the material world, as Marx himself pointed out:
"One of the most difficult tasks confronting philosophers is to descend from the world of thought to the actual world. Language is the immediate actuality of thought. Just as philosophers have given thought an independent existence, so they were bound to make language into an independent realm. This is the secret of philosophical language, in which thoughts in the form of words have their own content. The problem of descending from the world of thoughts to the actual world is turned into the problem of descending from language to life.... The philosophers have only to dissolve their language into the ordinary language, from which it is abstracted, in order to recognise it, as the distorted language of the actual world, and to realise that neither thoughts nor language in themselves form a realm of their own, that they are only manifestations of actual life." [Marx and Engels (1970), p.118. Bold emphases alone added. Paragraphs merged.]
Indeed, Engels's approach (in this area) began to falter when he attempted to squeeze some metaphysical juice out of such desiccated philosophical lemons; that is, when he tried to extract 'paradoxical' conclusions from a few rather innocent-looking words -- which had been suitably doctored, of course.
Naturally, only those who already accept the theory that 'reality is fundamentally contradictory' will automatically agree with the conclusions Engels drew. Others, however, might be forgiven for remaining sceptical, particularly those who (not unreasonably) think that Engels's 'solution' is far more paradoxical and puzzling than the original 'problem' had ever been. Indeed, if the nature of motion is 'problematic', calling it "contradictory" -- while making no attempt to explain how that actually accounts for anything -- explains nothing. Nor has it any practical applications; hence, as such, it worse than useless. So, if the 'contradictions' Engels claimed to have discovered in moving bodies does no work (as was argued earlier, here and here), their presence is, at best, a hindrance. That is because we can now see that they are the product of an over-active imagination, compounded by a gullible acceptance of the Idealist gobbledygook Hegel and Zeno inflicted on humanity. Because of that this theory has led Dialectical Marxists off in an entirely negative theoretical and practical direction, one that has served us badly for well over a century (as will be demonstrated in detail in Essays Nine Part Two and Ten Part One), to put it mildly!
In that case, Engels's 'analysis' is a serious obstacle to our understanding (anything!), which will, of course, need to be rejected and abandoned if science -- let alone Marxism -- is to advance.
As we are about to see, Engels failed to consider several other far more likely possibilities. It looks like it never even occurred to him that his 'contradictory' conclusions might fail to follow if he had instead given consideration to the full range of words and/or meanings available to ordinary language users in this area of discourse. These resources are easily accessed by those determined to employ the vernacular with a far greater concern for consistency, honesty and sensitivity than Engels, Hegel, or Zeno ever seem to have managed (in this respect).24
Engels clearly wanted to make a specific point about the paradoxical implications of a handful of seemingly innocent-looking, ordinary words. As we will see, he did this by unwittingly altering their everyday use/meaning while imagining that the meaning of several other ordinary terms normally associated with them remained unaffected.
In so doing he wasn't, of course, alone. Semantic sleight-of-hand (aka 'word magic') has been the sport of choice throughout the entire history of Traditional Philosophy; and this 'time-honoured' practice continues to this day. Even careful philosophers are guilty of this, often failing to notice that their own ideas are predicated on what can only be described as "piecemeal selectivity" over their use of language. Indeed, many simply assumed it was possible to tinker around with a handful of words while the meaning of other terms normally associated with them remained unaffected. That is, they imagined there was no knock-on, 'ripple' effect at work here. Piecemeal selectivity like this is, alas, double-edged. In fact, these associated words -- whose meanings in this case Engels also simply took for granted -- prove to be equally (if not more) problematic than those on which he chose to focus his attention.
As we are about to discover, this unexpected turn of events will not only undermine Engels's (sketchy) 'analysis' of motion, it will vitiate every single classical theory, too.
If, according to Hegel and Engels, an ordinary word like "motion" possess 'contradictory' implications, then perhaps other terms they failed to consider might have analogously paradoxical connotations, especially given this perverse way of viewing language. What about the word "place", for instance? What if it turns out to be just as 'problematic'? In such circumstances, could we continue to accept the validity of Hegel and Engels's conclusions (about "motion") if the interplay between these two intimately connected words is more complex than they both imagined? That is, where an alteration to one of these terms only succeeds in radically changing the other? More pointedly: What if certain uses of the word "place" end up neutralising Hegel and Engels's (quirky) interpretation of the word "move"?
Clearly, Engels's argument requires the meaning of "place" to remain fixed while he tinkered around with "move". But, if "place" itself has no single meaning, any conclusions based on the supposition that it has just one and only one such will automatically come under suspicion. Worse still, any argument based on a specific aspect of the ordinary meaning of "place" that undercuts the supposed 'philosophical' implications of "motion" will be thrown into even greater doubt. That is because, in view of their intimate connection, if the meaning of "move" is compromised by the slippery meaning of "place"/"space" (or, indeed, vice versa), the import of neither will remain unscathed.
In fact, as we are about to find out, close connections like this have the (salutary) effect of deflating the philosophically grandiose conclusions Engels and others thought they could derive from a handful of ink marks on the page -- when, for example, they employed a non-standard use of "move" with what they took to be a standard use of "place"/"space", and vice versa.
Ordinary Objects Regularly Do The Seemingly Impossible
We have already seen that many of the ambiguities in Engels's analysis of motion seem to depend on overall vagueness in the meaning of the word "place" and its cognates. Even when translated into the precise language of coordinate geometry/algebra the meaning of "place" doesn't become much clearer (when used in such a weird context) -- or any the less ambiguous, as we are about to find out.
Of course, such criticism isn't aimed at the vernacular; imprecision is one of its many strengths. Nor is it to malign mathematics! But, when ordinary words are employed by Philosophers, who almost always assume (implicitly or explicitly) they have a single unique (or 'essential') meaning that only they can access, problems invariably arise. Indeed, as Marx pointed out (quoted below) and Wittgenstein also reiterated:
"I think that essentially we have only one language, and that is our everyday language.... [O]ur everyday language is the language, provided we rid it of the obscurities that lie hidden in it. Our language is completely in order, as long as we are clear about what it symbolizes." [Waismann (1979), pp.45-46. Paragraphs merged.]
"You ask why grammatical problems are so tough and seemingly ineradicable. -- Because they are connected with the oldest thought habits, i.e., with the oldest images that are engraved into our language itself (Lichtenberg).... Language has the same traps ready for everyone; the immense network of easily trodden false paths. And thus we see one person after another walking down the same paths....
"One keeps hearing the remark that philosophy really doesn't make any progress, that the same philosophical problems that occupied the Greeks keep occupying us. But those who say that don't understand the reason this must be so. The reason is that our language has remained constant and keeps seducing us into asking the same questions. So long as there is a verb 'be' that seems to function like 'eat' and 'drink', so long as there are the adjectives 'identical', 'true', 'false', 'possible', so long as there is talk about a flow of time and an expanse of space, etc., etc. humans will continue to bump up against the same mysterious difficulties, and stare at something that no explanation seems able to remove....
"I read '...philosophers are no nearer to the meaning of 'Reality' than Plato got...'. What a strange state of affairs. How strange in that case that Plato could get that far in the first place! Or that after him we were not able to get further. Was it because Plato was so clever?" [Wittgenstein (2013), pp.311-12e. Italic emphases in the original; quotation marks altered to conform with the conventions adopted at this site. Some paragraphs merged.]
A point underlined by (ordinary language) philosopher, Margaret Macdonald:
"Philosophical theories which claim to state facts in much the same sense as physical theories will be found, I suggest, to appeal for evidence not to experience but to 'what we say' in certain relevant circumstances. They depend for their understanding, as scientific theories do not, entirely upon the known uses of ordinary words. They do not extend the use of these words but generally only misuse them. It is for this reason that such philosophical propositions have been called senseless. They try to operate with ordinary words when they have deprived them of their ordinary functions. They recombine known words in an unfamiliar way while trading on their familiar meanings. But [this leads] to hopeless difficulties and so it seems that philosophical problems are never solved at all. Nor could they be solved, or even tackled satisfactorily, while the verbal character of both questions and answers was realised only half, or not at all. But if it is realised and is correct, then the only help we can get in tackling philosophical problems is from understanding the uses of words and their use and misuse by philosophers." [Macdonald (1963), pp.82-83. Bold emphases alone added.]
Which seems to me to be the same advice that Marx was dishing out a century earlier (mentioned above):
"The philosophers have only to dissolve their language into the ordinary language, from which it is abstracted, in order to recognise it, as the distorted language of the actual world, and to realise that neither thoughts nor language in themselves form a realm of their own, that they are only manifestations of actual life." [Marx and Engels (1970), p.118. Bold emphasis added.]
An approach endorsed even more recently (in relation to the Philosophy of Mind, but the points made apply equally well in this area):
"As to the widespread disparagement of attempts to resolve philosophical problems by way of appeals to 'what we would ordinarily say', we would proffer the following comment. It often appears that those who engage in such disparaging nonetheless themselves often do what they programmatically disparage, for it seems to us at least arguable that many of the central philosophical questions are in fact, and despite protestations to the contrary, being argued about in terms of appeals (albeit often inept) to 'what we would ordinarily say...'. That the main issues of contemporary philosophy of mind are essentially about language (in the sense that they arise from and struggle with confusions over the meanings of ordinary words) is a position which, we insist, can still reasonably be proposed and defended. We shall claim here that most, if not all, of the conundrums, controversies and challenges of the philosophy of mind in the late twentieth century consist in a collectively assertive, although bewildered, attitude toward such ordinary linguistic terms as 'mind' itself, 'consciousness', 'thought', 'belief', 'intention' and so on, and that the problems which are posed are ones which characteristically are of the form which ask what we should say if confronted with certain facts, as described....
"We have absolutely nothing against the coining of new, technical uses [of words], as we have said. Rather, the issue is that many of those who insist upon speaking of machines' 'thinking' and 'understanding' do not intend in the least to be coining new, restrictively technical, uses for these terms. It is not, for example, that they have decided to call a new kind of machine an 'understanding machine', where the word 'understanding' now means something different from what we ordinarily mean by that word. On the contrary, the philosophical cachet derives entirely from their insisting that they are using the words 'thinking' and 'understanding' in the same sense that we ordinarily use them. The aim is quite characteristically to provoke, challenge and confront the rest of us. Their objective is to contradict something that the rest of us believe. What the 'rest of us' believe is simply this: thinking and understanding is something distinctive to human beings..., and that these capacities set us apart from the merely mechanical.... The argument that a machine can think or understand, therefore, is of interest precisely because it features a use of the words 'think' and 'understand' which is intendedly the same as the ordinary use. Otherwise, the sense of challenge and, consequently, of interest would evaporate.... If engineers were to make 'understand' and 'think' into technical terms, ones with special, technical meanings different and distinct from those we ordinarily take them to have, then, of course, their claims to have built machines which think or understand would have no bearing whatsoever upon our inclination ordinarily to say that, in the ordinary sense, machines do not think or understand." [Button, et al (1995), pp.12, 20-21. Italic emphases in the original. Quotation marks altered to conform with the conventions adopted at this site.]
Engels didn't think he was using "move" or "place" (etc.) in a technical sense, but in a way he hoped was familiar to us all (which explains why he offered no definition or explanation of their meaning), when he said things like this:
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them.... But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
"All motion is bound up with some change of place, whether it be change of place of heavenly bodies, terrestrial masses, molecules, atoms, or ether particles. The higher the form of motion, the smaller this change of place. It in no way exhausts the nature of the motion concerned, but it is inseparable from the motion. It, therefore, has to be investigated before anything else." [Engels (1954), p.70. Bold emphasis added.]
As it turns out, in ordinary discourse there is no such thing as the meaning of the word "place", or, for that matter, even of "move".
Unfortunately, this also spills over into the use of technical terms associated with either word. The construction and application of a coordinate system, for example, requires the use of rules, none of which are self-interpreting. [The point of that comment will emerge presently.]
Nevertheless, it is relatively easy to show -- by means of the sort of selective linguistic adjustment beloved of metaphysicians, but applied in areas and contexts they generally fail to consider (or, rather, which they choose either to ignore or downplay) -- that ordinary objects and people are quite capable of doing the 'metaphysically impossible' on a regular basis. The flexibility built into the vernacular actually 'enables' the mundane to do the 'miraculous', and every day of the week, too. Such mundane 'prodigies' don't normally bother us -- well, not until some bright spark tries to do a little 'philosophising' with them.24a
If the ordinary word "place" is now employed with/in one or more of its usual senses, it is easy to show that much of what Engels had to say about motion becomes either false or uninteresting. Otherwise, we would be forced to concede that ordinary people and objects can behave in extraordinary -- if not 'miraculous' -- ways.
Consider the following (seemingly innocuous) example:
L41: The strikers refused to leave their place of work and busied themselves building another barricade.
Assuming that the reference of "place" is clear from the context (that it is, say, a factory), L41 actually depicts objects moving while they remain in the same place! But that is contrary to what Engels said (or implied) was possible. Indeed, if this (familiar, everyday) sort of motion is interpreted metaphysically, it would involve ordinary human beings doing the impossible: moving while staying still!
For what else is remaining in the same place other than keeping perfectly still?
In this case, ordinary workers seem capable of doing the physically impossible, moving while not moving!
A 'contradiction', surely?
Well, only to the philosophically naive.
Or, rather, only to the Idealist Mystics among us who want to undermine our ordinary view of 'reality' and claim there is a hidden, 'contradictory' world behind 'appearances' that is 'more real' than the physical universe. A world of 'concepts', 'ideas', 'negations', 'abstractions', 'essences'...
But, who in their left mind would want to do that? Even worse: who would be prepared to believe a word they said?
Ah, yes: only the politically and philosophically gullible...
That's who.
Of course, one obvious response to the above 'contradiction' would be to claim that L41 is a highly contentious example, and not at all what Engels (or other metaphysicians) had in mind by their use of the word "place".
[That was a point actually made a few paragraphs back!]
But, Engels didn't tell us what he meant by this term; he simply assumed we would 'understand' his use of it.
[Again, that was also the point of all the preamble set out in the last few paragraphs and sub-sections.]
Here is what he did say:
"Motion in the most general sense, conceived as the mode of existence, the inherent attribute, of matter, comprehends all changes and processes occurring in the universe, from mere change of place right up to thinking.... All motion is bound up with some change of place, whether it be change of place of heavenly bodies, terrestrial masses, molecules, atoms, or ether particles. The higher the form of motion, the smaller this change of place. It in no way exhausts the nature of the motion concerned, but it is inseparable from the motion." [Engels (1954), pp.69-70. Bold emphasis added; paragraphs merged.]
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added]
There is nowhere in there where tells his readers exactly what he meant by "place".
[I have been checking for more years than I care to count, but Engels nowhere tells us what he meant either by "place" or "position". If anyone thinks differently, please email me with the (correct) details.]
It could be countered that it is perfectly clear what he meant by his use of these words, but as we are about to find out, that isn't so.
[In what follows I will largely focus on "place" and its cognates.]
However, if it is now claimed that Engels clearly didn't mean by "place" a sort of vague "general location" (such as the factory mentioned in the above example), then that would confirm the point being made in this part of the Essay: Engels didn't say what he meant by "place" since there was nothing he could have said that wouldn't also have ruined his entire argument. Tinker around with the word "place" and the meaning of "motion" can't fail to be compromised (again, as noted earlier). That can be seen by considering the following highly informal 'argument':
L42: Nothing that moves can stay in the same place.
L43: If anything stays in the same place, it can't move. [L42 re-worded.]
L44: A factory is one place where workers work.
L45: Workers move about in factories.
L46: Any worker who moves can't stay in the same place (by L43 and modus tollens).
L47: Hence, if workers move they can't do so in factories (by L44 and L45).
L48: But, some workers remain in factories while they work; hence, while they are there, either they can't move or they can't work (by L43).
L49: Therefore, either workers work and do not work (in factories) -- or they move and they do not move.
As soon as one meaning of "place" is altered (as it was in L44), one sense of "move" is automatically affected (in L45 and L46), and vice versa (in both L47 and L48). In one understanding of "place", things can't move (in another sense of "move") while staying in one place (in yet another meaning of "place"). But, in still another sense of both they can, and what is more they typically do both. Failure to notice this produces 'contradictions' to order, and everywhere (as we saw in L49).
If the above example confuses anyone, think about moving around your flat or house. It is quite clear that while doing that you move but remain in the same place. In that case, if moving means you have to change place, then you must move and not move at the same time -- either that, of move house!. Here a looser sense of "place" undermines one meaning of "move", for it seems you have "moved" while remaining perfectly still -- i.e., while remaining in the same "place"!
That appears to contradict Engels:
"All motion is bound up with some change of place, whether it be change of place of heavenly bodies, terrestrial masses, molecules, atoms, or ether particles. The higher the form of motion, the smaller this change of place. It in no way exhausts the nature of the motion concerned, but it is inseparable from the motion." [Engels (1954), pp.69-70. Bold emphases added.]
Does that mean that when you move around your house/flat you actually have to move to another house/flat? But Engels says you do: "All motion is bound up with some change of place...."! Either that, or you can't actually move around inside your house/flat.
Of course, no one believes that, but Engels's careless use of words suggests the above is indeed the case.
Even so, who believes that workers work and do not work in factories? Or, that they move and do not move while staying in the same place? Who believes that you are able to move around your house or flat while remaining still? That is, every day of your life, you move and do not move at the same time?
Who really believes such absurd 'contradictions'?
Maybe only those who 'understand' dialectics...?
'Dialectical Objects' Do The Oddest Things
Moving While Remaining Perfectly Still
The previous sub-section raised serious concerns over Engels's careless use of language, but it might still be thought that if we focus on what Engels obviously meant by his employment of words like "move" and "place", and ignore specious objections (like those aired above about workers moving and while they supposedly remain still), his theory will clearly remain intact.
However, what Engels says about motion has to be able to take account of ordinary moving objects in everyday situations if it is to apply to the real world, not philosophical abstractions and physically meaningless mathematical 'points'. Unfortunately, as we are about to find out, that is precisely what his 'theory' can't do.
In response, it could be objected that it might be possible to understand what Engels and Hegel were trying to say if "place" was delineated precisely without altering the meaning of "move", contrary to what was argued earlier. In that case, it could be maintained that if "place" were defined by the use of crystal clear spatial coordinates (henceforth, SCs), Engels's account of motion would continue to be viable.
Or, so some might like to think...
Of course, the problem here is that in the example given above (concerning those contradictory mobile/stationary workers), if we try to define the meaning of the word "place" a little more precisely, it will start to mean something like the following:
F1: Df. Place: A finite three-dimensional region (of space) large enough to contain the required object.
Well, plainly, in that sense things can and do move about while they remain in the same region (i.e., "place") -- since, by default, any object occupies such a region as it moves; that is, it must always occupy a three-dimensional region of space large enough to contain it. They certainly don't occupy larger or smaller spaces in that sense (unless they expand or contract)! Moreover, objects occupy such finite regions while they move -- or they wouldn't be able to move!
Hence, if defined that way, moving objects always occupy the same space, and, if we were to believe Engels, they wouldn't be able to move while they are doing that! Hence, if they always stay in the same space, they can't move -- if we insist on characterising "motion" the way Engels and Hegel thought they could and we define space along lines suggested by F1 above.
After all, this is what Engels had to say:
"Motion in the most general sense, conceived as the mode of existence, the inherent attribute, of matter, comprehends all changes and processes occurring in the universe, from mere change of place right up to thinking.... All motion is bound up with some change of place, whether it be change of place of heavenly bodies, terrestrial masses, molecules, atoms, or ether particles. The higher the form of motion, the smaller this change of place. It in no way exhausts the nature of the motion concerned, but it is inseparable from the motion." [Engels (1954), pp.69-70. Bold emphasis added; paragraphs merged.]
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added]
The obvious implication of the above is that if an object isn't involved in a "change of place", it can't be moving! But, as we have just seen, objects always occupy the same space (if we accept F1), even as they move.
So, if we insist on being paradoxical, when defined this way objects either move and don't move, or they remain in the same place and are therefore motionless -- hence they only seem to move! Appearances are here 'contradicted' by 'underlying reality'!
F1 and Engels's words clearly seem to imply the above paradoxical conclusion (over and above what he concluded about the 'contradictory nature of motion'). However, it is equally clear that he didn't intend for his theory to imply that moving objects only seem to move, while they don't really do so (since they always remain in the same place (according to F1)).
Plainly, in order to circumvent this latest 'difficulty', we need to be even more precise and clear about what we mean.
Of the many problems and confusions that still remain unresolved (concerning "motion" and "place"), the following handful of Options seem most relevant or the most pressing (with respect to the immediate issues at hand):
(1) If an object always occupies the same space (which fits it like a glove, as it moves), then it can't actually move! [This follows directly from F1.]
(2) If an object occupies a larger space as it moves, it must expand.
(3) If an object moves about in the same region of space (such as a factory), it still can't move! [This seems to be another implication of F1.]
[Or: (3a) If an object remains in the same place, it can't be moving. (From F1, again.)]
[(4) However, if an object successively occupies spaces equal to its own volume as it moves, the situation is even worse, as we will soon discover.]
So, if the 'region' concerned (in which, by means of which, or even through which an object is said to move) is constrained too much, nothing would be able to move -- that is Option (1). Hence, if we put each worker in a tightly-fitting steel box (that fits him or her exactly) they would be rooted to the spot. All locomotion would cease.
On the other hand, put that worker in a larger region of space and it looks like they still won't be able to move -- this is Option (3). That is because if we define motion as successive occupancy of regions of space within a broader region, then this worker still can't move since he/she is always in the same broader region, the same space -- for example, a factory.
Or this is you, as you move around your house or flat.
Again, this seems to follow directly from F1 and what Engels had to say about motion and "change of place".
The difficulty here is plainly one of relaxing the definition of the required region that an object occupies sufficiently enough to allow it to move from one place to another without stopping it moving altogether. Hence, this problem revolves around preventing Option (3) from undermining what we might ordinarily want to call motion/locomotion, the successive occupancy of certain regions of space -- i.e., the first half of Option (4) --, all the while providing an account that accommodates the movement/locomotion of sized objects in the real world.
(3) If an object moves about in the same region of space (such as a factory), it still can't move!
(3a) If an object remains in the same place, it can't be moving.
(4) However, if an object successively occupies spaces equal to its own volume as it moves, the situation is even worse....
But, just as soon as that is done -- if we, say, relax the definition of the space or the place involved, making it larger, for example -- the above difficulties immediately re-appear. That is because in such an eventuality an object will still move while staying in the same place -- i.e., if the place allowed is big enough for it to do just that! [This is Option (3) -- or (3a), again: If an object remains in the same place, it can't be moving!]
Indeed, the obverse of the above enables most (if not all) of the locomotion in the entire universe:
(5) Everything that moves does so in the same place: i.e., the universe!
Clearly, in the limit, if anything moves in nature, it must remain in the same place -- i.e., it must remain in the universe! Unless an object travels beyond the confines of the universe (if such a thing were possible!), that must always be the case. So, the said object moves while remaining in the same place -- i.e., it remains in the universe!
Of course, that relaxes the definition of "same place" far too much. But, the problem now is how we are to tighten the definition of "place" so that objects aren't put in straight-jackets once more. [I.e., Option (1).]
(1) If an object always occupies the same space (which fits it like a glove, as it moves), then it can't actually move!
If everything that moves does so in the same place -- i.e., the universe -- and if staying in the same place means that such objects don't move [Option (3a)], then nothing at all moves in the entire universe, even while it plainly does!
(3a) If an object remains in the same place, it can't be moving.
The reader may now perhaps appreciate how such a (deliberately!) sloppy use of language (that already has vagueness built into it!) results in the easy creation of a 'contradiction' of such prodigious proportions (seemingly out of nowhere): that there is and there isn't any motion at all in the entire universe!
But who believes that that is a contradiction?
Anyone who cared to gamble on this would, I think, win a pretty large bet that there isn't a single DM-fan on the planet who thinks this is a 'dialectical contradiction' (or even an ordinary one)! And yet, such individuals are prepared to believe Hegel and Engels who manufactured a 'contradiction' on the basis of a similar sloppy use of (vague) language.
Be this as it may, at first sight the above (pro-DM) objection (concerning the provision of a precise enough definition of "place") seems reasonable enough. Engels clearly meant something a little more specific than a vague or general sort of location (like a factory). But what? He didn't say, and his epigones haven't, either. There has been well over a century of silence on this issue! Indeed, it is by now perfectly clear that DM-fans fail even to recognise this as a problem, so slapdash has their thought become. [And good luck finding a clear definition in Hegel! Even more such luck eliciting an answer from DM-fans! I've been trying for over thirty years!]
It might still seem possible to rescue Engels's theory if tighter rules for defining "place" were prescribed -- perhaps involving a reference to "a (zero volume) mathematical point in three-dimensional space, located by the use of precise SCs". But, that option would embroil Engels's account in far more intractable problems, since it would (plainly!) involve mathematical point locations, or even the movement of mathematical points themselves -- and, as we saw earlier, that is itself a non-starter.
[SC = Spatial Co-ordinate.]
Clearly, things can't move about in such points -- but that has nothing to do with the supposed 'nature of reality'. Mathematical points can't contain anything, and that is because they aren't containers. They have no volume, no shape, no circumference, no diameter and are made of nothing. If that weren't the case, they wouldn't be mathematical points, they would be regions, or volume intervals. [More on those, presently.]
As noted above, if Engels meant something like this (by his use of "place"), his account would fail to explain (or accommodate) the movement of ordinary material bodies in the world around us. Clearly, they don't occupy mathematical points.
And, it is no use appealing to larger numbers or sets of such points (located by SCs or other technical devices); no material body can occupy an arbitrary number of points, since points aren't containers.
Perhaps we could define a region, or a finite volume interval by the use of SCs, or even via set theory? Maybe so, but that would only introduce another classical conundrum (which is itself a variation on several of Zeno's other paradoxes): how it is possible for a region (or a volume interval) to be composed of points that have no volume. Even an infinite number of zero volume mathematical points adds up to zero. Now, there are those who think this conundrum has a solution just as there are those who think it doesn't, but it would seem reasonably clear that the difficulties surrounding Engels's 'theory' aren't likely to be helped by importing several more 'problems' from another set of equally perplexing paradoxes -- especially when those paradoxes gain purchase from the same linguistic ambiguities and vagaries about "space" and "volume" that bedevilled "motion" and "place"!
We seem to be going round in circles.
[No irony or pun intended. For a reader-friendly book dealing with these topics, cf., Sorensen (2005). The above 'paradox' is covered on pp.45-48. Also see Huggett (2018), especially here.]
Be this as it may once more, it is far more likely that Engels's use of the word "place" is itself an implicit reference to a finite three-dimensional volume interval (whose limits can be defined by the application of well-understood rules in Real and Complex Analysis, Vector Calculus, Set Theory, Coordinate Algebra and Differential Geometry, etc., etc.).
Clearly, such volume intervals must be large enough to hold (even temporarily) a given material object. If so, this use of "volume interval" would in principle be no different from an earlier use of "place" to depict the movement of those workers! If objects can move about in locations big enough to contain them, and remain in the same place while doing so, Engels's moving objects must be able to do likewise, except they would now have a more precise "place" or region in which to do it.
However, and alas, this sense of "place" is no use at all, for, as already noted, when objects (such as workers) move, they will, by definition, stay in the same place! So, it seems this must be the case with Engels's moving objects, if we try to depict "place" this way. But, that is just Option (3a), again!
(3a) If an object remains in the same place, it can't be moving.
Such 'moving' objects wouldn't therefore be moving (in one sense of that word)! All we would be left with was a more precise location in which they would be stationary/'moving'!
So, not even greater precision seems to help Engels.
Naturally, the only apparent way to circumvent this latest 'difficulty' would be to argue along the following lines:
F2: The location of any object must be a region of space (given by a volume interval) equal to that object's own volume.
But, this is just a re-statement of one of the classical definitions -- i.e., F1, from earlier. In that case, one way to avoid the above problem would be to point out that as the said object moved, its own exact volume interval would move with it. Such a containing volume/space would follow each moving object everywhere it went, and it would do so more faithfully than its own shadow, more doggedly than a world-champion bloodhound. But, clearly, if that were the case, it would mean that any such object would still move while staying in the same place! Plainly, any object (even one that moves) always occupies a space equal to its own volume, which would on this view travel everywhere with it -- like a sort of metaphysical glove. Worded that way, F2 is no use to Engels (or Hegel)! That is because it is Option (1) and Option (3a), again!
(1) If an object always occupies the same space (which fits it like a glove, as it moves), then it can't actually move!
(3a) If an object remains in the same place, it can't be moving.
As should seem reasonably clear: in relation to F2 we now have two problems where once there was only one. That is because we should have to explain not only how bodies can move but how it is also possible for volume intervals to move so that they can faithfully shadow the objects they contain!
A detachable, moveable volume interval big enough to contain a moving body as it moves only adds to our problems; it doesn't help resolve them!
Furthermore, and perhaps worse, not only would we have to explain how locations (i.e., these volume intervals) are themselves capable of moving, we would also have to explain what on earth they could possibly move into!
What sort of 'ghostly regions of space' could we appeal to in order to allow other regions of space to move into them?
Even worse still: these 'moving volume intervals' must also occupy volumes equal to their own volume if they are to move (given this 'tighter' way of characterising motion, expressed in F2 (repeated below)). And, if they do that, then these new 'extra' volume intervals (containing the original volume intervals which also contained the said moving body!) must now act as secondary 'metaphysical containers', as it were, to the original 'ontological gloves' we met earlier. Metaphorically speaking, this theory, if it took such a turn, would be moving backwards, since an infinite regress would soon confront us as spatial mittens, inside containing gloves, inside holding gauntlets pile up alarmingly to account for each successive spatial container and how any of them could possibly move without another 'metaphysical box' enabling it to do just that! As seems reasonably clear, we would only be able to account for locomotion this way if each moving object were situated at the centre of some sort of 'ontological onion', each with a potentially infinite number of 'skins'!
F2: The location of any object must be a region of space (given by a volume interval) equal to that object's own volume.
But that is just an Iterated version of Option (1)!
(1) If an object always occupies the same space (which fits it like a glove, as it moves), then it can't actually move!
It could be objected that even though objects occupy spaces equal to their own volumes, as they moved they would then proceed to occupy successive spaces of this sort (located in the path of the moving body, for example), all of which would be the exact volume needed to contain them, all of which can be located or defined precisely. Given this revised scenario, moving objects would leave their old volume intervals (their old containers) behind, successively occupying a series of new volume intervals along their trajectories, as they barrelled along.
Perhaps this is the direction we need to take?
[No pun intended.]
'Dialectical Objects' -- Do They Move Or Simply Expand?
The above (proffered) pro-DM-response now redirects our attention to Option (2) and/or Option (4) -- modified to Option (4a) --, from earlier:
(2) If an object occupies a larger space as it moves, it must expand.
(4a) An object successively occupies spaces (or volume intervals) equal to its own volume as it moves.
Option (2) is clearly absurd. If anyone wants to defend it, they are welcome to all the headaches it will bring in its train -- some of which will be detailed below.
Consider first Option (4a), which, as we are about to find out, implies Option (2):
Let us suppose that Option (4a) is a correct interpretation of what Engels meant --, and it proved to be a viable alternative, and if sense could be made of these newly accommodating, successive locations without re-duplicating the very same problems noted in the previous section. Unfortunately, even supposing all this, no DM-theorist could afford to adopt it. That is because dialecticians themselves claim that moving bodies occupy at least two such "places" at the same time, being in one of them and not in it at the same moment. Clearly, if motion were defined in such terms -- that is, if moving objects successively occupied spaces equal to their own volumes -- then they would occupy at least two such volume intervals, at once.
If that were so, 'dialectical objects' wouldn't so much move as stretch or expand!
But that is Option (2)!
(2) If an object occupies a larger space as it moves, it must expand.
To see this point more clearly (again, no pun intended!), it might be useful to examine the above claims a little more closely:
If the centre of mass [COM] of a 'dialectically moving' object, B, were located at, say, (Xk, Yk, Zk) and (Xk+1, Yk+1, Zk+1), at the same time (to satisfy the requirement that moving bodies occupy at least two such "places" at once, being in one of them and not in it), it would have to occupy a space larger than its own volume while doing so.
Let us call such a space, "S", and let the volume interval containing (Xk, Yk, Zk) and (Xk+1, Yk+1, Zk+1) be δV1, leaving it open for the time being whether S and δV1 are the same or different. Thus, if the COM of B is in two such places (i.e., (Xk, Yk, Zk) and (Xk+1, Yk+1, Zk+1)) at once, it would plainly be in S and would occupy δV1.
But, once again, that would mean that B would move while remaining in the same place -- i.e., it would remain inside S, or inside δV1 (whichever is preferred), as its COM moved from (Xk, Yk, Zk) to (Xk+1, Yk+1, Zk+1), in the same 'moment'.
Unfortunately, that is just Option (3a), again!
(3a) If an object remains in the same place, it can't be moving.
B would therefore be motionless (if we were also to rely on Engels's definition.]
[Except, of course, we can't speak of a 'dialectal object' moving from one point to the next since that would imply it was in the first before it was in the second, and that it was in the second after it was in the first. As we have seen, if such an object is in both places at the same time, there can be no "before" and no "after", here, and hence no "moving from (Xk, Yk, Zk) to (Xk+1, Yk+1, Zk+1)", either!]
Or, perhaps better: B would move while remaining in the same place -- i.e., it would remain inside S, or inside δV1 (whichever is preferred), as its COM is located at both (Xk, Yk, Zk) and (Xk+1, Yk+1, Zk+1), in the same 'moment'. [Option (3a), again!]
Now, the only way to avoid the conclusion that B moves while occupying the same place, or space, S and/or δV1 --, and hence that it remains motionless while it appears to move, just like the 'mobile/stationary' workers we encountered earlier -- would be to argue that such spaces remain where they are while B moves into successively new locations or spaces. That seems to be the gist of Option (4a):
(4a) An object successively occupies spaces (or volume intervals) equal to its own volume as it moves.
But, as B moves it still occupies δV1, only we would now have to argue that as it does so it also moves into a new δV each time -- say, δV2 -- except this new δV2 must now contain (Xk+1, Yk+1, Zk+1) and (Xk+2, Yk+2, Zk+2) -- otherwise it wouldn't be a new containing volume interval that satisfied the requirement that moving bodies occupy at least two such "places" at the same time, being in one of them and not in it.
Plainly, all objects have to occupy some volume interval or other at all times (or they would 'vanish into thin air'). However, in B's case it has to do this while also occupying a new volume interval as it locomotes -- otherwise, as we saw, it would move while being in the same place, which implies it didn't move, after all!
[Except, of course, we have already seen that there is no "while" applicable to moving DM-objects. I will, however, ignore that annoying 'problem' for now.]
So, if B occupies only one S, or only one δV, at once, it would be at rest there. [This is Option (1) and/or Option (3a).] Hence, it must occupy at least two of these at the same time (if, that is, we accept the 'dialectical theory' of motion).
(1) If an object always occupies the same space (which fits it like a glove, as it moves), then it can't actually move!
(3a) If an object remains in the same place, it can't be moving.
That being the case, the only apparent way of avoiding the conclusion that B-like objects move while staying still is to argue that they occupy two successive Ss, or two successive δVs (perhaps these partially 'overlap', perhaps they don't), at once. Unfortunately, this would now mean that B-like objects would have to occupy a volume, or a volume interval, bigger than either one of S or δV on their own, at once, and hence they must expand or stretch!
It could be objected that two such successive δVs would contain (Xk, Yk, Zk) and (Xk+1, Yk+1, Zk+1) between them -- that is, δV1 would contain (Xk, Yk, Zk) and δV2 would contain (Xk+1, Yk+1, Zk+1) --, so the above anti-DM objection is misguided.
Maybe so, but the point is that dialectical objects must occupy two δVs at once, and if that is so, both δVs will contain (Xk, Yk, Zk) and (Xk+1, Yk+1, Zk+1), jointly or severally, otherwise moving objects couldn't occupy two spaces (two δVs) at the same time.
Anyway, let us assume that the above pro-DM objection is valid and that B is still moving while it occupies δV2. As we saw earlier (in an analogous context) B must also occupy δV3 at the same time, otherwise it will be stationary while in δV2, and it must also occupy δV4 at the same time, otherwise it will be stationary while in δV3, and so on... Successive applications of this argument would have B occupying bigger and bigger volume intervals (i.e., δV1 + δV2 + δV3 + δV4 +..., + δVn), at the same time. In the limit, B would then fill the entire volume interval encompassing its own trajectory, at the same time, if it moves and if Hegel and Engels are to be believed!
There thus seems to be no way to depict the motion of 'dialectical objects' that prevents them from either:
(i) Moving while staying still [Option (3a)]; or,
(ii) Expanding alarmingly, like some sort of metaphysical Puffer Fish [Option (2)].24b
(3a) If an object remains in the same place, it can't be moving.
(2) If an object occupies a larger space as it moves, it must expand.
Figure Four: At Last! An Organism That Really Does Seem
To 'Understand' Dialectics...
Either way, Engels's theory has dropped itself into yet another Hermetic Hell Hole.
The reader should now be able to see for herself what mindless mystical mayhem is introduced into our reasoning by such a cavalier (mis)use of (vague) metaphysical language. When one sense of "move" is altered, one sense of "place" can't remain the same, nor vice versa.
Of course, no one believes these ridiculous conclusions, but there appears to be no way of avoiding them if all we have to hand are the radically defective, recklessly meagre conceptual and logical resources DL supplies its unfortunate victims -- compounded, as usual, by a cavalier misuse of ordinary language and an irresponsible disregard of common sense.
[DL = Dialectical Logic.]
Self-induced 'Dialectical Headaches' like these don't stop there, either, for It seems that 'dialectical objects' must also concertina as they move.
Consider a simple, composite body, B, made of 3 connected parts: P1, P2 and P3, all arranged in the same line (with P3 at the front), so that there are no gaps between them. Let B move such that at t1, the centre of the leading edge of P1 is at (X1, Y1, Z1), the centre of the leading edge of P2 is at (X2, Y2, Z2), and centre of the leading edge of P3 is at (X3, Y3, Z3). Let us also assume that the centre of the leading edge of P3 now moves to (X4, Y4, Z4). Finally, let us assume that the distance between each of (X1, Y1, Z1), (X2, Y2, Z2), (X3, Y3, Z3) and (X4, Y4, Z4) is the same --, i.e., d metres.
["Composite" means there are no gaps or holes in the body in question. One such might be, say, a javelin thrown at an athletics meet, or a brick thrown at some scabs.]
Now, if all moving objects occupy two places at once, and if B moves in a line parallel to the line joining the centre of the leading edge of P1 to the centre of the leading edge of P3, then the centre of the leading edge of P1 must occupy (X1, Y1, Z1) and (X2, Y2, Z2), the centre of the leading edge of P2 must occupy (X2, Y2, Z2) and (X3, Y3, Z3), and the centre of the leading edge of P3 must occupy (X3, Y3, Z3) and (X4, Y4, Z4), at the same time. In effect, B would concertina as it moved, with the front end of, say, P1 crushing or penetrating the back end of P2 and overlapping it right up to its own leading edge -- in effect, wiping P2 out! The same would happen with respect to the leading and trailing edges of P2 and P3.
Again, this result depends on the answer to an earlier question: How far apart are the two places a moving body occupies if we were to accept Engels's theory? If this distance is left indeterminate, then, as we have seen, any length will do (in this case, d metres). Even if a specific length is decided upon (say, q metres), we could make the distance between P1, P2 and P3 equal to that length, and the same result would follow. [That has been done below, anyway.]
It can't be the case that the trailing edge of P2 will leave (X2, Y2, Z2) just before the leading edge of P1 reaches it, since, as we have already seen, there is no before and no after in connection with such moving objects, since all such motion (in relation to the "places" occupied) must take place at the same time for it to constitute 'dialectical movement'.
Of course, it could be objected that P1 and P2, for example, will occupy the space between (X1, Y1, Z1) and (X2, Y2, Z2) and (X2, Y2, Z2) and (X3, Y3, Z3), respectively, as B moves, but, that isn't possible. That is because there are no gaps between any of the three parts of this object for any of them to move into. So, if B were, say, the javelin from earlier, then P1 would comprise its rear third, P2 the middle third and P3 the front end. This view of motion would therefore have each part of this object (partially or completely) crushing the one in front of it.
[Anyway, I have considered the option that there are such gaps/holes, below.]
It might be argued that the structural properties of B (i.e., intermolecular forces, etc.) will prevent the above from happening. That is undeniable, but such a response has the unfortunate consequence that while B may be in two places at once as it moves, none of its parts would be! And that in turn would imply that while B was barrelling along, none of its parts would be racing along with it -- since they aren't allowed to be in two places at once by these 'structural properties'!
However, similar problems confront any 'dialectical object' undergoing circular (or more complex forms of) movement, such as helical or spiral motion, as we are about to find out.
So, consider disc, D, of negligible thickness divided by a diameter line, Γ, into two semi-circular sectors, S1 and S2:
.jpg)
Figure Five: Disc D, With Sector, S1, On The Bottom Right, Sector, S2, On The Top Left;
Γ Is Slanted At 45º (π/4 Radians) To The Horizontal And Passes Through Centre, O
[p1 & p2 Aren't Shown. They Lie Under A & B Respectively And Don't Move.]
If we now define the centre, O, of D as the origin (through which Γ passes), and let it rotate anti-clockwise. As D rotates, the leading edge of S1 will be the top right half of Γ. Let there be a point, p1, on Γ, r units from O, with co-ordinates (r, θ1), where θ1 = π/4 radians (or 45º). As D rotates, the leading edge of S2 will be the bottom left half of Γ. Let there be another point, p2, r units from O, with co-ordinates (r, θ2), where θ2 = 5π/4 radians (or 225º). Hence, p1 and p2 are equidistant at r units from O, but in diametrically opposite directions along Γ. Also, as D rotates p1 and p2 remain fixed in mathematical space (assumed here to be Euclidean/Cartesian, but see below), and do not move.
[It is necessary to fix these two points in this way so that the following argument can proceed. θ is the angle between the horizontal and Γ as D rotates. In addition, I have used polar co-ordinates in two-dimensional Euclidean Space in order to simplify both the mathematics and my description.]
Let two small objects, A and B, be attached to Γ. Initially, A is located at p1 and B at p2 (see Figure Five). As D rotates they will move along two arcs, Q1 and Q2, respectively (which, after each complete revolution of D, will trace the circumference of an inner circle, C, radius r, centre O, pictured in Figure Six, below). So, as D rotates through the first π radians (or 180º), A will move from p1 to p2 (thus replacing B), while B will move from p2 to p1 (replacing A). In addition, the two halves of Γ (that form two radii of the circle) are initially positioned over p1 and p2, but as D rotates Γ will sweep anti-clockwise past p1 and p2 (each half of Γ moving rather like a car's wiper blade sweeps past a fixed mark on its windscreen).
Elementary mathematics tells us that the arc lengths, L1 and L2, between p1 and p2 (respectively) in either direction is πr units. That is because when A and B move along Q1 and Q2 -- A from p1 to p2, along Q1, B from p2 to p1 along Q2 -- they will both trace an arc of length 2πr/2 units = πr units. So, L1 = L2 = πr.
Hence, A and B will both travel πr units as they pass between p1 and p2 (further if D continues to rotate).
[In general, the arc length, L, of a circle radius, r, is given by the formula, L = rθ (where θ is measured in radians). In this case, the radial distance between p1 and p2 is 2r units (since p1 and p2 are both r units either side of O).]
.jpg)
Figure Six: The Inner Circle, C, Above Represents The Path Of A & B As They Move
Between p1 & p2
[Γ Has Been Removed And The Circle Colouring Changed!]
Now, if all moving objects occupy two places at once, and D rotates counter-clockwise, A must be located at p1 and p2, at the same time, and B must be located at p2 and p1, at the same time, too! D rotates through the first π radians (or 180º)
But, that result is as fatal as the one derived a few paragraphs back in relation to linear 'dialectical' movement. That is because, in this case, when D has rotated through the first π radians (180º), either:
(i) D will either totally or partially disappear when S1 and S2 occupy the same semi-circle that the other used to occupy -- that is, when A and B are both located at p1 and p2 at the same time -- the leading edge of S1 having smacked into the leading edge of S2, thus compressing one or both sectors into a region with zero area; or,
(ii) S1 and S2 will both stretch over the entire disc, covering each other as they rotate -- i.e., when A and B meet in the above manner -- the leading edge of S1 having smacked into the leading edge of S2, and the leading edge of S2 having smacked into the leading edge of S1.
As before, the above results depend on a clear answer being given to an earlier question, namely: How far apart are the two places a moving 'dialectical object' occupies at the same time, if we were to accept Engels's theory?
There are only three possibilities here:
(1) If that distance is left indeterminate, then, clearly, any distance will do -- such as the one considered above, i.e., arc lengths, L1 and L2, between p1 and p2, both of which are πr units long.
(2) On the other hand, if a specific length were finally decided upon (that is, if the day ever dawns when DM-theorists suddenly developed an uncharacteristic concern for precision), we could label whatever 'dialectical distance' they finally choose, "LH" -- which is short for, "Hegel Distance". In that case, all we need do is make D itself so small that L1 = L2 = LH (i.e., LH = πr, since D and r will now both be microscopically tiny).
(3) Alternatively, we could slice D into enough tiny sectors so that L1 = L2 = LH, again. That would greatly reduce rθ, once more, but this time this would be the result of θ -- the angle between any two radii -- approaching zero radians (0º), as we can see in Figure Seven, below (except there would be vastly far more divisors and sectors, way too many to draw!).
All three of the above options would yield the same result -- except that in relation to (3), any two extremely thin contiguous sectors will either completely or partially wipe each other out (along lines suggested by (i), from earlier), or they will overlap one another (along the lines suggested by (ii)), and for the same reason.
Figure Seven: Divide, And Help Conquer Hegel
It might be thought possible to defend Engels's theory (when it is applied to rotating and linearly locomoting 'dialectal objects') by arguing that each of the above examples has been deliberately chosen and described in ways that are prejudicial to DM.
For example, concerning the linear case (from earlier), with respect to moving object, B, while the centre of the leading edge of P3 might move to (X4, Y4, Z4), the distance between (X3, Y3, Z3) and (X4, Y4, Z4) needn't be equal to the distance between (X1, Y1, Z1) and (X2, Y2, Z2), or between (X2, Y2, Z2) and (X3, Y3, Z3). Let us say, therefore, that the distance between (X3, Y3, Z3) and (X4, Y4, Z4) is δe. In that case, B will move forward δe units, as will each of B's parts.
This means that S1 would no longer move to (X2, Y2, Z2), but to some intermediate point, (X1+δx, Y1+δy, Z1+δz), with something similar in relation to the other leading edges. The same would be the case with the trailing edge of S2, which was (let us say) at (Xi, Yi, Zi), at t1. But, to state the obvious, the trailing edge of S2 and the leading edge of S1 can't occupy the same space. In that case, let us also say that the distance between (X1, Y1, Z1) and (Xi, Yi, Zi) can be made as small as we like -- stipulating it is, say, δu (leaving it open whether or not δu > δe). In that case, there would be a gap, δu, between at least two of the parts.
Hence, the trailing edge of S2 would move to (Xi+δx, Yi+δy, Zi+δz) while the leading edge of S1 moves to (X1+δx, Y1+δy, Z1+δz).
[The reader should note that the subscripts i+δx (etc.) and 1+δx (etc.) here aren't the same, which means the points mentioned in the previous sentence are different.]
If so, S1 won't smash into the back of S2, contrary to what was claimed earlier. The same general result can be obtained in connection with rotating discs.
Or, so it could be argued...
Unfortunately, the above pro-DM reply fails. That is because the centre of the leading edge of S1 has to occupy two places at once, if Engels and Hegel are to be believed. So, the centre of the leading edge of S1 has to occupy (X1, Y1, Z1) and (X1+δx, Y1+δy, Z1+δz), and the centre of the trailing edge of S2 has to occupy (Xi, Yi, Zi) and (Xi+δx, Yi+δy, Zi+δz), at the same time. Now, if δu = 0, (X1+δx, Y1+δy, Z1+δz) will lie beyond (Xi, Yi, Zi), which means that the leading edge of S1 will smash into the back of S2. The same will happen if δu < δe. But, if δu > δe, a gap will open up between S1 and S2, which will progressively widen as B continues to move. So, B will either begin to fragment or it will concertina, as it moves. The same will happen to disc, D.24b1
Despite the above, it is possible to show, by other means, that the 'gap problem' (that resulted from the pro-DM objection aired a few paragraphs back) is in the end spurious.
Consider, therefore, a composite body, B, moving with velocity, v.
Again, if such an object is composite, it is assumed to have no gaps between its constituent parts. I will deal with bodies that have gaps or holes in them, presently. So, such a body might be an ingot of metal travelling along a production line, a rock cascading down a mountainside, or the brick mentioned earlier, lobbed at scabs. Admittedly, such bodies are 99.999999% 'empty space', if we are to believe modern science (and that should not be taken to mean I don't believe what scientists tell us!). I am here trying to deal with every conceivable example. In which case I have to consider bodies that might not be like this. As I said, I will return to consider objects that are mostly 'empty space', presently. Anyway, if Spacetime Substantivalism is correct, even 'empty space' isn't 'empty', it is a continuous substance (albeit rather mysterious), which therefore has no gaps! Here is John Norton again (in an article about 'The Hole Argument' -- but the use of the word "hole" here has nothing to do with the use of that word in this part of the Essay, it is connected with Einstein's Field Equations):
"What is space? What is time? Do they exist independently of the things and processes in them? Or is their existence parasitic on those things and processes? Are they like a canvas onto which an artist paints; they exist whether or not the artist paints on them? Or are they akin to parenthood; there is no parenthood until there are parents and children? That is, is there no space and time until there are things with spatial properties and processes with temporal durations?
"These questions have long been debated and continue to be debated. The hole argument arose when these questions were asked in the context of modern spacetime physics, and in particular in the context of Einstein's general theory of relativity. In that context, space and time are fused into a single entity, spacetime, and we inquire into its status. One view is that spacetime is a substance: a thing that exists independently of the processes occurring within it. This is spacetime substantivalism. The hole argument seeks to show that this viewpoint leads to unpalatable conclusions in a large class of spacetime theories. In particular, it seeks to show that spacetime substantivalism leads to a failure of determinism, meaning that a complete specification of the state of the universe at a given time, alongside the laws of nature of the theory under consideration (e.g., the laws of general relativity, which are Einstein's field equations), fails to determine uniquely how the universe will evolve to the future. It also presents a verificationist dilemma, for it appears to lead to the unexpected conclusion that there are facts about the world which we can never know. Although these problems are neither logically contradictory nor refuted by experience, many would nevertheless regard them as being unpalatable." [Norton (2023); bold emphasis and links added.]
So, when objects that are mostly 'empty space' move they either (a) carry this 'substance' along with them or (b) simply move through it. [To some, this theory will now look uncannily like a modern-day version of the old, defunct 'Ether Theory'. I have dealt with that topic in Essay Eleven Part One. I will say no more about it here, so readers are directed to the aforementioned Essay for more details.]
In what follows, I will ignore alternative (b) above since it seems to bear no relevance to the topic in hand. However, if (a) is the case, then this composite 'substance' (i.e., spacetime') will also move. [I have to say, any theory that argues space, or spacetime, can move faces the insuperable problems aired earlier, But, once again, I need to consider every possibility.]
Let us assume, therefore, this 'substance' does move. If so, when any molecule embedded in it moves, it will carry this 'substance' along with it. If that is the case, then any given molecule, even though it is mostly 'empty space', turns out to be completely composite in the above sense of that word -- the 'empty space' inside each one being 'filled' (as it were) by this 'substance'. The same will be the case with the 'empty space' between any two such molecules. In that case, the molecules referred to below should be viewed in that light -- as composite bodies.
With that in mind, consider two contiguous molecules, m1 and m2, in one of the objects mentioned earlier (for instance, that brick lobbed at those scabs, which, for this example, is now B). Assume m1 and m2 inside B are located at two contiguous points, p1 and p2, respectively (in the same line of action in relation to a suitable coordinate system -- which means that m1 and m2 are also moving in the same line of action). According to Engels, both will move such that they occupy two points at once, being in one of them and not in it at the same time, t1. Finally, let p3 be the very next point in line. If so, m1 will be located in p1 and p2 at t1, while m2 will be located in p2 and p3 at t1. Hence, m1 and m2 will both occupy p2 at t1, thus wiping one or both of them out, as the first smacks into the second and occupies exactly the same space. Furthermore, what applies to m1 and m2 will apply to every other molecule in B -- and, indeed, to every particle (i.e., every elementary particle, atom, molecule, cell, etc., etc.) in any moving body (of this type) in the entire universe! Every such particle will crash into and annihilate the one in front as it occupies exactly the same space.
So, if Hegel and Engels are to be believed, every moving body (of this type, anywhere in the universe) will self-destruct!
But, what about bodies with gaps/holes in them?
Consider a non-composite body, B, moving with velocity, v. [If such an object is non-composite, it is assumed to have gaps/holes between some of its constituent parts, a classic example of which would be a lump of Swiss Cheese or a foam pillow. Or, indeed, it could be any 'solid object' whatsoever if Spacetime Substantivalism turns out to be false. In what follows, m1 and m2 are molecules once again, but they could be any two particles in a non-composite body, separated by a gap. The result will be no different.]
Take any two molecules/particles in that body, m1 and m2, but this time separated by a gap of arbitrary width, g1 (otherwise those two molecules/particles [henceforth, m/p] would be contiguous). All three (i.e., these two m/ps and the gap that separates them) are located at three points, p1, p2 and p3 (respectively -- so m1 is at p1, g1 is at p2 and m2 is at p3), in the same line and are moving such that all three occupy two points at once, being in one of them and not in it, at the same time, t1. Finally, let p4 be the very next point. If so, m1 will be located at p1 and p2 at t1, g1 will be located at p2 and p3 at t1, and m2 will be located in p3 and p4 at t1.
[We have to assume the gap moves just like those two molecules, otherwise it will either widen or close. I will consider both possibilities presently. As noted earlier, this gap could even lie between any two elementary particles in B. However, given that QM, or, rather, given that Quantum Field Theory, has now replaced particles with "excitations" in a given field and probability distributions -- which means elementary articles no longer exist (on that, see Essay Seven Part One). Hence, it might prove difficult to explain how such particles can move if they don't exist. {Exactly how an "excitation in a field" can move 'dialectically' I will pass over in silence, except I will leave both of these knotty problems for DM-fans to solve -- after all, this is their theory, not mine!} Anyway, such complications won't change the results about to be obtained. (The reader is left to work out the details of that thankless and pointless task for herself -- no pun intended!)]24b2
[QM = Quantum Mechanics.]
From this we can see that m1 and g1 will both occupy p2 at t1 (hence the gap will either disappear/close, or m1 will vanish having turned into a gap!). In addition, g1 and m2 will both occupy p3 at the same time (so the gap will either disappear/close or m2 will vanish having also turned into a gap!). Hence, all such non-composite bodies will either lose their gaps as they move (in which case, this example simply turns into the one described a few paragraphs back), or their constituent molecules will vanish (as they all turn into gaps)! In that case, once again, if Hegel and Engels are to be believed, every moving body (of this type) will self-destruct!
Let us now assume the gap itself doesn't move just like those two molecules (it does so either faster or slower). In that case, each one will either close or widen (depending on whether the gap moves faster or slower than each molecule). If the former, this example also turns into the one considered several paragraphs ago. If the latter, the gap will widen but the following will still be the case: m1 will be located in p1 and p2 at t1, g1 will be located at p2, p3 and also possibly at p4 at t1 (so it could be in three places at once!), while m2 will be located in p3 and p4 at t1. In that case, not much will have changed; m1 and g1 will both occupy p2 at t1 (hence m1 will vanish having turned into a gap!), and g1 and m2 will both occupy p3 and p4 at the same time (so m2 will vanish, a gap having turned into it!).
But what if each gap is bigger than any given molecule? In that case, just replace m1 and m2 with larger parts or segments of B -- namely, s1 and s2 -- that are the same size as each gap. The above conclusions would then follow.
Either way, if Hegel and Engels are to be believed, every moving body (of the above type(s)) will either self-destruct or vanish!
Clearly, that means this part of DM is still adrift in a Sea of Absurdity.
It is way past time we gave it a decent burial!
Those of us who help run this site are heartily sick of reading it The Last Rites...
Despite this, it could be argued that if the ordinary word "place" is as vague as has been suggested, it should be replaced with more precise concepts -- those defined in terms of SCs, once more. But, as the following argument shows, that would be another backward move (no pun intended!). We begin with a fairly uncontroversial and innocuous looking assumption:
L50: Any place or location can be defined by the use of SCs.
L51: SCs are comprised of ordered real number 3-tuples in R3 (i.e., precisely defined number triples -- see L52). [R3 is just shorthand for 3-dimensional Euclidean/Cartesian space.]24c
L52: However, when written correctly, the elements in such 3-tuples must occupy their assigned places (by the ordering rules). Consider then the following ordered triplet: <x1, y1, z1>. Each element in this SC must be written precisely, with xi, yi, and zi in their correct places.
L53: But, the situating of such elements can't itself be defined by exact SCs, otherwise an infinite regress/vicious circle will ensue.
L54: Consequently, the latter sense of "place" (i.e., that which underlies the ordering rules for SCs) can't be defined (without circularity) by means of SCs.
[SC = Spatial Coordinate.]
This means that a definition of "place" by means of SCs depends on the ordinary meaning of "place" and that sense of "place" must already be (independently) understood if a co-ordinate system is to be set-up correctly, or at all.
Therefore, the ordinary use of the word "place" can't be defined without circularity (or regress) by means of a coordinate system!
In short, the precision introduced by means of SCs is bought at the expense of presupposing (mundane or every day) linguistic protocols such as these. But that shouldn't surprise us given what has been argued elsewhere at this site (for example, throughout Essays Twelve Part One and Thirteen Part Three, as well as here and here).
Of course, this isn't to denigrate or depreciate coordinate geometry, it merely reminds us that any given branch of human knowledge (even one as technical and precise as modern mathematics) has to harmonise with ordinary language and everyday practice at some point if it is to be set-up to begin with, and if human beings (or machines programmed by human beings) are capable of using it. As Wittgenstein pointed out, everyday facts like these are soon forgotten in the course of one's education, since we are taught to ignore, quash or dismiss them early on. As a result we inherit the mythological structures that previous generations have built on top of still earlier layers of such unexamined foundations.
We might even adapt a famous passage from Marx's Eighteenth Brumaire to illustrate this point (no pun intended):
"Mathematicians and scientists construct their theories and systems, but they do not do so as they please; they do not make them under circumstances of their own choosing, but under circumstances already existing, given and transmitted from the past. The tradition of all dead generations of theorists weighs like a nightmare on the brains of living researchers." [Edited misquotation from Marx (1968), p.96.]
On the other hand, if a typographically identical word (i.e., "place") were to be defined in the above way, using SCs, and then put to use in mathematics or physics, it wouldn't be the same term as the ordinary word "place" upon which that definition itself had been predicated. And, should this new term, "place", be used to define the motion of 'dialectical objects', the movement of ordinary objects in the material world would still be unaccounted for.
It could be objected that it is surely possible to disambiguate the ordinary word "place" so that it could be used in a DM-analysis of motion, meaning it was no longer confused with a far less precise term, such as "general location".
Since this has yet to be done (even by DM-advocates, who, up to now, have shown that they aren't even aware of problems like this and are highly likely to ignore them even when they have been pointed out to them -- brushing them off as just so much "pedantry!") it remains to be seen whether such a promissory note is redeemable. However, even if it were, it would still be of little help. As we have seen, and will see again, the word "place" (even as it is used in mathematics) is itself ambiguous, and necessarily so. [There is more on this in Note 25.]
Furthermore, Engels's account requires motion to be depicted by a continuous variable, while one or both of time and place are held to be discrete, otherwise a contradiction wouldn't have ensued (which is, of course, something even Hegel recognised).24d These theoretical dodges were facilitated either by:
(i) The simple expedient of ignoring examples of discrete forms of motion (several of which are given below); or,
(ii) Failing to consider instances where both time and place are continuous; while,
(iii) Imagining that the relevant words drawn from ordinary language (and used to depict both time and location) are being used in their usual meanings, but which haven't been affected by forcing them to assume such bizarre, non-standard roles, or by using them in radically unusual contexts.25
Even assuming a stricter sense of "place" could be cobbled-together, somehow, that would still be of little help. That is because it would either make motion itself impossible -- or, if possible, incomprehensible -- since, given Engels's account, a moving object would have to be everywhere along its trajectory if it is anywhere, and it wouldn't so much move as expand, stretch, concertina or completely vanish (as pointed out earlier).
Ordinary Objects Behave 'Miraculously'
If the points made above are valid (again, no pun intended!), they mean that in a perfectly ordinary sense, bodies can both move and stay in the same place while doing so. Indeed, they are quite capable of remaining stationary even as they are undergoing a radical change of place, moving and not moving, all at once!
The first of the above possibilities was depicted earlier -- with respect to those stationary/mobile workers; the second -- where something can both move and not move all at once (and, in this case, it would involve a discrete sense of "move", too) --, is illustrated by the next example:
L55: NN was second in line when MM, who had been first in the queue, suddenly dropped out. Hence, NN moved to the front of the queue even though he remained rooted to the spot.26
In L55, we have a perfectly ordinary example where a fellow human being manages to do the 'metaphysically impossible' without breaking into a non-dialectical sweat, moving while staying still (relative to some inertial frame). Clearly, it is possible to move to the front of a queue (in one sense of "move") even without moving at all (in another sense of "move"), relative to some inertial frame. This example even satisfies Engels's caveat that such movement is connected with a "change of place" (in this case, their place in a queue).
To compound the problem even further, a queue doesn't have to be composed of a line of people actually standing anywhere, cars waiting to board a ferry, or planes waiting for permission to take-off, etc. For example, a queue could consist of a list of the names of patients due for an operation, or a group of people waiting for a call centre operator to answer their calls. Clearly, in such circumstances, there could be instances of movement (in one sense) even where no movement (in another sense) has occurred; that is, if one or more in each queue dropped out and others were moved up the list while 'not moving' anywhere (in another sense of "move").
In such cases, 'queue jumping' could also occur when the proposed interloper was neither on the list nor was located anywhere near anyone on the list (there being no list in this case, just an electronic queue). Here, those in the queue would move (up or down the list, forward or backward in the queue) while remaining stationary, as, indeed, would any queue jumpers.
This illustrates how, when background details surrounding the use of certain words are either filled in or are altered sufficiently, word connotations (and that of any other terms associated with them) will change accordingly (and, indeed, vice versa), something Engels and the vast majority of philosophers appear not to have noticed. Or, they simply ignored them since such scenarios were far too ordinary and mundane, or they weren't 'philosophical' enough!
[This "change of meaning" refers to what we would say and how we would interpret any of the words used in such situations. (I merely add this comment in order to forestall complaints that this contradicts what was said about 'contextualism' in Essay Thirteen Part Three.)]
However, in their everyday use of language, DM-theorists and Traditional Philosophers aren't quite as semantically-challenged as they appear to be when they try to 'philosophise'. Indeed, they only become 'Linguistic Philistines', as it were, when they attempt to con their readers with another dose of a priori Super-Science (i.e., Metaphysics). They thus end up employing words as if they were complete novices, were using a foreign language for the very first time, or had some sort of 'learning difficulty'. Hence, it seems to be the case that linguistic crudity/naivety (real or feigned) is the price one has to pay for the low grade skills required by anyone who wants to discover 'philosophical truths' solely from language or thought. In fact, this is the first hurdle philosophical novitiates find they have to negotiate successfully if they, too, want to join the ranks of such linguistic barbarians.
~~~~~~oOo~~~~~~
Interlude Four: Further Examples Of Movement Where There Is No Movement
Update 16/05/2011: I recently watched a film called Poodle Springs. Among other things, it was about a mythical town (and an unfinished detective novel by Raymond Chandler) situated somewhere on the California/Nevada border. Near the end of the film one of the characters revealed a sinister plot to have the border moved a few miles to the west so that Poodle Springs would then be in Nevada, not California. Of course, had that border been relocated (apparently it wasn't in the end), Poodle Springs would have moved from California to Nevada while not having moved at all.
This is yet another perfectly ordinary sense of "move" that Traditional Metaphysicians studiously ignore. Indeed, for centuries, whenever borders between countries were relocated (often after war) many hundreds, and possibly thousands of square miles of territory are moved from one country to another, while remaining perfectly motionless (relative to some inertial frame), along with countless citizens, towns, farms, buildings, roads, fields, mountains, valleys, lakes, plants, rocks and trees. [Border changes since WW1, for example, have been listed here.]
Another relevant case springs to mind: In 1974, the British Government re-organised the UK County Boundaries. Overnight, millions of Brits found they had moved counties while not having moved an inch! Regulatory agencies in many countries often revise the electoral maps of their country or state. When that happens hundreds of thousands of voters are moved (in one sense of that word) from one constituency/precinct/ward to another, many without moving (in another sense of the same word) an inch, with respect to some inertial frame. Here are several examples of just such boundary changes in the UK (for 2023). Changes like this are commonplace. Think of what happens when one capitalist company takes over another and all the employees of the second firm are legally moved from the list of those employed by the second firm to the list of those employed by of the first. Think about the many splits that occur in politics (and not just in relation to revolutionary parties/tendencies). If Party, A, splits into Parties, B and C, and they share their members between them (with 10,000 going to B and 15,000 to C), we would have 25,000 members being moved out of A and into B or C, even if that were to take place while they were all tucked up in bed, fast asleep.
Once again: here we have movement (in one sense) while there is no movement (in another sense).
The question is: did any of these significant 'movements' of voters, residents, employees, members, land and real estate mean that any were "both in one place and in another place at one and the same moment of time...in one and the same place and also not in it"? But, how could they possibly be described that way if they moved and didn't move? All that happened (in many cases) was that new lines were drawn on a map somewhere, a few documents/contracts were signed, a few signposts, fences or border checkpoints were altered, removed or relocated, etc. Sure, some of those items certainly moved, but nothing else did, even while everything else did!
Is any of this a 'contradiction'?
Surely only to those who are half asleep -- or, maybe, those off their heads on something a little too potent!
Update 09/03/2014: We now read this from the BBC:
"Kiruna: How to move a town two miles east
"This spring work will begin to move Sweden's northernmost town two miles to the east. Over the next 20 years, 20,000 people will move into new homes, built around a new town centre, as a mine gradually swallows the old community. It's a vast and hugely complicated undertaking. 'When people hear that we're designing, creating and building a whole new city from scratch they think we're doing a utopian experiment,' says architect Mikael Stenqvist. But there's too much at stake to think of it as an experiment, he says....
"More than 3,000 apartment blocks and houses, several hotels and 2.2m sq ft (0.2m sq m) of office, school and hospital space will be emptied over the next two decades -- while alternatives are built on the new site. The old church voted Sweden's most beautiful building in 2001 will be taken apart, piece by piece, and rebuilt. 'We want to have as much of the existing character from the old city as possible, but costs and market mechanics mean we can't move everything,' says Stenqvist. The move has been dictated by the local iron mine -- one of the most valuable iron ore deposits in the whole of Sweden, and Kiruna's largest employer. The story began in 2004, when the state-owned mining company, Luossavaara-Kiirunavaara AB (LKAB), sent a letter to the local government explaining that it needed to dig deeper into a hill just outside the town, which could cause the ground beneath thousands of apartments and public buildings to crack or give way. A decade later, sure enough, huge fissures are appearing across the city, creeping towards the centre.
"'Everyone that lives in Kiruna has known that the city will eventually be relocated -- everyone can see the mines eating up the city,' says Viktoria Walldin, one of the social anthropologists hired to work on the relocation. 'The question has always been when.'... Before anyone can move, LKAB has to buy their existing property, so that they can buy a new one in the new town. But the sums are nightmarish. 'The general idea is for LKAB to purchase people's homes from them at market value plus 25%, and then sell them a property in the new city,' says Stenqvist. 'But how do you work out what the market value is for a house in a city that doesn't even exist?'.... 'It's a new situation and no-one really knows how to handle it,' says Yvel Sievertsson, urban transformation officer at LKAB. 'We have hundreds of people working on the issue alone, including researchers at the University of Stockholm. The goal is to have the new city centre ready before we start to move everyone over, and then to move everyone at once in one or two stages, to impact people's businesses as little as possible.'
"'We have been around the world looking at how other countries like Germany and parts of Africa have handled similar projects, but they are just moving small villages and houses, not huge city centres,' says Sievertsson. 'We're using all the expertise we can to help us, but it's a completely unknown situation.'..." [Quoted from here. Accessed 09/03/2014. Quotation marks altered to conform with the conventions adopted at this site. Several paragraphs merged. Link and bold emphases added. This move will apparently take another eighty or ninety years to complete!]
Hence, Kiruna is and isn't being moved. It is being moved in the sense that it will reappear in a new location two miles to the east of where it is now, but it isn't being moved in the sense that the vast majority of the buildings will remain where they are (or they will probably be demolished). What is being moved are the residents --, and, even then, they are being moved in a piecemeal fashion. This means that they will be in countless places at once, in and not in any of them -- since a population like this is an extended aggregate, not a single body --, even when it isn't being moved, let alone when it is.
Furthermore, while the population will be in Kiruna, it won't be in any one place in Kiruna -- that is, not unless all 20,000 of them are squeezed into a cupboard in someone's house! But even then, unless they are all compressed into the same geometric point, they still won't be in the same place, in one sense of "place", even while in another sense of "place", they will be (i.e., they will be in the same town/country/solar-system/galaxy...). So, they will be in and they won't be in Kiruna -- and that would be the case even if they were all either rooted to the spot or engaged in a collective fun run! Hence, this is yet another spurious 'contradiction' that has nothing to do with motion, even though it seems so!
The question is: Is this town going to be in two places at once, in one spot and not in it at the same time, as it moves and doesn't move? Hardly, since the town will be in two places for many years (i.e., while the residents were being relocated piecemeal), and yet it won't be both in one place and not in it at the same time. Nevertheless, when the move has been completed, the town will still have been moved. However, during the move, there will plainly be two towns, both called by the same name; that means the original town, Kiruna, won't in fact be in two places at once (in one sense of "place"), there will be two towns in two places, both with the same name at the same time!
Even more annoying for Hegel-groupies, there will be two towns with the same name in one place --, i.e., in Sweden! -- even while there will be two towns with the same name in numerous places -- i.e., Sweden, Scandinavia, Europe, the Western Hemisphere, the Earth, the Solar System...
Just try saying any of that in Hegel-speak!
Moreover, the old church (mentioned above) will be taken apart and moved piece-by-piece to the new site. While its dismantled parts will certainly move, the church itself will cease to exist between locations (if by "church" we mean the assembled article, not its disassembled parts). So, while this church moves, it, too, won't occupy two places at once, in one of them and not in it, since there will be no church in existence between these locations for it to do any such thing. This is, of course, yet another example of discontinuous motion -- and, indeed, discontinuous existence.
Discontinuous movement like this is quite commonplace; it often happens when people move home, or when a company moves offices, or an army moves camp, for instance.
Furthermore, the above point about 'non-existence' applies to countless things that are disassembled and then moved, or which are transported in another form while that is happening. For example, consider someone moving from one address to another. Let us suppose they dismantle several items of furniture (e.g., a bed, a few bookcases, a wardrobe, several tables, etc., etc.), move them across town and re-assemble them at their new location. Since each of these items ceases to exist during the move (while their parts certainly continue to exist), they will still have moved even though they haven't been in two places at once, in one and not in the other.
Suppose, further, you are reading a book displayed on an e-reader. This book will have been transmitted, or transferred, to that reader in a form different from the one in which it was originally written or published (especially if the book in question had been committed to paper many years ago). But, while it is being transmitted (etc.) to that e-reader it is no longer a book, it is a series of electronic signals. Now, even though the book itself (or a copy of it) has certainly been moved, it manifestly wasn't in two places at once, in one of them and not in it at the same time. That is because it ceased to exist as a book between locations. Plainly, this applies to countless analogous examples of movement -- for instance, the transmission of TV programmes, text messages, phone conversations (or, indeed, any conversation whatsoever, since they are all transmitted through the air, or via some other medium, and any words spoken cease to exist while 'they' are being carried as pressure waves through that medium), coded messages and emails.
Well, we needn't labour the point (no pun intended); we are all familiar with moves like these (where there is no 'movement', or it is discontinuous) -- but, that seems to be the case only when we aren't trying to do a little 'philosophising', at which point we somehow become word-blind, linguistic imbeciles.
[No pun intended, again!]
~~~~~~oOo~~~~~~
Naturally, it could be argued that the above examples have been intentionally mis-described (or each has been deliberately slanted) so that they seem to violate Engels's description of motion. For instance, while it is true that a text message (of the sort mentioned above) is transmitted in a different form to the one it had while it was being typed, it still exists in an electronic state between locations. In fact, it always is, and remains, in electronic form, even as it is being written. And the electronic signals themselves certainly conform to Engels's description of motion.
Or, so it might be argued...
The first part of the above counter-point might be correct (e.g., the fact that a text message exists in an electronic form between locations -- but we have already seen there appear to be insuperable problems facing anyone who tries to re-configure this type of motion in 'dialectical' terms), but the message on the sender's screen -- the actual letters and/or pixels -- don't exist while it/they are being transmitted, so the remarks made above still stand.
Of course, the second part (about Engels's description of motion being correct) has been put under sustained pressure throughout this Essay, so a belated appeal to it would be ill-advised until and unless the criticisms aired here have been resolved.
And I am not holding my breath on that score. Long experience has taught me that DM-fans blithely ignore anything they can't answer or prefer not to have to face. A bit like other mystics.
How odd...
Notice, once again, that as soon as we understand the surrounding circumstances relevant to each case, apparent 'contradictions' simply vanish.
Yet More 'Anti-Dialectical' Scenarios Hegel And Engels Blithely Ignored
Indeed, it is possible to think of further cases of discontinuous (i.e., discrete) motion where, even though something once moved, nothing need now be moving -- and yet in one sense something still moves. This would also involve whatever it was that managed to do all this 'moving while not moving, at the same time' doing so in a different sense to L55 (repeated below). In fact, it is possible to show that some things can move (again, in a discrete sense of that word) while they occupy none of the intervening places between successive locations. All of these are illustrated below:
L56: The footprints moved across the snow-covered yard, indicating where the scabs were hiding.
L57: Easter moves to a new date each year. [Same with Ramadan.]
L58: "See, the page numbers in the book you sold me move about erratically. It has been printed and bound all wrong!"
L59: The ground staff moved the cricket pitch to the other side of the square.
L60: The organisers of the rally have moved the meeting to seven o'clock tomorrow evening.
L61: The strobe light moved across the floor picking out each dancer, one at a time.
[L55: NN was second in line when MM, who had been first in the queue, suddenly dropped out. Hence, NN moved to the front of the queue even though he remained rooted to the spot.]
In L56, we have stationary 'objects' (i.e., footprints created by individuals who had earlier walked across the said yard), which still move (across that yard) even while each item (each footprint) is stationary. We might try to substitute other words for "move", here, such as "stretch", but in such circumstances they would merely be synonyms for "move", which means this example still poses problems for traditional theories of motion, like the once Hegel and Engels tried to sell us.
In L57, nothing actually moves even while it still does! In that case, does Easter occupy two locations at once, being in one of them and not in it at the same time? Is that even true of Easter's date (entered perhaps on a calendar, maybe across successive years as each new date is pencilled in)?
In L58, nothing moves (once again) even though something clearly does move (namely the faulty numbering), and it does so discretely while not occupying any of the intervening spaces -- which spaces don't exist either for anything to move into!
Of course, in such circumstances, we would probably use "jump about" instead of "move about". But, to jump is also to move.
A similar picture emerges in L59, where a discrete object (a cricket pitch) was moved a certain distance (between, say, twenty and thirty metres = sixty to ninety feet), but which object (the cricket pitch) doesn't exist while it is being moved, nor does it occupy any of the intervening spaces on its 'journey' -- but which intervening spaces do exist! The same outcome results if someone moves a vegetable patch from the side of the house to the back, or someone else deletes their name from one part of an on-screen form and re-types it further down; or, indeed, if someone "moves the goal posts". There are countless examples of moves like these where nothing actually moves, even while 'it does', and whatever it is that 'moves and does not move' doesn't occupy two places at the same time, being in one of them and not in it at the same time. Similar situations are illustrated by L60 and L61.
Not only that, but continuously discrete -- and yet stationary -- objects can move while remaining still:
L62: As I look down on the scene, an immobile line of pickets moves out of sight, curling right round the block; each worker holding her ground, rooted to the spot.
L63: The wire moves in a spiral around that tree over there. It's been in the same spot so long the tree has partially grown over it.27
Finally, some things can move -- but to nowhere in particular -- and they can remain quite still while they are doing it:
L64: This road is going nowhere.28
Several other examples of motion (that aren't easy to force into Engels's 'dialectical straightjacket') include the following:
(1) Imagine a situation where the sun is shining intermittently through the clouds, sometimes casting shadows, sometimes not. In such circumstances, someone could say:
M1: "An hour ago, the shadow of that telegraph pole was over there, now it's moved over here."
In this case, although it would be correct to say that the shadow had moved, its episodic existence -- when the Sun disappears behind the clouds only to reappear several minutes later -- means that there will be no continuity between each successive location of this shadow. Here, we would have something that moved, which had been in at least two (possibly) widely separated locations, but which hadn't been in any of the intermediate points between them, and which had ceased to exist during that time. In this instance, therefore, we would have something that moved that did not move!
It could be objected that since a shadow isn't a moving object it isn't a counter-example to Engels's claims about motion. However, according to Lenin an object only has to be external to the mind for it to be material, and shadows certainly qualify on that score.
"[T]he sole 'property' of matter with whose recognition philosophical materialism is bound up is the property of being an objective reality, of existing outside our mind." [Lenin (1972), p.311. Italic emphasis in the original.]
"Thus…the concept of matter…epistemologically implies nothing but objective reality existing independently of the human mind and reflected by it." [Ibid., p.312. Italic emphasis in the original.]
Hence, even intermittently existing shadows count as (discretely) moving material objects (in Lenin's sense).
But, even if they weren't objects (even though Engels never actually told his readers what he counted as an object in such circumstances), shadows certainly move, which means his comments about motion must apply to them, too. In fact, Engels actually spoke about "things" moving:
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them.... But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphases added.]
So, even though Engels spoke about "a body" in motion he also referred to "things in their motion", and a shadow is certainly a "thing".
Anyway, it seems we may only rescue Engels by contradicting Lenin -- or, vice versa. Of course, if shadows aren't to be counted as material 'things', then motion and matter aren't linked in the way Lenin and Engels imagined they were. [On that, see Essay Thirteen Part One as well as Point (2), below.]
But then again, maybe not, since this is what he actually said:
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Ibid. Bold emphases added.]
Hence, it is "motion itself" -- not so much moving bodies -- that interested Engels, and, once more, shadows certainly move.
(2) Consider a example where, say, a woman is consulting the plans for her new house, but upon being shown the latest drawings produced by the architect exclaims:
M2: "Wait a minute, you've moved the back door. We agreed it should go here next to the window, but you've put it over there near the sink!"
Here we would have an intentional object (a planned door) that had been 'moved' to a new location, represented perhaps by pencil or ink marks on a sketch or diagram. Furthermore, since the original intentional object doesn't yet (actually) exist (after all, in this case, there is as yet no house and so no door, merely marks on sheets of paper!), this would be a clear case of movement that doesn't involve a material object --, but which 'object' can't have occupied two places at once (in one sense of "place", etc.), nor, indeed, any in between.
Naturally, this means that motion isn't the sole property of matter; again, contrary to what Engels and Lenin believed:
"Motion is the mode of existence of matter. Never anywhere has there been matter without motion, nor can there be…. Matter without motion is just as inconceivable as motion without matter. Motion is therefore as uncreatable and indestructible as matter itself; as the older philosophy (Descartes) expressed it, the quantity of motion existing in the world is always the same. Motion therefore cannot be created; it can only be transmitted…." [Engels (1976), p.74. Bold emphasis alone added. For Lenin's comments, see Essay Twelve Part One, where I have given several other examples of non-material 'things' that move.]
If intentional objects can also move -- and if 'mind' isn't matter (but the supposed product of it) -- then not everything that moves is material.
Be this as it may, and it turns out that intentional objects are material, even if an actual drawing of a door had been moved (as opposed to an intentional object having done so), it still needn't have occupied two places at one and the same time since there might not even be two places for it to occupy.
There are a host of possibilities here: The door could now be in the 'same place' (i.e., right by the window), but in a different room (hence, it would be in one and the same place, but which place had now moved -- while the object itself hadn't (in another sense of "move") --, if the same 'intentional window' had also been re-located in a new room. So, the door would still be next to the window in this new room -- and hence in the same place relative to another intentional object (the door), but in a different place relative to a third (the new room); or even in the 'same place' in a different building; or in the 'same place' with new surroundings (e.g., the walls could have been altered); or the county boundaries (from earlier) were changed, so this intentional house (along with its intentional windows) would now be in a new county(!), and so on...
Moreover, even if the drawing of the original door had been erased and re-drawn elsewhere, while it would still be correct to say that something had moved, it wouldn't be true to say that whatever it was that had moved had been in these two places at the same time, nor even that it had occupied any intervening locations. Of course, no one imagines that in such circumstances a drawing of a door actually slides across the page -- even though in some cases it could do just that, if, say, this were part of a computer-aided design (maybe using a site like this). But even then, that would be an example of simulated movement (i.e., it would be represented by groups of pixels successively lit up or darkened on a screen), which is similar to the strobe light example mentioned earlier. And that, too, would be yet another example of discontinuous movement.
Again, just try saying any of that in Hegel-speak!
In ordinary language, of course, it's a doddle...
Here is another (relatively familiar) example of discontinuous motion:
"Wildflowers moving north as climate changes, citizen survey shows
"Wildflowers are moving northwards as temperatures rise, prompting calls to manage landscapes to make space for plants in the face of climate change. Results from the first five years of the government-funded National Plant Monitoring Scheme, using data from 15,000 surveys by volunteer citizen scientists, already shows the impact of a warming world on the UK's plants. The National Plant Monitoring Scheme looks at 30 different habitats, from woodland and hedgerows to blanket bogs and streams, with around 30 wild flowers to search for in each type of place. Data is collected by volunteers, co-ordinated by wildlife charity Plantlife and analysed by botanists from a range of organisations led by the UK Centre for Ecology and Hydrology (CEH). Southern marsh-orchids, a tall plant found in damp grasslands, was once restricted to the southern half of the UK but records have come in from as far north as Newcastle-upon-Tyne. Bee orchids were not previously found in Scotland, but volunteers have discovered the plants, whose flowers resemble a bee's backside, at several sites around Glasgow and Edinburgh.
"Other specialist plants are moving outside their usual range, including mossy stonecrop, a succulent once only found in the New Forest and East Anglia, which is spreading to sandy habitats in Scotland. Early meadow-grass was formerly only found on the Lizard Peninsula in the extreme South West of England but has now been recorded in Fishguard, south-west Wales, as well as Rosslare in Ireland, and central London. There are also concerns about the threat and extinction risk to plants which have no further to go, for example Arctic and alpine species which cannot go further up the mountains, such as Highland saxifrage. And the increased risk of drought due to climate change puts many smaller, short-lived species at risk, with fairy flax, yellow-wort, soft brome and common mouse-ear suffering from heat and lack of water in 2018's drought. But the results from the monitoring scheme also show a rise in species able to cope with drought.
"These include salad burnet, a dark crimson flower found in old hay meadows, which has a longer root so it can reach down to moist soil, and wild thyme, which manages water loss with its tiny leaves. The analysis also reveals the impact of nitrogen pollution from fertilisers, with nitrogen-hungry stinging nettles the most frequently recorded native species in woodlands. Dr Trevor Dines, Plantlife's botanical specialist, said experts had previously thought that it would 'take an awful lot' for plants to start moving northwards because their dispersal is very slow. 'To actually start seeing that now, coming through so strongly, is a real wake-up call,' he said. 'It proves to us that climate change is having a real impact.'
"And he said: 'Our concern is that we live in such a fragmented landscape, there aren't the places for these plants to go.' Growing chances of drought, particularly in the crucial spring months of April, May and June, is even more of a risk than general warmer conditions, he warned. 'Any climate change that involves drought scenarios is going to affect plant populations much more quickly.' He said tackling climate change is about making the landscape as permeable as possible so things can move around, by creating habitat where flowers can bloom and set as much seed as possible, through grazing animals and hay cutting at the right time of year. Road verges, which are corridors through the landscape, should not be subject to repeated mowing, while moving livestock, machinery and distributing wildflower-rich hay can all move seed around the landscape, he said. And while rewilding can play a part on a small scale, Dr Dines said agri-environment schemes could be used to get habitat management in place to suit wildflowers on as wide a scale as possible." [Quoted from here; accessed 25/03/2020. Several paragraphs merged; links and bold emphases added. Quotation marks altered to conform with the conventions adopted at this site.]
Moving plants!? Surely not! Are they perhaps Triffids? Maybe they are Euglena, then? No, neither. But move they certainly do. And they do so in many different ways. One way is in the discontinuous manner described above. Plant seeds are spread by the wind, by water, by animals and by human beings. But while the above flowers don't themselves move, they still move as their seeds are propagated. So, they move in the sense that the plant colonises a new area or patch of ground. But, while they move, no single plant is in two places at once, "here and not here". This is a perfectly ordinary, and, indeed, scientific, sense of "move" that neither Engels nor subsequent DM-fans even so much as mention.
It could be objected that the atoms that make up the seeds involved certainly move in a continuous manner, so the above remarks are misleading.
Maybe so, but the plants that grow in these new locations are composed of atoms that didn't move with those seeds, since they were absorbed from their new surroundings as they grew. Let any such seed (initially, whenever the DM-clock is set to start) be composed of N atoms; the mature plant that grows from it will be composed of K atoms (where K is very much greater than N). Let us further suppose that M atoms were absorbed from the air (and they certainly moved in Engels's sense, if we pretend for a moment we understand what he meant!), and where K > M, too. In that case K-(N+M) atoms won't have moved with that seed (or moved to it in the air, either). In that case, the above remarks aren't misleading. Not every atom involved actually moved from the old location to the new, but the plant concerned did in the end move there, discontinuously.
And what applies to flowers, also applies to trees and any other organism that reproduces itself in like manner -- including bacteria and fungi (via spores) -- or in other ways. So, while an egg might be laid by a fish in a river and could be swept downstream, the fish that hatches out of that egg hasn't moved downstream, even though it has moved! Hence, it has and hasn't moved! This is yet another 'contradiction' which results from the ambiguous meaning of "move" and "fish". Hence, animals that lay eggs also propagate in a discontinuous manner, just like plants. And that includes mammals and human beings, too. Before a baby is born, or even conceived, its mother might move from one part of the country to another over the space of two years -- from, say, Seattle to New York via St Louis. Assume this mother has a partner and two children when she left Seattle and conceives while the family lives temporarily in St Louis. Assume also that the new baby is born after they settle in New York. It would be perfectly normal to say this family moved from Seattle to New York, but the discontinuous nature of that family means it has and hasn't moved. The family, as it existed in Seattle, isn't the same as the family that arrived and now lives in New York; it has one more member. Since this new arrival (call her "NN") is now part of the family, she both moved and didn't move from Seattle to New York. Later in life, NN could say with complete honesty and justification "My family moved from Seattle to New York, but I didn't!" And she could also say with equal honesty and justification "My family moved from Seattle to New York and so did I!" Or, she could even say "My family and I didn't move from Seattle to New York, since I didn't exist until after we arrived in that city!".
No doubt others can think of permutations of the above examples that might illustrate even more complex examples of motion that are and aren't instances of movement.
Once more: nature and society are far too complex to fit the dialectical boot into which Hegel and Engels tried to force it.
(3) Furthermore, some things can move while not changing place, even as they do just that! Another contradiction?
Consider these examples:
(i) A line of soldiers marching along a road in strict order;
(ii) The numbers on the face of a watch (on someone's wrist) on a moving train;
(iii) The words in a book on that same train; or,
(iv) The numbers on the screen of a calculator (or the letters on a computer screen) as they are being typed or entered, etc.
In each case, several things remain in the same place (i.e., they stay in the same order relative to one another: first, second or third (etc.) in line), even while they are moving (i.e., they are changing their location, in another sense, relative to something else).
Hence, in the first example above, soldier MN could be second in line (and could stay second) as his squad marched down the road -- and he could remain second even if the distance between each soldier alters continuously. In that case, MN would remain in the same place (i.e., second) even as he and his comrades marched along a road.
The same is the case with numbers entered into a calculator (or letters on a computer screen) on that train (in examples (ii) (iii) and (iv)). In relation to (iv), consider the number π = 3.1415926535...; as the calculator screen hurtles along at 100 mph relative to the passing countryside, the "3" at the front still remains in the same place, at the front, as does the "3" in tenth place!
[It is also worth recalling that numerals are unambiguously material objects, so this isn't an 'abstract' example.]
Again, (in relation to (iii)) the words in a book certainly remain where they are while travelling at 100 mph; train journeys do not normally scramble the printed page! So such words, too, can stay in the same location, and remain in the same order, while they barrel along. When was the last time your socialist newspaper became unreadable while you were sat on a bus?
Admittedly, several of the above examples depend on the use of figurative (or in some cases slightly stilted) English, but Engels's own use of language in this respect is even more non-standard, and it is hardly less figurative. Nevertheless, I have generally, but not completely, ignored overtly figurative uses of "move" and its cognates -- such as a committee moving a composite motion, or a passage of music moving listeners to tears, etc. --, in the examples I given in this Essay. The same is the case with respect to the figurative use of other rather familiar verbs -- for example, a highly motivated individual can run a protest movement, someone else can climb the 'greasy pole' in a capitalist organisation, two individuals can fall in love, the conduct of the police can quickly descend into violence (etc., etc.) --, whether or not any of these are in fact dead metaphors.
Anyway, the rather odd sentence constructions I have employed in several of the examples aired in the main body of this Essay (and above) were a direct consequence of the fact that I had to limit myself to using the word "move" (and its cognates) to make each point seem relevant. Less stilted versions could easily have been devised if a wider selection had been chosen of the many words we have in ordinary language for the depiction of motion and change. That would, of course, allow the same points to be made in less stylistically-challenged ways. Indeed, the last example listed in the main body of this Essay (L64) did precisely that:
L64: This road is going nowhere.
If a more intelligent use were made of the countless words we have in English connected with how we speak about motion and change (and I am sure the same is the case in other languages -- it certainly is in French, one language I speak reasonably well, as it is in German and Spanish, two languages spoken by close relatives), the number of 'contradictions' language appears to generate would multiply alarmingly -- but only for those who insist on using or comprehending the vernacular as if they were linguistic philistines or were on a day trip from one of the moons of Jupiter.
Consider the next series of examples (although it is arguable that some of them use dead metaphors):
(4) Coal seams run through mountains (and they remain stationary even while they do it) just as roads run through tunnels, mountains and cliff faces. Messages can run through sticks of rock just as they can also travel along a line of stationary messengers who pass them to one another by word of mouth (and a stationary message can run along a line of bill boards, with one or more words on each board).
Figure Eight: Blackpool Rock -- Messages That Run Through Rock
Even While Remaining Perfectly Still
Figure Nine: One Stationary Message Running Along Three Motionless Billboards
[From The Film -- Three Billboards Outside Ebbing, Missouri.]
Indeed, paths can climb hills (without moving) just as easily as tracks can ascend them, too. Stairs, lifts, escalators and steps invariably connect floors in hotels, offices, schools, theatres, malls, flats, houses and sports stadia. Furthermore, parties can fall in the polls, a candidate can enter an election campaign without moving anywhere (just by signing a document), and pop records can rise in the charts.
If we think about the last sort of example (of discrete motion), a record rising in the charts (say, from tenth to fifth), or even a team rising in a League Table (from fourth to first) -- is it really the case that they occupy all the places inbetween those two locations at the same time? Does a record that rises from tenth to fifth really occupy fifth and tenth place at the same time, or even all the positions inbetween? Does a rising soccer team occupy fourth and first at the same moment? Does it also have to be in third and second place at the same time? [In such circumstances, of course, a name just disappears from a list to be re-written a second or so later higher up.] If not, these are perfectly ordinary and well understood instances of discrete movement that don't do what Hegel and Engels tell us must be true about every example of motion in the entire universe.
To which one can add a consideration of the sort of lists that different individuals/organisations often compile (especially these days on the Internet) -- for example, the Top One Hundred Films of all time, the Best Fifty Guitarists, the Twenty Greatest Novels You Must Read, or the Ten Most Amazing Places You Must Visit, or even a Bucket List Of Things To Do Before You Die, etc., etc.
So, for example, The Shawshank Redemption might be fourth in NN's and seventh in NM's list of the Top One Hundred Films. Would anyone find this comment (by NN) at all weird or unacceptable? "Hey, NM, you've moved The Shawshank Redemption down to seventh in your list! Why?" We're all familiar with such lists and how things can move about in any of them, or can even vary between lists in the above manner, none of which changes pay any attention to the confused ramblings of mystical Idealists like Zeno and Hegel, or Historical Materialists like Engels and Lenin -- who should know better!
There are plenty more examples of different types of movement like these: perfectly motionless wiring can wind effortlessly about inside TV sets, radios, computers and old phones; fences cemented into the ground can descend into valleys, surround farmsteads, disappear over the horizon and encircle fields. String can coil round a parcel, and perfectly still moats can encircle castles; buildings can rise above one another just as cliffs tower over intrepid climbers. Bandages can cover heads and towels can wrap around bodies; stories can switch to new locations even as they remain in books that have been left to gather dust on the same shelves for decades. Tram lines can cut through city centres just as panic can sweep through a crowd, and behaviour patterns can propagate through human and animal populations. Or, indeed, feelings of anger and resentment can spread through a group of irate workers sat motionless in a canteen as management reveals its latest 'fair offer'.
And lists like this are extendable...
Once again, try saying any of that in Hegel-speak!
Even falling dominoes move is a discrete/continuous manner, when it isn't easy to say what moves in such cases (is it energy?). In the following video something is clearly moving along these columns of dominoes, but exactly what that is, is difficult to say:
Of Video Two: What Exactly Moves Along These
Columns Of Falling Dominoes?
Further, we read the following in a recent book about the spread (and even the decay) of knowledge, in this case concerning events surrounding an assassination attempt on George Wallace in 1972:
"On that same day -- May 15, 1972 -- a group of telephone interviewers happened to be undergoing preparation for that day's assignment at the Consumer Research Corporation, a small market research firm. When David Schwartz, the firm's owner, heard the news of the shooting, he realised this was a rare opportunity: They could use the assassination attempt to actually measure how long it takes for important news to travel and spread through a population. He re-directed some of the phone-bank interviewers to examine this, and his team began dialling individuals in the New York City area, attempting to see how the news spread each hour. They carefully called hundreds of people over the course of several hours, and in doing so extracted a clear mathematical curve of how news diffuses over time. Each hour, a larger and larger fraction of those surveyed had heard the news of the shooting. By 10:00 P.M. that night, nearly everyone they spoke with had already heard the news, through a combination of radio, television, and personal contacts. This important piece of information spread extremely rapidly but not instantaneously. The news flashed around New York City in a measurable and predictable way." [Arbesman (2013), pp.66-67. Bold emphases added.]
This is clearly a legitimate use of the verb "to move", but it is difficult to see how it can be shoe-horned into Engels's highly restrictive and radically confused account of movement/locomotion.
To be sure, the "mathematical curve" alluded to above will be smooth, but that graph will have been drawn through discrete, plotted points; the curve itself will be an interpolation (and possibly even an extrapolation) of the data set collected. But, whatever the curve amounted to, no one (it is to be hoped!) imagines that during this series of events discrete packets (or pockets) of news were in two places at once, being in one place and not in it at the same time.
To that end, imagine there is a house, H(1), on ABC Street, Brooklyn, New York, and another house, H(2), on the same street and next door to H(1). Let us further suppose that (i) the above news reaches H(1) at, say, 07:01pm on the day of the shooting (perhaps the occupants saw it on TV), and that (ii) the news reaches H(2) at 07:33pm the same evening (perhaps the occupants received a phone call from a relative). Now while some form of electronic energy flowing into each house conveyed this information, none at all will have passed between these two houses carrying that message. So, anyone plotting a graph of how the news spread along this street will have observed it (seemingly) spread from H(1) to H(2) in a discrete manner, being registered at the former dwelling a good thirty-two minutes before it made it to the second. Although the news spread along this street, there is no way it actually passed from H(1) to H(2), even though it seemed to have done so. Any graph of the dissemination of this story across this neighbourhood would show that it nevertheless did spread along the street from H(1) to H(2), although nothing (relevant) passed between these two houses. In that case, if concentric circles were drawn on a map of this part of New York, they would presumably show a 'ripple effect' as the news fanned out across the city, but, concerning any two points in the city, (H(1) and H(2), for instance), nothing will necessarily have travelled between them. As we saw, nothing did pass between H(1) and H(2). So, even though the news moved through the city, nothing might actually have moved in one sense of "move", even while something did move in another sense of the same word.
Again, just try saying any of that using only Dialectical Gobbledygook!
But it is laughably easy in the vernacular, as I have just shown
Ordinary language is a truly amazing resource.
There are many other possibilities in addition to those illustrated above. Ordinary language and our common understanding allow for countless alternatives undreamt of in the philosophical ramblings of Idealist bumblers like Hegel and Zeno -- and that comment unfortunately also applies to the ill-advised and incautious ruminations of revolutionaries like Engels, Plekhanov and Lenin.
Such mundane examples (using perfectly ordinary words, in circumstances we all readily recognise and comprehend) demonstrate that the seemingly 'obvious' metaphysical principles that thinkers like Hegel and Engels dreamt-up (or tried to milk from instances like these) actually depend on non-standard applications (i.e., they are based on a distortion) of the vernacular (indeed, as Marx pointed out); they also run against common understanding, into the bargain.
So, what might at first sight seem to be decidedly odd situations (like those above) now turn out to be nothing more than a rather mundane set of circumstances with which we are all cognisant and which would hardly even raise an eyebrow in everyday life. When was the last time any of my current readers (even if they're atheists or non-Christian) were completely puzzled by the date of Easter being moved every year, or were totally bemused by a meeting that had been moved to a new venue or to a different time? Is anyone mystified by the fact that information can spread in the way described above? And who in their left mind has ever scratched their head wondering how plants or shadows are capable of moving (in the manner depicted earlier)?
[This illustrates how we all collectively find examples like these easy to comprehend -- which is part of what the phrase "common understanding" means. Follow the above link for more details.]
Of course, it could be objected that the above examples of "motion" aren't at all what Engels meant by that word. Indeed, he was quite careful to emphasise that he was only interested in one sort of motion: continuous change of place with respect to time:
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
"All motion is bound up with some change of place, whether it be change of place of heavenly bodies, terrestrial masses, molecules, atoms, or ether particles. The higher the form of motion, the smaller this change of place. It in no way exhausts the nature of the motion concerned, but it is inseparable from the motion. It, therefore, has to be investigated before anything else." [Engels (1954), p.70. Bold emphasis added.]
"Among natural scientists motion is always as a matter of course taken to mean mechanical motion, change of place. This has been handed down from the pre-chemical eighteenth century and makes a clear conception of the processes much more difficult. Motion, as applied to matter, is change in general." [Ibid., p.247. Bold emphasis alone added.]
Engels was perfectly clear and specific that he meant "simple mechanical change of place", which is radically different from the non-standard use of the word "move" (and its cognates) in the last few sections of this Essay.
Or, so it could be argued...
[Of course, that doesn't mean Engels didn't recognise other, perhaps more complex forms of motion; quite the opposite, in fact!]
Unfortunately, however, we have discovered that it isn't easy to determine what (if anything!) Engels actually had in mind by "simple mechanical change of place". Indeed, much of what he said is compatible with no movement having occurred so that the supposedly 'contradictory' aspects of an object's trajectory (as he pictured it) fail to distinguish moving from stationary bodies. Moreover, as we have also seen his words imply that 'dialectical objects' expand alarmingly, concertina destructively or spread out and occupy their entire trajectories whenever they move. In addition, it was also shown that scientific, mathematical and even philosophical uses of the word "place" are themselves highly ambiguous and problematic (that Is unless the ordinary use of that word is invoked to clarify things!). Engels certainly didn't clear any of this up, even if he was aware of it. In that case, his employment of the phrase "mechanical change of place" is far from perspicuous.
Furthermore, dialecticians can't appeal to what we 'all know' about the meaning of the word "motion", nor should they suppose we 'all know perfectly well' what Engels meant when he spoke about it. As the above examples reveal, there is no one thing we all mean by this word and its associated terms, even though most (if not all) speakers know what they mean when such language is used in ordinary contexts (like those depicted earlier). Indeed, we have just seen that objects can move even while they undergo no "change of place", contradicting Engels. What is more, they can undergo a "change of place" while remaining perfectly still.
As far as a scientific understanding of "place" is concerned, we can't talk about the "same place" even there, as Professor Brian Cox points out in this YouTube video. Because the earth is moving, a 'stationary' object on the earth (such as an office building) isn't actually stationary. Along with every other 'stationary object' on the planet it is moving pretty fast relative to the Sun. Nevertheless, that building is still in 'the same place' relative to other objects (other buildings, and only if we ignore minor movements in the Earth's crust, etc.), which means it is in fact moving while remaining absolutely motionless (again, if we rely on a naive reading of Engels's garbled definition)! Moreover, because the Earth orbits the Sun, and the Solar System orbits the Galaxy, which is also flying through space at an eye-watering speed. In fact, the Galaxy is embedded in 4-space, and it is that space that is moving (or expanding), not so much the Galaxy, which is simply being carried along, as it were, rather like a leaf swept along by the current. Hence, the aforementioned building can't return to the 'same space' it occupied 24 hours earlier. That remains so even though it won't have left the (relative) space it occupied during that time. So, here we have a change of place with no change of place! Motion with no motion!
That represents yet another 'contradiction' -- but, only for the radically confused or the wilfully ignorant among us.
Did Engels mean this sort of 'change of place'?
Not unless he beat Einstein to the punch.
And, with respect to Engels's (more ordinary) use of such terms, we may only agree that DM-theorists know what he meant by "motion" when they succeed in explaining to the rest of us -- with a level of clarity that has hitherto escaped them on this and every other topic connected with DM -- precisely what that is!
Unfortunately, to date, there have been no significant moves in that direction (irony intended).
Furthermore, it is worth pointing out that the vast majority of the above examples were deliberately drawn from everyday situations, those that are readily understood. It is Engels's (Hegelian) use of the word "move" that turns out to be non-standard and, in the end, totally incomprehensible.
Finally, it might be felt that the above emphasis on the ordinary sense of words is hardly appropriate in relation to a scientific or philosophical study of motion and change. That objection was neutralised earlier, but it has also been covered in much more detail elsewhere at this site. Anyway, Engels himself used what look like ordinary words to make his point -- which was that every example of motion in the universe involves a contradiction, including those that are depicted by a use of the vernacular.29
Inferences From Language To The World
Thought Experiments In Place Of Scientific Investigation
Again, it could be argued that any theory of motion has to involve contradictions because of what must be the case if objects in reality -- independent of thought -- actually move, which they clearly do. Hence, despite what we might say, the real world exhibits countless examples of motion and change, each of which involves contradiction.
The use of modal terms (e.g., "must" and "has to") here is revealing in itself since it confirms something that has been implicit all along (and has been hinted at several times already): DM belongs to a long tradition that relies on inferences drawn from the alleged meaning of a few specially-selected words -– albeit given an idiosyncratic twist, often isolated from other associated terms and divorced from their ordinary contexts of use -- to a set of 'necessary truths' about fundamental aspects of 'reality', valid for all of space and time. 'Deductions' like these are invariably made before there has even been a perfunctory gesture in the direction of experimental or observational evidence --, which, in the case of DM-theorists, are never carried out anyway, even after the event (as we discovered in Essay Seven Part One). How many experiments did Engels, Plekhanov, Lenin and Trotsky carry out? Indeed, how many did Hegel perform? In relation to DM, not HM, how much (new primary) data do contemporary DM-fans collect or even process? When has a single one of them initiated, let alone completed, a serious, controlled scientific investigation of Engels's Three 'Laws', for instance? Or the supposed contradictory nature of motion (perhaps to determine how far apart the two "places" are that Engels spoke about -- currently, no one knows!). Even in the formed Soviet Union (or, for that matter, in any of the former and current 'communist' states) there appear to have been none. [If anyone knows differently, please email me with the details.] At best, all we find are a few paragraphs or maybe a few pages of specially-selected, secondary/tertiary evidence, much of it mis-described or irrelevant, none of which turns out to support the theory anyway (again, as we saw in Essay Seven Part One -- link above). In the meantime, the same old hardy perennials are regularly wheeled out (boiling and freezing water, etc., etc.), while the mountain of contrary evidence is simply ignored. [Essay Seven Part One, again!]
The Super-Truths such 'hyper-bold DM-inferences' appear to 'reflect' are, as a result, are regarded as absolutely certainty, which their 'discoverers' find impossible it to question (or if they dare do that, they have the dread word "Revisionist!" thrown at them -- or worse still if your name is Rosa Lichtenstein). That is, of course, because they are based on language, not evidence. [On that in general, see Essay Twelve Part One. Why DM-fans react this way is explored in Essay Nine Part Two.]
As pointed out earlier, Engels performed not one single controlled experiment, he made no detailed observations and carried out zero surveys before (or even after) he drew several universal DM-conclusions about every single moving body in the entire universe for all of time -- in the present case, from the following seriously limited linguistic base:
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them.... But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152.]
In fact, it is impossible even to describe a single observation or experiment (other than a thought experiment, of course, which would itself depend on the sort of ambiguities highlighted earlier in this Essay) that could conceivably 'confirm' Engels's claims about motion. That is partly because 'contradictions' themselves can't be observed, and partly because of the universal, necessary and omni-temporal character of the conclusions themselves.30
This means that the only substantiation Engels offered, and could conceivably have offered, in support of the claims he made about motion are language-based. If asked for proof, all he could have done in response is refer his critics to what certain words 'really meant'. It would be no good asking non-believers to look closer or even harder at the phenomena or the evidence (of which there was none, anyway!). Nor would it be any use telling them to refine their search or their observations, or suggest they redo their experiments and then re-check their data.
That is, of course, why we find no evidence at all in books and articles on dialectics that confirm, or even vaguely support, Hegel and Engels's theory that motion is contradictory.
What we find in its place is a set of dogmatic assertions, which are themselves linked to a half-baked, sketchy, mis-described thought experiment, and one based solely on a perfunctory consideration of the alleged meaning of a handful of words (or concepts), which have themselves been completely bent out of shape.
Readers are invited to check! Email me if you know of, or have ever encountered, seen or even heard of any such evidence in the DM-literature, or you have managed to contact a DM-supporter who offered, referenced or cited any at all in support of the theory that motion is 'contradictory'. Speaking for myself, in over thirty-five years I have yet to find any, or, indeed, encounter anyone in real life or on the Internet who either claimed there was any or who actually produced any. And that includes evidence presented in academic literature in general (DM- or non-DM). There has been none produced in the entire history of mathematics, science and philosophy! As noted above, DM-fans sometimes offer a few paragraphs or maybe even a few pages of (secondary or tertiary) evidence in support of Engels's 'three laws' (which Essay Seven Part One was able to show doesn't support them, anyway), but not one single paragraph, never mind a single page, of actual physical evidence has ever been presented in support of Engels's claims about motion in well over a hundred-and-forty years by the DM-fraternity. Why is that? At least they make some attempt to substantiate Engels's three 'laws' with what turns out to be an embarrassingly perfunctory display of (p*ss poor) 'evidence', but not one of them has ever even gestured at doing the same with respect to the 'contradictory nature of motion'. And, what is perhaps even odder still, DM-fans appear not to have noticed this glaring disparity, either --, even though they all spare no effort telling us that their theory is 'based on the facts', not foisted on them! Again: why is that?
In addition, this doctrine can't even be confirmed in practice! What practice could possibly confirm the doctrine that motion is contradictory? One would have thought that that fact alone would have rung a few alarm bells in a few dialectical heads!
Thus, Engels's only 'evidence' was based on a quirky, 'philosophical' use of language -- in fact, on Hegel and Zeno's use of language --, but not on how such words are employed in everyday life. This predicament (which Engels shares with all other metaphysicians) invariably passes unnoticed because this approach to 'the discovery of philosophical knowledge' is almost totally universal and has been practised ('East' and 'West') for well over two thousand years. In all that time, it was imagined that simply by mulling over the (supposed) meaning of certain words (i.e., their 'real meanings'), Armchair Philosophers were really examining the world itself, and not imposing the alleged meaning of a few specially-selected terms on the world.
We have already seen that Marx and Engels warned us about this over 170 years ago:
"One of the most difficult tasks confronting philosophers is to descend from the world of thought to the actual world. Language is the immediate actuality of thought. Just as philosophers have given thought an independent existence, so they were bound to make language into an independent realm. This is the secret of philosophical language, in which thoughts in the form of words have their own content. The problem of descending from the world of thoughts to the actual world is turned into the problem of descending from language to life.
"We have shown that thoughts and ideas acquire an independent existence in consequence of the personal circumstances and relations of individuals acquiring independent existence. We have shown that exclusive, systematic occupation with these thoughts on the part of ideologists and philosophers, and hence the systematisation of these thoughts, is a consequence of division of labour, and that, in particular, German philosophy is a consequence of German petty-bourgeois conditions. The philosophers have only to dissolve their language into the ordinary language, from which it is abstracted, in order to recognise it, as the distorted language of the actual world, and to realise that neither thoughts nor language in themselves form a realm of their own, that they are only manifestations of actual life." [Marx and Engels (1970), p.118. Bold emphases alone added.]
The Idealist implications of the traditional approach to 'knowledge' were once again highlighted for us by George Novack:
"A consistent materialism cannot proceed from principles which are validated by appeal to abstract reason, intuition, self-evidence or some other subjective or purely theoretical source. Idealisms may do this. But the materialist philosophy has to be based upon evidence taken from objective material sources and verified by demonstration in practice...." [Novack (1965), p.17. Bold emphasis added.]
[The reason for the age-old confusion of talk about talk with talk about things (i.e., the confusion of language with what it supposedly 'reflects' or 'represents') is examined in detail in Essay Twelve Part One (as well as the rest of Essay Twelve -- summary here). Why Engels changed his mind about philosophical language and once again began to draw inspiration from Hegel twenty or so years after the above was written is explained in Essay Nine Part Two.]
Nevertheless, the presumed denotation of the obscure jargon concocted by Traditional Theorists is simply taken for granted; indeed, the question whether such words actually have a denotation to begin with is seldom even asked.
The unremittingly negative opinion expressed about the sort of 'philosophical word-juggling' we have witnessed in this Essay (from the likes of Zeno, Hegel, Engels and Lenin) gains support from the additional fact that 'problems' like this can't be solved by an appeal to evidence. That is because they depend solely on "distorted language" (as Marx and Engels described it), which is why that is all Engels ever offered his readers in support of his theory of motion, and why it is all that DM-theorists could ever offer their readers (in the way of support) on this topic.
Nevertheless, Engels restricted his comments neither to examples of motion he had personally investigated, nor to the entire range experienced by humanity up to his day. Despite this, he still felt confident that he could extrapolate from his own understanding of a few ordinary-looking words to conclusions that were applicable to every conceivable example of motion anywhere in the entire universe, for all of time:
"Never anywhere has there been matter without motion, nor can there be…. Matter without motion is just as inconceivable as motion without matter. Motion is therefore as uncreatable and indestructible as matter itself…." [Engels (1976), p.74. Bold emphases added.]
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Ibid., p.152. Bold emphasis added.]
In fact, what Engels actually did -- and this was the extent of the 'careful' scientific research he carried out in this area -- was to reproduce the 'analysis of motion' he found in Hegel's 'Logic'!
Unfortunately for DM-fans, Hegel hasn't gone down in history as an experimental scientist of any note, either.
As we shall see (in Essays Nine Parts One and Two, and Essay Twelve (summary here)), these all too easily overlooked facts possess several revealing ideological implications of their own.
Engels's feeling of confidence in the conclusions he so effortlessly drew, no doubt arose from his consideration of a rather narrow interpretation of one connotation of "motion". Hence, we find him claiming that:
"[E]ven simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it." [Engels (1976), p.152.]
But, how could Engels possibly have known this? How could he have been so sure that every single episode of motion throughout the entire universe, for all of time, could only proceed in the way he supposed? If we rule out the absurd idea that Engels was a deity of some sort, there are in fact only two conceivable answers to that question:
(1) His certitude was based on his grasp of the 'concept of motion itself'. But, as seems obvious from his comments, Engels actually based his conclusions on his own understanding of a strictly limited set of words about motion -- indeed, on those he had imported from Zeno and Hegel -- not on the 'concept of motion itself' (if there actually is such a thing). Neither he nor anyone else has access to any such concept independently of the words that supposedly allude to, or express, 'it'.
And yet, divorced from the wide variety of ways we ordinarily talk about motion (illustrated in and by the many diverse examples aired in this Essay), who is to say what is the correct way to understand such words especially when they are used in novel contexts like these? Or, whether the meaning of any technical terms that have been co-opted and pressed into service are the same as. or are different from, the meaning of the ordinary words they supposedly resemble, replace or supersede? Or, that there is only one way to interpret them? Or, that Engels and Lenin had somehow hit upon the correct way to do that (and then only after reading Hegel, as opposed to sifting through the relevant scientific literature)? Or even: whether the language they actually employed means anything at all?
[As we have repeatedly seen, there is considerable doubt about that, and not just in relation to attempts to describe motion in such terms.]
However, and more to the point: precisely who decided that such off-the-cuff conclusions about substantive features of the world, true for all of space and time, can just be read-off from the alleged meaning of a few words?
Did the rest of us miss a meeting?
(2) The second possible answer revolves around a likely response that might have already occurred to the reader, namely this: Surely a rejection of Engels's understanding of motion would be paradoxical, if not contradictory. That is because it would clearly represent a repudiation of what the concept of motion itself actually implies. Consequently, given that view, anyone who fails to interpret motion in a way that failed to represent it as involving a body being in two places at once (etc., etc.)) would only succeed in exposing their own failure to understood what motion is in-itself. Either that, or they will have inadvertently revealed they had devoted far too little thought to the problem. Indeed, such a lack of comprehension would itself contradict what we all ordinarily understand motion to be.
Or, so it could be claimed...
However, Engels's analysis of motion is itself paradoxical, if not openly contradictory. No dialectician has yet been able to explain exactly how a moving body can be in two places at the same time -- other than keep asserting it for an alleged fact -- and with zero actual evidence in support. So even by its own lights, there appear to be equally good reasons to reject his interpretation of motion as there are for accepting it -- that is if either alternative were based on paradox alone. If it is paradoxical to reject Engels's theory, it is equally paradoxical to accept it.
[In fact, as we have seen Engels's theory itself carries such ridiculous implications that no rational person could possibly accept it.]
Anyway, an appeal to experience to decide between these two alternatives (i.e., the rejection or the acceptance of Engels's theory of motion) would be of little help -- and for at least four reasons:
(i) As has already been pointed out, Engels drew these conclusions about motion without referring to any evidence at all. Hence, his theory of motion clearly wasn't based on experience. In fact, his ideas in this area sought to interpret reality beyond any and all conceivable evidence/experience.
(ii) Our experience of motion is as varied and ambiguous as the words we use to depict it are. The examples given earlier (and in Note 28, for instance) indicate that our ordinary ways of speaking about motion are far more complex than Zeno, Hegel, Engels, Plekhanov or even Lenin imagined. Of course, in their everyday lives and their ordinary use of language they will all have shown that they were abundantly aware of this. As has happened countless times in the history of our species, it is only when a few 'bright sparks' began to 'philosophise' that they allow themselves to be led astray and use language as if they were complete novices, had serious 'learning difficulties' or were visitors from another planet. Anyway, not even an indefinitely large (finite) number of observations of cats moving on and then off mats (and the like) could confirm whether motion is or isn't:
(a) Continuous;
(b) Discontinuous; or if it is,
(c) Composed of countless discrete or even staccato, concatenated 'sub-movements'; or, indeed, whether it is,
(d) Something else for which we have as yet no words.
Even with advanced technological assistance, we still wouldn't be able to tell if motion is the one or the other.
[Indeed, as we have seen, there is no one thing that motion is. Our use of this word (and its associated terms) is far too complex to be forced into this ill-fitting dialectical boot. (On that, see the next two points.)]
(iii) Ordinary language, and thus everyday experience -- as an indisputable matter of fact --, allows for both types of motion: discrete and continuous. That has been repeatedly demonstrated in the examples given throughout this Essay. It is only a metaphysical prejudice that calls for the labelling of certain ways of depicting or of viewing motion as a "mere appearance" (to be ignored by all 'genuine philosophers'), or blames it on "commonsense" and "formal thinking", while other (preferred) ways of describing it supposedly deal with "reality itself". Or even claim that one specific (and favoured) type of motion is primary, all the rest are secondary or 'unreal'.
(iv) The notion that there are such entities as "things-in-themselves" (or that there is something called "motion-in-itself", or "motion itself") is hopelessly confused, and that isn't just because it expresses, or even hides, a thinly disguised reference to "absolute" motion -- as will be argued elsewhere at this site (until then, see Note 10). As we will see, reference to "motion-in-itself" is unintelligible; small wonder then that it has yet to be explained by anyone.31
Nevertheless, and once more, a repeated use of the word "must" in response to the above -- as in, for example, a retort that might well have occurred to one or two readers: "That's all very well, but motion must involve a body being in two places at once…(etc., etc.)" -- could itself only have been based on a conceptual or linguistic analysis of a limited range of words associated with this phenomenon. Again, that would simply confirm the view maintained in these Essays that dialecticians are happy to derive 'universally true' conclusions from a handful of specially-selected, narrowly-interpreted words, which 'results' they are then quite happy to foist on 'reality'.
When pressed to provide actual evidence in support of their claim to have what can only be described as Super-Scientific Knowledge of 'motion itself' -- that applies in and across all regions of space and time -- all that recalcitrant DM-theorists could offer in support would be the supposed meaning of a few words!
Once again, apart from an absurd alternative explanation (i.e., that those making these claims are deities of some sort, and hence have access to a mystical source of knowledge that has revealed to them the "true nature of reality-in-itself", but which source is unavailable to the rest of humanity), some form of conceptual/linguistic 'juggling' is the only way that hyper-bold 'dialectical doctrines' like these could ever have been cobbled-together.
And that explains why Engels omitted any reference to data supporting his 'theory' -- and no one since has bothered to supply any.32
It might be felt that this Essay and this site completely miss the point: DM deals with real material contradictions in the actual world, verified by careful empirical investigation, tested in practice. Not only that, it is based on the theory that reality is contradictory, and that idea is itself founded on a scientifically confirmed belief in universal change. Furthermore, this theory goes way beyond the (erroneous) allegation that this is only true of the language we use to depict nature. It might be the case that contradictions in nature and society are difficult to capture or express in ordinary language, but that is because ordinary language is inadequate in such areas (as, indeed, TAR itself maintains; cf., Rees (1998), pp.45-52), even if it is perfectly acceptable in other, everyday surroundings. That certainly doesn't show reality is a contradiction-free zone.
Or, so it could be argued, once more...33
However, that (proffered) pro-DM response won't do. Admittedly, the world is the way it is independent of language and human knowledge, but unless we are capable of expressing ideas about the world in a clear and determinate manner we are in no position to draw valid and definitive conclusions about it, least of all extrapolating any such ideas across all regions of space and time --, for example, like this:
"Never anywhere has there been matter without motion, nor can there be…. Matter without motion is just as inconceivable as motion without matter. Motion is therefore as uncreatable and indestructible as matter itself…." [Engels (1976), p.74. Bold emphases added.]
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Ibid., p.152. Bold emphasis added.]
"All motion is bound up with some change of place, whether it be change of place of heavenly bodies, terrestrial masses, molecules, atoms, or ether particles. The higher the form of motion, the smaller this change of place. It in no way exhausts the nature of the motion concerned, but it is inseparable from the motion. It, therefore, has to be investigated before anything else." [Engels (1954), p.70. Italic emphasis added.]
"Among natural scientists motion is always as a matter of course taken to mean mechanical motion, change of place. This has been handed down from the pre-chemical eighteenth century and makes a clear conception of the processes much more difficult. Motion, as applied to matter, is change in general.... The first, simplest form of motion is the mechanical form, pure change of place: (a) Motion of a single body does not exist -- [it can be spoken of] only in a relative sense -- falling." [Ibid., pp.247-48. Italic emphasis in the original.]
"If we wish to make motion clear to ourselves, we say that the body is in one place and then it goes to another; because it moves, it is no longer in the first, but yet not in the second; were it in either it would be at rest. Where then is it? If we say that it is between both, this is to convey nothing at all, for were it between both, it would be in a place, and this presents the same difficulty. But movement means to be in this place and not to be in it, and thus to be in both alike; this is the continuity of space and time which first makes motion possible. Zeno, in the deduction made by him, brought both these points into forcible opposition. The discretion of space and time we also uphold, but there must also be granted to them the over-stepping of limits, i.e. the exhibition of limits as not being, or as being divided periods of time, which are also not divided. In our ordinary ideas we find the same determinations as those on which the dialectic of Zeno rests; we arrive at saying, though unwillingly, that in one period two distances of space are traversed, but we do not say that the quicker comprehends two moments of time in one; for that we fix a definite space. But in order that the slower may lose its precedence, it must be said that it loses its advantage of a moment of time, and indirectly the moment of space." [Hegel (1995), pp.273-74; partially quoted in Lenin (1961), p.257. (The editors of Lenin's text have clearly used a slightly different translation of Hegel.)]
That is all the more so with respect to DM, where we have seen every attempt to render it perspicuous fail miserably -- indeed, as we have just seen in relation to Engels's theory of motion (and as we will continue to see with respect to other key areas of DM throughout the rest of this site). Contrary to what the (proffered) pro-DM-objection claimed, we now know that it is impossible to confirm Engels's claims about motion "by careful empirical investigation, tested in practice". Indeed, as we will see in Essay Ten Part One, practice itself turns out to be an unreliable guide when it comes to testing the truth of a theory.
Moreover, Engels certainly thought he could derive what he took to be a contradiction from a consideration of what he thought were the meanings of several ordinary words used to depict motion and change. But, as we have also seen, Engels and Hegel's conclusions (in support of the theory that motion is contradictory) are shot-through with vagueness and ambiguity, to such an extent that the rational basis of any claim that 'reality is contradictory' has itself been totally undermined. These ideas slide further into oblivion when it is recalled that they were based on a series of egregious logical blunders committed by Hegel, uncritically imported into Marxism, for no good reason (as the Essays at this site have also demonstrated). What is more, this theory will remain in 'epistemological limbo' until DM-theorists produce actual evidence that motion everywhere in existence (past, present and future) is as they say it is. Or, of course, until they succeed in demonstrating that they have access to an alternative (and vastly superior) way of directly 'intuiting deeper aspects of reality' that are (mysteriously) unavailable to the rest of us, which 'allows' them to bypass (or ignore) the need to provide any supporting evidence.
Objects and processes in nature don't confront humanity already sorted, labelled and categorised into neat boxes or categories. We don't literally see contradictions around us 'in reality'. They require considerable argumentative stage-setting, even before dialecticians themselves are in any position to assert that they exist, never mind prove they do to the rest of us. However, as we have seen (here, here and here, for example -- as well as earlier in this Essay), DM-supporters tend to see 'contradictions' everywhere they look, and have developed an annoying habit of asserting they exist without even a perfunctory analysis or examination of the evidence. Nor do they even attempt to derive them 'logically' like Hegel, at least, tried to do. Hence, the question whether or not there are any 'objective contradictions' in nature and society -- based (as that very idea is) on what turned out to be a misuse of language -- is itself irredeemably confused. And, of course, to such non-questions there can be and are no answers.34
Plainly, it is the hasty, non-standard and quirky interpretation that Hegel and DM-theorists imposed on ordinary words that conjured into existence the paradoxes they then labelled "contradictions" -- that is, when, on the rare occasion, they even manage to get that word right.
In which case, far from reality being 'contradictory'/'paradoxical', it is dialecticians' use of language that is incoherent and paradoxical.
In this Essay, we have seen that Engels's theory of motion not only lacks precision it is shot-through with confusion, ambiguity and equivocation. Hence, his ideas in this area are irredeemably obscure. As a result it is far from clear exactly what he was trying to say. But, even if we knew what he was banging on about, his 'analysis' depends on an asymmetric convention that places no restriction on the divisibility of space while it sets a limit on the divisibility of time. That is what seems to generate the 'contradiction' he claimed to be able to see in motion.
Even if the above asymmetric division were waved aside, it turns out that Engels's theory implies that moving objects, if they are anywhere, they are everywhere in their trajectories, all at once, and that they do not so much move as expand (or even contract) alarmingly. Or, they simply fragment.
Finally, we have also seen that his conclusions (again, even if we knew with any clarity what they were) only seem to follow if we ignore the many changes in meaning that words like "place" and "move" undergo when they are used in entirely novel or non-standard contexts. As a result we saw that no sense at all can be made of anything Engels was trying to say about motion and change.
[FL = Formal Logic.]
But, what about the so-called 'Law of Identity'? Doesn't it imply that change and motion are impossible? If so, doesn't that show FL is little or no use in such circumstances? Does it not also suggest that much that has been argued in this Essay is beside the point and can therefore be ignored?
It is to pseudo-problems like those that I now turn.
1. The material that used to be here has now been moved to the main body of this Essay.
2. Engels was, of course, openly borrowing from Hegel. The relevant passages from Hegel's 'Logic' and another of his works have been quoted here.
3. An alternative translation -- which appears in Volume 25 of Marx and Engels Collected Works (MECW) -- renders the last sentence as follows:
"And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [MECW, Volume 25, p.111. This can be accessed here.]
The above rendition manages to neutralise some of the criticisms set out in the main body of this Essay, but not all. Who, for instance, "solves" these contradictions and how exactly do they do it? More to the point, how do they manage to do this quite so quickly (i.e., "simultaneous" with the "origination" of each new contradiction)? And how do they succeed in doing it so many times, too? There must surely be billions of these 'solutions' dotted all along the trajectory of even the shortest journey -- unless we somehow suppose there is just one long 'contradiction' smeared out along the entire path each moving object traverses.
When any such (supposed) contradiction is "solved", does this mean that a moving object is no longer in two places at once, in one of these and not in it at the same time? If not, what does it mean to 'solve' a 'contradiction' like this?
Perhaps even more significant: has a single DM-fan ever asked these questions, let alone tried to answer them? Or have they become so theoretically supine and inert that their critical faculties have now completely atrophied?
[Having said that, Thomas Weston has published some sort of explanation how 'contradictions' can be 'resolved' -- in Weston (2012). {This links to a PDF.} On that, see Note 4a and Appendix A.]
In addition, the above version of Engels's words introduces several difficulties of its own, for it leaves it entirely mysterious from whence these contradictions 'originated'. Indeed, the passage appears to promote contradictions above, or ahead of, motion. They seem to cause it, not it them.
Naturally, in a theoretical system (that has descended with modification from Absolute Idealism itself and where 'reality' is just the 'development of Mind'), the ability of 'contradictions' to cause change, or make things move/happen, seems to make some sort of crazy sense.
Apart from that, it doesn't.
3a. There is more on this in Essay Seven Part One, where I connect issues like this with the sort of low grade Mickey Mouse Science one finds all too often in books and articles about DM written by its defenders.
Several logical topics connected with those raised in this Essay were discussed in much more Essay Four Part One; other related DM-'laws' -- such as the "interpenetration of opposites" and change through "internal contradiction" -- were covered in Essay Seven Parts One and Three, as well as Essay Eight Parts One, Two and Three.
Any who (erroneously) think that this site doesn't address 'dialectical contradictions' (whatever they turn out to be, if we are ever told with any clarity!) should read this, this, this, this and this, and then perhaps think again.
4. On that, see Note 3, above.
However, as we discovered in several other Essays published at this site, dialecticians regularly make this mistake, imagining that they are talking about the world when in fact they are indirectly drawing attention to their own idiosyncratic use of language and the supposed implications thereof. That is, of course, part of the reason why DM is classified at this site as a form of LIE. [For more on that, see Essays Three Part One and Twelve Part One.]
[LIE = Linguistic Idealism.]
4a. I have just read Thomas Weston's 'answer' to some of these questions -- i.e., Weston (2012). [This links to a PDF.]
[AD = Anti-Dühring, i.e., Engels (1976); MECW = Marx and Engels Collected Works.]
Among other things, Weston sought to show that Marx's view of 'dialectics' (especially in Das Kapital [DK]) was the same as Engels's view expressed in, for example, AD, citing several passages from DK in support (all of which have been shown not to imply what Weston imagines they do -- in Essay Nine Part One). I have also argued throughout Essay Eight Part Two (links below) that Weston's interpretation of a specific passage taken from DK (quoted below) doesn't support the theory that Marx believed there were 'dialectical contradictions' in nature and society -- modelled, for example, by opposing forces:
"We saw that the process of exchange of commodities includes relations that contradict and exclude one another. The development of the commodity does not overcome [aufhebt] these contradictions, but creates a form within which they can move themselves. This is in general the method through which real [wirkliche] contradictions solve [losen] themselves. It is a contradiction, for example, for one body to continuously fall into another, and just as constantly fly away from it. The ellipse is one of the forms of movement in which this contradiction is actualised [verwirklicht] just as much as it is solved [lost]." [Marx, quoted in Weston (2012), pp.5-6. This links to a PDF; italic emphases in the original. This is Weston's translation of the passage below.]
"We saw in a former chapter that the exchange of commodities implies contradictory and mutually exclusive conditions. The differentiation of commodities into commodities and money does not sweep away these inconsistencies, but develops a modus vivendi, a form in which they can exist side by side. This is generally the way in which real contradictions are reconciled. For instance, it is a contradiction to depict one body as constantly falling towards another, and as, at the same time, constantly flying away from it. The ellipse is a form of motion which, while allowing this contradiction to go on, at the same time reconciles it." [Marx (1996), p.113.
This links to a PDF; italic emphases in the original.]
[I have quoted Weston's translation of the aforementioned passage alongside the one found in MECW. For my (contrary) view of this passage and criticism of Weston's interpretation of its significance, see here, here, here, here, here and here.]
However, Weston also added a few pages of comments concerning the 'resolution' of 'dialectical contradictions' that weren't covered in Essay Eight Part Two. I have now added several of my own thoughts about this to Appendix A, where I have re-quoted very nearly all of Weston's remarks on this topic.
[Spoiler Alert - 03: As Appendix A shows, Weston failed miserably to explain how such 'contradictions' can be "solved"/'resolved'.]
5. The overall picture is, of course, far more complicated than this opening salvo might initially suggest. Later on in this Essay examples will be given where we find that both stationary and moving objects occupy two places at once. Nevertheless, it is reasonably clear that Engels didn't have them in mind when he spoke quite so boldly and confidently about the supposedly 'contradictory nature of motion'. On the other hand, if he had taken them into account, his whole 'analysis' would have been undermined from the start.
Quantum phenomena that allegedly falsify the claim that there is no evidence that moving objects occupy two places at once (etc.) in fact fail to do so. For instance, no one supposes that experiments which suggest an electron can be in two places at once mean that it moves from one of these locations to the other, or, indeed, that it does so in no time at all. What is supposed to have happened (but, only under certain interpretations -- physicists are still trying to make up their minds about how to understand this phenomenon) is that when one electron is aimed at a double slit and focused on a screen it appears to have taken two separate paths at the same time. In that case, it hasn't moved between these two trajectories, jumping from one to the other. It has, it seems, merely followed two paths. Why some DM-supporters view this a confirmation of their theory, is, therefore, a mystery.
It could be argued that the fact one object can take two paths at once is obviously a contradiction, which confirms the theory that nature is fundamentally contradictory. Even if that were the case (however, as I have pointed out in Essay Seven Part One (on this, follow the links below) there is good reason to question that interpretation), the question still remains: Is this a 'dialectical contradiction'? In that case, do these two paths 'struggle' with and then turn into one another (which they should do if the DM-classics are to be believed)? Do they imply each other, such that one can't exist without the other, like the proletariat supposedly can't exist without the capitalist class? The existence of one class logically implies the existence of the other (or so we are told). But, if that were the case, physicists could simply have 'reflected on the concept of an electron' to see this logical truth follow from it. They certainly didn't need to conduct any expensive, time consuming experiments in order to discover this (supposed) fact about electrons. After all, who, still in command of their senses, runs tests to find out if Vixens are really female foxes? How many Dialectical Marxists conduct experiments to see if the proletariat actually does imply the capitalist class (and vice versa)? In that case, whatever else this phenomenon is, it can't be a 'dialectical contradiction'.
Furthermore, as noted above, there are scientific realists who question the standard interpretation of such experiments. On that, see Essay Eleven Part One, for example, here and here.
[This topic is obviously connected with so-called wave-particle duality, which has become -- shall we say -- 'problematic' now that Quantum Field Theory informs us that the electron isn't a particle, after all, but an "excitation" in "the field". I have said much more about that, here and here. Finally, I have questioned the 'dialectical link' that is supposed to exist between the proletariat and the capitalist class (i.e., the idea that these two classes imply one another, etc.) in Essay Eight Part Two.]
5a. However, and independently of the comments made in the main body of this Essay, if instants have no duration then, according to Trotsky, they don't exist. Presumably, that is because they are merely 'abstractions'. But, if that is so, exactly from what they have been 'abstracted' or what they are predicated upon, Trotsky unfortunately neglected to say. How does one abstract an instant? Insubstantial spectres such as these can't be a property that all temporal intervals share. Physically non-existent, durationless (mathematical) 'points' are not what each second, minute or hour have in common. How could this be what duration is composed of? A set of 'instants' that not only have no duration, but don't actually have the decency to exist?
[On this, also see Note 6.]
6. 'Abstractionism' (especially the mutant DM-version) was critically examined and taken apart in Essay Three Parts One and Two.
Instants in time share nothing with our experience of time, and so they can't be derived from it by a 'process of abstraction'. Moreover, attributing such durationless points to "moments in time", or even to "temporal intervals", would be to attribute them with properties, qualities and features they don't have -- that is, of course, zero duration and non-existence! Ordinarily, we associate a "moment in time" with a few seconds (depending on the context). If someone were to say "Wait a moment!", and that moment were meant to be durationless, it would be tantamount to saying "Don't wait at all"! The phrase "a couple of moments" would, in effect, be the equivalent of "no moments whatsoever". In which case, such 'instants' aren't even idealisations of time, since, as already noted, they share nothing with them.
Of course, it could be argued that despite the above scientists and philosophers regularly extrapolate from finite moments in time (i.e., from non-zero temporal intervals) to the above sort of instant. Hence, as such, while they are Ideal constructs, they can still be mapped onto the Real Numbers, for example.
That argument/analogy has been neutralised below (and, in general, in the two Essays to which I have just linked a few paragraphs back); however, the following material comes from Essay Six (slightly edited):
Again it could be argued that identity criteria for temporal instants could be specified by mapping them onto the Real Numbers; since the latter are distinguishable, the former must be, too. Given this scenario, such instants would be isomorphic to the Reals.
In response to this, several points are worth making:
(1) This view assumes that 'time itself' (as opposed to the measurement of time) is composed of discrete units, and that they can therefore be counted (or, at least, mapped onto the Positive Integers/The Positive Reals). But, that sits rather awkwardly with the idea that temporal instants can be measured, which appears to suggest that time must be both discrete and continuous.
[Dialecticians might be happy with that implication, but just watch them then (i) Squirm when asked to explain (in physical terms) how that is even possible, or (ii) Reach for yet another Nixon card.]
(2) The only 'evidence' for the validity of such a manoeuvre derives from the proposed isomorphism itself. In that case, any criteria of identity for instants in time that result from this mapping would clearly be a reflection of the imported properties of Real Numbers, which is precisely the point at issue. If 'instants' in time have no identity -- that is, if they aren't discrete (or, rather, if their ordering isn't the result of the application of an inductive law to a discrete variable; or, indeed, to any variable at all) -- an isomorphism like this would simply amount to their conventionalised re-description.
Hence, the proposed isomorphism could end up misrepresenting the very thing being mapped -- especially if this is considered to be the only way to view time -- since it makes something that appears to be continuous look as if it were discrete, imposing on time a structure it might not possess. [To be sure, mathematicians since Dedekind have regarded the Reals as both dense and continuous. But even then, there is no suggestion that Real Numbers merge into one another, that they have no discrete identities or that they can't be distinguished. Cf., Sanford (2005).] In that case once more, dividing time into temporal instants in this way would impose on it something it might not have.
It seems, therefore, that time can only be broken up into metaphysical instants if it is mapped onto something that is already fragmented (in the above sense), like the Reals. On the other hand, if time is only continuous (and isn't composed of discrete 'instants'), then it can't be mapped, without distortion, onto the Real Numbers, which are discrete but continuous (again, in the above sense -- that is, there are no 'gaps' between them, with "gap" defined in a specific way in Real Analysis (this links to a PDF)). Of course, any supposition to the contrary would suggest that it isn't in fact time which has been mapped onto Real Numbers, but Real Numbers that have been mapped onto themselves, and then misleadingly re-labelled "instants".
[That comment disposes of the claim that scientists are actually speaking about time when they talk this way. What they are in fact doing is talking about Real Numbers in drag. (On this in general, see Read (2007), pp.79-115, on which many of my own ideas have been based.)]
(3) A successful isomorphism would itself depend on an application of the LOI (interpreted as a rule, not as a 'philosophical truth'), making this attempt to patch up the argument of little use to DM-theorists --, or, at least to those who are still concerned to observe even a modicum of consistency.
[LOI = Law of Identity.]
Of course, all this is independent of the fact that isomorphisms are creatures of convention; they don't actually populate the universe. Any attempt to use them to shore up DM would be unwise, therefore, since it would imply that whatever is concluded about the LOI would likewise be a product of convention, and hence not at all 'objective'.
[There is an interesting, if somewhat metaphysical, discussion of this topic in Adamson (2002), pp.5-58. Nevertheless, Adamson's 'solution' (based on the radically confused writings of Henri Bergson) seems to be far worse than the problem it was meant to solve. (It is also worth adding that Adamson's characterisation of Analytic Philosophy is highly misleading. However, I don't propose to defend that accusation here.)]
7. The material that used to be here has now been moved to the main body of this Essay.
7a. That is to say, our everyday -- or even our scientific -- thoughts about motion aren't contradictory, whereas the ideas concocted by Idealist Philosophers may very well be. Always assuming, of course, that sense can be made of anything they actually come out with!
8. The material that used to be here has now been moved to the main body of this Essay.
The reason why this ancient view of the world was both conducive to, and supportive of, wider ruling-class priorities and interests will be covered in Essay Twelve (summary here), but it was hinted at earlier. [See also, here and here.]
8a. Of course, it could be argued that since everything in the universe is in motion, the question, "Which came first, motion or contradiction?" hardly arises -- no more than would asking which kicked in first, numbers or counting. However, as we will see, things aren't quite this simple and straight-forward. Quite the reverse, in fact. [No pun intended.]
9. Passages from Lenin (and others) concerning 'internal contradictions' and self-development (etc.) were quoted in Essay Two. [Cf., Rees (1998), p.7.] This topic is also examined in greater detail in Essay Eight Parts One and Two.
9a. Although Woods and Grant [W&G] clearly came very close to asserting they believed there is just such an internal motor powering moving objects and which keeps them moving when they argued as follows:
"So fundamental is this idea to dialectics that
Marx and Engels considered motion to be the most basic characteristic of
matter.... [Referring to a quote from Aristotle:] [T]his is not the
mechanical conception of motion as something imparted to an inert mass by an
external 'force' but an entirely different notion of matter as self-moving.... The essential point of dialectical thought is not that it is based on the idea
of change and motion but that it views motion and change as phenomena based on
contradiction.... Contradiction is an essential feature of all being. It lies at
the heart of matter itself. It is the source of all motion, change, life and
development. The dialectical law which expresses this idea is the unity and
interpenetration of opposites....
"The universal phenomena of the unity of opposites is, in reality, the
motor-force of all motion and development in nature. It is the reason why it is
not necessary to introduce the concept of external impulse to explain movement
and change -- the fundamental weakness of all mechanistic theories. Movement,
which itself involves a contradiction, is only possible as a result of the
conflicting tendencies and inner tensions which lie at the heart of all forms of
matter.... Matter is self-moving and self-organising." [Woods and Grant (1995),
pp.43-45, 47, 68, 72. Bold emphases added. Several paragraphs merged.]
The long quotation from Hegel (given above) shows where W&G 'discovered' these decidedly odd ideas; they certainly didn't obtain them from any contemporary scientists living on this planet (or, rather, any who weren't already in thrall to these ancient, mystical ideas themselves). [On that, see Essay Eight Part One.]
10. In fact, Engels himself torpedoed the idea that forces could be viewed as 'contradictory opposites' when he reasoned as follows:
"All motion is bound up with some change of place…. The whole of nature accessible to us forms a system, an interconnected totality of bodies…. [These] react one on another, and it is precisely this mutual reaction that constitutes motion…. When two bodies act on each other…they either attract each other or they repel each other…in short, the old polar opposites of attraction and repulsion…. It is expressly to be noted that attraction and repulsion are not regarded here as so-called 'forces', but as simple forms of motion." [Engels (1954), pp.70-71. Bold emphasis added.]
As will be argued in detail in Essay Eight Part Two, that observation pulls the rug from under anyone who wants to maintain that forces can be used to model contradictions -- especially those who regard Engels as some sort of authority on such matters.
Anyway, and despite the above, the 'DM-theory of motion' does no real work. No 'explanation' of this phenomenon is advanced so much as one nanometre by re-describing or re-configuring it as "contradictory". The supposed 'contradiction' in motion (whereby a body (supposedly) is and is not in a given place, and is in two such locations at the same time, etc., etc.) neither initiates, changes nor sustains movement. It simply re-describes it.
Furthermore, if Absolute Space is left out of the picture, the precise nature of any motion in a system clearly depends on the inertial frame chosen. It doesn't depend on the 'simultaneous occupancy and non-occupancy' of certain point locations. This can be seen by the fact that given a particular frame of reference, a body would be at rest relative to that frame, but with respect to another frame it would be in motion. In that case, motion is inertial-frame-sensitive, not 'vaguely-located-point-occupancy-and-non-occupancy'-dependent.
It would seem, therefore, that unless DM-theorists believe in Absolute Space, their insistence that motion is contradictory (because their quirky theory holds that moving bodies occupy two points at the 'same moment', etc.) is unsustainable. Theories that promote the idea that space and time are relative clearly seem to imply that the supposed 'contradictory' behaviour of moving bodies is a consequence of a change of reference frame. Hence, the very same body will be in motion in one frame but stationary in another. If so, they wouldn't be either moving or not moving because of the alleged contradictions inherent in 'motion itself'. In which case, even if we were determined to describe motion in such paradoxical terms, its 'contradictory nature' can't be an 'objective' feature of 'reality' if it promptly disappears as soon as a different inertial frame is chosen. That is, if in one such frame the same body is moving, while at the same time in another it isn't!
It could be objected that just because motion apparently stops and starts according to the choice of reference frame that no more means its contradictory nature isn't objective than it would mean that, say, the boiling point of water wasn't really 100ºC if it were measured in degrees Fahrenheit (212ºF) or in degrees Kelvin (373.2ºK).
Unfortunately, if that were an effective reply to the anti-DM argument outlined above, it would also be fatal to DM itself. That is because it openly concedes that scientific knowledge is conventional.
Again, exception might be taken to that response. It could be argued that the fact that the temperature of a body can be read on two or more different conventionalised scales doesn't imply that temperature itself (or whatever it supervenes upon) isn't an objective feature of reality. The same goes for the depiction of motion in different reference frames.
However, these two cases aren't analogous. No matter what system we use, a body has some temperature or other (with the latter defined perhaps in terms of its energetic profile). That isn't the case with motion and the choice of inertial frame (unless, of course, we count a zero velocity as a velocity by default -- but, even then, the alleged 'contradiction' would still vanish).
In one particular frame, a body could be in motion and, if we assume DM is correct, that movement might appear to be 'objectively' contradictory. But, in another frame -- at the same time -- that body could be stationary/motionless and objectively non-'contradictory' (even in Engels's sense of that word), too. Hence, at the same time a body could be moving and not moving, 'contradictory' and 'not contradictory'. Which of these options is finally settled upon will be a consequence, not of the nature 'motion itself' (whatever that means), but of the choice of reference frame. Since reference frames aren't 'objective' features of the world (they are human inventions!), and since the 'contradictory' nature of motion is sensitive to choice of frame, the conclusion seems inescapable: the (supposed) 'contradictory' nature of motion isn't an 'objective' feature of 'reality', either.
Several readers might now focus on the following sentence and point out that it is contradictory itself; so a contradiction appears somewhere, at least:
"Hence, at the same time, a body could be moving and not moving, 'contradictory' and 'not contradictory'."
In response it is worth noting that that sentence is just another ambiguous string of words; its allegedly contradictory nature will therefore disappear upon disambiguation --, as we saw, for example, here.
It could be argued that the anti-DM points advanced earlier suggest motion itself isn't in fact an objective feature of reality. That must be the case if it disappears when a different reference frame is chosen.
However, that isn't so, for if an object is moving in one frame and is stationary with respect to a second frame, then other objects will be moving in a different way with respect to that frame. So, while (all or some of the) motion would disappear inside the frame in question, motion in the wider system wouldn't. For example, if the first reference frame is centred on a volume interval that contains only the Moon (such that the Moon would be stationary -- in at least one direction -- with respect to that frame, even if only momentarily), other planets (such as the Earth) will be moving relative to that frame. Swap the reference frame to a volume interval that contains only the Earth, mutatis mutandis, and the Moon will now be moving relative to a stationary Earth.
[Sure, the Earth will still be rotating, but all we have to do is make the reference frame relative to a finite region on the Earth's surface, and the Earth (itself) would stop rotating with respect to this new frame. For example, from where you are now sat, or stood, the Earth doesn't appear to be rotating, which phenomenological fact used to be one of the strongest arguments in favour of the idea that our planet is stationary, situated at the centre of the universe. (On whether or not the Earth is even 'objectively' in motion, see here.)]
This means that relative motion (at least as it is viewed in and by contemporary Physics and Applied Mathematics) is a conventionalised bi-product of the choice of inertial frame. If DM-theorists want to rescue the 'objectivity' of their 'theory of motion' from the trashcan of 'subjectivity', it looks like they will have to postulate the existence of Absolute Space. Otherwise, they will be forced to concede that the 'contradictions' they attribute to motion are in fact artefacts of the choice of reference frame, not something inherent to moving bodies. That also appears to be the implication of something Engels had to say:
"Among natural scientists motion is always as a matter of course taken to mean mechanical motion, change of place. This has been handed down from the pre-chemical eighteenth century and makes a clear conception of the processes much more difficult. Motion, as applied to matter, is change in general.... The first, simplest form of motion is the mechanical form, pure change of place: (a) Motion of a single body does not exist -- [it can be spoken of] only in a relative sense -- falling." [Engels (1954), pp.247-48. Bold emphasis alone added.]
As I point out below, if the "motion of a single body does not exist", and can only be spoken of "in a relative sense", it can't be an inherent property of moving bodies. Any attempt to argue motion is an "inherent property of moving bodies" must therefore reject the relativity of space and time and appeal to the old Absolutes of Newtonian Physics, or their equivalent. [On this, see Rynasiewicz (2011).]
It isn't easy to see a way out of this DM-cul-de-sac, at least one that makes no further concessions to conventionalism -- or, indeed, one that doesn't require further unwelcome accommodations with Space-Time Absolutism. [On this, see Huggett et al (2021).]
That partly explains why, a few generations ago (in Stalinist Russia, for instance), philosophers and scientists found it difficult to square Einstein's theory with DM, and why several ended up rejecting the TOR. If (some) revolutionaries still seem unaware of these 'problems', STDs seventy or eighty years ago certainly weren't. [Cf., Graham (1971), pp.111-38; see also Joravsky (1961), Krementsov (1997), Vucinich (1980, 2001), and Wetter (1958). On this, see also Essay Eleven Part One, here and here.]
[STD = Stalinist Dialectician; TOR = Theory of Relativity.]
Once more, it could be objected that even if the above were correct, just as soon as a given object is set in motion (in a suitable inertial frame) it will be doing something contradictory. Or: the frame itself will be, since it will be moving with respect to other possible frames.
My reply to those rejoinders will occupy much of the rest of this Essay.
11. This isn't meant to single out Engels for special attention; it is equally impossible to determine what, if anything, Zeno, Hegel or Lenin were trying to say about motion.
However, if it is still maintained that systems of supposedly contradictory forces are responsible for the contradictions apparent in moving bodies, then it would be difficult to account for un-accelerated motion. Clearly, that takes place where no net forces are operating. If so, the exact source of the alleged contradictions (in such situations) would be even more obscure.
Of course, one consequence of this aspect of DM seems to be that there might be no un-accelerated motion anywhere in the universe. That is because:
(i) The opposite contention would involve a body possessing identically the same velocity from moment to moment, which would, of course, constitute an awkward concession to the LOI; or,
(ii) Any body so involved wouldn't be moving in a gravitational field, which, in this universe, isn't possible (so we are told).
Nevertheless, DM-inspired conundrums like this won't, I take it, worry genuine scientists too much, or for very long.
[LOI = Law of Identity.]
And all this is, of course, quite apart from the fact that such a DM-view of velocity (if such it may be called) will have to be imposed on nature.
But, as far as (ii) above is concerned -- given the additional fact that gravitational forces have been edited out of the picture in and by Relativity Theory (on that, see here) --, even if (ii) were to be ruled out, an appeal to such forces to account for acceleration would be no help, since there are none!
Moreover, in relation to (i), if a suitable frame of reference were chosen, any body could be said to have zero velocity and be undergoing acceleration for about as long as it takes hard-core DM-fans to abandon their criticisms of the LOI.
So, consider a body, B, moving at v kmph relative to the centre of mass of the Galaxy; let a reference frame for it also move at v kmph with respect to that centre of mass. In that case, B will have zero velocity with respect to that frame. The only response a DM-fan could make to this would it seems have to involve some sort of reference to 'abstractions' (i.e., in that this example involves the (implicit) use of "abstract identity"). That last ditch and rather desperate DM-defence will also be examined, and neutralised, in Essay Six.
The foregoing is quite apart from the fact that this example relates to the motion of a material object, and so it can't be a case of 'abstract identity'.
11a. Hegel's 'analysis' of Identity was partially covered here, and again (indirectly) throughout Essay Six; it will be examined more fully in Essay Twelve (summary here).
11b. That is because, if it is unclear what is being proposed -- as is the case with L9, given the convention introduced in L7 -- then nothing has yet been proposed.
L9: B is at (X1, Y1, Z1), at t1 and not at (X1, Y1, Z1), at t1, and B is at (X2, Y2, Z2), at t1.
L7a: (X1, Y1, Z1) is not the same place as (X2, Y2, Z2), nor is one contained by the other.
Of course, if L9 depicts one of the ambiguous cases mentioned already (that is, if B is in fact stationary -- like the car that was half in and half out of a garage), then it will be clear what is being proposed. But, in that case, L9 won't provide us with the required necessary and sufficient conditions for movement, and we would be right back at square one.
Anyway, in order to see if some sense can be made of what Engels was trying to say, I have ignored that annoying 'difficulty' for the present. However, I will return to it later, since it will soon become apparent that his theory can only be made to 'work' if we ignore the ordinary use of certain words and replace them with distorted 'philosophical' jargon --, as both Marx and Engels pointed out:
"The philosophers have only to dissolve their language into the ordinary language, from which it is abstracted, in order to recognise it, as the distorted language of the actual world, and to realise that neither thoughts nor language in themselves form a realm of their own, that they are only manifestations of actual life." [Marx and Engels (1970), p.118. Bold emphases added.]
Engels clearly forgot about the above warnings -- when he began to import Hegelian gobbledygook into Marxism, twenty-five years later!
[Why he changed in this way was explained in Essay Nine Part Two.]
This isn't a minor, or even a trifling, point; it is in fact central to understanding why Traditional Philosophers and dialecticians find they have to impose their theories on the world, and why the latter invariably collapse into LIE. [On this, see Essay Twelve Part One and Essay Two.]
12. A detailed discussion of these aspects of Zeno's 'analysis of motion' can be found in Angel (2002). See also, Note 19a and Note 24, below.
[This way of looking at the Reals is outlined in Newton-Smith (1980). On whether time is composed of 'instants', see Read (2007), pp.79-115. Indeed, time might not be composed of anything -- i.e., the word "composite" might not be applicable to it. I will put that knotty 'problem' to one side for now, too.]
12a. But Trotsky wasn't, of course, the only one to ignore this distinction. Engels also failed to consider the possibility that an object could be in two times for the same place -- i.e., in and not in one instant, at that place. But, if time advances while bodies move (or, indeed, while they remain still), and everything is contradictory, then this must surely be possible. And if that is so, what is to stop us saying that a moving body occupies the first place in one of these 'odd instants', and the next place in the second overlapping instant -- locating the alleged contradiction in time, and not in space or in motion? Or, perhaps, even eliminating both if we juggle around with each of these options until we get the 'right' result...?
Admittedly, it could be argued that if a body is in two times for the same place, it must be stationary.
In order to neutralise that response it might be wise to examine the subtle differences that exist between the following two sentences:
B1: Body, B, is in two different times for the same place.
B2: Body, B, is in the same place at two different times.
I don't propose to do that here, but it is worth noting that neither of these imply that the said object is stationary, since that object could still be moving and could return to the original location at a later time; hence, it could be in the same place at two different times. Think about circular motion, for example, or a moving object attached to a spring. Or even you, dear reader, returning home later in the day!
13. This is taken to be an important DM-assumption since it appears to be the only way that Engels's claims about the contradictory nature of motion can be defended, as argued at length in the main body of this Essay.
14. It is worth pointing out that L13 doesn't say that B is both at P1 and not at P1 at t. What it does say is this:
L13: For some B, for just one instant, t, for three places, P1, pi and pk, B is at P1 at t, but not at pi at t, and B is at pk at t (where pi and pk are proper parts of P1).
So, a finer-grained analysis of position allows for the fact that while at the macro-level an object might be located in one place (say, P1), in a given 'instant', at the micro-level it could still be in the same place (i.e., it will still be in P1), when it is also in one or other of the sub-spaces of P1 (for example, pk), at the same time. So, B could be in P1, and while not in all of P1 (i.e., not in, say, pi, which is also a part of P1) it would still be in P1 (in this case, in, say, pk, which is part of P1, too).
Hence, B could be in P1 at t, but not in every part of P1 at t -- and it could either be in motion or it could be stationary at that time -- meaning that it would be in two places at once: P1 and pk. So, if the location of bodies can be given in finer-grained detail -- even if that manoeuvre is inconsistently disallowed of time -- a body could still be in one place and not in it, and be in two places at once while remaining stationary, with no contradiction implied.
[I have given a more perspicuous example of this possibility in the main body of the Essay, concerning a ship in dry dock. This is the simplest of these cases; the reader is left to determine more involved examples for herself. The complex nature of ordinary, or even technical, language allows for the depiction of motion and location in ways undreamt of by Zeno, Heraclitus and Hegel -- or by Engels, Plekhanov and Lenin -- that is, in their 'philosophical' deliberations, not their everyday use of language. On that, see below and the main body of this Essay.]
Some might think the above ignores what Engels actually said, namely this:
"[A]s soon as we consider things in their motion, their change, their life, their reciprocal influence…[t]hen we immediately become involved in contradictions. Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it. And the continual assertion and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
However, as we saw earlier, it is far from clear what Engels actually meant by the following:
"...even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it." [Ibid.]
Here, Engels says that a moving object is "in one and the same place and not in it." He is clearly using "in" in a rather odd way, and it isn't too clear whether he (or any other dialectician) is capable of explaining how "in" used this way could be taken literally -- save, of course, they just label the results a 'contradiction', take their bat and ball home, and then retreat into a by-now-all-too-familiar dialectical sulk. But, that just highlights the problem: any such DM-response wouldn't make it an easier to decide what Engels was proposing, or even if he was proposing anything determinate, to begin with.
In that case, it is worth pointing out that in L13:
L13: For some B, for just one instant t, for three places, P1, pi and pk, B is at P1 at t, but not at pi at t, and B is at pk at t (where pi and pk are proper parts of P1),
B would be in P1 and not in it, in the following sense: it is in P1 but not in all of P1 at the same time. That is just as legitimate an interpretation of Engels's words as the traditional (but hopelessly obscure) version is.
This reading of his words might be contested on the grounds that, simply by fiat, it removes the contradiction from Engels's analysis.
But, we have just seen it isn't clear that what Engels's was trying to say is contradictory to begin with. That is because little sense can be made of his words as they stand. If we can form no clear idea what Engels was attempting to say, then based on what he committed to paper, it can't be concluded that it was an unambiguous or unequivocal depiction of a "contradiction".
"Motion itself is a contradiction; even simple mechanical change of place can only come about through a body being both in one place and in another place at one and the same moment of time, being in one and the same place and also not in it." [Ibid. Bold emphasis added.]
That is because we have already seen that extended bodies can be in two places at the same time -- even while they are stationary -- with no contradiction implied. If so, the 'contradictory' aspect of motion must arise from the following clause:
"...being in one and the same place and also not in it [at the same moment in time -- RL]."
Well, is this the contradiction we have been led all along to accept?
If so, imagine the following scenarios:
[1] NN is queuing for tickets and finds herself at the front of the queue at 11:55am (before the Ticket Office opens at noon). Unfortunately, she needs to leave the queue to visit the bathroom. So, she asks MM to act as her proxy in the queue while she is away, which she does at 11.57am. Here, NN is both in and not in the queue at 11:57. She is still in the queue since MM is guarding her place, but she isn't in the queue since she has left to visit the bathroom. Here we have a moving body/individual, NN, who is both in the queue and not in it at the same time, with no contradiction implied. Plainly, this only appears contradictory because of the equivocal meaning of "in" used here.
[2] NM is selected by his coach to play for the first team. The latter leaves for the next match at 15:00 hours the day before kick-off but, unfortunately, NM misses the bus. So, NM is in the team (the coach hasn't dropped him), but not in the team (since he isn't accompanying them), at the same time -- and that could be true whether or not NM is moving. [Some might want to point out that a team is not a place, but quite apart from the fact that Engels was unclear what he meant by "place", we often speak about a player securing or gaining their "place in the team".] Here, once again, the equivocation centres on both "in" and "place", which together have manufactured yet another bogus 'contradiction'.
[3] Consider next, a Klein Bottle:
Figures Ten And Eleven: The Non-Dialectical Klein Bottle
Consider an object sliding down the central tube running through the bottle. This object then stops at some point. Now, because the inside of this bottle is the same as the outside, that object will be inside and outside (i.e., it will be inside and not inside) the bottle at the same moment; hence, it would be in and not in a given place at the same time. And, that would be the case whether or not the said object was still moving. Here, the equivocation centres on "same place", i.e., whether "inside" can be the same as "outside" in certain circumstances. In an ordinary sense the object will either be inside or outside this bottle, but that wouldn't be the case in a mathematical sense. A somewhat similar ambiguity also features in example [5], below. Hence, here we have yet another fake 'contradiction' (and one that has zero to do with motion).
An analogous 'contradiction' can be manufactured for a stationary object on the surface of a Möbius Strip -- or even worse, on a set of Möbius Gears:
Figure Twelve: A Möbius Strip -- Concocted By The CIA?
Figure Thirteen: Möbius Gears -- A CIA Invention?
Video Three: And, As If To Rub It In, Möbius Gears
In Action
[See also here. A stationary object on these gears would be both on top and not on top of one surface, at once. So, equivocations like this aren't confined to the use of the preposition "in".]
[4] MN has had her amputated arm replaced by a prosthetic limb. At 02.20pm on Friday September 9, 2023 she inserts the artificial hand part of the limb into a glove. Hence, her hand is both in and not in that glove. It is in the glove in the sense that this is her hand now, but it isn't in the glove in the sense that it isn't a real hand, the hand she was born with. Here the equivocation concerns "same object" -- or, in this case, "same hand".
[5] NN is in the corridor of a hotel outside her room at 17:30 hours on the above Friday, but she is also inside the hotel at the same time. So, she is outside and inside (or outside and not outside), all at once -- she is inside the hotel but outside her room. There are countless examples of this use of prepositions and adjectives. For example, a book on a shelf may be above the floor but below the roof. So, it is at one and the same time above and not above. A box might be stored near a wall but far from the door; so, at one and the same time it is both near and not near. Ancient Greek Philosophers made much of these equivocations -- as, indeed, do DM-theorists. [In fact, Plato's dialogue, Parmenides, is full of equivocations like these -- i.e., Plato (1997b).] Here we have apparent contradictions all over the place, even though the items in question could be stationary with respect to some inertial frame.
It might be objected that not only are these examples highly artificial, they are all forced. Clearly, they are not at all what Engels had in mind.
Or, so it might be argued...
But, as we have seen (several times!), it isn't at all clear what Engels 'had in mind'. Anyway, only [3] is (even arguably) artificial. None of them are forced; they all depict situations with which we are all familiar, some on a day-to-day basis, too. Who among those reading these words has not stood outside a room inside a hotel? Who has never heard of a team member missing a bus/train/plane, but not being dropped as a result? Of those who haven't, who thinks such things never happen? Who thinks that no one has ever asked anyone (ever) to save their place in a queue? Who has never heard about artificial limbs, or artificial hands/feet being inserted in gloves, socks or shoes?
The examples mentioned in [5] above aren't artificial, either. There are countless instances of this sort of equivocation right across the planet (nay, right across the universe). So, Pluto is both near the Earth (compared to that dwarf planet's shortest distance from Proxima Centauri), but it is also far from (i.e., not near) the Earth (compared to its shortest distance from Neptune). Hence, Pluto is both near and not near the Earth. Does anyone in their left mind think this is a contradiction, never mind a 'dialectical' one?
The following example isn't forced, either; it concerns a story (aired by the BBC) concerning the 3000 metre steeplechase final at the 2014 European Games:
"France's Mahiedine Mekhissi-Benabbad has been stripped of his 3,000m steeplechase gold medal at the European Championships for taking his shirt off on the home straight. Mekhissi-Benabbad put his top in his mouth after pulling clear of the field. Initially he appeared to be shown a yellow card by an official but was subsequently disqualified. Frenchman Yoann Kowal now wins gold, Poland's Krystian Zalewski gets silver and Spain's Angel Mullera wins bronze." [Quoted from here. Accessed 15/08/2014. Paragraphs merged.]
As a result of this, Yoann Kowal was moved from second to first, Krystian Zalewski from third to second, and Angel Mullera from fourth to third, even while none of them were in two places at once, in one and not in it at the same time. So, these Olympic officials moved and did not move Yoann Kowal. He is and he isn't winner of the Gold Medal at these Games; he was both in and not in first place. He is in first place in the sense that he has now been declared winner, but he isn't in first place since he didn't cross the winning post ahead of everyone else.
The above story isn't a one-off, either. It happens quite frequently in sport these days (when those who cheat by taking performance enhancing drugs are found out). Here is a recent example:
"Russia's Natalya Antyukh has been stripped of 400m hurdles gold from London 2012 on the basis of historical data from a Moscow testing laboratory. Antyukh, now 41, is already serving a four-year ban after being named in a World Anti-Doping Agency (Wada) investigation into cheating by Russia. American Lashinda Demus will be promoted to gold in her place. All three gold medals won on the track by Russian athletes at London 2012 have now been rescinded on doping grounds. Mariya Savinova and Yuliya Zaripova, the initial winners of 800m and 3000m steeplechase gold, have been disqualified. Ivan Ukhov's high jump title and Tatyana Lysenko's hammer victory in the field have also been wiped from the record books....
"Antyukh has struck a defiant tone on social media. Her last Instagram post on 18 August is a photo of her showing off her silver and bronze medals from the 2004 Olympics. Those medals remain unaffected by the latest AIU decision. The International Olympic Committee (IOC) can now promote Jamaica's Kaliese Spencer to the bronze medal position after Antyukh did not appeal against her punishment, with Czech Republic's Zuzjana Hejnova in line for an upgrade to silver." [Quoted from here; accessed 08/10/2023. Links in the original; several paragraphs merged. Bold emphases added.]
Are any of my readers taken in, or even puzzled for one second, by these 'contradictions'? That is, does anyone scratch their head wondering how someone can be moved from second to first place without actually moving? Or, how someone can 'change places' while remaining perfectly 'motionless'? If so, I have a nice bridge in Brooklyn to sell you...
Moreover, as we will discover: in the abstract, while Engels's 'theory' might seem (to some) to be eminently sound, when we look at concrete examples (like those above, or even those set out later in this Essay), his words can be seen for what they are: artificial and forced, themselves.
[For more examples like the above, see here, Note 16 and the main body of this Essay.]
14a. It could be objected that (X1, Y1, Z1) is a mathematical point, so it can't have other points located inside it. Hence, it can't be the case that (xk, yk, zk) and (xi, yi, zi) are both located inside (X1, Y1, Z1).
That 'problem' is easily resolved:
L13c: A stationary body, B, observed over the course of an instant, is located in a finite region, ℛ, and at (xk, yk, zk), but not at (xi, yi, zi), where (xk, yk, zk) and (xi, yi, zi) are both located inside ℛ.
L13d: A moving body, B, observed over the course of an instant, is located in a finite region, ℛ, and at (xk, yk, zk), but not at (xi, yi, zi), where (xk, yk, zk) and (xi, yi, zi) are both located inside ℛ.
It could also be stipulated that ℛ is a volume interval; the other points, (xk, yk, zk) and (xi, yi, zi), could be replaced by volume sub-intervals, too.
14b. It might be objected that the ship example is ridiculous since the places occupied by the ship (while in the said port) don't lie along the same line. Engels comments about motion clearly imply that a moving body will do so along a given trajectory. Such a moving object wouldn't be all over the place, which is what this ship homily clearly suggests.
Or, so it could be argued...
However, if Engels were only referring to rectilinear motion (i.e., motion in a straight line), much of the movement in the rest of universe wouldn't be covered by his theory, since there is very little, if any, of that outwith this planet (so far as we know). And if we take into consideration the curvature of the earth, there is precious little even on this planet!
Having said that, it is highly implausible to suggest that Engels was only referring to rectilinear motion. Hence, if he wasn't, no adjustment need be made to the argument presented in the main body of this Essay (concerning that ship).
Anyway, the objection itself has been neutralised here, where the ship example has been translated into vector algebra.
Less technically, if it turned out that Engels was speaking exclusively about rectilinear motion, we would only need add an additional codicil to the example given in the main body of this Essay -- to the effect that the relevant parts of the aforementioned port all lie along a single straight line.
15. The material that used to be here has now been moved to the main body of this Essay.
16. This observation would remain true even if such 'spatial location sentences' employed co-ordinates (expressed perhaps by real number triples). However, as we are about to discover, technical specifications like this aren't free from ambiguities of their own.
An example of just such an equivocation might be the following: in <x1, y1, z1> and <x1, y1, z1> (that isn't a typo!), each variable letter is in the "same place" -- i.e., they are even though typographically identical variable letters have been used (twice), each is situated in its respective ordered triple, in first place, second place and third place, while also being in a different place on the page or screen, at the same time.
Indeed, not only are the above letters in the same place, they are also in different places while they are in the same place (i.e., they are on your screen and mine -- and, clearly, we and they are both in the solar system, and in The Milky Way, and...), at the same time!
Consider, too, this variation on the same theme: <x1, y1, z1> and <y1, z1, x1> (that isn't a typo, either!); here, each letter is in the same place (they are on the same page or screen -- or they are even in geographical location -- as one another), and yet each letter is in a different place from the other elements relative to the rest. So, each letter is and is not in the same place.
Did anyone see anything move?
Now, just try saying any of that in Hegel-speak!
Again, the above would be 'contradictions' only in the mind of the radically confused, the easily bamboozled or the irredeemably purblind.
From this is should now be reasonably clear that the use of highly technical SCs doesn't rid (even) such language of ambiguity.
[SC = Spatial Coordinate.]
Moreover, these are very simple examples! I won't do so here, but it would be possible, if not all that easy, to construct examples that are genuinely difficult to follow, since they would involve much greater detail, using increasingly complex equivocations buried in iterated layers of relative clauses. But, with sufficient concentration and determination it would be possible to grasp their content, all of which would make the same point: that it is ridiculously easy to manufacture 'contradictions' to order using equivocal language, whether the latter is ordinary or technical.
[Of course, with respect to formal languages, the equivocations emerge only when they are translated into ordinary language (unless any of the relevant definitions -- if there are any! -- were defective to begin with). Despite this, no one working with ordered n-tuples, for example, would regard them as 'inherently contradictory'. (In fact, in Essay Six I have posted several examples that are analogous to the above, but expressed in words that are normally associated with identity and difference.)]
To paraphrase Wittgenstein: the conventions of ordinary language are exceedingly complex. Dialecticians ignore them at their non-dialectical peril.
17. Some might try to claim that this is because ordinary language is defective (at least, when used in certain areas -- for example, those connected with change and development); cf., TAR pp.45-50.
However, the view that ordinary language is in any way defective isn't shared by the present author; the opposite is in fact the case. This topic will be addressed in detail in Essay Twelve Part Seven. [Until that is published, a summary of what will be argued there can be accessed here.]
18. At this point we appear to have reached linguistic bedrock. This means that in order to proceed we will have to question, revise or reject certain fundamental linguistic conventions -- or, alternatively, invent new rules. In the present case, for instance, we would need to promulgate a non-symmetrical stipulation concerning the (presumed) 'infinite' divisibility of space and time -- allowing the former but not the latter. So, this latest "must", in the main body of the Essay, would in effect be little more than a rhetorical equivalent of thumping the table.
18a. This particular 'assumption' (repeated below) is a key part of the theory that motion is an 'inherent property' of moving bodies.
L15: If an object is located at a point it must be at rest at that point.
This certainly appears to be the implication of Graham Priest's interpretation of Hegel's views on this subject. [Priest (2006), pp.175ff.] The most obvious problem with such a theory is that a body can be moving in one reference frame while stationary in another. Hence, the idea that there is something 'inherent' (or 'intrinsic') to moving bodies seems to rely on the further assumption that space is Absolute!
Priest, however, denies he is committed to an absolutist interpretation of space and time (ibid., p.172), but it is difficult to see how his theory can avoid absolutism in this regard. I can find nowhere in his work where he tackles this problem.
First of all, it is worth pointing out that much of what Priest has to say about the (supposed) 'contradictory' nature of motion is susceptible to many of the objections raised in this Essay -- such as those involving the numerous ambiguities surrounding words that have traditionally been used by theorists working on this 'problem' (such as "move", "place", "time", and "instant", etc., etc.). As is the case with several of the other terms usually employed in this area, Priest just helps himself to many of them and rarely, if ever, considers the sort of equivocations highlighted in this Essay. So, it is hardly surprising that he, like Hegel, Engels and all the rest, finds 'contradictions' popping up all over the place (no pun intended).
Second, one of the problems presented by Priest's work (especially to those new to modern logic) is that, like so many other mathematical logicians, he uses non-standard symbols. For example, instead of expressing a contradiction in the more usual way as, "p & ¬p", he uses expressions like this: "α & ¬α". He then often compounds the problem by peppering his books and articles with other non-standard Greek symbols. For instance, instead of labelling four possible alternatives connected with what he calls "the instant of change", "A", "B", "C", and "D", he labels them "A", "B", "Γ" and "Δ" (where the first two letters are in fact the capital versions of α and β). It isn't too clear why he does this -- except it might make his work look more technically sound and sophisticated (or perhaps, more confusing and intimidating!) to less experienced eyes than would otherwise be the case if he employed the usual symbols.
[Priest in fact uses the logical symbol "Λ" in place of "&" employed in this Essay. I have employed the latter symbol throughout since it is easer to access on my computer. Admittedly, I also use Greek symbols from time-to-time, but only where they are already in standard use on logic (so that those who read, for example, commentaries on Frege, etc., aren't thrown by their appearance). Elsewhere, I endeavour to employ the usual symbols wherever possible. Unfortunately, that can't be said of Priest.]
Far worse, though, is this: his analysis of motion leads to a fatal infinite regress (and readers will soon see why I made the second of the above two points when they try to understand the following quote -- which is also why I have endeavoured to translate it into more ordinary terms straight after):
"We have seen that a certain kind of change from α holding to β holding produces a nexus where α & β holds. We may, however, go a step further. We may take the nexus state produced to be the state of change itself. The state described by α & ¬α just is the state described by α changing into the state described by ¬α. Thus, there is such a thing as the state of change, and it does take time, if only an instant.... Not only is there a state of change that takes time, but it commences while the prior state obtains and terminates only after the posterior state has begun." [Priest (2006), p.170. Bold emphases added. I have to say, this isn't the clearest thing Priest has ever written!]
Translated, this reads as follows:
"We have seen that a certain kind of change from α happening to β happening produces a combination where α & β both happen together. We may, however, go a step further. We may take the combined state that has occurred to be the state of change itself. The state described by α & ¬α just is the state described by α changing into the state described by ¬α. Thus, there is such a thing as the state of change, and it does take time, if only an instant.... Not only is there a state of change that takes time, but it commences while the prior state obtains and terminates only after the posterior state has begun."
So, the above is supposed to describe any change from a state depicted by α to one depicted by not α (i.e., ¬α); but, in order for that to be the case, there must be an instant when both α and not α obtain together. Priest calls the occurrence of α and not α together, "the state of change".
[Notice, however, that not even Priest tells us how long one of these "instants" is supposed to last!]
However, if these three states are α, α & ¬α, and then finally ¬α (illustrated by P2, below), and if all change follows this pattern, the change from α to α & ¬α must obey this pattern, too:
P1: (i) α, (ii) α & ¬α, and (iii) ¬α.
The general point (no pun intended) appears to be that in order to pass from one state to another, where the second is the negation of the first, there must be an intermediate state where the system involved is in both states at once. So, for state, A, to pass over into state, B (where B is depicted by the negation of any proposition depicting A) there must be a stage where both A and B occur together. So, if, as before, α is the proposition depicting A and ¬α is the proposition depicting B, then the intermediate stage, A and B, must be depicted by α & ¬α.
But what about the transition from state, A, to state, A and B? Does that not also need an intermediate stage? It seems it must if this is to count as a model that applies to all such change. In that case, there must be an intermediate stage which is the negation of A and B. Call it C -- where C is ¬(α & ¬α). So, the move from α to α & ¬α must be mediated by ¬(α & ¬α).
The same now applies to that transition, too. It must have its own intermediate stage between α and ¬(α & ¬α), namely, (α & ¬(α & ¬α)). Hence, the transition from α to α & ¬α must itself go through an (α & ¬(α & ¬α)) stage, otherwise Priest's analysis is fundamentally flawed. That is, we must have these stages/states in the transition from α, to (α & ¬α) -- via (α & ¬(α & ¬α)) -- on one interpretation of his theory, namely:
P2: (i) α, (ii) (α & ¬(α & ¬α)), (iii) ¬(α & ¬α), (iv) (α & ¬α), (v) ¬α.
But, given Priest's analysis, if we concentrate on the change of (α & ¬(α & ¬α) into ¬(α & ¬α), that must also go through an intermediary stage of its own: (α & ¬(α & ¬α) & ¬¬(α & ¬α))! Here is part of that change:
P3: (i) α, (ii) (α & ¬(α & ¬α)), (iii) (α & ¬(α & ¬α) & ¬¬(α & ¬α))...
However, the real problems actually kick in much earlier. As we have just seen, if α changes into ¬(α & ¬α), it can only do so through an intermediary state, (α & ¬(α & ¬α)). But, if there is change here, too, we must also have the following as α itself changes into (α & ¬(α & ¬α)). That is, it must also go through yet another intermediary state, (α & ¬(α & ¬(α & ¬α))), yielding:
P4: (i) α, (ii) (α & ¬(α & ¬(α & ¬α))),...
But, the same applies to the transition from α to (α & ¬(α & ¬(α & ¬α))); it, too, must go through its own intermediary state, (α & ¬(α & ¬(α & ¬(α & ¬α))))..., and so on as intermediary stages multiply, ad infinitem:
P5: (i) α, (ii) (α & ¬(α & ¬(α & ¬(α & ¬α))))...
P6: (i) α, (ii) (α & ¬(α & ¬(α & ¬(α & ¬(α & ¬α))))...
P7: (i) α, (ii) (α & ¬(α & ¬(α & ¬(α & ¬(α & ¬(α & ¬α))))))...
P8: (i) α, (ii) (α & ¬(α & ¬(α & ¬(α & ¬(α & ¬(α & ¬(α & ¬α)))))))...
This is a fatal infinite regress, since, as I argued in Essay Seven Part Three -- in relation to the idea that dialectical contradictions are actually based on a 'tendency to change' -- this theory implies tendencies within tendencies within tendencies...and so on, forever. [On why that is so, follow the above link.] But, that would lead to the universe grinding to a halt within a faction of a second of it having started (if it did); here is why:
But, do these inner inner "tendencies" go on forever, as a series of "tendencies" within "tendencies" within "tendencies"? It seems they must if all change -- including each and every change experienced by such "tendencies", morphing them into whatever they become -- is a result of "internal tendencies". If not, these "tendencies" themselves would be forever changeless (since they would not have their own "inner tendencies" to bring such changes about) -- if the DM-classics are to be believed.
This seems to imply that every single change must involve a potentially infinite number of "tendencies" within "tendencies" within "tendencies". Let us suppose it does imply this, and that each interaction between these inner "tendencies" takes, say, 10-10 seconds to act in the way they do (i.e., each one takes one ten-billionth of a second to act). Let us further suppose that there is a series of, say, 10100 of these "tendencies" within "tendencies" within "tendencies". Now, even though this number is huge (i.e., it is one followed by a hundred zeros, and it is even called a Googol), it is way short of infinity. But, let us suppose there is this number of inner, inner "tendencies" involved in each 'dialectical' change involving an object or process developing into its opposite (i.e., A into not-A). If each such change (to these inner, inner "tendencies") takes 10-10 seconds to complete, then any individual such change will take 10-10 x 10100 = 1090 seconds to complete. If a year is 60 x 60 x 24 x 365 = 31,536,000 seconds, then each such change will take 1090/31,536,000 = 3.171 x 1083 years -- that is, approximately 3 followed by 83 zeros, years! If we now take into consideration the latest estimate for the age of the universe -- at approximately 14 billion years (that is, fourteen followed by nine zeros) --, then each 'dialectical change' -- even assuming there isn't an infinite number of these inner, inner "tendencies" -- would take approximately 2 x 1073 (i.e., 2 followed by 73 zeros) times longer than the entire length of time that has elapsed since the 'Big Bang'!
On the other hand, of course, an infinite series of these inner, inner "tendencies" will take an infinite number of years to complete. The 'dialectical' universe would grind to a halt just as soon as it 'began'.
If each of the above 'tendencies' is replaced by one of Priest's 'intermediary stages', his theory would see the universe grinding to a halt!
Priest attempted to block this infinite regress with a comment he relegated to a footnote(!) -- perhaps hoping no one would notice it if it was half-buried:
"If we suppose there to be states of change, does this not start an infinite regress? For what if the change between, e.g. the prior state described by α, and the state of change, described by α & ¬α? There is no infinite regress. The nexus state between these two states is described by α & ¬(α & ¬α), i.e. ¬(α & ¬α), which is the original nexus state. Thus to be changing into a state of change is already to be in that state of change, as one might expect." [Priest (2006), pp.170-71, ftn.17. Bold emphases added.]
But, if all change is to be modelled on this pattern (outlined in the 'general point' a few paragraphs back), then it won't do to ignore the change from one state to each intermediary state, howsoever they are described or labelled. It certainly won't do to try and block this with the following bluff response: "Thus to be changing into a state of change is already to be in that state of change", since if that changed stage is itself a state of change, then any transition to it from whatever went before must also be governed by this overall process, involving its own 'contradictions'. So, if there is to be development from a state that isn't a state of change into one that is a state of change, there has to be a conjunction between propositions depicting what went before and what is about to follow -- i.e., there has to be an A and B, even here. Simply labelling a certain state a "state of change" in no way explains how that "state of change" came about, nor does it exclude or exempt it from the process itself. If such a "state of change" wasn't there before, and now it is, there must be transitional state intermediate between it and whatever preceded it.
In fact, if this were the case: "To be changing into a state of change is already to be in that state of change", there would be no change, unless we were told how that setup itself changed from a state that isn't changing to one that is. If Priest wants to exempt such a change from his own model of change, he can hardly complain if others exempt every instance of change from this model, too. Hence, if this model fails at each moment of change, or if it can't be consistently applied to such, then it fails everywhere or can't be applied anywhere. On the other hand, if the change from no change to a state where there is change is itself abrupt, and doesn't pass through an 'intermediary contradictory state of affairs', then all change must be like this, too. Alternatively, once more, if the change from no change to a state where there is change itself passes through an 'intermediary, contradictory state of affairs', then that change must either be abrupt, involving no contradiction, or it must involve its own intermediary contradictory state, implying an infinite regress. But, as we have just seen, that would see the process grinding to a halt.
Either way, such changes are (a) abrupt and hence non-contradictory or (b) they lead to an infinite regress (and a 'frozen universe').
In other words, Priest can't in the end explain change, and all his careful and convoluted work in this area is just so much wasted effort -- indeed, as one would expect of anyone who unwisely takes logical and philosophical advice from a Christian Mystic.
[All this is, of course, in addition to the other logical confusions that have been highlighted by Priest's critics.]
However, far more important for Dialectical Marxists are the following questions: Even if Priest's theory was 100% valid, are any of the above changes/contradictions 'dialectical'? If they aren't then there would seem to be no point to any of this. In that case, do these states of affairs struggle with and then change into each other, as we are told they must by the DM-classics? If they do, does that mean α & ¬α struggles with and then changes into α?
If so, every such change will in fact go backwards!
If not, Priest's theory is of no interest to Dialectical Marxists, since it violates core principles expressed in and by the aforementioned classics.
Further questions force themselves upon us: Do these changes imply one another such that neither can exist without the other (like the proletariat can't exist without the capitalist class, so we are told)? That must be the case if these stages are to count as 'dialectical opposites', so that any 'contradiction' involved is 'dialectical'. As far as I can tell, Priest is silent about this. Indeed, it isn't easy to see how any of these considerations could be the case given Priest's account of motion.
Anyway, and once again, there doesn't seem to be any point to this because these 'contradictions' play no causal role in motion. [I have dealt with that 'dialectical difficulty' more extensively here; readers are directed there for more details.]
Finally, what Priest has to say appears to contradict Engels:
"Among natural scientists motion is always as a matter of course taken to mean mechanical motion, change of place. This has been handed down from the pre-chemical eighteenth century and makes a clear conception of the processes much more difficult. Motion, as applied to matter, is change in general.... The first, simplest form of motion is the mechanical form, pure change of place: (a) Motion of a single body does not exist -- [it can be spoken of] only in a relative sense -- falling." [Engels (1954), pp.247-48. Italic emphasis in the original.]
If the "motion of a single body does not exist", it can't be an inherent property of that body.
[Further comments about other relevant parts of Priest's theory will be included in a future re-write of this (or another) Essay, as will several others concerning Marquit (1978, 1982); that is, in addition to a few remarks about the views of other dialecticians who have expressed significant, or even relevant, opinions on this topic. I have commented on some of Lenin's remarks about motion in Note 18b1, below.]
18b. L15 is taken to mean:
L15a: If an object is wholly located at a point it must be at rest at that point.
It is also assumed that for such an object to be stationary it must be located at this point (and nowhere else) over a finite interval of time -- i.e., L15b is assumed to be the case:
L15b: If an object is wholly located at a point for at least two contiguous moments in time, it must be at rest at that point.
Or perhaps, more colloquially:
L15e: If an object is wholly located at a point for a while, it must be at rest at that point.
L15f: If an object is wholly located at a point for a few moments, it must be at rest at that point.
18b1. Lenin had the following to say about this particular option:
"Movement is the presence of a body in a definite place at a given moment and in another place at another, subsequent moment -- such is the objection which Chernov repeats (see his Philosophical Studies) in the wake of all the 'metaphysical' opponents of Hegel. This objection is incorrect: (1) it describes the result of motion, but not motion itself; (2) it does not show, it does not contain in itself the possibility of motion; (3) it depicts motion as a sum, as a concatenation of states of rest, that is to say, the (dialectical) contradiction is not removed by it, but only concealed, shifted, screened, covered over." [Lenin (1961), p.257. Italic emphases in the original; bold added. Quotation marks altered to conform with the conventions adopted at this site.]
Lenin here talks about "motion itself", but how he knew what "motion itself" amounted to he annoyingly kept to himself. Unfortunately for him, as we will see, there are plenty of examples of motion that actually make what this character, Chernov, had to say true. [On that, see Note 18c.]
Anyway, the point is that Lenin rejected the idea that motion is a "concatenation of states of rest", which means that the assumed truth of L16 underpins his understanding of 'dialectical motion', and possibly also of 'motion itself':
L16: Hence, a moving body can't just be located at a point otherwise it wouldn't be moving, it would be at rest.
But in support of this claim did Lenin even think to quote a single scientist, or, indeed, someone who might be qualified enough to conduct a few controlled experiments or perform a series of careful observations? Not a bit of it. Instead, he quoted that notorious non-scientist and Christian Mystic, Hegel, as if he were some sort of expert!
[I have reproduced the whole passage from Hegel that Lenin only partially quoted.]
"If we wish to make motion clear to ourselves, we say that the body is in one place and then it goes to another; because it moves, it is no longer in the first, but yet not in the second; were it in either it would be at rest. Where then is it? If we say that it is between both, this is to convey nothing at all, for were it between both, it would be in a place, and this presents the same difficulty. But movement means to be in this place and not to be in it, and thus to be in both alike; this is the continuity of space and time which first makes motion possible. Zeno, in the deduction made by him, brought both these points into forcible opposition. The discretion of space and time we also uphold, but there must also be granted to them the over-stepping of limits, i.e. the exhibition of limits as not being, or as being divided periods of time, which are also not divided. In our ordinary ideas we find the same determinations as those on which the dialectic of Zeno rests; we arrive at saying, though unwillingly, that in one period two distances of space are traversed, but we do not say that the quicker comprehends two moments of time in one; for that we fix a definite space. But in order that the slower may lose its precedence, it must be said that it loses its advantage of a moment of time, and indirectly the moment of space." [Hegel (1995), pp.273-74, partially quoted in Lenin (1961), p.257. (The editors of Lenin's text have clearly used a slightly different translation of this passage.) Bold emphasis added.]
[An attempt has been made to clarify (i.e., reduce the obscurity of) certain aspects of Hegel's argument in lines L18-L27 in the main body of this Essay.]
[Lenin had plenty more to say about Hegel and Zeno (and the latter's paradoxes) in the surrounding pages of PN, but much of it isn't relevant to the aims of this Essay so I will say no more about it.]
Here, though, is John Somerville, making heavy weather of the idea that motion is a contradiction (also failing to tell us how long one of these "instants" lasts):
"Implicit in Engels' reasoning is the conception that a moment of time, even the tiniest instant, is a duration, an interval during which something happens. Time is obviously a flow, in which things happen. If we could not say that much, then we would not be able to say that the world (or the self) had a history. In that case there probably would be no problem. The problem arises after we have perceived that there are events, happening in sequence. If this is real, what follows? It seems quite clear that if time were made up of moments or instants during which or at which nothing happens, then nothing would happen or move, as Zeno saw long ago. However, if things do happen and move, it must be that even the smallest instant or interval of time is a flow. What Engels is saying, then, is that motion of anything is a process during which the moving thing is both at a given point and beyond that given point (in the sense of 'being in one and the same place and also not in it,' not simply in the sense of one part being in and another part not) during the smallest possible instant of time; and that if this were not possible, then there would be no motion.
"Now this assertion, that moving body X is simultaneously (during the same instant of time) at point Y and not at point Y, is recognized by all concerned to be a contradiction, as formal logic construes contradiction. But if body X could not manage that, then it could never move, since the only other alternative would keep it at point Y, or some other point, at every nonflowing instant. If all instants were instants at which a given thing is stationary, it is obvious there could never be a time at which anything could move, as time is by definition made of instants. The alternative is that time is made up of instants during which a change takes place, which means the possibility of a simultaneous A and non-A, the possibility of the same part of X simultaneously being at Y and not being at Y. Strictly speaking there are no instants at which, only instants during which. (But among these are instants during which the changes taking place in A make no difference to a certain problem, instants during which the motion of X makes no difference in relation to a certain point of view and a certain scale of measurement.)" [Somerville (1968), pp.66-67 -- these are in fact the page references to the 1974 reprint. Quotation marks altered to conform with the conventions adopted at this site. Bold emphases alone added.]
I think Max Black manages to cut through the confusion manifest in what Somerville and Engels were trying to say (always assuming, of course, that Somerville succeeded in interpreting Engels correctly, which, as we have seen, isn't as easy and straightforward as many appear to think):
"It would be startling indeed if examination of experience could show that the traditional principles of logic were sometimes false. Somerville's argument to this effect does not inspire confidence. He cites, with approval, the hackneyed and muddled text from Engels in which it is alleged that 'motion is a contradiction' and that a moving body is constantly 'asserting' and simultaneously 'solving' this contradiction. That an inanimate body should assert anything at all does not strike Somerville as being odd. At any rate, he himself is prepared to assert that the moving body 'is simultaneously (during the same instant of time) at point Y and not at point Y.' Well, what does Somerville mean by an 'instant'? If he really means an instantaneous moment, then he really is contradicting himself, in the most elementary fashion.... But, if by 'instant' he means a small interval of time, then, of course, there is no logical contradiction in saying that during such an interval a moving body will at one instant be at Y and at another instant during the same interval will not be at Y.... If this is the kind of reasoning that 'dialectical logic' encourages, the chief function of that pseudodiscipline would seem to be to facilitate conceptual confusion.... It is time to let the ghost of Engels rest." [Black (1974), pp.76-77. Quotation marks altered to conform with the conventions adopted at this site. Italic emphases in the original; paragraphs merged.]
As Black points out, there is no contradiction if these Engelsian 'moments' are temporal intervals. On the other hand, they would be mathematical abstractions if they really were actual instants, and if that were the case, there would be no "before" and no "after" applicable here, hence no "during", either (as we have already seen, here and here -- but see also Note 22b, below).
Be this as it may, let us suppose Somerville is 100% correct, and that this is indeed a 'formal contradiction'; what he has yet to show is that it is also a 'dialectical contradiction'. But, he doesn't even attempt to do that. Nor did Engels, Plekhanov and Lenin! As far as I know, no other DM-fan or Engels's interpreter has thought to do so, either.
They don't even ask the relevant questions, for goodness sake!
[Such as those posed earlier in this Essay -- here and here, for instance.]
The same can be said about Hegel and his epigones/'commentators'.
[If anyone thinks differently -- i.e., if anyone knows of a single theorist (Hegelian or Marxist) who has ever even tried to show this is a dialectical contradiction (never mind succeeding!), as opposed to assuming it is an ordinary contradiction -- email me with the details. After over thirty years of looking, I have been unable to find even one.]
18c. In fact, there have been important theorists who have argued that motion is discontinuous, a 'stop-go', staccato sort of affair. For example, Gassendi and the early Leibniz.
[Although, as with anything one asserts about Leibniz's philosophical views, the above needs to be heavily qualified. On Leibniz's arguments for the discontinuity of motion and his debt to Gassendi, see Leibniz (2001), pp.xxvii-xxix, lxxix, 77-83, 93-99, 159-63, 169-73, 187-203. See also Wilson (1989), pp.77, 169-70, 205. On the general background to this aspect of Leibniz's work, see Mcdonough (2024). For everyday examples of discontinuous motion -- that Hegel groupies totally ignore, as do DM-fans -- see here.]
Leibniz argued that if motion were continuous, it would be impossible to explain faster or slower speeds. If speed is the number of points a body traverses along its trajectory in a given unit of time, an increase in speed would involve that body traversing more points in the same temporal interval. But, the number of points in a body's trajectory is infinite; if so, it can't traverse more points in the same space of time, since all such infinities are equal (i.e., in modern parlance, they have the same cardinality). The only way to account for different speeds -- given this view of trajectories and infinities -- is to argue that at a lower speed a body rests at each point a bit longer, and the opposite when it moves faster. [Leibniz connected such observations with the theory that motion is in fact illusory!] To be sure, we now think we know more about the nature of infinity than philosophers and mathematicians in Leibniz's day (i.e., following on from Cantor's work), but I suspect that Leibniz would have seen through this spurious area of modern mathematics reasonably quickly (but not necessarily along the lines set out here).
[On this, incidentally, see Gefter (2013).]
Moreover, there are versions of the Block Theory (involving the so-called B-Theory of Time and Perdurantism) that imply motion is, indeed, illusory, and only appears continuous because of our 'subjective' perception of the passage of time.
[On this, see Hawley (2004, 2020), although I am not suggesting that Hawley has adopted this particular interpretation of that family of theories. For a different take on this, see Gallois (2003). On the complexities underlying our perception of both rapid and slow motion, alongside the complexities involved with 'zoëtrope motion', see Phillips (2011).]
For a preview of how I would respond to metaphysical theories of time like this (that is, if this were the main topic of these Essays, which it isn't!), see here and here -- but, in general, here (summarised here).
19. On this, see Note 18c, directly above.
19a. Of course, when they have been laid out in all their glory, these and other premisses, assumptions, 'proofs' and conclusions are far more complex than this Essay might otherwise appear to suggest. [Hegel's actual argument can be found in Note 18b1.] However, since this Essay isn't meant to be an academic exercise, nor is it intended to be a contribution to 'academic Marxism', what I take to be the most relevant assumptions have been stripped down to their 'bare essentials', so to speak.
Readers who want to study this topic in more detail should perhaps begin with Mazur (2007), Angel (2002), Blay (1998), and Huggett (2018). Also see Note 12 and Note 24. On the various infinites and 'paradoxes' of the Reals, and much else besides, see Hunter (1996), Lavine (1998), and Moore (2001, 2011). For more traditional approaches to this 'paradox', see Grünbaum (1967) and Salmon (1970).
20. The status of these indicative sentences (i.e., L28 and L29) will be left somewhat vague for the time being. [However, this topic is connected with another set of ambiguities discussed in later sections of this Essay.]
Nevertheless, in the main body of this Essay, the following were taken to be contradictories:
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L29: A body can be at rest and in motion at the same time in the same inertial frame.
Strictly speaking these should be:
L28: A body can't be at rest and in motion at the same time in the same inertial frame.
L29a: It is not the case that a body can't be at rest and in motion at the same time in the same inertial frame.
However the more colloquial L29 has been adopted for obvious reasons.
[Having said that, if the term "can", in the above examples is here held to be the equivalent of "it is possible that...", then the negation of any sentence in which that word appears will clearly be far more complex. I have ignored such complications in this Essay. On this, however, see, for example, Garson (2023).]
21. The material that used to be here has now been moved to the main body of this Essay.
22. Naturally, a full derivation here would involve a potentially infinite number of steps, but that doesn't prevent the implications of the theory being clear (if they are a result of the expression of a rule).
Of course, small changes in direction could mean that a body might occupy all of space as it moves (i.e., the entire universe)! [Anyone familiar with "space filling curves" will know what I mean.] However, since my argument doesn't depend on that rather extreme conclusion, I will neither promote nor defend it here.
22a. Any inference to the contrary would plainly be motivated by the realisation that appearances don't, after all, 'contradict underlying essence'. [Irony intended.] When the full implications of DM are exposed, this 'prize' theory (supposedly) penetrates to the heart of 'Being' and ends up concluding that in 'essence' the universe is actually the opposite of the way it appears to be. For DM-fans it appears to be Heraclitean, so, if this theory is to be believed, the universe must be the opposite of that. Hence, in 'essence' the world must be Parmenidean!
No good complaining.
You clearly don't 'understand' dialectics!
22b. As noted in the main body of this Essay, if these were indeed genuine implications of DL, there could be no such thing as "during", and therefore no such thing as "while". That is because this 'path-breaking theory' means there is no "before" and no "after" in relation to a moving object. That in turn is because moving ('dialectical') objects occupy two places at once with no time having elapsed in connection with it being in those two locations. Hence, if that is so, such objects don't move from the first of these locations to the second since they are in both at the same time. If they were to move from the first to the second, there would be a time lapse between the two places occupied; they would therefore be in the first before they were in the second. But that isn't allowed. Moving 'dialectical objects' have to be in both at the same time. And that is why, if there is no before and no after, there can't be a during or a while, either.
The above observation has the following unfortunate consequence: Even though you might think you have to wait an hour for a bus to arrive at your stop, this 'cutting-edge theory' tells you that that 'appearance' is illusory. In 'essence' you have been waiting no time at all; the bus arrived at your stop the exact same moment it left the depot!
That 'paradoxical' result follows on from an earlier point (no pun intended!): DM-fans have yet to say how far apart the two locations are that a moving 'dialectical object' occupies, at the same time. If any distance will do, then the distance between the depot and your stop is as good as any, and if a moving bus is also in two places at the same moment that crazy conclusion follows! No good complaining that this is paradoxical or patently ridiculous; once more, you clearly don't "understand" (the truly bizarre consequences of) dialectics!
In fact, these absurdities might in effect be the physical correlates of the infamous Medieval Ex Falso Quodlibet argument -- that is, the theory that from a contradiction anything follows. In this case, we have also seen that, based on the theory that motion involves a contradiction (or is contradictory), it follows that all moving bodes are everywhere along their trajectories at the same time (no matter the distance between the beginning of a journey and its end).
[On this, see also Note 24b, below, where I reject the above 'logical theory'. Nevertheless, several other absurd consequences of the DM-'theory of motion' will be exposed later in this Essay.]
23. This 'puzzle' isn't, of course, motivated solely by the confused musings of DM-theorists, nor is it a disaster area that only they occupy. Traditional Philosophers (i.e., metaphysicians) still can't explain motion, either, and neither can modern science -- if by "explain motion" we mean "provide a metaphysical, or 'necessarily true', theory of 'motion itself'". Differential equations, vectors, tensors, geodesics and scalar energy gradients can't physically move anything (even if they can be used to help account for it). Motion isn't the product of some sort of Inverse Square Law of Abstraction.
That comment isn't meant to undermine, minimise, depreciate or even denigrate science. All that is being denied here is the ability of anyone to provide a metaphysical explanation of motion (or, indeed, an explanation of anything whatsoever), as opposed to constructing a scientific theory of it.
[Exactly why metaphysicians can't explain anything will be left for Essay Twelve Part One to reveal.]
24. For a much more illuminating analysis of these issues and terms, cf., Black (1954b, 1954c, 1954d). See also Grünbaum (1967), Salmon (1970), as well as Note 12 and Note 19a, above.
24a. Needless to say, the alleged fact "that ordinary objects and people are quite capable of doing the metaphysically impossible" is meant to be taken ironically! Such 'prodigies' are only 'possible' if we insist on using (i.e., distorting) the vernacular in the same naive, if not crude, way that metaphysicians have done for centuries --, and now, DM-fans.
24b. Once more, this might look like a topological version of the infamous 'Quodlibet' argument (that is, from a contradiction everything follows). I haven't used that hackneyed objection to Hegelian 'logic' in these Essays, since, if it is applied unrestrictedly, it isn't a principle with which I would agree. If a contradiction is senseless, nothing can follow from it (a point made by Wittgenstein). However, applied here, restrictedly, that argument seems to imply that a moving 'Hegelian object' must fill the entire universe (or, at least, the entire volume interval comprising its trajectory), at the same time.
[A simplified derivation of Ex Falso Quodlibet can be accessed here. Having said that, the use of contradiction in reductio arguments in logic (i.e., in indirect proof, etc.) is fully legitimate. There, nothing actually follows from the contradiction itself, but from our commitment to validity.]
24b1. In fact, these results will also apply to every atom in a 'dialectically moving body'; they will have to be in two places at once as each of them (and as that body) moves, which means the entire body must either concertina alarmingly or collapse into a point! I have worked out the details, here.
24c. Or, in spherical polar co-ordinates: <r, θ, φ>, and in cylindrical polar co-ordinates: <ρ, φ, z>. I have confined this argument to three-dimensional space, or R3, to minimise the complexity.
[Readers can find out what these variable letters mean by following the above two links.]
24b2. Having said that, physicists still speak about "particles"; but as Essay Seven Part One showed, that word is, for many of them, just a convenient shorthand.
Concerning the movement of these 'particles' we read the following:
"The observed 'particle traces', e.g., on photographic plates of bubble chambers, seem to be a clear indication for the existence of particles. However, the theory which has been built on the basis of these scattering experiments, QFT [Quantum Field Theory -- RL], turns out to have considerable problems to account for the observed 'particle trajectories'. Not only are sharp trajectories excluded by Heisenberg's uncertainty relations for position and momentum coordinates, which hold for non-relativistic quantum mechanics already. More advanced examinations in AQFT [Axiomatised QFT -- RL] show that 'quantum particles' which behave according to the principles of relativity theory cannot be localized in any bounded region of space-time, no matter how large, a result which excludes even tube-like trajectories. It thus appears to be impossible that our world is composed of particles when we assume that localizability is a necessary ingredient of the particle concept. So far there is no single unquestioned argument against the possibility of a particle interpretation of QFT but the problems are piling up." [Kuhlmann (2020). Bold emphases and links added.]
24d. On this in general, see Read (2007), pp.79-115.
25. "Context" in this instance is meant to be interpreted linguistically, not circumstantially. The latter form of contextualism is examined in more detail in Essay Thirteen Part Three, and refers to the intentional, social, contingent or interactive circumstances surrounding or motivating any specific utterance. Linguistic contextualism relates to the sentential role that a given word, phrase or clause occupies in a longer linguistic expression (a clause or a sentence), and is otherwise known as speaker's meaning. So, in relation to single words, this sort of context will be whether or not such a word functions as a noun, adjective, verb, adverb (etc.) -- and that will further involve a consideration of what sort of noun, adjective, verb, adverb, etc., it is. In addition, "context" here refers to the logical role that more complex expressions occupy, whether they are predicative or relational, involving property tokens or psychological states, processes and events, etc., etc. In addition, with respect to a given sentence (or clause), it will also involve a consideration of the mood of its main verb -- e.g., whether it is indicative, interrogative, optative, imperative, subjunctive (etc.). In other words, "context" here can be taken to be related to grammar.
[An example of what can only be called wider philosophical issues (which Wittgenstein labelled "grammatical" -- but he meant by that word something completely different from the ordinary use of that word) involved in relation to linguistic and conversational contexts was given in Essay Twelve Part One. For what Wittgenstein meant by "grammar", see Savickey (1999) -- with a much shorter explanation in Glock (1996), pp.150-55.]
In addition to cases where ordinary objects seem to be able to move while remaining in the same place (i.e., move while being perfectly still), there are numerous examples where two or more objects (howsoever they are defined, described, characterised or interpreted) can occupy the same place or space at the same time (thus further illustrating the 'miraculous' properties of the word "place" and the truly extraordinary nature of the ordinary):
(1) Consider the following 4-tuples: <x1, y2, z3, t1> and <x4, y2, z3, t1>.
Here, at least two variables (i.e., x1 and x4) occupy the same place at the same time, namely, the first place in their respective 4-tuples (by the ordering rules). This is just as legitimate a use of "same place" as any that DM-fans employ when trying to explain what Engels meant; any who object will find it impossibly difficult to study mathematics and science).
And, we don't have to rely on 'abstract' (or maybe even contentious) examples like the one above to make the same point (anyway, there is a more 'concrete' version of (1) below -- check out (8)):
(2) Consider two waves travelling across the surface of a body of water, but orthogonal to one another (or, indeed, at any angle greater than zero but less than 360º). At some point, these two waves will cross, and the moment they (or parts of them) do, they will both be in the same place at the same time. Plainly, this would still be the case whether or not it is true to say that motion is contradictory, or even whether time is 'composed' of instants or intervals.
In fact, ordinary examples of such 'impossibilities' are even easier to find:
(3) Imagine two workers in the same canteen at work at precisely 10:01am on the same day. Here we have two 'objects' in the same place at the same time -- namely, these two workers in the said canteen at 10:01am.
(4) Part of a mother and her unborn baby occupy the same space at the same time. So do any your internal organs; part of you and they occupy the same space at the same time. .
(5) Ten workers complete the same application form (for a new job). Each puts his/her name in the same box at the top of the page. Here, there will be 10 names occupying the same place (namely, the top of each form) at the same time. Alternatively, a teacher tells a class of thirty children to write their names in the same place, namely in a box at the top of an otherwise blank A4 sheet paper that she has just placed in front of them. Once again, there would be thirty 'objects' in the same place (i.e., in the same box at the top of each page), even though they are also physically located in different places (i.e., on different pages, in different parts of the room -- or their names are in slightly different locations inside the boxes at the top of each page).
(6) This sentence ends in the same place as the next. This sentence ends in the same place as the next. This sentence ends in the same place as the next. This sentence ends in the same place as the next…
(7) Countless copies of the same novel (in this case, Ragged Trousered Philanthropists) all end in the same place, namely on page 567. [This is a reference to Tressell (1965), which ends on that page. However, if you are using this online edition of the same book, it will end in the same place as everyone else who uses it, namely on page 1036. (This links to a PDF.)] What applies to novels applies to every copy of the same edition of every book, booklet or pamphlet ever produced (provided they weren't incorrectly printed!). This also applies to countless films and every journey ever made (the latter of which always end in the same place, namely, the end of that journey!).
In relation to the above examples, it won't do to claim that exactly same place wasn't meant every time. So, for instance, it could be argued that there is no way that every single child mentioned in (5) will write their names in exactly same spot, even if they all managed to write their names in the box at the top of each page. Indeed, but the whole point of these examples was to show that not every use of "same place" implies "exactly the same place" (even though some uses of it clearly do -- for instance, in (6) above each sentence ends in exactly the same place). And, even if that weren't the case, as we have seen, there is no way that "exactly the same place" can be defined without the use of the much looser, ordinary sense of "place".
However, in (5) there is a box at the top of the page in which the children have to write their names, so "place" will be defined by that box, as will "exactly the same place". So, if every child writes their name in that box, they will all have written them not just in the same place, but in exactly the same place -- namely in the box at the top of the page! That will be the case since "place" here defines a region and not a mathematical abstraction (like "point" sometimes does), and this clearly means the phrase "exactly the same place" will define anywhere inside that "place", region or box.
Of course, if someone were now to stipulate that "exactly the same place" does define a mathematical abstraction (which means they would be using this phrase in its strictest possible sense), they would only end up having problems with Engels's attempt to analyse motion:
"[S]o long as we consider things as at rest and lifeless, each one by itself, alongside and after each other, we do not run up against any contradictions in them. We find certain qualities which are partly common to, partly different from, and even contradictory to each other, but which in the last-mentioned case are distributed among different objects and therefore contain no contradiction within. Inside the limits of this sphere of observation we can get along on the basis of the usual, metaphysical mode of thought. But the position is quite different as soon as we consider things in their motion, their change, their life, their reciprocal influence on one another. Then we immediately become involved in contradictions. Motion itself is a contradiction: even simple mechanical change of position can only come about through a body being at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it. And the continuous origination and simultaneous solution of this contradiction is precisely what motion is." [Engels (1976), p.152. Bold emphasis added.]
If no two objects can occupy "exactly the same place" (at any time) -- since there will always be a slight difference (as in the objection to (5) above) -- then there is no way Engels can speak about a moving object being anywhere at exactly "the same moment", either -- since there will always be a slight difference here, too. Any attempt to deny this will not only undermine the above objections to (5) and (6), it would torpedo Engels's conclusions about motion on that very score.
So, any attempt to impose (impossibly) strict rules on the interpretation of "same place!" that do not apply to "same moment" will clearly be guilty of the asymmetric bias exposed earlier. Indeed, if Engels meant "exactly the same place" when he wrote that a moving object will be "at one and the same moment of time both in one place and in another place, being in one and the same place and also not in it" (bold added) DM-critics can hardly object to the above use of "exactly the same place" in Example (5).
Who in their left mind would criticise any of the workers mentioned in (5) if they failed to write their name in exactly the same place, strictly interpreted to mean they should all have written their names on the very same page as one another, covering exactly same fibres (and molecules!) of paper in exactly the same spot, but (mischievously!) chose to write their names where they had been told to do so, in the box provided at the top of each page?
Even more 'miraculously', countless objects can occupy exactly the same place at the same time, where all agree that "exactly the same place" is meant to be taken in its strictest sense (and even if they are miles apart!):
(8) Consider two individuals NN and NM. NN is stood second in line to buy tickets to a film, while at the same time, miles away, NM is also stood second in line waiting to be served a hamburger. Here we have two individuals in exactly the same place -- namely second in line --, at the same time, even though they aren't physically located in the same place!
Is this yet another 'contradiction'?
Only to those who need professional help.
Of course, the same applies to everyone in the entire history of queuing (in person, electronically or 'virtually') who is second in line in their respective queues. And what applies to second place applies equally well to those who are first, third, fourth, fifth..., and so on, in separate queues, at the same time.
Indeed, this also applies to anyone who ever came first, second, third, fourth.., etc., in any competition (in sport, a quiz, a parlour game, a Spelling Bee, an episode of 'Dancing With The Stars', an election (political, union, financial, etc.), in all of human history, providing they took place at the same time as one another. As should seem obvious, this means that there have been, or there are now, countless thousands in exactly the same place as one another (namely first, second, third, fourth...), even if they were/are separated by thousands of miles (provided they were in place at the same time as one another).
Look how easy it is say all that in the vernacular; just try saying it using only Hegel-speak!
In addition to the above, it is possible to imagine cases where moving objects somehow manage to remain stationary even while they are moving -- revealing yet another amazing 'contradiction':
(9) Consider an object located at (x1, y1, z1, t1) with respect to some inertial frame. Let that frame itself move with respect to another inertial frame. In that case, the object in question could remain stationary with respect to the first frame, while it moves with respect to the second.
Again, an ordinary example will suffice to illustrate this 'contradiction':
(10) A child is ascending a descending escalator in such a way that she remains stationary (even momentarily) with respect to an arbitrary point not on that escalator.
Of course, in all such cases, the alleged 'paradoxes' and 'contradictions' they 'reveal' are easily resolved by clarifying the many equivocations and ambiguities they contain. Unfortunately, that eminently reasonable strategy isn't available to DM-fans -- or, at least, not without it threatening to undermine what few examples of 'real contradictions' they have managed to scrape together over the last century-and-a-half in support their ramshackle 'theory'.
26. Update 28/05/2014: Again, we read this from the BBC:
"Yarm people vote to join North Yorkshire
"Residents of Yarm have voted for the town to leave Teesside's Stockton Borough Council and join the Hambleton district of North Yorkshire. The poll of residents on Tuesday was called by the town council and is not legally binding. About 89% of those who took part supported the idea. Campaigners claim the borough council has ignored people over issues such as parking and housing. Critics say the proposal is unlikely to be introduced.
"Any change to Stockton Borough Council's boundaries would require the unanimous support of Hambleton, North Yorkshire and Stockton councils as well as a review by the Local Government Boundary Commission for England. Paul Smith, of the Yarm 4 Yorkshire campaign, said residents in the market town did not feel properly represented by Stockton Borough Council and that its 'historic roots' were in North Yorkshire. Stockton Council said a move to North Yorkshire would not in itself resolve the town's parking and housing issues. The poll attracted a turnout of 24%, with 1,465 votes in favour of the move to Hambleton and 177 against.... Traditionally, Yarm was part of Yorkshire until the re-organisation of local authority boundaries in the 1970s...." [Quoted from here; accessed 28/05/2014. Quotation marks altered to conform with the conventions adopted at this site. Several paragraphs merged. Bold emphases and link added.]
It looks like this 'reactionary' part of the UK just doesn't 'understand' dialectics, either. I will resist making the same points as I have in the main body of this Essay (in relation to moves like this). Enthusiastic readers can fill in the details for themselves.
27. I have had to use slightly stilted language in relation to some of these examples so that the use of the word "move" is clear for all to see. Normally, one would employ other, more appropriate verbs. However, that having been done, the obvious danger would be that dialecticians might miss the point (indeed, experience has taught me that many of them are experts at 'failing to see' what they don't want to see, a bit like some members of the Trump family have suspiciously 'leaky' memories when it suits them). For instance, L63 would more normally be written as L63a, or even perhaps better as L63b:
L63a: The wire winds in a spiral around that tree over there. It's been in the same spot so long the tree itself has partially grown over it.
L63b: The wire spirals around that tree over there. It's been in the same spot so long the tree itself has partially grown over it.
[L63: The wire moves in a spiral around that tree over there. It's been in the same spot so long the tree has partially grown over it.]
In the above example, it is irrelevant whether the wire in question has actually shifted position over the years, because that particular sense of "move" isn't the same as the one intended in this case. Wires can move around trees (with no change of place implied) just as gaps can run through crowds and holes through Polo Mints and mountains (i.e., as tunnels). Here, the wire moves around the tree (i.e., it winds or spirals through the same 360º of turn, perhaps several times) while not itself rotating around the tree's geometric centre (in one sense of "rotate"), whether or not the radius of each turn alters over the years. Winding or spiralling around a tree is a different sort of movement from, say, gripping it more or less tightly over time -- or, indeed, from slipping down the trunk, or even circling that tree (as someone would if they walked around it).
And, of course, we can speak for instance about a DNA molecule spiralling around its geometric centre without implying any (relative) movement at all:
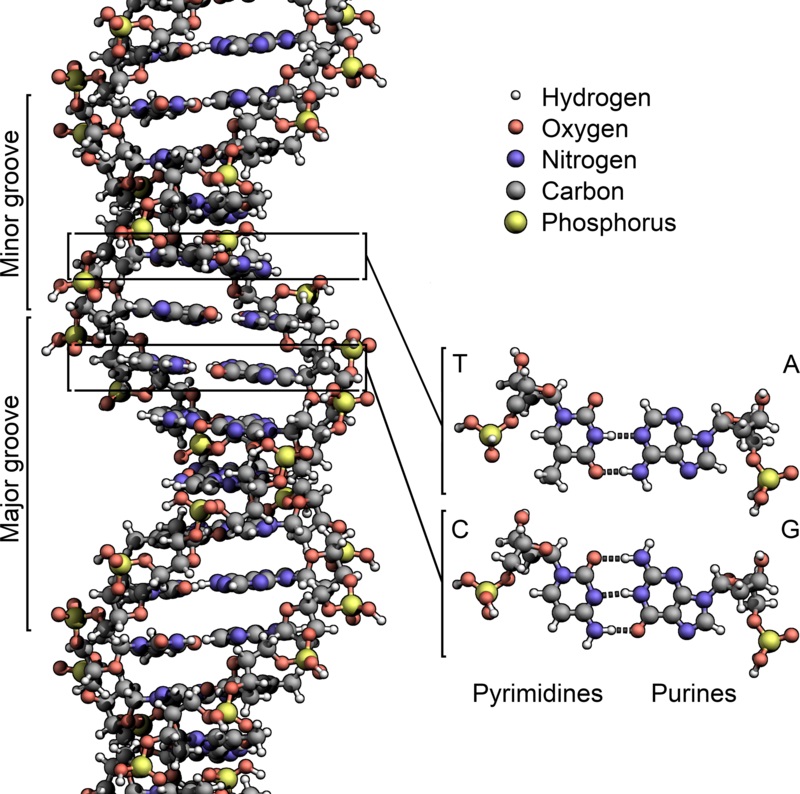
Figure Fourteen: A DNA Molecule Spiralling While
Remaining Perfectly Motionless
[Credit: Zephyris https://commons.wikimedia.org/w/index.php?curid=15027555. The legal owner of the above image has nothing to do with this site or with any of my political opinions!]
28. Another familiar example concerns the different aspects a shape can assume while being observed -- even though nothing actually 'changes places':
M3: "The front of this Necker Cube moves to the back when I look at it for a few seconds."
Figure Fifteen: Motion With No Movement?
Above, in relation to Figure Fifteen, it would be odd to say that the front and back of the said cube both occupied and did not occupy the two places which they had moved into (i.e., the back or the front) at the same time, and that both were in two places at once (i.e., front or back, again), even though it would be perfectly normal to say that the front and the back had moved (i.e., "changed places"), as indicated. Of course, that is mainly because front and back occupy the same place all the time -- namely a white square on your screen! So, how do these amazing shapes manage to move while remaining in exactly the same place?
[Spoiler: blame ambiguity, again!]
Other examples of 'gestalt' switches (in Figures Sixteen to Nineteen) could be described equally well in the same uncontroversial manner. Here shapes move, or change, but it isn't easy to say how they do this, or, indeed, where they move to, or what 'places' they occupy while they are doing it! In such cases, we would have at least two intentional/perceptual objects occupying no places at all, even while they moved!
And that would remain the case even though they occupy exactly the same place on your screen -- and they would still manage to do that while you were scrolling the page!
Figures Sixteen To Nineteen: Four More Dialectically-Annoying,
But No Less 'Reactionary', Objects
There are also optical illusions that appear to move themselves; I will post only one example of such, but there are scores more like it on the Internet:
Figure Twenty: Movement Without Movement
[That Happens When The Viewer Moves Her Head Away From Or Toward The Screen.]
The yellow shapes in Figure Twenty appear to rotate as the observer stares at the black dot in the middle and moves her head toward the screen/page or away from it.
Are those shapes in two places at once when they don't actually move? But, how can they move if they all remain in exactly the same place?
One US comrade (Brian Jones) objected to the use of examples like these, along the following lines:
"There are countless silly examples on Lichtenstein's website. She claims, for example, that Necker cubes are qualitatively different from regular cubes with no quantitative difference, and thereby are another refutation of dialectics. But by definition, these cubes are ambiguous in our perception of them. They are, after all, not even real cubes, only representations of cubes! Their qualitative difference from other cubes exists entirely in the realm of the idea of a cube. Lichtenstein has lost sight of the purpose of dialectics -- to understand the motion of things as we observe them in nature. I'm not sure what laws (if any) govern the transformation of one representation of a cube into another representation of a cube. Down here on earth, in order for one thing to truly change from one qualitative state to another, specific quantities of energy must be added or subtracted, in a manner exactly fixed for each individual case." [Quoted from here.]
Although comrade Jones's objection was aimed at what I had argued against Engels's First 'Law' (the alleged change of quantity into 'quality'), it could be used against the above comments about movement associated with gestalt transformations and optical illusions. Here is how I responded to him:
In fact I nowhere said that Necker cubes are qualitatively different from ordinary cubes. What I did say was this:
"However, other recalcitrant examples rapidly spring to mind: if the same colour is stared at for several minutes it can undergo a qualitative change into another colour (several optical illusions are based on this fact). Something similar can happen with regard to many two-dimensional patterns and shapes (for example the Necker Cube and other optical illusions); these undergo considerable qualitative change when no obvious quantitative differences are involved. There thus seem to be numerous examples where quantity and quality do not appear to be connected in the way that DM-theorists would have us believe." [Quoted from here.]
The difference here is between two views of the same Necker Cube, not between the latter and an ordinary cube.
However, as we have seen, it is Engels who wants to impose this 'Law' on nature, while I want to impose nothing on anything.
So, when comrade Jones says the following:
"Their qualitative difference from other cubes exists entirely in the realm of the idea of a cube. Lichtenstein has lost sight of the purpose of dialectics -- to understand the motion of things as we observe them in nature. I'm not sure what laws (if any) govern the transformation of one representation of a cube into another representation of a cube",
he has plainly ignored what Engels elsewhere tells us about his theory:
"Dialectics, however, is nothing more than the science of the general laws of motion and development of nature, human society and thought." [Engels (1976) p.180. Bold emphasis added.]
Ideas of Necker cubes are objects of thought, I believe. Perhaps comrade Jones thinks otherwise.
In which case, no supporter of DM can discount the movement of intentional objects like those illustrated above.
29. Engels's theory has to be able to account for the motion of both large and small bodies, otherwise it would be of no use to dialecticians trying to explain, say, the motion of cats on and off mats, let alone the historic, economic and social changes we see in Capitalism. But, if his theory can't account for the sort of mundane events easily expressed in ordinary language, it stands little chance of being used successfully to help make sense of the complex movement of, say, electrons or even social classes. So, no DM-fan can afford to turn their noses up and complain about the prosaic examples aired in this Essay.
30. This is argued in detail in Essay Seven Part One.
More importantly, Engels's conclusions are uncheckable, as we have seen. That is because they don't depend on anything in this world for their truth-status, having been divorced from it by an egregious misuse of language, deliberately carried out in order to engineer a quirky, Ideal result.
In so doing, dialecticians and Traditional Philosophers not only ignore the most important resource the human race has available to it for understanding anything that is capable of being comprehended -- i.e., ordinary language (which has been tested and refined in and by social practice for thousands of years) --, they wilfully distort it into the bargain. Small wonder then that it isn't possible to make sense of anything they say.
[Those who think ordinary language is defective or of limited use -- and hence isn't the most important resource we have, or even that it can't be trusted -- are encouraged to shelve those qualms until Essay Twelve has been published (preliminary summary here), and then perhaps think again. In the meantime, they should check this out.]
Of course, there are some, like Graham Priest, who think we can observe contradictions. His ideas will be examined in a later Essay; in the meantime readers are directed here, and Note 18a, for further details. However, not even Priest thinks that the 'contradictions' that are supposed to exist in motion can be observed. Even he accepts the truth of the assertion that motion is contradictory because of a series of 'philosophical' arguments/'thought experiments' [Priest (2006)], and on them alone.
The fact that this area of DM is based solely on thought experiments was inadvertently confirmed by the following comments posted over at the IMT's website:
"Heraclitus, the ancient Greek philosopher, famously said that 'everything changes and nothing remains the same' and that 'you can never step twice into the same stream' [That isn't what Heraclitus actually said, this is: 'On those stepping into rivers staying the same other and other waters flow' -- RL]. It is the ideas of ceaseless change, motion, interconnectedness and contradiction that define dialectical thought.
"The philosopher Zeno famously tried to illustrate how essential dialectical thinking is to our understanding of the world by using thought experiments. He poses the following: Imagine an arrow in flight. At any one durationless instant in time (like the freeze-frame in a film) the arrow is not moving to where it is going to, nor is it moving to where it already is. Thus, at every conceivable instant in time, there is no motion occurring, so how does the arrow move? To answer this we are forced to embrace what appears on the surface to be a contradictory idea -- that the arrow is, at any one time, in more than one place at once. This thought experiment serves to highlight the contradictory nature of the movement of matter in the world.
"The German philosopher Hegel further developed the dialectical [sic] in a systematic form. Instead [of] trying to discard contradictions Hegel saw in them the real impulse for all development. In fact Hegel saw the interpenetration of opposites as one of the fundamental characters of all phenomena. Hegel's philosophy is one of interconnectedness where the means and the end, the cause and the effect, are constantly changing place. It explains progress in terms of struggle and contradiction, not a straight line or an inevitable triumphal march forward...." [Quoted from here; accessed 02/08/2015. Bold emphases and link added. Quotation marks altered to conform with the conventions adopted at this site. Several paragraphs merged.]
Even though the author admits these ideas were dreamt up by ancient and early modern Mystics, he still somehow thought it legitimate to import them into the workers' movement.
What next? Weird passages from The Book of Revelation?
31. Hyphenated, metaphysical jargon like this (e.g., "Things-in-themselves", "Being-for-itself", "Being-for-us", etc.) litter much of post-Kantian Continental Philosophy (and, alas, DM-texts, too), where they only succeed in further clouding issues that were already hopelessly confused. Working in tandem with the 'magical power' of innocent-looking quotation marks (for example, as they wrap around phrases like the following, "Thing-in-itself", and "Being-for-us", etc.), this neat typographical trick 'allows' those enamoured of such dodges to 'penetrate right to the heart of reality' while sat at a keyboard, or chilling out in an armchair.
Who in their left mind can complain?
Hence, any riposte advanced by me (in response to objections raised against the criticisms aired at this site) that is similarly be-decked in such talismanic finery should work like magic, too. In that case, I could reply to anyone who objects to the anti-DM points rehearsed in this Essay in like manner. To that end what is to stop me saying that metaphysical and dialectical terminology is "Intrinsically-Incomprehensible" since it is thoroughly "Obscure-in-itself", having been concocted by "Mystics-R-Us".
That should put an end to Traditional Philosophy/DM -- just as it removes any need to justify further the use of this handy device aimed at the "Long-overdue-termination" of DM.
Those still unconvinced by the above linguistic gyrations would do well to be equally sceptical when DM-theorists themselves indulge in the use of such jargon. Until then they are clearly not "Thinking-as-such"; they should stop "Being-picky-in-themselves" over which "Quotation-marks-and-hyphens-they-take-seriously", and which use they fail to find "Convincing-in-itself". Clearly, a "Quotation-mark-hyphenated-response" wins every time. "End-of-story".
The Awesome Supernatural Power possessed of hyphens and quotation marks will be examined in more detail in Essay Twelve.
32. Nor has a single DM-theorist ever even looked for any! And that includes scientists who (unwisely) accept Engels's theory.
Or if they have, they have kept it well hidden.
Again, it could be argued that the entire analysis of motion in this Essay is completely misguided since DM-theorists are really only interested in the movement of material bodies in objective reality. The examples considered here hardly address that issue at all.
Or, so it could be maintained...
In reply, it is worth noting that (with respect to dialecticians who are, at least, Leninists), if we define matter as objective existence external to the mind, then few of the examples given in this Essay would fail to be genuine instances of movement in the real world. In which case, the objection is itself misguided.
Until DM-theorists themselves tell us what they take matter to be (having prevaricated on this issue for over a hundred and forty years -- as demonstrated in Essay Thirteen Part One), they are in no position to find fault with the counter-examples aired in the present work and at this site.
33. These objections have been examined throughout this Essay. On the dubious commitment to 'universal change', for example, see here. On whether DM can account for it, see here.
34. This argument was laid out in extensive detail in Essay Twelve Part One.
Appendix A: Thomas Weston On How To 'Resolve' A 'Contradiction'
Here is Weston's attempt to answer the question: How are 'contradictions' "resolved" (I have omitted all footnotes):
"Although Hegel and Marx agree fairly closely on the basic features of oppositions and contradictions, they have quite different views on the process and the result of the resolution...of real contradictions and oppositions. According to Hegel's treatment in the Science of Logic, contradictions are resolved by incorporating them into a more inclusive whole, a 'higher sphere' in which the contradiction is 'overcome'.... A contradiction is 'overcome' if its two sides are altered by incorporation into a higher sphere, but are also preserved in this altered form so that they no longer contradict each other. This overcoming is the result of mediation, providing a link between the opposite sides, which then form a more inclusive totality. Something 'is overcome, only insofar as it enters into a unity with its opposite'. The result of this mediating process is a situation in which a 'contradiction has not abstractly vanished, but is resolved and reconciled'....
"In his early critique of Hegel's Philosophy of Right, Marx not only denied the possibility of mediation of specific social oppositions and contradictions, such as those between the monarch and civil society, but also presented a general critique of mediation, which claimed that 'Real...extremes cannot be mediated precisely because they are real extremes.' Opposites are real opposites, however, only if they are 'opposed in essence', and 'do not supplement each other'.... Hegel's chief error, according to Marx, had been to conceive of contradiction as a contradiction of appearances, but also a 'unity in essence, in the Idea', when the contradiction is actually a contradiction in essence. Hegel was also wrong to regard the intensification of the struggle of opposites, their 'inflammation...to a decision', that is, to the defeat or destruction of one side, as 'something possibly to be prevented or something harmful', which required mediation....
"The evidence from Marx's later work does not support this claim [that he abandoned the above view -- RL], although it does show some changes in terminology. In particular, the best equivalent in Marx's later terminology to his early expression 'real extremes' that are 'opposed in essence' would probably be the term 'real contradiction'. In Capital, Volume 1, Marx does give examples of extremes that are mediated; for example, money thrown onto the market and money withdrawn from it are mediated by purchase and sale. He several times characterises the oppositions occurring in circulation as 'supplementary', thus not 'opposed in essence', as the earlier term 'real extremes' required. Marx does say, however, that these oppositions can become contradictory....
"The key point of Marx's critique of mediation of contradictions is not that mediation of a contradiction does not occur at all, but that even when the mediating links exist, they do not resolve the contradiction of the opposite sides or prevent its intensification. The textual evidence shows that, early and late, Marx maintained that mediation only resolves apparent contradictions, not real ones, and this resolution by mediation takes place only in the realm of theories and concepts. Resolution of real contradictions requires a process called 'development', in which the contradiction becomes sharper and more intense. This is very close to Marx's conclusion in his early critique of Hegel. Since the resolution of real contradictions is one of the themes of the ellipse passage, we must review some of the evidence that Marx held this view....
"The idea that a contradiction can be mediated only if it is apparent, rather than real, is clear in the few examples Marx gives in his works of contradictions that can actually be mediated, examples dealing mainly with contradictions within theories. He notes, for example, that zero divided by zero appears to be a contradiction, but intermediate links may be provided that show how such an expression can make sense. Marx takes James Mill to task for trying to resolve theoretical contradictions 'by phrases', without finding 'intermediate links...' between the concrete and abstract aspects of his theory. He criticises Ricardo for trying to resolve a prima facie' contradiction without intermediate links. For many cases of non-theoretical contradictions, however, Marx explicitly rejected mediation. He stated that certain contradictions or oppositions are unmediated; for example, that 'The function of money as means of payment contains an unmediated...contradiction.' He ridiculed the 'critical moralists' who 'know how to unite contradictions', and rejected Roscher's account of economic phenomena as trivial, since 'the word mediation decides everything'.... In the Communist Manifesto, Marx and Engels criticised 'utopian' socialists who tried to blunt class struggle and mediate opposites. In 1879 Marx and Engels criticised the leaders of German socialism for their attempts at mediation of social oppositions instead of struggling to defeat the capitalists and the government.
"According to Hegel, mediation of contradictions was supposed to reconcile the two sides. As with mediation, Marx made both philosophical and political critiques of attempts to reconcile contradictions. He characterised some individual contradictions as 'irreconcilable...', and rejected attempts by J. S. Mill, who denied the contradictions of capitalism, to 'reconcile the irreconcilable'. He ridiculed idealistic attempts at reconciliation of contradictions and oppositions.... Later Marx wrote that the illusion of reconciliation of parties that represent conflicting interests only promotes domination by the interests of one of the parties. We have already noted Marx's view that resolution of a real contradiction requires 'development'. Marx took this concept from Hegel, for whom it meant the unfolding of the inner potential of something. Marx endorsed this broad conception of development as possibility becoming 'reality...' and applied it to some things other than contradictions that undergo development, such as the forces of production and commodity relations.
"Marx's conception of the development of real contradictions appears to involve at least three features: (1) becoming simpler, (2) becoming more apparent, and (3) becoming sharper, more intense, or being 'driven to a peak'. We will briefly discuss how each of these features appear in Marx's texts. Simplification and intensification are both cited as features of development in a passage in the 1844 Manuscripts. There, Marx described the transformation of landowners into capitalists as a movement of reality' that 'will simplify the opposition [between labour and capital], drive it to a peak and therefore accelerate its resolution...'. In the Communist Manifesto, the bourgeois epoch is described as one that 'has simplified class oppositions. Society is more and more split into two great hostile camps, into two great classes directly confronting one another', which eventually leads to the 'forceful overthrow' of the bourgeoisie. The division of Germany and Austria into separate countries took place by simplification of various oppositions.
"Developing oppositions and contradictions also tend to become more apparent. The contradictions of the lawgiving power in bourgeois society are 'driven into appearance'. In a crisis of the world market, 'the most developed phenomenon of capitalist production', the 'contradictions and oppositions of bourgeois production become striking...'. The aspect of development that Marx most emphasises is intensification. Here are a few of numerous instances: A 'sharper and deeper...opposition...develops all the more'; 'driven to a peak..., this opposition is necessarily the peak, the limit...and the destruction...of the whole relationship'. 'This opposition becomes sharper every day...and pushes toward a crisis.' A reasonable interpretation of increased intensity or sharpness of a contradiction is an increase in the mutual interference of the two sides. As the contradiction undergoes the fullest possible development and nears resolution, this interference is increased to such an extent that the two sides cannot coexist any longer, and one must defeat the other, either by destroying it or by weakening it so completely that it can no longer interfere with the victorious side.
"Marx does not appear to have made a categorical claim that real contradictions can only be resolved by development, but he does make statements from which this is a reasonable conclusion. In the 1844 Manuscripts, he claims that development does in fact lead to resolution. There he wrote that opposition of capital and labour is private property as its developed relation of contradiction, hence...an energetic relation driven to resolution...'. Here the term 'hence' indicates that the resolution takes place because the contradiction is developed. In a number of specific cases Marx claims that development is a necessary condition for the resolution of real contradictions. In Capital, Volume 1, he wrote: 'The development of the contradictions of an historical form of production is, however, the only historical path of their resolution...and new formation.' Marx and Engels ridiculed Bruno Bauer for presenting a contradiction as having 'found its resolution not in the course of its development...' but in 'elements' that already existed independently of the contradiction. Part of the case for the inevitability of proletarian revolution in the Communist Manifesto is the claim that 'the development of class opposition keeps step with the development of industry', so that the intensification of that opposition is inevitable.
"We have found Marx rejecting resolution of contradictions by mediation -- except in some theoretical cases -- and asserting resolution by development for many instances and some general categories of real contradictions and oppositions. The most reasonable interpretation of his views on this topic is the one already mentioned, that real contradictions or oppositions are resolved by development. The ellipse passage also asserts that motion is required for resolution of actual contradictions. It might be reasonable to add motion as a fourth feature of development, or to regard it as an additional condition for resolution of real contradictions. 'Real contradictions' are not limited here to contradictions in society or nature, but also might include some contradictions in theory, since Marx mentions contradictions in a theoretical trend that undergo development. The majority of the real contradictions whose resolution he discusses, however, are not theoretical, including the ellipse case that concerns us here. If the thesis defended here is correct, that real contradictions are only resolved by development but some (apparent) theoretical contradictions can be resolved by mediation, then Marx's dialectics, considered as a logic of concepts, and his dialectics as an explanation of real historical change have some significant differences, since the former permits both sides to be preserved while the latter involves defeat or destruction of at least one side....
"The central interpretative problem of the ellipse passage is the meaning of the assertion that elliptical motion solves...an actual contradiction but does not overcome...it. Exploring this idea requires a closer examination of Marx's terminology. In various texts, Marx used all of the terms 'lösen', 'auflösen', and 'aufheben' to describe the resolution of a contradiction. The meaning of the term 'overcome [aufheben]' is fairly straightforward. Generally it means to cancel, but in a few cases Marx seems give it Hegel's sense of both cancelling and also preserving in a modified form. If a contradiction is overcome, it ceases to be a contradiction. The other terms are more problematic. In ordinary German, 'lösen' means solving a problem or a difficulty, or loosening something. 'Auflösen' means to dissolve, resolve or disintegrate. Parallel to 'aufheben', a resolved contradiction is no longer a contradiction. Unfortunately it is difficult to find in Marx any systematic difference in the use of 'lösen', 'auflösen', and forms deriving from them, to describe processes taking place in contradictions. Marx used 'lösen' more often to describe the elimination of theoretical contradictions, but he also sometimes used that term to describe the resolution of economic and political contradictions.
"Marx's typical usage of 'lösen', 'auflösen', and 'aufheben' seems to make them synonymous when applied to contradictions. Doing so in the ellipse passage, however, would produce an absurdity. 'Lösen' must at least be different from 'aufheben', since otherwise Marx would be flatly contradicting himself by asserting one and denying the other. Several authors have concluded that 'lösen' must not mean 'auflösen' in that context. Andreas Arndt, for example, has identified lösen with removal of a contradiction while preserving the totality that provides the conditions for its existence. He identifies auflösen with the removal of the contradiction by the breaking up of that totality. Christian Iber has claimed that, for Hegel and Marx, a real contradiction can be 'coped with or solved', but not overcome by theoretical development.
"By using 'lösen', Marx is at least asserting that the contradiction between two tendencies of motion is sustained, and not subject to immediate resolution. My best guess is that for Marx, elliptical motion 'solves' the contradiction by constituting a partial realisation of both of the two contradictory tendencies, which cannot both be fully realised because of their incompatibility. That is, the gravitational tendency is partially realised since the planet or satellite moves nearer to the central body than it would if gravity were absent, but does not actually hit the central body. The tangential tendency is partly realised, since motion in the tangential direction takes place, but only within limits....
"Comparing the German and French versions of the ellipse passage throws some light on the significance of 'lösen'. Marx worked over J. Roy's French translation extensively. He found it too literal and revised it for readability; the revisions were extensive enough that he claimed that the French version had a scientific value independent of the original. Here is a translation of the French version of the ellipse passage:
'The exchange of commodities cannot, as one has seen, take place without fulfilling contradictory conditions, which exclude one another. Its development, which makes commodities appear as something with two aspects, use-value and exchange-value, does not make these contradictions disappear..., but creates the form in which they can move themselves. This is in any case the only method for resolving...real contradictions. It is, for example, a contradiction that a body should fall constantly toward another, and also constantly fly away from it. The ellipse is one of the forms of movement by which this contradiction realises itself and resolves itself...at the same time.' [Underlining in the original -- RL.]
The underlined words do not correspond to words in the German text. The 'overcome' versus 'solved' distinction is preserved here by Roy and Marx, using 'make disappear...' for 'aufheben' and 'resolve itself...' for 'lösen'....
"Although it was prepared after Marx's death and thus gives no direct evidence of Marx's intentions in the ellipse passage, it is worthwhile noting the remarkably inaccurate and misleading character of the Aveling-Moore translation of the ellipse passage, the version followed in the English Marx/Engels Collected Works. Here is that translation:
'We saw in a former chapter that the exchange of commodities implies contradictory and mutually exclusive conditions. The differentiation of commodities into commodities and money does not sweep away these inconsistencies, but develops a modus vivendi, a form in which they can exist side by side. This is generally the way in which real contradictions are reconciled. For instance, it is a contradiction to depict one body as constantly flying towards another, and as, at the same time, constantly flying away from it. The ellipse is a form of motion, which, while allowing this contradiction to go on, at the same time reconciles it.'
"There are at least three ways in which this translation is incorrect. The phrase that these contradictions 'develop a modus vivendi, a form in which they can exist side by side' renders a German phrase which actually says that the contradictions create a form in which they can move. The terms 'modus vivendi' and 'exist side by side' are glosses, not translations, and movement, which is a key point of this passage, is not adequately rendered by 'modus vivendi', which suggests accommodation, not motion. Second, 'lösen' is twice translated as 'reconciliation', which is surely incorrect. The German term for reconciliation is 'versöhnen', not 'lösen', and both Marx and Engels polemicised against the idea that real contradictions can be reconciled. Third, the phrase 'it is a contradiction to depict' conveys an idea directly opposite to the assertions of the German text. The contradiction is not only in the depiction of elliptical motion; it is in the motion itself. This is the clear sense of the German text's assertions that the contradictions are 'real...', are 'actualised...', and that the sides of the contradiction are the two tendencies of motion that are mentioned, not their depictions. Other passages in the Aveling-Moore version of Capital, Volume 1, that use dialectical terminology are also not sufficiently accurate for use in philosophical discussion.
"It is puzzling that Engels, who worked over this translation in detail, would allow such a defective English version to be published. Engels was not happy with the dialectical development in the early part of Capital, Volume 1. He preferred a more historical presentation, and maintained that '...the philistine [reader] is not used to this kind of abstract thought and will certainly not be pleased to torture himself for the sake of the form of value'. Later he wrote to Marx that in the English translation of Capital, 'what is inevitably lost in the really dialectical places' could be made up on other subjects. It seems that Engels had very low expectations for an accurate and comprehensible English translation of dialectical passages from Capital. The ironical result is that although Engels has been accused of importing the dialectics of nature into Marxism, the translation he edited obscured an important example of it in the English version of Capital.
"We have seen that Marx claimed that development is a necessary condition for the resolution of real contradictions. In the ellipse passage, however, Marx denies that development of the commodity form overcomes its contradictions, but only solves them. This remark suggests that development is not a sufficient condition for resolution of a real contradiction. The comparison with elliptical motion follows immediately, where the contradiction in elliptical motion is said not to be overcome, although it is not claimed to be developed or developing. Does this mean that some real contradictions do not develop or move toward resolution? Several authors have proposed this interpretation. In his discussion of the ellipse passage, Hubert Horstman has claimed that it shows that for Marx, '[n]ot every contradiction drives toward resolution'. Chinese philosopher Hu Zhengping goes further than Horstman and claims that there is a mode of resolution of contradictions called 'dynamic equilibrium...'. Hu considers this a contribution to the project that dominates dialectical philosophy in China today, that of harmonising social contradictions, in particular, 'combining a market economy with socialism'. [Looks like Hu is saying such contradictions can be 'reconciled', contrary to Marx and Engels -- RL.] Both Hu's and Horstman's views are part of a trend of dialectical thought in Russia and China dating back to the 1930s, a trend that claims there is a special kind of 'non-antagonistic' contradiction, including all the social contradictions of socialism, that does not tend to become more intense. The ellipse passage is the whole of the slender evidence Hu finds in Marx for dynamic-equilibrium resolution, which he explains as follows:
'It [the dynamic-equilibrium mode of resolving contradictions] differs from the mode of one side [of the contradiction] annihilating the other or of one side overpowering the other. It forms a kind of comparatively harmonious...pattern of motion in the interaction of both sides. These intrinsic contradictions are not overcome..., but still exist while they do not intensify...at all. They [the two sides] remain dependent on each other and supplement each other, but although they have opposition and a struggle in which the expansion of one eliminates the other, both sides are also in a continuous process of resolution...of the contradictory motion, achieving a greater balance and coordination overall, thus realising at a certain stage a thing’s higher development of dynamic equilibrium.'
"Why Hu regards dynamic equilibrium as a mode of resolution of contradictions is something of a mystery, since he admits that a contradiction subject to it continues to exist. A contradiction is not resolved by finding a compromise or an optimal adjustment between the opposing sides, as long as those sides continue to exist and interfere with each other. Hu appears to describe the solution, not the resolution of a contradiction. In any case, both Horstman and Hu believe that Marx sees some actual contradictions as lacking the tendency to develop. Let me explain why I believe this conclusion is not warranted by the ellipse passage.
"First let us notice that there are two contradictions -- or perhaps types of contradiction -- in the ellipse passage, those involving the circulation of commodities and those realised in elliptical motion. Marx made clear that contradictions between use-value and exchange-value, or between commodities and money, are capable of becoming very intense and causing crises. Marx may also have had this view of planetary motion. It was a widely circulated view in nineteenth-century England that the solar system would eventually run down. Earth's orbit around the Sun, for example, might eventually decay and lead to the end of its (nearly) elliptical orbit. A comment by Marx in Capital, Volume 1, shows that he was aware of this idea. Engels actually held this view a few years later, claiming that the Earth would 'circle in constantly narrower orbits' around a 'dying Sun' and finally fall into it. If Marx agreed with this -- and we do not know that he did -- it would be quite consistent with the ellipse passage to conclude that both the contradictions in the circulation of commodities and in elliptical motion, while not necessarily becoming more intense or driving toward resolution at every moment, would eventually do so. The ellipse passage says nothing about decay or intensification, and Marx is silent on whether or how the contradiction in elliptical motion might end. The conclusions of Horstman and Hu are supported by the ellipse passage only if this silence could be taken as evidence of Marx's view that intensification or driving toward resolution would not happen in an actual system undergoing elliptical motion. I think that Marx's silence on this point does not constitute such evidence, and examining Marx's reasons for introducing the topic of elliptical motion helps show this....
"It is clear from the ellipse passage that Marx is making use of an example of a contradiction in nature both to illustrate a general assertion about contradictions and motion, and to make a point about contradictions in the circulation of commodities. Using the ellipse case to do this would make no sense at all unless Marx assumed that natural processes have dialectical features and that his readers would accept this fact. Besides the ellipse passage, Marx used a similar argumentative strategy in several other places. In the Grundrisse, he criticised a superficial and excessively abstract way of describing buying and selling, making an argument by analogy against it in this way: '...thus it is the same as it would be to maintain that there is no difference, much less opposition and contradiction, between natural bodies..., since they, for example, are grasped in the determination of weight, and all are heavy and thus are equal; or are equal since they all take up three-dimensional space'. Here the existence of opposition and contradiction between natural bodies is taken for granted in order to argue that it is incorrect to deny opposition and contradiction in buying and selling. This argument is similar enough to that made later in the ellipse passage that it may actually be an early version of that passage.
"The pattern of analogy in which a natural opposition is taken for granted also appears in a remark by Marx about magnetism in Capital. Discussing the oppositions that he has brought out within the forms of commodities, Marx notes that such oppositions are not obvious: 'People do not by any means regard the general immediate form of exchangeability as an oppositional commodity form, one that is just as inseparable from the form of nonimmediate exchangeability as the positivity of one pole of a magnet is from the negativity of the other pole.' This passage and some others make it clear that Marx thought that there was opposition in a magnet. Although these passages and the ellipse passage would not make sense if Marx were not assuming that there are contradictions and oppositions in nature, the point of his argument is about economics, or, in the ellipse passage, about economics and dialectics. Thus Marx did not give any more detail about the interpretation of his physics examples than is necessary to make these points. Indeed his rhetorical strategy only works if the physical facts are taken for granted. Thus it is not reasonable to make inferences about Marx's interpretation of the dialectical features of the physics in these examples based on what he omits. That is the inference, however, which is required by Horstman's and Hu's interpretations.
"Whether the arguments above prove that Marx saw dialectics in nature depends in part on what dialectics is. Marx himself saw contradiction as the central concept of dialectics. In Capital, Marx wrote that 'Hegelian "contradiction" is the source of all dialectics.' So if there are contradictions in nature, then there is dialectics in nature, as Marx understood dialectics. Lukács gave his own list of the most essential features of dialectics, and contradiction was not among them. Hence he might have argued that there is no dialectics of nature in his sense. Obviously this would not constitute evidence that Marx differed from Engels on this issue. In this paper, we have only mentioned Engels's views in passing. Engels wrote quite a bit on the question of elliptical motion, however, and his focus and his views are somewhat different from Marx's. In particular, Engels pursued Hegel's argument that there is no tangential tendency of motion (that is, inertia) in elliptical motion. Nothing we have from Marx shows concern with this aspect of Hegel's argument. Engels's views on this subject might make an appropriate topic for further study." [Weston (2012), pp.19-32. Several paragraphs merged; links and bold emphases alone added. Quotation marks altered to conform with the conventions adopted at this site.]
I take issue with much of this article (and parts of the above passage) in Essay Eight Part Two -- here, here, here, here, here and here. However, Weston signally fails to explain how the 'contradictions' in the ellipse passage under discussion are 'resolved' or how those Engels thought he saw in motion are, either! He draws a distinction between what he calls "real contradictions" and those he calls "apparent", but we aren't really told how to tell the two apart. [I will say more about that in the next re-write of Essay Eleven Part One.] In what follows I will ignore the second type of 'contradiction' since Weston claims the sort involved in elliptical motion are 'real' and that must also rope in those DM-fans see in motion, too.
As far as the 'resolution' of 'real contradiction's is concerned, Weston says this:
"Marx's conception of the development of real contradictions appears to involve at least three features: (1) becoming simpler, (2) becoming more apparent, and (3) becoming sharper, more intense, or being 'driven to a peak'." [Ibid., p.23.]
If readers check the above long quotation (or the original article, if they feel I have left anything relevant out!), they will see Weston fails to show how these three conditions apply to elliptical motion, and he doesn't even try to show how they help us understand how Engels's 'contradiction' (revealed by moving bodies in general) can be "solved"/'resolved'. He certainly considers attempts made by others to explain this conundrum (whose ideas he rejects on what appear to be sound textual and interpretative grounds), but he nowhere tells us what his answer is. The closest he came was in this part of the above passage:
"First let us notice that there are two contradictions -- or perhaps types of contradiction -- in the ellipse passage, those involving the circulation of commodities and those realised in elliptical motion. Marx made clear that contradictions between use-value and exchange-value, or between commodities and money, are capable of becoming very intense and causing crises. Marx may also have had this view of planetary motion. It was a widely circulated view in nineteenth-century England that the solar system would eventually run down. Earth's orbit around the Sun, for example, might eventually decay and lead to the end of its (nearly) elliptical orbit. A comment by Marx in Capital, Volume 1, shows that he was aware of this idea. Engels actually held this view a few years later, claiming that the Earth would 'circle in constantly narrower orbits' around a 'dying Sun' and finally fall into it. If Marx agreed with this -- and we do not know that he did -- it would be quite consistent with the ellipse passage to conclude that both the contradictions in the circulation of commodities and in elliptical motion, while not necessarily becoming more intense or driving toward resolution at every moment, would eventually do so. The ellipse passage says nothing about decay or intensification, and Marx is silent on whether or how the contradiction in elliptical motion might end. The conclusions of Horstman and Hu are supported by the ellipse passage only if this silence could be taken as evidence of Marx's view that intensification or driving toward resolution would not happen in an actual system undergoing elliptical motion. I think that Marx's silence on this point does not constitute such evidence, and examining Marx's reasons for introducing the topic of elliptical motion helps show this...." [Ibid., pp.30-31. Bold emphasis alone added.]
Is Weston saying that the elliptical 'contradiction' will be 'resolved' when all such motion dies away as the solar system decays and then falls apart? He appears to be also appears not to be -- which somehow seems a fitting position to adopt in an article about contradiction.
Of course, all of Weston's problems would disappear if he acknowledged that Marx had waved 'goodbye' to that bumbling mystic, Hegel, and had abandoned him root-and-branch, when he came to publish the second edition of Das Kapital, as I have established in Essay Nine Part One, here and here. Again, I have dealt with other things Weston has to say in the above article here, here, here, here, here and here. [See also Note 4a.]
Finally, I have shown that the rather odd phase, "real contradiction" -- which grew out of some shaky reasoning Kant came out with -- also makes zero sense, in Appendix A to Essay Eight Part Two. Readers are directed there for more details.
So, we still don't know how 'contradictions', supposedly revealed by all moving bodies, can be "solved", let alone 'resolved'!
No big surprise, there then!
Finally, what Weston has to say flies in the face of Engels's thoughts on the matter, quoted earlier:
"If one does not loiter here needlessly, but presses on farther into the immense building, one finds innumerable treasures which today still possess undiminished value. With all philosophers it is precisely the 'system' which is perishable; and for the simple reason that it springs from an imperishable desire of the human mind -- the desire to overcome all contradictions. But if all contradictions are once and for all disposed of, we shall have arrived at so-called absolute truth -- world history will be at an end. And yet it has to continue, although there is nothing left for it to do -- hence, a new, insoluble contradiction. As soon as we have once realized -- and in the long run no one has helped us to realize it more than Hegel himself -- that the task of philosophy thus stated means nothing but the task that a single philosopher should accomplish that which can only be accomplished by the entire human race in its progressive development -- as soon as we realize that, there is an end to all philosophy in the hitherto accepted sense of the word. One leaves alone 'absolute truth', which is unattainable along this path or by any single individual; instead, one pursues attainable relative truths along the path of the positive sciences, and the summation of their results by means of dialectical thinking. At any rate, with Hegel philosophy comes to an end; on the one hand, because in his system he summed up its whole development in the most splendid fashion; and on the other hand, because, even though unconsciously, he showed us the way out of the labyrinth of systems to real positive knowledge of the world." [Engels (1888), p.590. Spelling modified to agree with UK English; quotation marks altered to conform with the conventions adopted at this site. Minor typo corrected.]
As pointed out in the main body of this Essay:
The above passage appeared in published work, so it represents Engels's more considered thoughts. Here he argues that "if all contradictions are once and for all disposed of, we shall have arrived at so-called absolute truth...". That would appear to mean contradictions aren't 'objective' or "real" features of the world but are the product of our limited knowledge. That is, they are only "apparent". Even though 'absolute truth' will never be attained, the fact that there is such a thing as, or there is a possible state of knowledge that can be described as, "absolute truth" implies contradictions aren't really real but are a consequence of humanity's imperfect knowledge. Why else would they disappear? If they were 'objective' features of 'reality', they would still exist even if humanity attained 'absolute truth'. Engels wouldn't have surmised that "all contradictions" could be "once and for all disposed of" if contradictions were 'objective'. That is because only 'subjective' or 'apparent' contradictions (those that are a result perhaps of limited knowledge) can be "disposed of". Engels pointedly speaks about "all contradictions", which must include those supposedly involved in motion. This further implies that the more we know the fewer contradictions we should observe in nature and society, or the fewer we should assert actually exist.
Plainly, this in turn implies that motion could one day come to a halt, all contradictions having been "solved"/"disposed of"! Indeed, if contradictions actually 'cause' motion (or they are a consequence of it), then their complete resolution/disposal should freeze nature in its entirety. On the other hand, maybe motion will just stop being (or appearing to be) contradictory and will simply carry on as normal? Or, does this mean that nature will simply slow down as it is better understood (i.e., if what we know about motion and change becomes less and less partial/relative and hence less and less 'contradictory-looking')?
Who can say? Certainly not DM-fans. In the 150 or so years since Engels wrote these enigmatic words they have been more content merely to regurgitate them than they have been concerned to raise, let alone consider or attempt to answer, these glaringly obvious questions.
Admittedly, DM-theorists distinguish between subjective and objective dialectics -- the former relating to our (perhaps decreasingly) partial grasp of the 'nature of reality', the latter referring to processes in the 'objective world' independent of our will or our knowledge. Even so, it is still unclear how that helps answer the above questions. If the human mind "solves" the contradictions involved in motion, wouldn't that mean things just stop moving? Or wouldn't it confirm the suspicion that movement only seems to be contradictory because of the partial nature of human knowledge, implying that it isn't really contradictory? Clearly, that is because these 'subjective contradictions' ought to disappear as knowledge grows, which in turn means that (in the limit) 'reality' can't be 'contradictory', after all. In that case, it is only our 'one-sided'/'partial' knowledge of nature that fools us into concluding otherwise!
As should now seem obvious, this implies 'objective reality' is now actually contradiction-free, which it must be if knowledge is only slowly catching up with that fact.
And those comments also apply to Weston's attempt to saddle Marx with this ridiculous theory.
Several of Marx and Engels's works listed below have been linked to the Marxist Internet Archive, but since Lawrence & Wishart threatened legal action over copyright infringement many no longer work.
However, all of their work can now be accessed here.
Adamson, G. (2002), Philosophy In The Age Of Science And Capital (Continuum).
Alexander, A. (2016), Infinitesimal. How A Dangerous Mathematical Theory Shaped The Modern World (One World Books).
Angel, L. (2002), 'Zeno's Arrow, Newton's Mechanics And Bell's Inequalities', British Journal for the Philosophy of Science 53, 2, pp.161-82.
Arbesman, S. (2013), The Half-Life Of Facts. Why Everything We Know Has An Expiration Date (Penguin Books).
Aristotle, (1995a), The Complete Works Of Aristotle. Volume One, edited by J. Barnes (Princeton University Press).
--------, (1995b), Physics, translated by R. P. Hardie and R. K. Gaye, in Aristotle (1995a), pp.315-446.
Bacon, F. (2001), The Advancement Of Learning, edited by G. W. Kitchin (Paul Dry Books).
Berto, F. (2007), How To Sell A Contradiction. The Logic And Metaphysics Of Inconsistency (College Publications).
Béziau, J-Y., Carnielli, W., and Gabbay, D. (2007) (eds.), Studies In Logic Volume Nine: Handbook Of Paraconsistency (College Publications).
Black, M. (1954a), Problems Of Analysis (Routledge).
--------, (1954b), 'Achilles And The Tortoise', in Black (1954a), pp.95-108; reprinted in Salmon (1970), pp.67-81.
--------, (1954c), 'Is Achilles Still Running?', in Black (1954a), pp.109-26.
--------, (1954d), 'The Paradox Of The Arrow', in Black (1954a), pp.127-47.
--------, (1974), 'A Comment On Professor Somerville's Paper', in Somerville and Parsons (1974), pp.75-77.
Blay, M. (1998), Reasoning With The Infinite (Chicago University Press).
Boer, R. (2013), Criticism Of The Earth: On Marx, Engels And Theology (Haymarket Books).
Boylan, P. (1922), Thoth. The Hermes Of Egypt (Oxford University Press).
Burger, A., Cohen, H., and DeGrood, D. (1980) (eds.), Marxism, Science, And The Movement Of History (De Grüner).
Button, G., Coulter, J., Lee, J., and Sharrock, W. (1995), Computers, Minds And Conduct (Polity Press).
Carver, T. (1989), Friedrich Engels. His Life And Thought (Macmillan).
Cerbone, D. (2000), 'How To Do Things With Wood: Wittgenstein, Frege And The Problem Of Illogical Thought', in Crary and Read (2000), pp.293-314.
Cohen, H. (1980), 'Countering The Revisionism Of Marxist-Leninist Philosophy' in Burger et al (1980), pp.105-35.
Conant, J. (1991), 'The Search For Logically Alien Thought: Descartes, Kant, Frege And The Tractatus', Philosophical Topics 20, pp.115-80. [A PDF of this paper is available here.]
Cornforth, F. (1976), Materialism And The Dialectical Method (Lawrence & Wishart, 5th ed.). [A PDF of the 2015 reprint of this book (which appears to be slightly different from the 1976 edition used in this Essay) is available here. A PDF of the 4th edition is also available here.]
Crary, A., and Read, R. (2000) (eds.), The New Wittgenstein (Routledge).
Davidson, D. (2001), Inquiries Into Truth And Interpretation (Oxford University Press, 2nd ed.).
Engels, F. (1888), Ludwig Feuerbach And The End Of Classical German Philosophy, reprinted in Marx and Engels (1968), pp.584-622.
--------, (1954), Dialectics Of Nature (Progress Publishers).
--------, (1976), Anti-Dühring (Foreign Languages Press).
Faivre, A. (1995), The Eternal Hermes. From Greek God To Alchemical Magus (Phanes Press).
Field, H. (2008), Saving Truth From Paradox (Oxford University Press).
Flew, A. (1963) (ed.), Logic And Language. First Series (Blackwell).
Forster, M. (1998), 'On The Very Idea Of Denying The Existence Of Radically Different Conceptual Schemes', Inquiry 41, pp.133-85.
Fowden, G. (1993), The Egyptian Hermes. A Historical Approach To The Late Pagan Mind (Princeton University Press, 2nd ed.).
Gallois, A. (2003), Occasions Of Identity. The Metaphysics Of Persistence, Change, And Sameness (Oxford University Press).
Garson, J. (2023), 'Modal Logic', Stanford Encyclopedia of Philosophy, edited by Edward N. Zalta (Spring 2023 Edition).
Gefter, A. (2013), 'The Infinity Illusion', New Scientist 219, 2930, 17/08/2013, pp.32-35.
Glock, H-J. (1996), A Wittgenstein Dictionary (Blackwell).
Goldstein, L. (1992), 'Smooth And Rough Logic', Philosophical Investigations 15, pp.93-110.
--------, (2004), 'The Barber, Russell's Paradox, Catch-22, God And More: A Defence Of A Wittgensteinian Conception Of Contradiction', in Priest et al (2004), pp.295-313.
Graham, L. (1971), Science And Philosophy In The Soviet Union (Allen Lane).
Green, J. (2008), Engels. A Revolutionary Life (Artery Publications).
Grünbaum, A. (1967), Modern Science And Zeno's Paradoxes (Wesleyan University Press).
Havelock, E. (1982), The Literate Revolution In Greece And Its Cultural Consequences (Princeton University Press).
--------, (1983), 'The Linguistic Task Of The Presocratics', in Robb (1982), pp.7-82.
Hawley, K. (2004), How Things Persist (Oxford University Press).
--------, (2020), 'Temporal Parts', Stanford Encyclopedia of Philosophy, edited by Edward N. Zalta (Spring 2023 Edition).
Hegel, G. (1975), Logic, translated by William Wallace (Oxford University Press, 3rd ed.).
--------, (1995), Lectures On The History Of Philosophy Volume One: Greek Philosophy To Plato (University of Nebraska Press).
--------, (1999), Science Of Logic, translated by A. V. Miller (Humanity Books).
Hessayon, A., and Apetrei, S. (2014) (eds.), An Introduction To Jacob Boehme: Four Centuries Of Thought And Reception (Routledge).
Huggett, N. (2018), 'Zeno's Paradoxes', Stanford Encyclopedia of Philosophy, edited by Edward N. Zalta (Winter 2019 Edition).
Huggett, N., Hoefer, C., and Read, J. (2021), 'Absolute And Relational Space And Motion: Post-Newtonian Theories', Stanford Encyclopedia of Philosophy, edited by Edward N. Zalta (Summer 2023 Edition).
Hunt, T. (2009), The Frock-Coated Communist. The Revolutionary Life Of Friedrich Engels (Allen Lane).
Hunter, G. (1996), Metalogic. An Introduction To The Metatheory Of Standard First Order Logic (University of California Press, corrected edition).
Inwood, M. (2002), Hegel (Routledge).
Joravsky, D. (1961), Soviet Marxism And Natural Science 1917-1932 (Routledge).
Krementsov, N. (1997), Stalinist Science (Princeton University Press).
Kuhlmann, M. (2020), 'Quantum Field Theory', Stanford Encyclopedia of Philosophy, edited by Edward N. Zalta (Summer 2023 Edition).
Kuusela, O., and McGinn, M. (2011) (eds.), The Oxford Handbook Of Wittgenstein (Oxford University Press).
Lavine, S. (1998), Understanding The Infinite (Harvard University Press).
Leibniz, G. (2001), The Labyrinth Of The Continuum. Writings On The Continuum Problem, 1672-1686, edited and translated by Richard Arthur (Yale University Press).
Lenin, V. (1921), 'Once Again On The Trade Unions, The Current Situation And The Mistakes Of Comrades Trotsky And Bukharin', reprinted in Lenin (1980), pp.70-106.
--------, (1961), Philosophical Notebooks, Collected Works Volume 38 (Progress Publishers).
--------, (1972), Materialism And Empirio-Criticism (Foreign Languages Press).
--------, (1980), On The Question Of Dialectics (Progress Publishers).
Macdonald, M. (1963), 'The Philosopher's Use Of Analogy', in Flew (1963), pp.80-100.
Magee, G. (2008), Hegel And The Hermetic Tradition (Cornell University Press).
Mao Tse-Tung, (1961a), Selected Works Of Mao Tse-Tung, Volume One (Foreign Languages Press).
--------, (1961b), 'On Practice', in Mao (1964a), pp.295-309.
Marcuse, H. (1968), One Dimensional Man (Abacus Books).
--------, (1973), Reason And Revolution (Routledge).
Marquit, E. (1978), 'Dialectics Of Motion In Discrete And Continuous Spaces', Science and Society 42, pp.410-25.
--------, (1982), 'Contradictions In Dialectics And Formal Logic', in Marquit, Moran and Truitt (1982), pp.67-83.
Marquit, E., Moran, P., and Truitt, W. (1982) (eds.), Dialectical Contradictions And Contemporary Marxist Discussions, Studies In Marxism Volume 10 (Marxist Educational Press).
Martin, L. (2014), 'Jakob Boehme And the Anthropology Of German Pietism', in Hessayon and Apetrei (2014), pp.120-41.
Marx, K. (1968), The Eighteenth Brumaire Of Louis Bonaparte, in Marx and Engels (1968), pp.94-179.
--------, (1975a), Early Writings (Penguin Books).
--------, (1975b), Economical And Philosophical Manuscripts, in Marx (1975a), pp.279-400.
--------, (1975c), A Contribution To The Critique Of Hegel's Philosophy Of Right, in Marx (1975a), pp.243-57.
Marx, K., and Engels, F. (1848), Manifesto Of The Communist Party, in Marx and Engels (1968), pp.31-63.
--------, (1968), Selected Works In One Volume (Lawrence & Wishart).
--------, (1970), The German Ideology, Students Edition, edited by Chris Arthur (Lawrence & Wishart).
--------, (1975a), Selected Correspondence (Progress Publishers, 3rd ed.).
--------, (1975b), MECW Volume 2 (Lawrence & Wishart).
--------, (1987), MECW Volume 25 (Lawrence & Wishart).
Mason, P. (2012), Science, Marxism, And The Big Bang. A Critical Review Of 'Reason In Revolt' (Socialist Publications, 3rd ed.).
Mazur, J. (2007), The Motion Paradox. The 2,500 Year-Old Puzzle Behind The Mysteries Of Time And Space (Dutton).
Mcdonough, J. (2024), 'Leibniz's Philosophy Of Physics', Stanford Encyclopedia of Philosophy, edited by Edward N. Zalta (Fall 2024 Edition).
Miguens, S. (2019) (ed.) The Logical Alien: Conant And His Critics (Harvard University Press).
Moore, A. (2001), The Infinite (Routledge, 2nd ed.).
--------, (2011), 'Wittgenstein And Infinity', in Kuusela and McGinn (2001), pp.105-21.
Newton-Smith, W. (1980), The Structure Of Time (Routledge).
Norton, J, (2023), 'The Hole Argument', Stanford Encyclopedia of Philosophy, edited by Edward N. Zalta (Summer 2023 Edition).
Novack, G. (1965), The Origins Of Materialism (Pathfinder Press).
--------, (1971), An Introduction To The Logic Of Marxism (Pathfinder Press, 5th ed.).
Phillips, I. (2011), 'Indiscriminability And Experience Of Change', Philosophical Quarterly 61, 245, pp.808-27.
Plato (1997a), Plato. Complete Works, edited by John M. Cooper (Hackett Publishing).
--------, (1997b), Parmenides, translated by M. Gill and P. Ryan, in Plato (1997a), pp.359-397.
Priest, G. (2006), In Contradiction (Oxford University Press, 2nd ed.).
Priest, G., Beall, J., and Armour-Garb, B. (2004) (eds.), The Law Of Non-Contradiction. New Philosophical Essays (Oxford University Press).
Read, R. (2007), Applying Wittgenstein, edited by Laura Cook (Continuum Books).
Rees, J. (1998), The Algebra Of Revolution (Routledge). [This links to a PDF.]
Robb, K. (1983) (ed.), Language And Thought In Early Greek Philosophy (Monist Library of Philosophy).
Russell, B. (1937), The Principles Of Mathematics (George Allen & Unwin, 2nd ed.). [This links to a PDF of the 2010 Routledge Edition.]
Rynasiewicz, R. (2011), 'Newton's Views On Space, Time, And Motion', Stanford Encyclopedia of Philosophy, edited by Edward N. Zalta (Spring 2022 Edition).
Salmon, W. (1970) (ed.), Zeno's Paradoxes (Bobbs-Merrill).
Sanford, D. (2005), 'Distinctness And Non-Identity', Analysis 65, 4, pp.269-74.
Savickey, B. (1999), Wittgenstein's Art Of Investigation (Routledge).
Shanker, S. (1998), Wittgenstein's Remarks On The Foundations Of Artificial Intelligence (Routledge).
Slater, H. (2002), Logic Reformed (Peter Lang).
--------, (2007a), 'Dialetheias Are Mental Confusions', reprinted in Slater (2007b), pp.233-46. [This can also be found in Béziau, Carnielli and Gabbay (2007), pp.457-66.]
--------, (2007b), The De-Mathematisation Of Logic (Polimetrica).
Solnit, R. (2004), Motion Studies: Time, Space And Eadweard Muybridge (Bloomsbury).
Somerville, J. (1968), 'Ontology, Logic, And Dialectical Materialism', International Philosophical Quarterly 8, pp.113-24; reprinted in Somerville and Parsons (1974), pp.57-70.
Somerville, J., and Parsons, H. (1974) (eds.), Dialogues On The Philosophy Of Marxism. From The Proceedings Of The Society For The Philosophical Study Of Dialectical Materialism (Greenwood Press).
Sorensen, R. (2005), A Brief History Of The Paradox. Philosophy And The Labyrinths Of the Mind (Oxford University Press).
Strawson, P. (1959), Individuals. An Essay In Descriptive Metaphysics (Methuen).
Suraya, S. (2019), 'The Causal Set Approach To Quantum Gravity', Living Reviews in Relativity 22, 5.
Thalheimer, A. (1936), Introduction To Dialectical Materialism. The Marxist World-View (Covici Friede Publishers).
Thomas, K. (1973), Religion And The Decline Of Magic (Penguin Books).
Tressell, R. (1965), Ragged Trousered Philanthropists (Granada). [This links to a PDF.]
Trotsky, L. (1971), In Defense Of Marxism (New Park Publications).
Vucinich, A. (1980), 'Soviet Physicists And Philosophers In The 1930s: Dynamics Of A Conflict', Isis 71, pp.236-50.
--------, (2001), Einstein And Soviet Ideology (Stanford University Press).
Waismann, F. (1979) (ed.), Ludwig Wittgenstein And The Vienna Circle (Blackwell).
Weston, T. (2012), 'Marx On The Dialectics Of Elliptical Motion', Historical Materialism 20, 4, pp.3-38. [This links to a PDF.]
Wetter, G. (1958), Dialectical Materialism (Routledge).
Wilson, C. (1989), Leibniz's Metaphysics (Manchester University Press).
Wittgenstein, L. (2013), The Big Typescript: TS 213, edited and translated by C. G. Luckhardt and M. A. E. Aue (Blackwell).
Woods, A., and Grant, T. (1995/2007), Reason In Revolt. Marxism And Modern Science (Wellred Publications, 1st ed. 1997; 2nd ed. 2007). [The on-line version now appears to be the second edition.]
Word Count: 96,180
Latest Update: 01/09/23
© Rosa Lichtenstein 2024
Hits Since March 2007: